
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Chem. , 25 April 2023
Sec. Analytical Chemistry
Volume 11 - 2023 | https://doi.org/10.3389/fchem.2023.1189308
The speciation of epinephrine (Eph−) in the presence of alginate (Alg2-) and two biological and environmental relevant metal cations (Cu2+, UO22+) was investigated at T = 298.15K, I = 0.15–1.00 mol dm−3 in NaCl(aq). The formation of binary and ternary complexes was evaluated and, since epinephrine can behave as a zwitterion, the Eph−/Alg2- interaction was studied by means of DOSY NMR. The dependence of the equilibrium constants on ionic strength was studied using an extended Debye-Hückel type equation and the SIT approach. The effect of temperature was investigated by means of isoperibolic titration calorimetry: the entropic contribution was the driving force for the Cu2+/Eph− complexes formation. The sequestering ability of Eph− and Alg2- on Cu2+, evaluated by the pL0.5 calculation, increased with pH and ionic strength. The determination of pM parameter showed that Eph− had a higher Cu2+ affinity with respect to Alg2-. The formation of Eph−/Alg2- species was also investigated by UV-Vis spectrophotometry and 1H NMR measurements. The ternary Cu2+/Eph−/Alg2- and Cu2+/UO22+/Eph− interactions were also studied. The “extra-stability” calculated for the mixed ternary species confirmed that their formation was thermodynamically favorable.
The onset of more frequent disorders owing to the accumulation of metal cations in the human body or in natural fluids is encouraging the scientific community to look at new chelators (Raymond and Carrano, 1979; Schiewer and Volesky, 1996; Schneider and Rubio, 1999; Barbero et al., 2013; Berto et al., 2018; Irto et al., 2018; Santander et al., 2021), which alone, or in combined effect together with other ligands, could efficiently sequester metal cations without involving drawbacks such as toxicity, economic inaccessibility, and absence of affinity towards biological membranes (Irto et al., 2021).
In recent decades, many molecules (such as amino acids, hormones, and carboxylic acids) participating in normal human body functions or compounds extracted by natural matrices (Grgas-Kužnar et al., 1974; Gergely et al., 1981; Kiss and Gergely, 1983; Schiewer and Volesky, 1996; Gerard and Hanane, 1997; Schneider and Rubio, 1999; Davis et al., 2003; Cigala et al., 2015; Piperea-Şianu et al., 2015; Idota et al., 2016; Vione et al., 2016) have been tested as potential metal chelators for biological or environmental applications.
Among them, epinephrine (Figure 1A), also known as adrenaline, is a pharmacologically active substance belonging to the catecholamine family. Its structure features an aromatic ring substituted with two -OH in ortho positions and an alkyl side chain with hydroxyl and secondary amino groups as substituents.
When the human body is in a situation of severe stress, the medullary adrenal gland, stimulated by the autonomic nervous system, releases epinephrine into the bloodstream in a three-step process. In particular, the hypothalamus produces hormones stimulating the pituitary gland. This latter in turn makes corticotrophin hormones that encourage the adrenal glands for the production of corticosteroid hormones and neurotransmitters like adrenaline (Barrington, 2000). The main functions of epinephrine are: increasing heart rate, the facilitation of blood flow to the muscles and brain, the relaxation of smooth muscle, help with the conversion of glycogen to glucose in the liver, raising the level of sugar in the blood, the acceleration of breathing, the modulation of memory consolidation, and employment as a haemostatic agent to prolong the action of local anaesthetics in postoperative treatments (Cahill and Alkire, 2003; Flint et al., 2007). Furthermore, it is used in the medical field for the treatment of glaucoma, asthma, and cardiac arrest.
On the other hand, among natural organic substances in the aquatic ecosystems, many biopolymers, such as cellulose, lignin, chitin and pectin derivatives, alginates, and humic substances are known to strongly sequester metal cations for possible applications in wastewater treatment and heavy metals removal from contaminated sites (Pandey et al., 2000; Santander et al., 2021), and also as possible carriers of molecules of biological interest.
Alginate (Figure 1B) is a polysaccharide derived from alginic acid, a copolymer of β-D-mannuronic and α-L-guluronic acids (Nussinovitch, 1997; Lee and Mooney, 2012) residues linked to each other by means of a 1,4 bond (De Stefano et al., 2005). It is mainly extracted from brown seaweed, such as the L. hyperborea in the North Atlantic coastal regions, the A. nodosum from North Europe and Canada, and the M. pyrifera from the western coasts of America (Nussinovitch, 1997). Alginate is usually found in the sodium(I), calcium(II), or magnesium(II) form: the presence of these metal cations makes it more stable than alginic acid, especially when alginate is interacting with bivalent metals (Pereira and Cotas, 2019).
Features such as high viscosity, gelling properties, and high stability make alginate an important industrial polysaccharide. Alginate has several applications in the pharmaceutical industry such as for the formation of gels, as stabilizing agents, and for localized drug delivery. The use of alginate hydrogels for tissue drug delivery is widely used nowadays, and it has often been encapsulated with several drugs to enhance its wound healing properties.
It is used as a 3D culture matrix because it provides support for the integration of cells and acts as a platform for cellular growth.
Unfortunately, the data reported in the literature on the stability of metal/alginate complexes are not always homogeneous and sometimes not in agreement with each other. These discrepancies could be possibly due to the different experimental conditions (such as pH range, ionic strengths, supporting electrolyte, and metal-to-ligand concentration ratios) used by the authors, or to the electrostatic effects owing to the nature and molecular weight of the polyelectrolyte and to the various thermodynamic models used to describe the alginate acid-base behavior (Mohammed et al., 2022).
The knowledge of a ligand’s thermodynamic properties (equilibrium constants, parameters for the dependence on I/mol dm−3, and temperature T/K) and of the different chemical forms in which it is distributed, namely the speciation (Templeton et al., 2000), at experimental conditions simulating those of real multicomponent systems (biological, natural fluids), are very important tools for gaining information about ligand bioavailability, toxicity, and environmental impact and for possible applications in real biological, environmental, and pharmaceutical cases studies.
In this light, this contribution reports the results obtained by performing a multi-technique (ISE-[H+] potentiometry, UV-Vis spectrophotometry, 1H NMR, isoperibolic calorimetry, and thermogravimetry) speciation study on the binary and ternary interactions of epinephrine (Eph−) with alginate (Alg2-) and two metal cations of biological and environmental relevance, such as Cu2+ and UO22+. Since adrenaline can behave as a zwitterion in aqueous solution (Antikainen and Witikainen, 1973), the possible ligand-ligand interaction between Eph− and Alg2- was also studied by ISE-[H+] potentiometry, UV-Vis spectrophotometry, and 1H NMR.
The investigations were carried out at T = 298.15K in NaCl aqueous solution, the main inorganic component of several biological (Buffle, 1988; Millero, 2001) and natural (Lentner, 1981) fluids, and at different ionic strengths. The dependence on ionic strength of the equilibrium constants of the Cu2+/Eph− and Cu2+/Alg2- species was studied using an extended Debye-Hückel type equation and, for the first system, also with the Specific ion Interaction Theory (SIT) approach (Biederman, 1975; Biederman, 1986; Irto et al., 2019a). The effect of temperature on Cu2+/Eph− speciation was investigated by isoperibolic titration calorimetry, allowing the determination of enthalpy changes values for the formation of some complexes. Thermogravimetry (TGA) measurements were carried out on solid samples collected at the end of potentiometric titrations to determine the precipitate stoichiometry and gaining information on the thermal stability of the systems.
The Cu2+ sequestering ability and affinity of epinephrine and alginate were evaluated by the determination of the pL0.5 (Crea et al., 2014) and pM (Raymond and Carrano, 1979) parameters at different ionic strength and pH conditions.
The ternary Cu2+/Eph−/Alg2- and Cu2+/UO22+/Eph− interactions were also investigated since the knowledge of the possible formation of mixed species is fundamental for the treatment of many real biological and environmental problems and because in many cases the formation of these mixed species increases the solubility and the availability of the metal cations. Furthermore, the extra-stability (Beck and Nagypál, 1991) of selected ternary complexes was determined to evaluate if the formation of mixed species could be thermodynamically favored with respect to the corresponding polynuclear binary complexes.
Sodium hydroxide and hydrochloric acid solutions were prepared using Riedel-de Häen (Seelze, Germany) concentrated ampoules and standardized by means of potassium hydrogen phthalate and sodium carbonate, respectively. The base solutions were preserved from atmospheric CO2 effects by employing soda lime traps. Fresh adrenaline solutions were prepared by weighing the (-)-epinephrine solid product, purchased by Sigma-Aldrich (Milan, Italy), without any purification. The ligand purity was tested by potentiometry by means of alkalimetric titrations and the results showed it to be >99%. Alginic acid sodium salt from brown algae (Sigma-Aldrich, Milan, Italy) was weighed for the preparation of alginate solutions. CuCl2·2H2O and Cu(NO3)2 hydrate salts, both purchased by Fluka (Darmstadt, Germany), were used to prepare the metal solutions. They were standardized employing EDTA standard solutions (Flaschka, 1959) and their purity was always ≥98%. UO22+ nitrate and diacetate salts (Fluka, Darmstadt, Germany) were used. The gravimetric determination of uranium after ignition to the U3O8 oxide was performed for the determination of uranyl products purity (Crea et al., 2003). The preparation of ionic medium solutions was carried out by weighing the pure sodium chloride salt purchased by Fluka (Darmstadt, Germany). The NaCl solid product was formerly dried in an oven for 2 hours at T = 383.15K. All the solutions were prepared by employing analytical grade water (R = 18 MΩ cm−1), reagents of the highest available purity, and grade A glassware.
Potentiometric measurements were carried out using a Metrohm (Herisau, Switzerland) Titrando 809 model and a potentiometer with a Ross type 8102 combined glass electrode (Thermo-Orion, Waltham, MA United States) plugged to an automatic burette. The mentioned apparatus was connected to a PC and automatic titrations were performed employing a Metrohm TiAMO 2.5 software for checking for titrant delivery, e.m.f. stability and data acquisition. The estimated accuracy values for e.m.f. (electromotive force) and titrant volume readings were ±0.15 mV and ±0.003 cm3, respectively. The experiments were carried out in thermostated cells under magnetic stirring. Purified presaturated N2(g) was bubbled into the measurements solutions to exclude O2(g) and CO2(g) presence inside.
The potentiometric measurements were carried out at T = 298.15K, at different metals and ligands concentrations, metal:ligand molar ratios, and ionic strengths, as reported in Table 1.
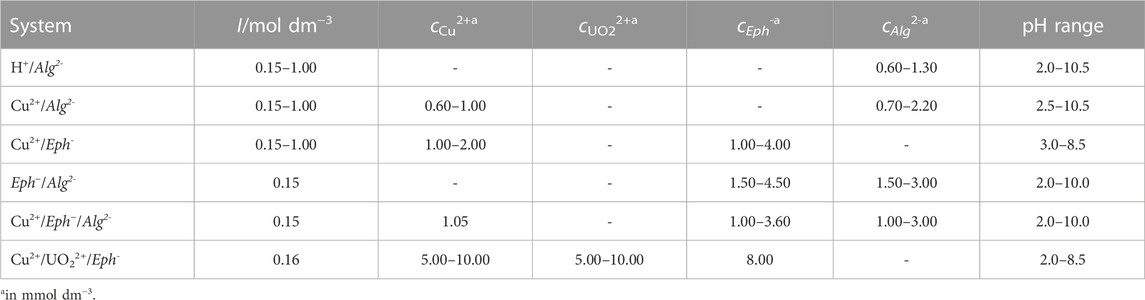
TABLE 1. Experimental details of the potentiometric investigations performed at T = 298.15K in NaCl(aq).
In the case of the H+/Alg2-, Cu2+/Alg2- and Cu2+/Eph−/Alg2- systems, acidimetric titrations were performed using titrant solutions of standard hydrochloric acid. On the contrary, for the other systems, alkalimetric measurements with standard sodium hydroxide solutions were carried out.
Spectrophotometric experiments were carried out by means of a Varian (Agilent Scientific Instruments, CA, United States) Cary 50 UV–Vis spectrophotometer, equipped with an optic fiber probe (path length: 1 cm). A personal computer was connected to the instrument and Varian Cary WinUV software was used for the acquisition of absorbance (A) signal vs. wavelength (λ/nm). Concurrently, potentiometric data were recorded by means of a combined Ross type 8102 glass electrode (Thermo-Orion, Waltham, MA United States) connected to a potentiometer. The titrant solutions were delivered in the thermostated experiments cells, employing an automatic burette, Metrohm (Herisau, Switzerland) 665 model. The solution’s homogeneity was ensured using a stirring bar. To rule out the presence of oxygen and carbon dioxide from the measurement solutions, before starting the experiments, gaseous nitrogen was bubbled through for 5 min.
The binding ability of epinephrine towards Cu2+ was studied by titrating with standard sodium hydroxide 25 cm3 solutions containing the ligand (cEph− = 0.05–0.10 mmol dm−3), the metal cation (cCu2+ = 0.05–0.10 mmol dm−3), hydrochloric acid (cH+ = 5.00–8.00 mmol dm−3), and NaCl at I = 0.15 mol dm−3, in the pH range 3.0–11.0 and 200 ≤ λ/nm ≤ 450.
UV-Vis investigations into the Eph−/Alg2- system were performed in the absence of ionic medium at T = 298.15K and in the wavelength range 200 ≤ λ/nm ≤ 450. In these investigations, 25 cm3 of solutions containing epinephrine, alginate, or both the ligands at cEph- = cAlg2- = 0.12 mmol dm−3 were titrated with standard HCl (0.0985 mmol dm−3) or NaOH (0.0956 mmol dm−3) solutions in the pH ranges 8.7–2.0 and 8.5–10.5, respectively.
The study of the heat of the reactions involved in the Cu2+/Eph- complexation was performed using a Calorimetry Sciences Corporation (CSC, Utah, United States) calorimeter (Model 4285) equipped with a constant temperature bath (Mod. 7211). The experiments were performed by titrating a 25 cm3 solution of ligand (cEph- = 2.5–5 mmol dm−3), previously salified with sodium hydroxide, with a titrant solution of Cu(NO3)2 hydrate at cCu2+ = 0.0715 mol dm−3 in NaCl(aq) at I = 0.50 mol dm−3, delivered by means of a Hamilton syringe, model 1002TLL (Sigma Aldrich, Milan, Italy), with a 2.5 cm3 capacity. The pH range investigated was 10.0 ≥ pH ≥ 4.5. Measurements were repeated at least three times for each selected experimental condition. The dilution enthalpy was measured before each experiment. The accuracies of calorimetric apparatus and of titrant volume were ±0.008 J and 0.001 cm3, respectively. Calibration measurements were carried out by titrating a THAM (tris-(hydroxymethyl)amino-methane) buffer with hydrochloric acid. The enthalpy change values to be used in the calculations for the water ionization has been already reported in the literature (De Stefano et al., 2001).
A Perkin Elmer Pyris Diamond thermobalance was employed for the thermal analysis and the analytical data were elaborated using the version 2.6 Muse Measurement thermal analysis software (supplied by Perkin Elmer Corp.). Precipitate collected at the end of Cu2+/Eph− potentiometric measurements were filtered with 0.45 µm cellulose filters. The solids were washed with little amounts of ultrapure water and treated with small aliquots of acetone and dried under vacuum. Approximately 2–10 mg of the obtained samples was heated in platinum crucibles at the following conditions, allowing for the best possible resolution of the thermogravimetric curves: a temperature range of 293.15 ≤ T/K ≤ 1123.15, an atmosphere of gaseous mixture of nitrogen and oxygen with 80% and 20% v/v, respectively, a flow rate of 100 cm3 min−1, and a scanning rate of 283.15 K min−1.
NMR spectra were recorded at T = 298.15K in D2O on a Varian 500 MHz instrument equipped with a pulse-field gradient probe. 1,4-Dioxane (δH = 3.75 ppm) was used as an internal standard. 1H NMR spectra were recorded using solvent suppression pulse sequences (PRESAT). Diffusion-ordered NMR spectroscopy (DOSY) studies were performed using a Doneshot pulse sequence (Pelta et al., 2002), optimizing the experimental parameters according to the sample under investigation. Diffusion gradients were progressively incremented over 15 steps, varying the gradient strength from 1.8 to 50.0 gauss/cm. Sixteen transients were acquired for each increment, with a diffusion-gradient length of 2–4 ms and diffusion delays in the 50–300 m range.
For the experiments, solutions of epinephrine (cEph− = 2.29 mmol dm−3), alginate (cAlg2- = 2.60 mmol dm−3), and Eph−/Alg2- (cEph− = 2.29 mmol dm−3, cAlg2- = 2.60 mmol dm−3) were prepared in D2O without addition of NaCl. The pH of the solutions was ∼9.5.
The BSTAC computer program (De Stefano et al., 1997) was used for the determination of E0, pKw, and ja parameters, the reagents analytical concentration, and the equilibrium constants. UV-Vis spectrophotometric data were analyzed by employing HYPERQUAD 2008 (Gans et al., 1996). The least squares LIANA program (De Stefano et al., 1997) was used for the determination of Debye–Hückel and SIT parameters as well as for the calculation of the formation constants at infinite dilution. The elaboration of calorimetric data recorded by means of isoperibol titration calorimetry was performed through the ES5CM program (De Stefano et al., 1997). The calculations of the species formation percentages and the distribution diagrams were carried out using the HySS program (Alderighi et al., 1999).
An extended Debye-Hückel type equation (Eq. 1a) was employed to model the dependence on ionic strength of the stability constants of the Cu2+/Eph− and Cu2+/Alg2- species:
where logTβpqr = equilibrium constant at infinite dilution:
The Debye- Hückel term (DH), can be expressed by:
C = empirical parameter for the dependence of the formation constants on ionic strength.
Regarding the Cu2+/Eph− system, the equilibrium constants and ionic strengths were calculated on the molal (m, mol kg−1H2O) concentration scale and the Specific ion Interaction Theory (SIT) (Biederman, 1975; Biederman, 1986) equation was used. In this case, the C parameter of Eq. 1a is replaced by the ∆ε value (Eq. 2) and the interactions between opposite charge ions participating to the equilibria are considered for the calculations.
For neutral species, the SIT coefficients are expressed by means of the Setschenow equation (Setschenow, 1889) and of the km parameter related to the activity coefficient by the Eq. 3
If all the interactions between the ionic components and species are considered, it is possible to calculate the single
where k (Cu(Eph)20) is the Setschenow coefficient of the neutral species (Setschenow, 1889). If a ternary hydrolytic species is formed, the activity coefficient of water must be considered (log aw = 0.015) in the calculation of the specific ion interaction parameter, as well as the specific ion interaction parameter ε(H+, Cl−).
The acid-base properties of epinephrine has already been studied by the research group, which also studied the solubility and concentration of the ligand neutral species at different experimental conditions as well as the dependence on the ionic strength and temperature of thermodynamic parameters (Bretti et al., 2015) and commented on the literature attribution of equilibrium constants to epinephrine protonable groups (Antikainen and Witikainen, 1973).
The protonation behavior of the alginate polyelectrolyte was experimentally investigated by potentiometry at I = 0.15–1.00 mol dm−3 in NaCl(aq) and T = 298.15K.
For the data elaboration of acid-base properties and metal complexation of polyelectrolytes, different approaches have been proposed and reported in the literature (Katchalsky, 1954; Högfeldt, 1988; Högfeldt et al., 1989) because the aqueous behavior of such high molecular weight ligands depends on factors such as ionic medium, ionic strength, electrostatic charge, temperature, and dissociation degree (α), thta as well known, depend on the pH of the solution.
Among the possible approaches, classical methods such as the modified Henderson-Hasselbalch equation proposed by Katchalsky (Katchalsky and Spitnik, 1947; Katchalsky, 1954) and the three parameters of the Högfeldt model (Högfeldt, 1988; Högfeldt et al., 1989) are particularly effective and accurate for describing the acid-base polyelectrolyte behavior in aqueous solutions as a function of α (Bretti et al., 2017). Unfortunately, employing the mentioned models require many calculations, and is often not simple for inexpert researchers.
In this light, a simplified approach, named the polyprotic-like model, was proposed, and reported in the literature (Crea et al., 2010). Briefly, this method allows a polyelectrolyte, in terms of acid-base and complexing ability, to be treated as a low molecular weight ligand, considering the minimum number of protonation sites useful to describe the system, independently of the dissociation degree. The polyprotic-like model was applied to many different classes of polyelectrolytes with various chemical structures, molecular weights, and functional groups. The data were compared with those obtained using the Henderson-Hasselbalch and Högfeldt models (Crea et al., 2010) and very similar results, without a significant loss of precision, were observed among the different approaches. A much simpler procedure for calculating the equilibrium constants characterized the data elaboration using the polyprotic-like model that was, therefore, considered a valid alternative to the classical equations for polyelectrolytes.
Based on all these considerations, the treatment of experimental data on alginate acid-base properties was carried out by applying the polyprotic-like model. In particular, the polyelectrolyte was assumed to behave as a low molecular weight ligand consisting of a monomeric unit of β-D-mannuronic acid and one of α-L-guluronic acid, with a total of two protonable sites, namely two carboxylic groups, and charge z = 2-. Alginate protonation constants, as reported in Table 2, were expressed by the following stepwise (Eq. 9) and overall (Eq. 10) equilibria:
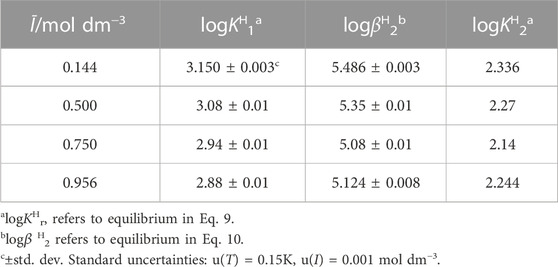
TABLE 2. Protonation constants of alginate (Alg2-) at different ionic strengths in NaCl(aq) and T = 298.15K.
As observable in Table 2, the protonation constants decrease with increasing ionic strength. Only in the case of logKH2 can a slight reversal trend be observed at I = 0.956 mol dm−3, with respect to the other experimental conditions. The effect of this variable on the protonation of alginate can be also observed in Supplementary Figure S1. The H2(Alg)0(aq) species reaches percentages of 66% and 57% at I = 0.144 and 0.956 mol dm−3, respectively, at pH ∼ 2.0. The formation of the H(Alg)- is shifted towards more acidic pH values when the ionic strength increases, achieving percentages of 57% at pH ∼ 2.8 and 51% at pH ∼ 2.6, respectively. Starting from pH ∼ 5.0, the ligand is present as a free ligand, namely Alg2-.
The results here obtained are comparable with those reported by De Stefano et al. (De Stefano et al., 2005). Authors used the same approach here employed for the determination of protonation constants, and at I = 0.50 mol dm−3 in NaNO3(aq), they calculated the following values: log KH1 = 3.135 and logKH2 = 2.581. These data are in good agreement with the experimental alginate protonation constants in Table 2, determined at the same I/mol dm−3 and T/K conditions.
The acid-base properties of Cu2+ and UO22+ were already studied at T = 298.15K and I = 0.15–1.00 mol dm−3 in NaCl(aq) (Baes and Mesmer, 1976; Gianguzza et al., 2004; Brown and Ekberg, 2016); these data are reported in Supplementary Tables S1, S2 of the Supplementary Information section.
Furthermore, since for the preparation of the dioxouranium(VI) (UO22+) standard solutions the UO2(Ac)2 salt (Ac: acetate, see Materials and Method section) was also used and this metal cation tends to form stable complexes with acetate (Crea et al., 2003), the formation constants of the UO22+/Ac− complexes were considered (see Supplementary Table S3 of the Supplementary Information section) as input in the speciation model of the UO22+/Eph− and mixed Cu2+/UO22+/Eph− mixed system.
The elaboration of the experimental data on metal-ligand systems collected at I = 0.15–1.00 mol dm−3 in NaCl(aq) and T = 298.15K using different analytical techniques led to the determination of metal/ligand complexes with different stoichiometry, owing to the various acid-base behaviors of ligands. The selection of the best speciation model was carried out applying some criteria already discussed in previous works (Irto et al., 2019b; Irto et al., 2020).
The study on the interaction of Cu2+ with epinephrine was carried out by potentiometric, UV-Vis spectrophotometric, calorimetric, and thermogravimetric techniques at I = 0.15–1.00 mol dm−3 in NaCl(aq) and T = 298.15K.
Potentiometric data were collected in the pH range 3.0–8.5 up to the formation of sparingly soluble species. During the titrations, the measurement solutions assumed an intense dark red colour, which became clearer, to a light red-orange, with increasing the pH. The UV-Vis data, recorded at lower Cu2+ and Eph− concentrations than those used for potentiometry (see Materials and Methods section), were elaborated at pH 3.0–10.5 without the observation of precipitate in the measurement solutions. The best possible speciation scheme featured five species: Cu(Eph)20(aq), Cu(Eph)2OH−, Cu2(Eph)OH2+, Cu2(Eph)3+, and Cu2(Eph)22+. The Cu(Eph)2OH− complex was not determined at I = 0.732 mol dm−3 in NaCl(aq).
The overall formation constants (Table 3) determined for these species are referred to the equilibria in Eqs 11, 12.
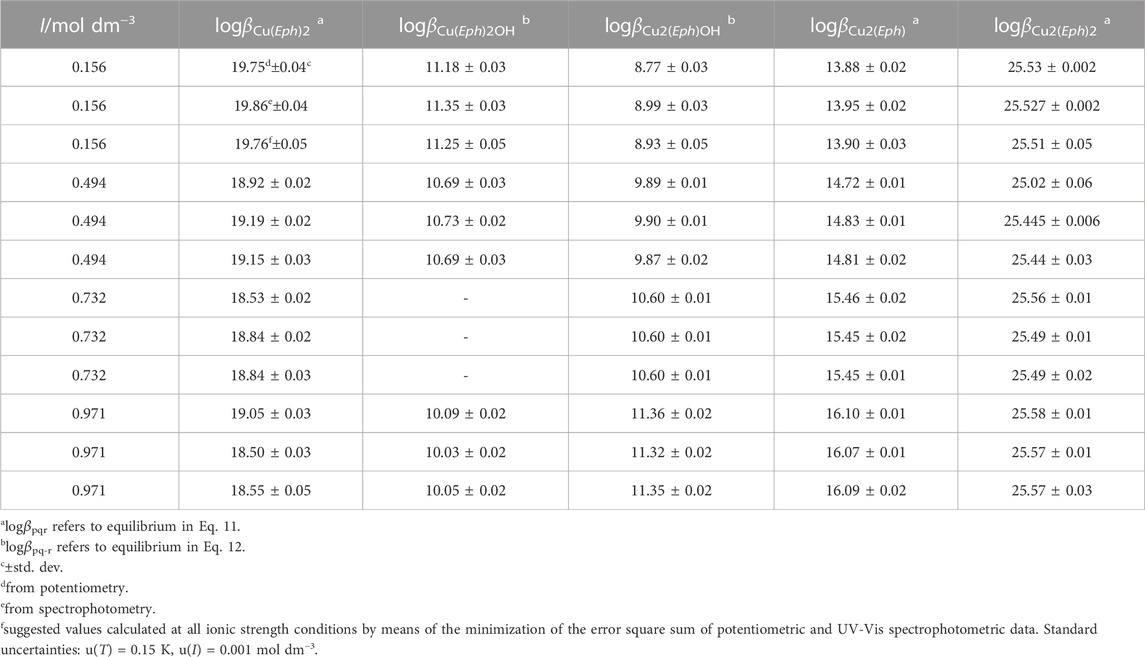
TABLE 3. Experimental and suggested overall formation constants of Cu2+/Eph− species at different ionic strengths in NaCl(aq) and T = 298.15K.
As observable in Table 3, the obtained data are in good agreement between the two analytical techniques. This allowed for the calculation of the Cu2+/Eph− species and by using the minimization of the error square sum (De Stefano et al., 1997) on the data obtained from the two techniques, it was possibile to calculate the corresponding “suggested” values.
It is evident that the Cu2+/Eph- complexes have high stability, allowing their formation also at low concentrations (i.e., from spectrophotometric data).
The possible formation of 1:2 complexes was already reported in different investigation on the Cu2+/Eph− system (Jameson and Neillie, 1965; Jameson and Neillie, 1966; Grgas-Kužnar et al., 1974; Rahaman and Korenkiewicz, 1976; Gergely et al., 1981; Kiss and Gergely, 1983; Materazzi et al., 2002; Al-Ayed et al., 2013; Krasnovskaya et al., 2020); the high complexes stability of the species can be interpreted taking into account the coordination that occurs through the phenolic groups of epinephrine rather than involving the side chain (Jameson and Neillie, 1965; Jameson and Neillie, 1966).
From the analysis of UV-Vis spectra in Figure 2 at I = 0.156 mol dm−3, it is possible to observe an absorption band at λmax = 280 nm and pH ∼ 3.1 which significantly increases in intensity with the rise in pH. Furthermore, a bathochromic shift occurs in the pH range ∼ 6.5–7.5 with λmax = 303 nm, providing possible evidence of the formation of metal-ligand species. Then, at pH > 10.5, a hypsochromic shift can be observed with λmax = 296 nm, possibly owing to epinephrine oxidation/degradation processes occurring at this experimental condition. For this reason, absorbance values recorded at more alkaline pH were not considered during data treatment.
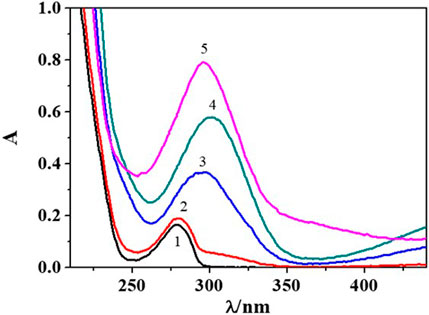
FIGURE 2. UV-Vis spectra recorded at some pH values for the Cu2+/Eph− system at I = 0.156 mol dm−3 in NaCl(aq), T = 298.15K, cCu2+ = 0.0566 mmol dm−3 e cEph- = 0.0622 mmol dm−3. 1. pH = 3.07; 2. pH = 5.26; 3. pH = 6.48; 4. pH = 7.49; 5. pH = 10.73.
The distribution of Cu2+/Eph− species can be investigated by means of the distribution diagrams in Supplementary Figure S2, drawn at I = 0.156 and 0.971 mol dm−3 using potentiometric data. Significant differences between the two ionic strengths can be observed in terms of percentages and pH of formation, in particular for the Cu2(Eph)3+, Cu2(Eph)OH2+, and Cu2(Eph)22+ species. At I = 0.156 mol dm−3, the main complex determined in the pH range of many natural waters and biological fluids (pH ∼ 5.0–8.2) are the Cu(Eph)20(aq) and Cu2(Eph)22+, while at I = 0.971 mol dm−3, the predominant species are the Cu2(Eph)OH2+ and the Cu(Eph)20(aq).
The effect of temperature on Cu2+/Eph- speciation was investigated by performing isoperibolic calorimetric titrations at T = 298.15K and I = 0.50 mol dm−3. The enthalpy change values for the protonation of Eph- (Bretti et al., 2015), hydrolysis of Cu2+ (Brown and Ekberg, 2016), and the dissociation of water (De Stefano et al., 2001) were taken from the literature.
The determination of the enthalpy change values of the Cu2+/Eph− species formation was limited by the low ligand solubility at the mentioned ionic strength condition (logS = ∼ −2.07) (Bretti et al., 2015), which did not allow the preparation of more concentrated solutions. For this reason, it was possible to experimentally calculate the enthalpy of formation of only two complexes, namely, Cu(Eph)20(aq) and Cu(Eph)2OH−. The formation of the Cu(Eph)20(aq) species was favored by an exothermic contribution (ΔHCu(Eph)2 = −35 ± 1 kJ mol−1), whilst the Cu(Eph)2OH− was favoured by an endothermic (ΔHCu(Eph)2OH = 19 ± 4 kJ mol−1) contribution (see also Figure 3).

FIGURE 3. Bar plot for the enthalpy and entropy change values of the Cu2+/Eph− system species at I = 0.50 mol dm−3 and T = 298.15 K.
The reliability of the obtained results is also supported by the low values of both the standard deviation for the global fit of experimental data (σ = 0.018) and the mean deviation of the variation of the heats of reaction (
The entropic contribution (TΔSCu(Eph)2 = 74 ± 3 kJ mol−1, TΔSCu(Eph)2OH = 80 ± 10 kJ mol−1) was the driving force for the Cu2+/Eph− complexes formation, and both the processes were spontaneous, as highlighted by the negative values of the calculated free Gibbs energy (ΔGCu(Eph)2 = −109.16 ± 0.16, ΔGCu(Eph)2OH = −60.83 ± 0.05).
In addition, the precipitates collected at the end of the potentiometric titrations were characterized by thermogravimetry (TGA) to determine the precipitate stoichiometry and gain information on the thermal stability. Three decomposition processes were observed, as reported in Figure 4.
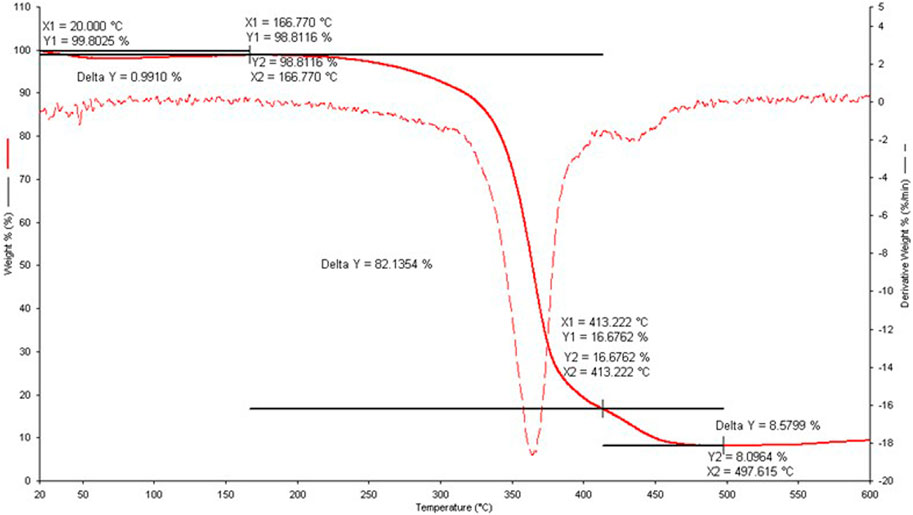
FIGURE 4. Thermogravimetric curve for the Cu2+/Eph− solid sample obtained at I = 0.156 mol dm−3, cCu2+ = 1.0 mmol dm−3 and cEph- = 4.0 mmol dm−3. Solid line: % weight loss as function of t/°C, dash line: derivative weight loss vs. t/°C.
The first decomposition process was characterized by a weight loss of 0.99%, significantly lower with respect to the two subsequent ones of 82.13% and 8.58%, respectively. For each process, the corresponding molecular weights of fragments lost were 9.81 g mol−1, 813.90 g mol−1, and 84.90 g mol−1, respectively. The residual of the decomposition process at 79.50 g mol−1 was assumed to be CuO (MW = 79.55 g mol−1). At the investigated conditions, the most probable stoichiometry for the precipitate formation was the 1:5 one, namely the Cu(Eph)5 species. This result is highly dependent on the component concentrations and molar ratios employed to prepare the samples.
The binding ability of epinephrine towards dioxouranium(VI) had been already experimentally investigated by the research group using the same experimental conditions (i.e., temperature, ionic medium, ionic strength, and component concentration) as selected for the present study (Crea et al., 2020). The speciation model featured the following metal/ligand species: UO2(Eph)+, UO2(Eph)OH0(aq); (UO2)2(Eph)22+ and (UO2)2(Eph)2(OH)20(aq). The dependence on I/mol dm−3 was modelled by means of a Debye-Hückel type equation and Specific ion Interaction Theory (SIT). By means of isoperibolic calorimetric titrations, the enthalpy change values of species formation were determined.
The elaboration of potentiometric data was performed in the pH range 3.0–10.0 due to the formation of sparingly soluble species occurring at pH ∼ 2.5–2.8. Owing to the low solubility of alginate in acid solutions, before the titration, the pH was corrected up to ∼10.5 and then the resulting solutions titrated with standard solution of HCl. The formation of precipitate was observed at pH values dependent on the experimental conditions (component concentration and ionic strength). Applying the above mentioned selection criteria, the best speciation model was obtained considering the following species: Cu(Alg)0(aq), Cu(Alg)OH−, Cu(Alg)(OH)22-, Cu(Alg)(OH)33-, and Cu(Alg)22-. The overall equilibrium constants determined for these species referred to the equilibria in Eqs. 13, 14:
In Table 4, the equilibrium constants obtained at I = 0.15–1.00 mol dm−3 in NaCl(aq) and T = 298.15K are reported; we can observe an increase of the overall stability constants (log β) with an increase in the ionic strength.
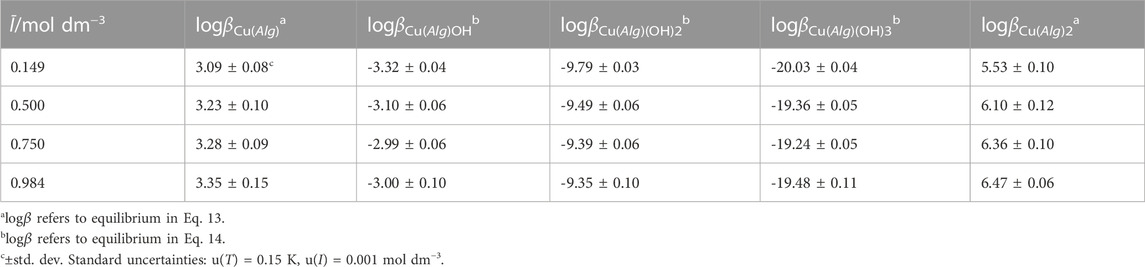
TABLE 4. Experimental overall formation constant of Cu2+/Alg2- species at different ionic strengths in NaCl(aq) and T = 298.15K.
De Stefano et al. (De Stefano et al., 2010) reported the results of a potentiometric study carried out using alginic acid and ISE-[Cu2+] and ISE-[H+] electrodes, at I = 0.098–0.727 mol dm−3 in NaNO3 ionic medium and T = 298.15K, but at different metal (cCu2+ = 0.25–0.35 mmol dm−3) and ligand (cAlg2-=4.51–4.62 mmol dm−3) concentrations, as well as pH range (4.1–4.7). The authors determined only one complex species, namely, the Cu(Alg)0(aq), whose stability constants at I = 0.10 mol dm−3 were: logβ110 = 3.626 and 3.586, respectively. Some differences can be observed among these data and the value (logβ110 = 3.09 ± 0.08) presented in Table 4 at I = 0.149 mol dm−3, possibly due to the different speciation models and experimental conditions.
The distribution of Cu2+/Alg2- species can be investigated by the analysis of distribution diagrams in Figure 5, drawn at I = 0.149 and 0.984 mol dm−3.
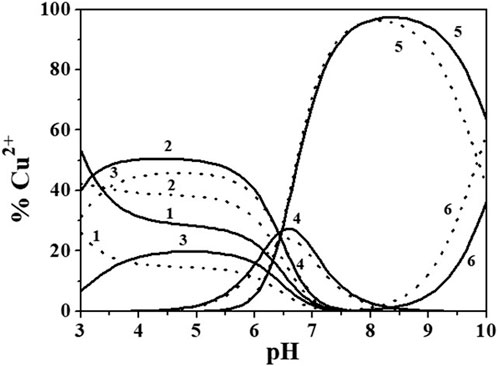
FIGURE 5. Distribution diagram of Cu2+/Alg2- species at I = 0.149 (solid line) and 0.984 (dotted line) mol dm−3, ccu2+ = 0.70 mmol dm−3, cAlg2- = 2.10 mmol dm−3. Species: 1. Free Cu2+; 2. Cu(Alg)0(aq); 3. Cu(Alg)22-; 4. Cu(Alg)OH−; 5. Cu(Alg)(OH)22−; 6. Cu(Alg)(OH)33-.
All the species reach formation percentages between 21% and 97%. The main complexes at physiological pH (pH ∼ 7.4) and at the pH of seawater (pH ∼ 8.1) are the Cu(Alg)OH− and Cu(Alg)(OH)22-.
The dependence on ionic strength of alginate protonation constants, Cu2+/Eph− and Cu2+/Alg2- complexes formation constants was modelled using an extended Debye-Hückel type equation and Specific ion Interaction Theory (SIT) approach (Biederman, 1975; Biederman, 1986). More details on these models are reported in Section 2.4. Models for ionic strength dependence. For both the binary systems, the stability constants at infinite dilution and the C empirical parameters are reported in Supplementary Tables S4–S6 together with the calculated values at I = 0.15–1.00 mol dm−3 in NaCl(aq), allowing for their prediction in experimental conditions of real systems like biological fluids or natural waters.
Applying the same extended Debye-Hückel type equation (Eq. 1a) and Section 2.4.), De Stefano et al. (De Stefano et al., 2010) determined for the Cu(Alg)0(aq) species the formation constants at infinite dilution (logTβ) and the parameter for the dependence on I/mol dm−3; the values they obtained are: logTβ = 5.05 and C = 0.69.
In the case of the Cu2+/Eph− system, the dependence of the formation constants on ionic strength was also investigated by means of the Specific ion Interaction Theory (SIT) approach, which requires the conversion of the concentrations and stability constants from the molar (mol dm−3) to the molal (mol kg−1H2O) concentration scale and the knowledge of the specific ion interaction parameters (ε) for all the ion-pairs involved in the complex formation equilibria. The values of 0.12 (Bretti et al., 2006), -0.219 (Bretti et al., 2015), and 0.08 (Grenthe and Puigdomenech, 1997) were used for the
The results of the calculation of the specific ion interaction parameter of the ionic species and of the Setschenow coefficient of the neutral one (k (Cu(Eph)20)) (Setschenow, 1889) are reported in Table 5.
Since Eph− behaves like a zwitterion and its secondary amine group is protonated up to pH∼10, we decided to investigate the possible ligand-ligand interaction with alginate, by means of potentiometric titration carried out at I = 0.146 mol dm−3 in NaCl(aq) and T = 298.15K. Tests performed at the ionic range I = 0.50–1.00 mol dm−3 displayed the formation of precipitate in the measurement solution at the starting pH of ∼10.0, not allowing us to carry out experiments at the mentioned experimental conditions. A comparison between the titration curves of the Eph−, Alg2- and Eph−/Alg2- systems at I = 0.146 mol dm−3 is reported in Supplementary Figure S3, where some differences can be observed due to the different ligands’ acid-base behaviors and the formation of Eph−/Alg2- binary species along the investigated pH range.
The experimental data collected in the pH range 2.0–10.0 were processed as already done for the other systems, and the best results were obtained for (Eph)(Alg)H2–, (Eph)(Alg)3-, (Eph)2(Alg)H22-, and (Eph)(Alg)25-. The overall stability constants were determined considering the general equilibrium in Eq. 15:
The stability constants (±std. dev.) at the indicated experimental conditions were: logβ(Eph)(Alg)H = 13.63 ± 0.05, logβ(Eph)(Alg) = 3.65 ± 0.05, logβ(Eph)2(Alg)H2 = 27.95 ± 0.02, and logβ(Eph)(Alg)2 = 6.43 ± 0.08.
Figure 6 reports a distribution diagram of the Eph−/Alg2- species at I = 0.146 mol dm−3 and T = 298.15K.

FIGURE 6. Distribution diagram of Eph-/Alg2- species at I = 0.146 mol dm−3 in NaCl(aq), T = 298.15K, cEph- = 3.00 mmol dm−3; cAlg2- = 1.50 mmol dm−3. Species: 1. H2(Alg)0(aq); 2. H(Alg)-; 3. (Alg)2-; 4. (Eph)(Alg)H2-; 5. (Eph)2(Alg)H22-; 6. (Eph)(Alg)3-; 7. (Eph)(Alg)2(5-).
From the distribution diagram in Figure 6, it is possible to observe that the interaction between the two ligands start at pH ∼ 5.5. Up to pH ∼ 5.0, alginate is partially protonated and over this pH the formation of the Eph-/Alg2- species occurs. At the experimental condition of Figure 6, the main species is the (Eph)2(Alg)H22- that reaches about 80% of formation at pH ∼ 9. The yield of formation of the other species reaches values of ∼10–20%.
To gain further information about this ligand-ligand system, UV-Vis spectrophotometric and Diffusion-Ordered NMR SpectroscopY (DOSY NMR) was carried out.
The UV-Vis spectra recorded for epinephrine showed a similar profile than the one reported in the literature at the same temperature, cEph- = 0.11 mmol dm−3 and I = 0.15 mol dm−3 in NaCl(aq) (Bretti et al., 2015). In the case of alginate, a weak absorbance (A ≤ 0.05) was observed at the selected experimental conditions.
The analysis of UV-Vis spectra of the binary Eph−/Alg2- system has been divided in two diagrams; the first one for the pH range 8.70–2.00 (Figure 7A), where an absorption band with λmax = 285 nm and a shoulder at λ = 243 nm at pH ∼ 8.70 was observed. The band features a slight hypsochromic shift at λmax = 281 nm and pH ∼ 7.9 and another one at λmax = 279 nm in the pH range ∼ 5.0–2.6 with absorbance increase. Then, at pH ∼ 2.0, the signal intensity decreases, and no other shifts are observed. The second graph, at alkaline conditions (pH range 8.50–10.50, Figure 7B), highlights a bathochromic shift and an absorbance increase. The mentioned band with λmax = 285 nm moves at λmax = 287, 295, and 298 nm at pH ∼ 9.0, 10.0, and 10.5, respectively, while the shoulder, already observed in Figure 7A, becomes a resolved band with λmax = 243 nm, increasing in intensity with pH. Furthermore, at pH ∼ 10.5, another shoulder is formed at λ = 336 nm.

FIGURE 7. UV-Vis spectra of Eph−/Alg2- system at cEph- = cAlg2- = 0.12 mmol dm−3, T = 298.15K and different pH values; (A) UV-Vis scans recorded in the pH range 8.71–2.02; (B) UV-Vis scans recorded in the pH range 8.46–10.49.
The elaboration of the UV-Vis data collected in absence of ionic medium allowed us to obtain the same speciation model determined at I = 0.146 mol dm−3. The formation constant values (±std. dev.) obtained were: logβ(Eph)(Alg)H = 13.54 ± 0.25, logβ(Eph)(Alg) = 3.834 ± 0.003, logβ(Eph)2(Alg)H2 = 28.35 ± 0.10, and logβ(Eph)(Alg)2 = 6.892 ± 0.002. Considering the different experimental conditions in term of ionic strength and ligands concentration with respect to the ones selected to perform the potentiometric measurements, the mentioned data can be considered in good accordance with the ones determined at I = 0.146 mol dm−3.
To gain additional information about the interactions between Eph– and Alg2–, the mixture was subjected to a series of NMR experiments. Simple mixing of the two compounds in D2O (T = 298.15K, pH ∼ 9.5) did not result in significant complexation-induced shifts of the resonances of epinephrine, providing no evidence of direct interaction. Analysis of the alginate resonances proved to be less straightforward, as they appear in 1HNMR spectra as broad overlapped multiplets (Belattmania et al., 2020).
Further insight was therefore gained by Diffusion-Ordered NMR SpectroscopY (DOSY NMR) experiments on separate solutions of epinephrine and sodium alginate, as well as on a mixture of the two components. DOSY experiments provide self-diffusion coefficients (D) of dissolved species that may be used also for the detection of intermolecular interactions (Gattuso et al., 2014; Barbera et al., 2015). Spectra recorded on separate solutions of Eph– and Alg2– (Figures 8A–C) allowed for the extraction of the self-diffusion coefficient of the free species, DEph-(free) and DAlg2-(free), which were found to be 6.05 ± 0.216 and 0.224 ± 0.0945 (× 10−10 m2 s−1), respectively, in perfect agreement with the very different molecular size of the two species (i.e., unimolecular Eph– vs. polymeric Alg2–). Upon admixing the two compounds (Figure 8B), DOSY analysis showed that alginate maintained its unaltered self-diffusion coefficient, whereas epinephrine significantly decreased to 4.41 ± 0.207 and 0.262 ± 0.111 (× 10−10 m2 s−1) for DEph-(obs) and DAlg2-(obs), respectively, indicating that, in the Eph–/Alg2– aggregates, the translational mobility of faster-diffusing Eph– is reduced as a consequence of its binding to slower-diffusing Alg2– polymer.
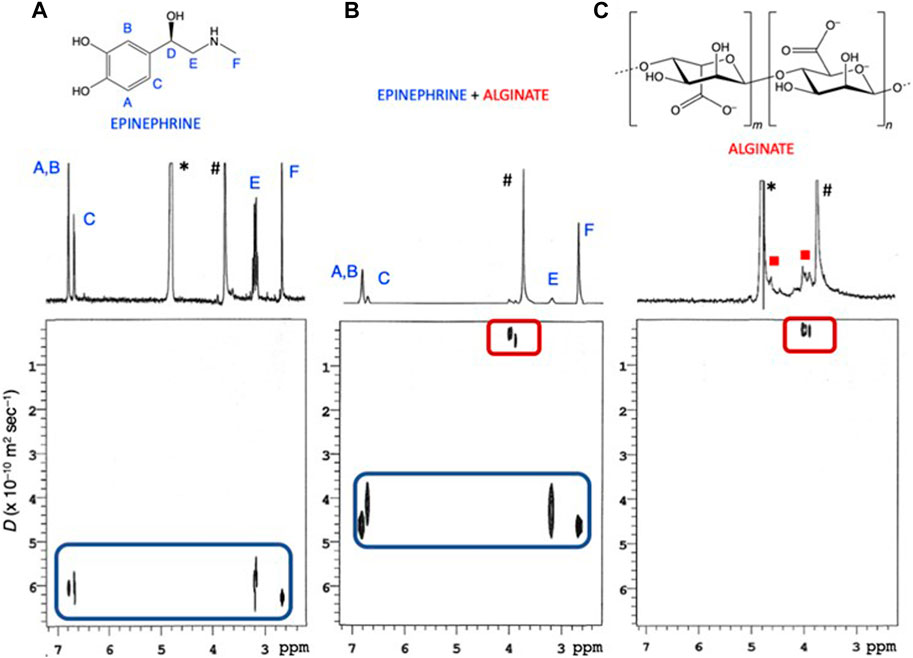
FIGURE 8. DOSY plots (500 MHz, T = 298.15 K, D2O) recorded on solutions containing: (A) cEph- = 2.29 mmol dm−3; (B) cEph- = 2.29 mmol dm−3 and cAlg2- = 2.60 mmol dm−3; (C) cAlg2- = 2.52 mmol dm−3 * Residual HOD solvent peak; #1,4-dioxane internal standard.
The diffusion coefficients obtained by DOSY experiments, in fast exchanging (on the NMR time-scale) systems, are weighted averages of all the free and bound species present at given pH/concentration values. As a result, D values for free and bound species can be used to estimate the mole fraction (χ) of Eph– bound to the larger Alg2–, according to Eq. 16:
where DEph-(obs) is the weighted average of the diffusion coefficients measured in the Eph– + Alg2– sample and DEph-(free) is the coefficient measured for Eph– alone. As for DEph-(bound), when considering the association of a small molecule, such as Eph–, to a much larger one (e.g., a polymer), the D value for the fully bound Eph– can be considered to be very close to that of Alg2– alone. As a consequence, the equation DEph-(bound) = DAlg2-(free) can be safely assumed, ultimately yielding a bound fraction value χ = 0.28.
Taking into account the concentration of Eph– and Alg2– in the DOSY experiment (cEph− = 2.29 and cAlg2- = 2.60 mmol dm−3, respectively), a simple calculation allowed us to deduce a logK value of 2.298 for Eph–/Alg2– association.
This data can be considered in good agreement with those obtained from potentiometric and UV-spectrophotometric measurements, taking into account that the logK value obtained from the DOSY experiments is valid at that condition.
The investigation on the ternary metal-ligand-ligand system were performed at I = 0.146 mol dm−3 in NaCl(aq), T = 298.15K, different components concentrations, and metal:ligands molar ratios. To avoid the formation of sparingly soluble species, the solutions were prepared with an excess of epinephrine, since from measurements carried out with an excess of alginate, the precipitate formed at pH ∼ 10, excluding the possibility of carrying out the experiments.
The data processing was performed in the pH range 3.0–10.0 because the formation of precipitate at pH ∼ 2.7 did not allow us to continue the experiments.
In Supplementary Figure S4, it is possible to appreciate an example of the color variation of the analysis solution during one of the measurements. At pH ∼ 10.0 the solution containing the metal and the two ligands assumed a yellow colour, becoming orange tending to red at pH ∼ 7.2, and then bright red at ∼ pH 6.5–6.8.
The elaboration of experimental data led to the determination of a speciation model featured by the following ternary species: Cu(Eph)(Alg)-, Cu(Eph)(Alg)H0(aq), Cu(Eph)2(Alg)2-, Cu(Eph)2(Alg)H−, and Cu(Eph)(Alg)2H2- whose formation can be expressed by Eq. 17:
The formation constant values (±std. dev.) determined for the mentioned ternary species were: logβCu(Eph)(Alg) = 15.81 ± 0.01, logβCu(Eph)(Alg)H = 21.35 ± 0.02, logβCu(Eph)2(Alg) = 24.55 ± 0.01, logβCu(Eph)2(Alg)H = 30.75 ± 0.04, and logβCu(Eph)(Alg)2H = 24.84 ± 0.02.
The analysis of the distribution diagram in Supplementary Figure S5 shows that, in slight epinephrine excess, the ternary species with the highest formation percentages are the Cu(Eph)(Alg)-, Cu(Eph)2(Alg)2-, and Cu(Eph)2(Alg)H−, reaching 33%, 47%, and 46% at pH ∼ 7.9, 9.0, and 4.3, respectively. At the mentioned conditions, the formation of binary Cu2+/ligands complexes is mainly observed in the pH range 5.0–10.0. The most significant binary Cu2+/Eph− species are the Cu2(Eph)22+ and Cu(Eph)2OH− which reaching 30% and 37% at pH ∼ 6.2 and 10.0, respectively. Concerning the Cu2+/Alg2- complexes, the hydrolytic Cu(Alg)(OH)22- and Cu(Alg)(OH)33- species forms at pH > 7.5 and exceeds 25% and 10% at pH ∼ 9.3 and 10.0, respectively. On the basis of these results, it is evident that a correct speciation study in multicomponent solutions cannot exclude the possible formation of mixed ternary complexes.
Based on these observations, in Supplementary Figure S6, a comparison between the sum of the mmoles of the metal/ligands species, considering and ignoring the ternary complexes, is shown.
The main differences between the two curves are found in the pH range 3.0–8.0, where the total contribution of the formed mixed species is much higher with respect to those given by the binary ones. On the contrary, at pH > 8.0, the only formed ternary species is the Cu(Eph)2(Alg)2- and the Cu2+/Eph− and Cu2+/Alg2- complexes prevail.
In the literature, Beck and Nagypal (Beck and Nagypál, 1991) asserted that the formation of hetero-metallic or ternary metal-ligand-ligand species is possible and statistically favored with respect to the corresponding homo-metallic or binary Mn+/L1z− and Mn+/L2v− (Mn+ = metal cation, L1z−, L2v− = ligands with different charge) complexes with the same stoichiometry. They suggested the possibility of the calculation of the “extra-stability” for the mixed ternary species using two approaches, namely, by means of the calculation of experimental (logXexp) and statistical (logXstat) extra-stability constants (Beck and Nagypál, 1991) and stated that if logXexp > logXstat, the formation of mixed complexes is thermodynamically favored.
As an application of this principle, a further analysis of the speciation schemes of Cu2+/Eph− (Table 3) and Cu2+/Alg2- (Table 4) systems suggested the presence of a common CuL2 species, namely, the Cu(Eph)20(aq) and Cu(Alg)22-, comparable with the Cu(Eph)(Alg)- complex displaying the same stoichiometry. Considering the indications reported in the literature, the logXexp can be expressed considering the following equilibrium (Eq. 18):
By performing a linear combination of the formation constants of binary and ternary complexes, it was possible to obtain:
Once the experimental extra-stability was determined, the corresponding statistical value (Xstat) was computed using the expression:
where h, i, and j are the stoichiometric coefficients in Eq. 18, namely, 1, 1, and 2, respectively. The Xstat and logXstat were calculated and resulted to be 4.00 and 0.60, respectively.
The comparison among the determined experimental and statistical extra-stability constant values suggested that logXexp > logXstat, confirming that the formation of ternary complexes is not only possible, but thermodynamically favored over the binary ones. The formation of the mixed ternary complexes and the extra-stability are very important factors both from an environmental and biological point of view, since in many cases, the extra-stability of the mixed ternary complexes favored the mobility and transport of these complexes, owing to an increase in the metal’s solubility.
The elaboration of potentiometric data recorded at I = 0.160 mol dm−3 in NaCl(aq), T = 298.15K, led to the determination of a speciation model featured by three ternary species, such as: Cu(UO2)(Eph)3+, Cu(UO2)(Eph)22+, and Cu(UO2)(Eph)2OH+. The overall formation constants, determined considering the equilibria in Eqs. 20, 21, were: logβCu(UO2)(Eph) = 15.44 ± 0.01, logβCu(UO2)(Eph)2 = 27.82 ± 0.01, and logβCu(UO2)(Eph)2OH = 22.61 ± 0.02:
The calculation of logXexp and logXstat values was performed to verify also whether the hetero-metallic Cu2+/UO22+/Eph− species formation could be thermodynamically favored with respect to the homo-metallic Cu2+/Eph− (Table 3) and UO22+/Eph− (Crea et al., 2020) complexes. The stability of the species with analogous stoichiometry to be analysed were the binary Cu2(Eph)22+ and (UO2)2(Eph)22+ with respect to the mixed Cu(UO2)(Eph)22+. The calculation of logXexp value referred to the following equilibrium (Eq. 22):
The experimental extra-stability constant was calculated as follows:
while the statistical value was determined using Eq. 19, with Xstat = 4.00 and logXstat = 0.60.
The calculated logXstat was lower with respect to the logXexp, confirming the Beck and Nagypal (Beck and Nagypál, 1991) assertation that the formation of hetero-metallic complexes is thermodynamically favored over the homo-metallic ones.
As further evidence of the mixed species significance, in Supplementary Figure S7, a comparison between the sum of the metals-ligand complexes formation percentages, considering and ignoring the ternary species, is shown. Up to pH ∼ 4.0–4.5, the two curves do not deviate from each other, while as the pH increases, significant changes in the sum of the formation percentages are observed, owing to the formation of the three ternary complexes with a ΔΣ of ∼30%.
The evaluation of the sequestering ability of a ligand towards one or more metal cations assumes a particular importance for the resolution of real biological and environmental issues, such as the treatment of human body for detoxification or the remediation of polluted sites, respectively, both involving the use of a chelating agent.
For this purpose, the calculation of an empirical parameter, the pL0.5, representing the total ligand concentration required for the sequestration of the 50% of a metal cation present at trace (cMn+ ∼ 10–12 mol dm−3) concentration in solution, was proposed by our research group (Crea et al., 2014). This objective and quantitative parameter can be described by a sigmoidal-type Boltzmann equation, with asymptotes equal to one for pL→-∞ and 0 for pL → +∞ (Eq. 23):
with:
xM = mole fraction of Mn+ complexed by the ligand; pL = -logcL; pL0.5 = -logcL, if xM = 0.5.
The higher the pL0.5 value, the stronger the sequestering ability of a ligand towards the selected metal cation.
The evaluation of the sequestering ability of epinephrine and alginate towards Cu2+ was carried out by means of the calculation of the pL0.5 at T = 298.15K, different ionic strengths in NaCl(aq), and pH values using the equilibrium constants determined from potentiometric data (Tables 3, 4). From the analysis of the values in Table 6 and the graphs in Supplementary Figure S8, it can be concluded that for both the ligands, the sequestering ability increases with pH raising, possibly owing to the gradual epinephrine and alginate deprotonation, favouring the Cu2+/ligands electrostatic interaction.
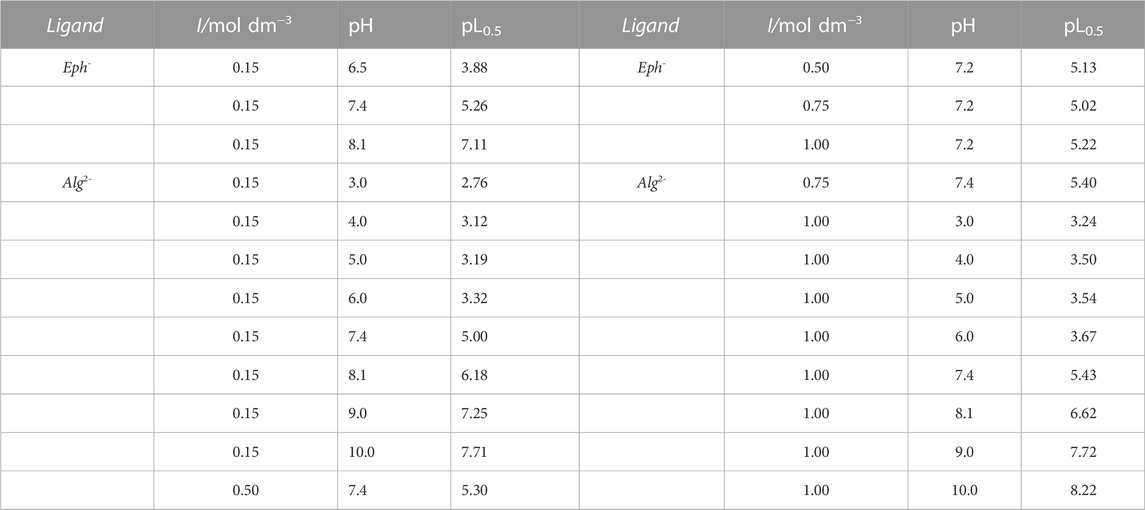
TABLE 6. pL0.5 values calculated using Eq. 23 at T = 298.15K, different ionic strengths in NaCl(aq), and pH conditions.
Concerning the effect of ionic strength, for both Eph− and Alg2-, the pL0.5 values calculated at pH ∼ 7.4, respectively, similar to the physiological value, follow the same trend of the stability constants reported in Tables 3, 4.
The pL0.5 calculation was a very efficient tool for the investigation of the sequestering ability of many ligands towards metal cations. Nevertheless, if, in a multicomponent system, one or more polynuclear complexes were determined, like in the case of the Cu2+/Eph- investigation where Cu2(Eph)OH2+, Cu2(Eph)3+, and Cu2(Eph)22+ species were obtained, they should not form at the trace (cMn+ ∼ 10–12 mol dm−3) concentration in which the empirical parameter is usually computed. In these cases, the comparison of the sequestering ability of a ligand towards two or more metals or two ligands towards a metal cation can fail, since the pL0.5 value calculated for the metal:ligand systems that have in the speciation model polynuclear species, cannot be effectively representative of the quantification of the binding ability. In this light, objective comparisons among the affinity of the ligands towards Cu2+ can be performed by determining the pM parameter (Raymond and Carrano, 1979) (see 3.7. Cu2+ affinity section), considering the free metal cation concentration for the calculations, without being affected by the presence of complexes with various stoichiometry.
The abovementioned drawbacks, observable in the calculation of the pL0.5 parameter for making a comparison between epinephrine and alginate affinity towards Cu2+ owing to the presence of polynuclear complexes in one of the systems, may be solved by means of the pM (Raymond and Carrano, 1979) parameter, in this case, the pCu, with pCu = -log [Cu]free and cCu2+ = 0.001 mmol dm−3. The calculations were performed at cEph− = cAlg2- = 0.01 mmol dm−3 and at different pHs, namely, pH ∼ 7.4 to simulate physiological conditions and pH ∼ 8.1 for seawater.
The pCu values were calculated for the two Cu2+/Eph− and Cu2+/Alg2- systems at I = 0.15 and 1.00 mol dm−3 in NaCl(aq), T = 298.15K, and compared to each other to evaluate which of the ligands could have the best efficiency towards the metal cation. As observable in Supplementary Table S7 and Figure 9, at all experimental conditions, epinephrine displays a higher Cu2+ affinity than alginate. This tendency could be possibly explainable looking at the “hard-soft acids and bases” theory (HSAB) (Pearson, 1963; Pearson, 1968), where interactions between hard acids and hard bases as well as soft acids and soft bases are kinetically and thermodynamically favored when compared with hard-soft interactions. As a consequence of these considerations, borderline acids and borderline bases interactions should be favored too. On this basis, the affinity between Cu2+, a borderline metal cation, and the borderline adrenaline amino group could be higher with respect to the hard functional groups (-COOH) present in the alginate structure. Both the ligands also featured other hard sites, namely, -OH groups.
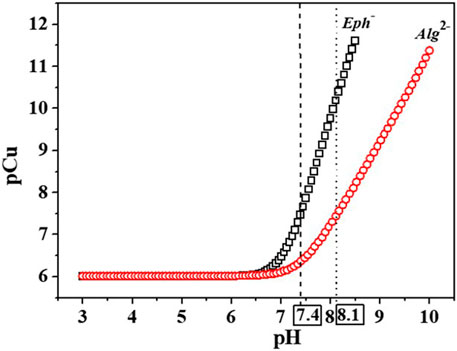
FIGURE 9. pCu values trend calculated vs. pH for the Cu2+/Eph- and Cu2+/Alg2- systems. pCu = -log [Cu]free with ccu2+ = 0.001 mmol dm−3 and cEph- = cAlg2- = 0.01 mmol dm−3.
The analysis of data in Supplementary Table S7 also suggests that the metal efficacy increases with pH, possibly due to the decrease of free copper(II) in solution in favor of the complexed form with the ligands binding groups, and with ionic strength increase, as already observed from the calculation of the pL0.5 values.
As a comparison, Supplementary Figure S9 reports the correlation between the pL0.5 values and pH for the Cu2+/Alg2- system at two different ionic strengths, I = 0.15 and 1.00 mol dm−3, respectively, where it is possible to observe a very similar trend to the one obtained from the pCu calculation.
The speciation of epinephrine (Eph−) in the presence of alginate (Alg2-) and two biological and environmental relevant metal cations (Cu2+, UO22+) was investigated at T = 298.15K, I = 0.15–1.00 mol dm−3 in NaCl(aq). The formation of binary and ternary complexes was evaluated and, since epinephrine can behave as a zwitterion in aqueous solution, the possible Eph−/Alg2- interaction was also studied.
The main results can be summarized as follows:
a) The equilibrium constants for alginate protonation and for the complexation with Cu2+ were determined by potentiometry and modelled for the dependence on ionic strengths using an extended Debye-Hückel type equation;
b) The complexing ability of epinephrine towards the metal cation was studied by means of potentiometry and UV-Vis spectrophotometry; the formation constants were in agreement among the two analytical techniques and the effect of ionic strength on the stability of the species was studied using an extended Debye-Hückel type equation and the Specific ion Interaction Theory (SIT) approach;
c) Isoperibolic titration calorimetry allowed us to determine the enthalpy change values of formation for only two Cu2+/Eph− complexes, owing to the solubility problems of epinephrine at the selected experimental conditions; the entropic contribution was the driving force for the Cu2+/Eph− species formation;
d) Thermogravimetric experiments gained information on the thermal stability and metal/ligand complexes stoichiometry;
e) The sequestering ability of Eph− and Alg2- towards Cu2+, evaluated by the pL0.5 calculation, increases with pH and ionic strength, and the calculation of the pM parameter confirmed that Eph− has a higher metal affinity with respect to Alg2- at selected pH and I/mol dm−3 conditions;
f) The formation of binary Eph−/Alg2- species was tested using UV-Vis spectrophotometry and 1H NMR measurements; the obtained results are in very good agreement with those determined from potentiometry both in term of speciation model and stability of the ligand-ligand species; moreover, the DOSY measurements allowed us to calculate a mean stability constant for the interaction between Eph− and Alg2-, logKEph−/Alg2- = 2.9. Taking into account that this stability constant can be considered as mean value of all the interactions between the component at those experimental condition, this data can also be considered reliable to quantify the interaction between the two ligands;
g) The ternary Cu2+/Eph−/Alg2- and Cu2+/UO22+/Eph− interactions were investigated by potentiometry and different speciation schemes were determined; the knowledge of the possible formation of ternary species is fundamental for the treatment of many real biological and environmental problems;
h) The extra-stability constants of selected Cu2+/Eph−/Alg2- and Cu2+/UO22+/Eph− species were calculated and their formation were more thermodynamically favorable than the corresponding binary ones with the same stoichiometry.
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Conceptualization, FC and AI; Methodology, FC, CD, and RC; Software, FC and AI; Validation, FC, AI, and RC; Formal analysis, FC, AI, CA, and CD; Investigation, FC, AI, and GG; Data curation, FC, AI, GG, and RC; Writing—original draft preparation, FC and AI; Writing—review and editing, FC and CD; Supervision, CD and FC; Project administration, FC; Funding acquisition, FC.
The publication fee will be partially covered by the Fondo di Finanziamento per le Attività Base di Ricerca (F.F.A.B.R.) year 2020. The authors thank the University of Messina for FFABR 2020 financing.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2023.1189308/full#supplementary-material
Al-Ayed, A. S., Al-Lohedan, H. A., Rafiquee, M. Z. A., Ali, M. S., and Issa, Z. A. (2013). Kinetics of the autoxidation of adrenaline and [copper(II)(adrenaline)]2+ in alkaline aqueous and micellar media. Transit. Metal. Chem. 38, 173–181. doi:10.1007/s11243-012-9675-3
Alderighi, L., Gans, P., Ienco, A., Peters, D., Sabatini, A., and Vacca, A. (1999). Hyperquad simulation and speciation (HySS): A utility program for the investigation of equilibria involving soluble and partially soluble species. Coord. Chem. Rev. 184, 311–318. doi:10.1016/s0010-8545(98)00260-4
Antikainen, P. J., Witikainen, U., Lindström, B., Enzell, C. R., Enzell, C. R., and Swahn, C. G. (1973). A comparative study on the ionization of catechol amines in aqueous solutions. Acta Chem. Scand. 27, 2075–2082. doi:10.3891/acta.chem.scand.27-2075
Barbera, L., Gattuso, G., Kohnke, F. H., Notti, A., Pappalardo, S., Parisi, M. F., et al. (2015). Self-assembly of amphiphilic anionic calix[4]arenes and encapsulation of poorly soluble naproxen and flurbiprofen. Org. Biomol. Chem. 13, 6468–6473. doi:10.1039/c5ob00703h
Barbero, M., Berto, S., Cadamuro, S., Daniele, P. G., Dughera, S., and Ghigo, G. (2013). Catalytic properties and acidity of 1,2-benzenedisulfonimide and some of its derivatives. An experimental and computational study. Tetrahedron 69, 3212–3217. doi:10.1016/j.tet.2013.02.053
Belattmania, Z., Kaidi, S., El Atouani, S., Katif, C. B., Reani, A., Sabour, B., et al. (2020). Isolation and FTIR-ATR and 1H NMR characterization of alginates from the main alginophyte species of the atlantic coast of Morocco. Molecules 25, 4335. doi:10.3390/molecules25184335Jama
Berto, S., Carena, L., Valmacco, F., Barolo, C., Conca, E., Vione, D., et al. (2018). Application of an electro-activated glassy-carbon electrode to the determination of acetaminophen (paracetamol) in surface waters. Electrochim. Acta 284, 279–286. doi:10.1016/j.electacta.2018.07.145
Biederman, G. (1986). “Introduction to the specific interaction theory with emphasis on chemical equilibria,” in Metal complexes in solution. Editors E. A. Jenne, E. Rizzarelli, V. Romano, and S. Sammartano (Padua, Italy: Piccin), 303–314.
Biederman, G. (1975). Ionic media, dahlem workshop on the nature of seawater, dahlem konferenzen. Berlin, Germany.
Bretti, C., Cigala, R. M., Crea, F., De Stefano, C., Gattuso, G., Irto, A., et al. (2017). Thermodynamic properties of O-donor polyelectrolytes: Determination of the acid-base and complexing parameters in different ionic media at different temperatures. J. Chem. Eng. Data 62, 2676–2688. doi:10.1021/acs.jced.7b00101
Bretti, C., Cigala, R. M., Crea, F., Stefano, C., and Vianelli, G. (2015). Solubility and modeling acid–base properties of adrenaline in NaCl aqueous solutions at different ionic strengths and temperatures. Eur. J. Pharm. Sci. 78, 37–46. doi:10.1016/j.ejps.2015.06.025
Bretti, C., Foti, C., Porcino, N., and Sammartano, S. (2006). SIT parameters for 1:1 electrolytes and correlation with pitzer coefficients. J. Solut. Chem. 35, 1401–1415. doi:10.1007/s10953-006-9068-3
Buffle, J. (1988). Complexation reactions in aquatic systems: An analytical approach. Chichester: Ellis Horwood.
Cahill, L., and Alkire, M. L. T. (2003). Epinephrine enhancement of human memory consolidation: Interaction with arousal at encoding. Neurobiol. Learn. Mem. 79, 194–198. doi:10.1016/s1074-7427(02)00036-9
Cigala, R. M., De Stefano, C., Irto, A., Milea, D., and Sammartano, S. (2015). Thermodynamic data for the modeling of lanthanoid(III) sequestration by reduced glutathione in aqueous solution. J. Chem. Eng. Data 60, 192–201. doi:10.1021/je500961u
Crea, F., De Stefano, C., Foti, C., Milea, D., and Sammartano, S. (2014). Chelating agents for the sequestration of mercury(II) and monomethyl mercury(II). Curr. Med. Chem. 21, 3819–3836. doi:10.2174/0929867321666140601160740
Crea, F., De Stefano, C., Irto, A., Lando, G., Materazzi, S., Milea, D., et al. (2020). Understanding the solution behavior of epinephrine in the presence of toxic cations: A thermodynamic investigation in different experimental conditions. Molecules 25, 511. doi:10.3390/molecules25030511
Crea, F., De Stefano, C., Manfredi, G., and Sammartano, S. (2010). Sequestration of some biogenic amines and poly(allyl)amine by high molecular weight polycarboxylic ligands in aqueous solution. J. Mol. Liq. 151, 138–144. doi:10.1016/j.molliq.2009.12.007
Crea, F., Robertis, A., and Sammartano, S. (2003). Dioxouranium-carboxylate complexes. Formation and stability of acetate species at different ionic strengths in NaClaq. Ann. Chim. (Rome) 93, 1027–1035.
Davis, T. A., Llanes, F., Volesky, B., and Mucci, A. (2003). Metal selectivity of sargassum spp. and their alginates in relation to their α-l-Guluronic acid content and conformation. Environ. Sci. Technol. 37, 261–267. doi:10.1021/es025781d
De Stefano, C., Foti, C., Giuffrè, O., and Sammartano, S. (2001). Dependence on ionic strength of protonation enthalpies of polycarboxylate anions in NaCl aqueous solution. J. Chem. Eng. Data 46, 1417–1424. doi:10.1021/je010142o
De Stefano, C., Gianguzza, A., Pettignano, A., Sammartano, S., and Sciarrino, S. (2010). On the complexation of Cu(II) and Cd(II) with polycarboxyl ligands. Potentiometric studies with ISE-H+, ISE-Cu2+, and ISE-Cd2+. J. Chem. Eng. Data 55, 714–722. doi:10.1021/je9004245
De Stefano, C., Gianguzza, A., Piazzese, D., and Sammartano, S. (2005). Modelling of proton and metal exchange in the alginate biopolymer. Anal. Bioanal. Chem. 383, 587–596. doi:10.1007/s00216-005-0025-6
De Stefano, C., Sammartano, S., Mineo, P., and Rigano, C. (1997). “Computer tools for the speciation of natural fluids,” in Marine Chemistry - an environmental analytical Chemistry approach. Editors A. Gianguzza, E. Pelizzetti, and S. Sammartano. (Amsterdam: Kluwer Academic Publishers), 71–83.:
Flint, R. W., Bunsey, M. D., and Riccio, D. C. (2007). Epinephrine-induced enhancement of memory retrieval for inhibitory avoidance conditioning in preweanling Sprague–Dawley rats. Dev. Psychobiol. 49, 303–311. doi:10.1002/dev.20212
Foti, C., Gianguzza, A., Milea, D., and Sammartano, S. (2002). Hydrolysis and chemical speciation of (C2H5)2Sn2+, (C2H5)3Sn+ and (C3H7)3Sn+ in aqueous media simulating the major composition of natural waters. Appl. Organomet. Chem. 16, 34–43. doi:10.1002/aoc.249
Gans, P., Sabatini, A., and Vacca, A. (1996). Investigation of equilibria in solution. Determination of equilibrium constants with the HYPERQUAD suite of programs. Talanta 43, 1739–1753. doi:10.1016/0039-9140(96)01958-3
Gattuso, G., Pappalardo, S., Parisi, M. F., Pisagatti, I., Patanè, S., and Patanè, S. (2014). Encapsulation of monoamine neurotransmitters and trace amines by amphiphilic anionic calix[5]arene micelles. New J. Chem. 38, 5983–5990. doi:10.1039/c4nj01184h
Gerard, C., and Hanane, C. (1997). Stability of metal complexes with ligands of biological interest: Dopamine and adrenaline. Bull. Soc. Chim. Fr. 134, 1069–1074.
Gergely, A., Kiss, T., Deák, G., and Sóvágó, I. (1981). Complexes of 3,4-dihydroxyphenyl derivatives IV. Equilibrium studies on some transition metal complexes formed with adrenaline and noradrenaline. Inorg. Chim. Acta 56, 35–40. doi:10.1016/s0020-1693(00)88544-8
Gianguzza, A., Demetrio, M., Millero, F. J., and Silvio, S. (2004). Hydrolysis and chemical speciation of dioxouranium(VI) ion in aqueous media simulating the major ion composition of seawater. Mar. Chem. 85, 103–124. doi:10.1016/j.marchem.2003.10.002
Grenthe, I., and Puigdomenech, I. (1997). Modelling in aquatic Chemistry. Paris: Nuclear Energy Agency.
Grgas-Kužnar, B., Simeon, V., and Weber, O. A. (1974). Complexes of adrenaline and related compounds with Ni2+, Cu2+, Zn2+, Cd2+ and Pb2+. J. Inorg. Nucl. Chem. 36, 2151–2154. doi:10.1016/0022-1902(74)80741-4
HöGfeldt, E. (1988). A useful method for summarizing data in ion exchange. 4. Extension and application to weak acid and chelating resins. J. Phys. Chem. 92, 6475–6476. doi:10.1021/j100333a057
HöGfeldt, E., Miyajima, T., Marinsky, J. A., Muhammed, M., Ruiz, J., Colacio, E., et al. (1989). Application of a simple three-parameter model to titration data for some linear polyelectrolytes. Acta Chem. Scand. 43, 496–499. doi:10.3891/acta.chem.scand.43-0496
Idota, Y., Kogure, Y., Kato, T., Yano, K., Arakawa, H., Miyajima, C., et al. (2016). Relationship between physical parameters of various metal ions and binding affinity for alginate. Biol. Pharm. Bull. 39, 1893–1896. doi:10.1248/bpb.b16-00127
Irto, A., Cardiano, P., Cataldo, S., Chand, K., Cigala, R. M., Crea, F., et al. (2019a). Speciation studies of bifunctional 3-hydroxy-4-pyridinone ligands in the presence of Zn2+ at different ionic strengths and temperatures. Molecules 24, 4084. doi:10.3390/molecules24224084
Irto, A., Cardiano, P., Chand, K., Cigala, R. M., Crea, F., De Stefano, C., et al. (2019b). A new bis-(3-hydroxy-4-pyridinone)-DTPA-derivative: Synthesis, complexation of di-/tri-valent metal cations and in vivo M3+ sequestering ability. J. Mol. Liq. 281, 280–294. doi:10.1016/j.molliq.2019.02.042
Irto, A., Cardiano, P., Chand, K., Cigala, R. M., Crea, F., De Stefano, C., et al. (2018). Bifunctional 3-hydroxy-4-pyridinones as effective aluminium chelators: Synthesis, solution equilibrium studies and in vivo evaluation. J. Inorg. Biochem. 186, 116–129. doi:10.1016/j.jinorgbio.2018.05.017
Irto, A., Cardiano, P., Chand, K., Cigala, R. M., Crea, F., De Stefano, C., et al. (2020). Complexation of environmentally and biologically relevant metals with bifunctional 3-hydroxy-4-pyridinones. J. Mol. Liq. 319, 114349. doi:10.1016/j.molliq.2020.114349
Irto, A., Cardiano, P., Chand, K., Cigala, R. M., Crea, F., De Stefano, C., et al. (2021). Bifunctional 3-hydroxy-4-pyridinones as potential selective iron(III) chelators: Solution studies and comparison with other metals of biological and environmental relevance. Molecules 26 (23), 7280. doi:10.3390/molecules26237280
Jameson, R. F., and Neillie, W. F. S. (1965). Complexes formed by adrenaline and related compounds with transition-metal ions—II complexes with copper(II). J. Inorg. Nucl. Chem. 27, 2623–2634. doi:10.1016/0022-1902(65)80166-x
Jameson, R. F., and Neillie, W. F. S. (1966). Complexes formed by adrenaline and related compounds with transition-metal ions—III: The stabilities of some first-row transition-metal complexes. J. Inorg. Nucl. Chem. 28, 2667–2675. doi:10.1016/0022-1902(66)80392-5
Katchalsky, A. (1954). Problems in the physical chemistry of polyelectrolytes. J. Polym. Sc. 12, 159–184. doi:10.1002/pol.1954.120120114
Katchalsky, A., and Spitnik, P. (1947). Potentiometric titrations of polymethacrylic acid. J. Polym. Sci. 2, 487–446. doi:10.1002/pol.1947.120020504
Kiss, T., and Gergely, A. (1983). Complexes of 3,4-dihydroxyphenyl derivatives. VI. Microprocesses of formation of proton and metal complexes of L-dopa. Inorg. Chim. Acta 78, 247–254. doi:10.1016/s0020-1693(00)86520-2
Krasnovskaya, O., Naumov, A., Guk, D., Gorelkin, P., Erofeev, A., Beloglazkina, E., et al. (2020). Copper coordination compounds as biologically active agents. Int. J. Mol. Sci. 21, 3965. doi:10.3390/ijms211139653965
Lee, K. Y., and Mooney, D. J. (2012). Alginate: Properties and biomedical applications. Prog. Polym. Sci. 37, 106–126. doi:10.1016/j.progpolymsci.2011.06.003
Materazzi, S., Vasca, E., Tentolini, U., Aquili, S., and Curini, R. (2002). A thermoanalytical study of unusual adrenaline complexes. Thermochim. Acta 389, 179–184. doi:10.1016/s0040-6031(02)00056-4
Mohammed, C., Lalgee, L., Kistow, M., Jalsa, N., and Ward, K. (2022). On the binding affinity and thermodynamics of sodium alginate-heavy metal ion interactions for efficient adsorption. Carb. Polym. Techn. Appl. 3, 100203. doi:10.1016/j.carpta.2022.100203
Pandey, A. K., Pandey, S. D., and Misra, V. (2000). Stability constants of metal–humic acid complexes and its role in environmental detoxification. Ecotoxicol. Environ. Saf. 47, 195–200. doi:10.1006/eesa.2000.1947
Pearson, R. G. (1963). Hard and soft acids and bases. J. Am. Chem. Soc. 85, 3533–3539. doi:10.1021/ja00905a001
Pearson, R. G. (1968). Hard and soft acids and bases, HSAB, part II: Underlying theories. J. Chem. Educ. 45 (581), 643. doi:10.1021/ed045p643
Pelta, M. D., Morris, G. A., Tschedroff, M. J., and Hammon, S. J. (2002). A one-shot sequence for high-resolution diffusion-ordered spectroscopy. Magn. Reson. Chem. 40, S147–S152. doi:10.1002/mrc.1107
Pereira, L., and Cotas, J. (2019). “Alginates - a general overview,” in Alginates - recent uses of this natural polymer, marine and environmental Sciences centre (MARE), 1–10.
Piperea-Şianu, A., Sîrbu, I., Mati, E., Piperea-Şianu, D., and Mircioiu, C. (2015). Study of synergic effect between some metal ions and adrenaline on human blood platelets aggregation. Farmacia 63, 828–834.
Rahaman, M. S., and Korenkiewicz, S. M. (1976). Electronic and Raman spectroscopic studies of copper adrenalin complexes and copper induced compounds. Can. J. Chem. 54, 3815–3823. doi:10.1139/v76-548
Raymond, K. N., and Carrano, C. J. (1979). Coordination chemistry and microbial iron transport. Acc. Chem. Res. 12, 183–190. doi:10.1021/ar50137a004
Santander, P., Butter, B., Oyarce, E., Yáñez, M., Xiao, L.-P., and Sánchez, J. (2021). Lignin-based adsorbent materials for metal ion removal from wastewater: A review. Ind. Crops Prod. 167, 113510. doi:10.1016/j.indcrop.2021.113510
Schiewer, S., and Volesky, B. (1996). Modeling multi-metal ion exchange in biosorption. Environ. Sci. Technol. 30, 2921–2927. doi:10.1021/es950800n
Schneider, I. a. H., and Rubio, J. (1999). Sorption of heavy metal ions by the nonliving biomass of freshwater macrophytes. Environ. Sci. Technol. 33, 2213–2217. doi:10.1021/es981090z
Setschenow, J. Z. Z. (1889). Uber die konstitution der salzlosungenauf grund ihres verhaltens zu kohlensaure. Phys. Chem. 4, 117–125. doi:10.1515/zpch-1889-0109
Templeton, D. M., Ariese, F., Cornelis, R., Danielsson, L. G., Muntau, H., Van Leeuwen, H. P., et al. (2000). Guidelines for terms related to chemical speciation and fractionation of elements. Definitions, structural aspects, and methodological approaches (IUPAC Recommendations 2000). Pure App. Chem. 72, 1453–1470. doi:10.1351/pac200072081453
Vione, D., De Laurentiis, E., Berto, S., Minero, C., Hatipoglu, A., and Cinar, Z. (2016). Modeling the photochemical transformation of nitrobenzene under conditions relevant to sunlit surface waters: Reaction pathways and formation of intermediates. Chemosphere 145, 277–283. doi:10.1016/j.chemosphere.2015.11.039
Keywords: epinephrine, alginate, Cu2+ and UO22+ sequestration, ligand-ligand interaction, ternary complexes, extra-stability, DOSY NMR
Citation: Irto A, Cigala RM, Alessandrello C, De Stefano C, Gattuso G and Crea F (2023) Binary and ternary complexes of epinephrine with alginate and biologically and environmentally relevant metal cations. Front. Chem. 11:1189308. doi: 10.3389/fchem.2023.1189308
Received: 18 March 2023; Accepted: 07 April 2023;
Published: 25 April 2023.
Edited by:
Taiping Qing, Xiangtan University, ChinaReviewed by:
George Gamov, Ivanovo State University of Chemistry and Technology, RussiaCopyright © 2023 Irto, Cigala, Alessandrello, De Stefano, Gattuso and Crea. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Francesco Crea, ZnJhbmNlc2NvLmNyZWFAdW5pbWUuaXQ=
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.