Characterization of Four Copper Materials for Application as Reference Materials for High Precision Copper Isotope Analysis by Laser Ablation Inductively Coupled Plasma Multi-Collector Mass Spectrometry

Laser ablation inductively coupled plasma multi-collector mass spectrometry (LA-MC-ICP-MS) allows rapid, in situ, highly precise measurements of Cu isotope ratios of native Cu and Cu-bearing minerals. However, the National Institute of Standards and Technology Cu-metal isotope standard NIST SRM976 that is commonly used to calibrate LA-MC-ICP-MS Cu isotope measurements of native Cu is no longer available. We have investigated the suitability of four Cu metal materials, SSC-1, SSC-3 and SSC-4 (cathode Cu metal rods) and CUPD-1 (Cu anode sawings), originally developed by the Canada Centre for Mineral and Energy Technology (CANMET) as certified reference materials for trace element analysis, as Cu isotope reference materials for LA-MC-ICP-MS analysis and solution nebulization (SN) of Cu. The Cu isotopic composition and homogeneity of these four materials were characterised by SN- and LA-MC-ICP-MS, and are reported for the first time. The bulk Cu isotopic compositions, expressed as δ⁶⁵Cu_SRM976 in per mil (‰) relative to NIST SRM976 with combined uncertainties (U, k = 2), of SSC-1, SSC-3 and SSC-4, determined utilizing SN-MC-ICP-MS, are identical within analytical uncertainty at 0.03 ± 0.07‰ (n = 29), 0.04 ± 0.04‰ (n = 28), and 0.05 ± 0.08‰ (n = 29), respectively; the composition of CUPD-1 is 2.14 ± 0.08‰ (n = 28). The compositions are 0.01 ± 0.07‰ (n = 29), 0.04 ± 0.06‰ (n = 29), 0.03 ± 0.06‰ (n = 28) and 2.15 ± 0.06‰ (n = 28), respectively, relative to the European Reference Material ERM®-AE633 Cu isotope standard. The Cu isotope homogeneity of the four new reference materials was assessed by determining whether multiple individual in situ Cu isotope measurements made by LA-MC-ICP-MS analysis (43 µm spot size), using each of the other three reference materials as a calibrator, approximate a single normal distribution. We also investigate whether there are statistically significant differences between the mean δ⁶⁵Cu values of three independent data sets for each of the Cu isotope reference materials using one-way analysis of variance (ANOVA). Normality tests (graphical assessment of normal distribution quantile-quantile plots, and the Shapiro-Wilk, Jarque-Bera and reduced chi-squared statistic tests) show that: 1) the Cu isotope data acquired on SSC-1, SSC-3, SSC-4 and CUPD-1 do not depart significantly from a normal distribution, 2) the scatter of the Cu isotope data is due to analytical uncertainty with 95% confidence, and 3) there are no other significant sources of scatter; e.g. heterogeneity of the reference materials. The results of one-way ANOVA reveal that the mean difference of the δ⁶⁵Cu value for each of the reference materials SSC-1, SSC-3, SSC-4 and CUPD-1 is statistically not significant at the 0.05 level. The mean δ⁶⁵Cu_SRM976 values with combined uncertainties (U, k = 2) of SSC-1, SSC-3, SSC-4 and CUPD-1, determined by LA-MC-ICP-MS using each of the other three reference materials as a calibration standard, are 0.03 ± 0.09‰ (n = 132), 0.05 ± 0.09‰ (n = 154), 0.03 ± 0.09‰ (n = 144) and 2.14 ± 0.10‰ (n = 106), respectively. These values are in agreement with those determined by SN-MC-ICP-MS analysis at the 95% confidence level and have excellent precision (2 s.d. ≤ 0.10‰). These results suggest that SSC-1, SSC-3, SSC-4 and CUPD-1 can be considered isotopically homogeneous at a spatial resolution of 43 μm, and they are suitable reference materials for calibration and quality control of in situ and solution nebulization Cu isotope analyses of Cu.

Introduction

Copper is economically an extremely important metal in our industrial and technological society. Copper is commonly concentrated in ores in the form of copper sulphides, such as chalcocite (Cu₂S), covellite (CuS), chalcopyrite (CuFeS₂) and bornite (Cu₅FeS₄), and other ore minerals formed during hypogene and supergene ore mineralization processes (Faure 1991; Larson et al., 2003; Mathur et al., 2009a; Baxton and Mathur, 2011; Mathur et al., 2013; Mathur et al., 2018). However, more rarely, it can also occur as native Cu metal, which was highly prized for production of tools and weapons prior to development of smelting technologies.

Copper is siderophile and highly chalcophile (Siebert et al., 2011). It is also a redox-sensitive element, having two oxidation states - cuprous Cu (I) and cupric Cu (II) that, in addition to metallic Cu, occur in natural environments (Moynier et al., 2017). Copper has two stable isotopes, ⁶⁵Cu and ⁶³Cu, with a nominal relative mass difference of 3.2%. Copper’s multiple oxidation states and the significant relative mass difference of its isotopes result in measurable isotopic fractionation during chemical processes involving Cu during ore formation, with recorded δ⁶⁵Cu values (⁶⁵Cu/⁶³Cu isotope amount ratio relative to a Cu isotope standard NIST SRM976 in per mil) ranging from −16.49‰ to +19.73‰ (Yuan et al., 2017).

The pioneering search for natural variations in Cu isotope ratios (Walker et al., 1958; Shields et al., 1965) was made using thermal ionization mass spectrometry (TIMS). Both of these investigations demonstrated Cu isotopic variations of several per mil in copper ores despite achievable analytical uncertainty poorer than 1.5‰ due to the time-dependent mass bias for Cu isotopes that was difficult to evaluate properly (Ikehata et al., 2008; Moynier et al., 2017). With the benefit of the much increased ionization efficiency of an ICP ion source, the advent of multi-collector inductively coupled plasma mass spectrometry (MC-ICP-MS) allowed precise and accurate measurement of Cu isotope ratios with analytical precision (2 s.d.) better than 0.08‰ (Gale et al., 1999; Maréchal et al., 1999; Zhu et al., 2000; Maréchal and Albarede, 2002; Archer and Vance, 2004; Mason et al., 2004a; Mason et al., 2004b). This made it possible to investigate the natural mass-dependent Cu isotope variations within single deposits where Cu isotopic variations are commonly < 2.0‰. In the 2 decades following the development of MC-ICP-MS, Cu isotope compositions, determined using solution nebulization (SN) MC-ICP-MS, have been used in studies of a wide spectrum of Cu ore-forming systems, including native Cu deposits (Zhu et al., 2000; Larson et al., 2003; Ikehata and Hirata, 2012; Dekov et al., 2013; Ikehata et al., 2015; Bornhorst and Mathur, 2017; Baggio et al., 2018) and also, increasingly, in archaeology for the purpose of investigating the provenance of Cu artefacts (Mathur et al., 2009b; Mathur et al., 2014; Wilson et al., 2016).

Coupling laser ablation (LA) sample introduction with MC-ICP-MS detection provided a new technique for rapid and precise in situ determination of Cu isotope ratios of native Cu and Cu-bearing minerals (Jackson et al., 2001; Jackson and Günther, 2003). The LA-MC-ICP-MS is especially important for resolving Cu isotope differences in Cu sulphides on fine scales (10’s of µm) (Ikehata et al., 2008; Ikehata and Hirata, 2013) where sampling extremely small domains by micro-drilling techniques for SN-ICP-MS analysis are almost impossible. Investigations of Cu isotopes, employing lasers with nanosecond (ns) pulse widths (Graham et al., 2004; Li et al., 2010) and lasers operating in the femtosecond (fs) regime (Ikehata et al., 2011; Ikehata and Hirata, 2013; Lazarov and Horn, 2015) have demonstrated that LA-MC-ICP-MS is a technique that can provide in situ measurements with precisions capable of resolving Cu isotope ratio variations in ore deposits. However, laser-induced isotopic fractionation, including transient and matrix-dependent isotopic fractionation effects (Jackson et al., 2001; Jackson and Günther, 2003; Hirata et al., 2003; Kuhn et al., 2007; Horn and von Blanckenburg, 2007; Poitrasson and d'Abzac, 2017) encountered in the determination of Cu isotope ratios by LA-MC-ICP-MS using ns lasers, pose a challenge for precise and accurate Cu isotope measurement. The source of this fractionation is generally considered to be related to an ablation mechanism that is dominated by thermal effects. This results in melting of the sample and generation of sample aerosols consisting of both condensed vapour and melt droplets, which are isotopically fractionated and which change in relative proportions with matrix and ablation time. Differential transport efficiencies of the different particle types, together with incomplete volatilisation of the larger particles (melt droplets) in the ICP (Jackson et al., 2001; Jackson and Günther, 2003; Horn and von Blanckenburg, 2007), generate the observed fractionation effects. Thus matrix-matched calibration standards and the standard-sample-standard bracketing (SSB) method are required to achieve accurate results and sufficient precision (ideally < 0.1‰) for resolving small natural isotopic variations present in individual primary native copper and copper sulphide minerals.

The Cu isotope standard NIST SRM976, a pure copper metal that has been used not only for solution nebulization but also for laser ablation Cu isotope analysis, is no longer available. New solution Cu isotope certified reference materials have been developed, including ERM®-AE633, ERM®-AE647 (Moeller et al., 2012), Cu isotope reference materials NWU-Cu-A and NWU-Cu-B (Yuan et al., 2017), and HICU-1 (Sullivan et al., 2020). These enable a continuous comparison of Cu isotope amount ratios in samples with NIST SRM976 for solution Cu isotope analyses. However, the lack of a suitable calibration standard is no doubt limiting widespread application of LA-MC-ICP-MS for in situ Cu isotope analysis of native Cu ores and artefacts.

In this contribution, we investigated the suitability of four Cu metal materials SSC-1, SSC-3, SSC-4 and CUPD-1 as Cu isotope reference materials for LA-MC-ICP-MS analysis. These materials were all certified by the Canada Centre for Mineral and Energy Technology (CANMET) as reference materials for trace element analysis of impurities in Cu. The Cu isotopic composition and homogeneity of these four new reference materials were characterised by SN- and LA-MC-ICP-MS, and are reported for the first time. The bulk Cu isotopic composition from dissolutions of 0.15–1.95 g size fragments of each of the reference materials was measured against NIST SRM976 and ERM®-AE633 Cu isotope standards by SN-MC-ICP-MS. Multiple individual in situ Cu isotope measurements were made by LA-MC-ICP-MS analysis of each of four new reference materials at 43 µm spot size, using each of other three reference materials as a calibration standard. These measurements allowed assessment of the Cu isotope homogeneity of the four new reference materials at the sampling scale using statistical approaches, including graphical assessment of normal distribution quantile-quantile plots, and the Shapiro-Wilk (Shapiro and Wilk, 1965, 1968; Royston, 1982), Jarque-Bera (Jarque and Bera, 1980, 1987) and reduced chi-squared statistic (mean square weighted deviation (MSWD); Wendt and Carl, 1991; Ludwig, 2003) tests, as well as one-way analysis of variance (ANOVA). Furthermore, the effects of laser induced isotopic fractionation of Cu during ns pulse LA-MC-ICP-MS isotopic analysis were investigated and the achievable precision and accuracy of in-situ Cu isotope measurements of native Cu were evaluated. Finally, the suitability of the Cu isotope reference materials was further validated by analysis of Cu isotope reference materials ERM®-AE647 and Romil Cu by SN-MC-ICP-MS, and a native copper sample, NMC 12864, by LA-MC-ICP-MS.

Materials and Methods

Standards and Samples

Two calibration standards were used for SN-MC-ICP-MS Cu isotope analysis. They were a solution Cu isotope standard NIST SRM976 prepared by digestion of a 0.4 g aliquot of the Cu metal standard at Queens University, Canada, and Institute for Reference Materials and Measurements (IRMM) ERM®-AE633 solution Cu isotope reference material. NIST SRM976 is certified for its absolute isotopic abundance ratio (⁶³Cu/⁶⁵Cu = 2.2440 ± 0.0021; Shields et al., 1964; Shields et al., 1965; Thomas, 1994). ERM®-AE633 was reportedly prepared directly from NIST SRM976 by the IRMM and has a nominally identical Cu isotopic composition (δ⁶⁵Cu value of NIST SRM976 Cu relative to ERM®-AE633 = −0.01 ± 0.05‰) (Moeller et al., 2012).

The solution Zn isotope standard NIST SRM683, originally developed as a certified reference material for trace element analysis (Rasberry, 1988) and newly developed as a Zn isotopic reference material (Yang L. et al., 2018), was used for instrumental mass bias correction. Its isotope composition relative to JMC-Lyon, the Zn isotope reference material against which Zn isotope data are commonly reported (Maréchal et al., 1999), is δ⁶⁶Zn = 0.12 ± 0.04‰ (Yang Y. et al., 2018). The Zn isotope reference material JMC‐Lyon, obtained from Johnson Matthey^©, London United Kingdom, is nearly exhausted and no longer produced. Its δ⁶⁶Zn = −0.29 ± 0.05‰ relative to IRMM-3702 (Moeller et al., 2012), where the IRMM-3702 is an available Zn isotope reference material from IRMM and is certified for absolute isotopic abundance ratios. The certified Zn isotope composition of IRMM-3702 is ⁶⁶Zn/⁶⁴Zn = 0.56397 ± 30 (Ponzevera et al., 2006). Thus, the calculated ⁶⁶Zn/⁶⁴Zn ratio of NIST SRM683 used in this study is 0.56388. Additionally, the Cu isotope reference material ERM®-AE647 Cu from IRMM and a commercial single-element Cu solution, Romil Cu (Romil Ltd., Cambridge, United Kingdom), that has been widely characterised for its Cu isotopic composition (Zhu et al., 2000; Chapman et al., 2006; Peel et al., 2008; Larner et al., 2011; Moeller et al., 2012) were used for quality control of the SN-MC-ICP-MS Cu isotope analyses. The δ⁶⁵Cu values of ERM®-AE647 Cu and Romil Cu relative to NIST SRM976 Cu are 0.21 ± 0.04 and 0.18 ± 0.06‰, respectively (Moeller et al., 2012).

The four Cu metal materials being investigated as Cu isotope reference materials for LA-MC-ICP-MS analysis were all originally developed as certified reference materials for trace element analysis of impurities in Cu by the CANMET. These materials are no longer commercially available but significant quantities still exist for distribution¹. SSC-1, SSC-3, and SSC-4 are commercial purity Cu metal rods. Weighed quantities of several elements were added to high-purity Cu anode swarf. The mixture was cold-pressed into pellets that were added to melted cleaned cathode Cu. The melt was poured into a mould and subsequently hot rolled into 8 mm diameter copper rods (Malson, 1975). Trace element contents range from 40 to 90 μg g⁻¹ for Sn and Pb in SSC-1, Fe and Ni in SSC-3, and S and Fe in SSC-4; other trace elements that occur at significant levels (10–35 μg g⁻¹) are S, Fe, Ni, and Zn in SSC-1, S, Zn, Ag, and Sn in SSC-3, and Ni, Zn, Ag, Cd, Sn, Sb, and Pb in SSC-4 (Malson, 1975).

CUPD-1 consists of sawings (60 μm to hundreds μm in diameter) of Cu anode (Salley and Leaver, 2009). It contains impurities of several trace elements, including, most importantly, Ni, As, Se, Ag and Sb in the range of 150–310 μg g⁻¹, and Fe, Te, Pb, and Bi with contents in the range of 40–69 μg g⁻¹ (Salley and Leaver, 2009); all other elements documented in the four Cu isotope reference materials occur at <10 μg g⁻¹ (Malson, 1975; Malson, 2003; Cooper et al., 2008; Salley and Leaver, 2009). In addition, a native copper sample, NMC 12864, originating from the Coppermine River area, Northwest Territories, Canada, and sampled from the National Mineral Collection (NMC), Geological Survey of Canada, was also used for this study. The impurities in this sample include Hg (0.4–4.9 μg g⁻¹), Zn (3–8 μg g⁻¹), Ni (7–21 μg g⁻¹), As (12–45 μg g⁻¹), Ag (146–333 μg g⁻¹) and Sn (9–163 μg g⁻¹), and low trace amounts (0.01–1.25 μg g⁻¹) of Co, Se, Sb, and Au (Cooper et al., 2008).

An aliquot of SSC-1, SSC-3, and SSC-4 (1.62–1.95 g) were cut from the Cu rods and polished to a 0.25 μm finish to remove the sawn surfaces. The CUPD-1 sawings (0.15 g) were checked and handpicked under a binocular microscope. These reference materials were rinsed three times with Milli-Q water and ethyl alcohol (C₂H₅OH) in turn, followed by three 15 min rounds of ultrasonic cleaning in each of ethyl alcohol and Milli-Q water. The materials were then rinsed three more times with Milli-Q water, and dried in a fume hood. Each of the reference materials was carefully weighed and placed in a 50 ml polytetrafluoroethylene (PTFE) beaker. The reference materials were digested using 9 ml of 8 M HNO₃ followed by 3.1 ml of 16 M HNO₃. The solutions were reduced to dryness on a hot plate at 100°C and then re-dissolved to produce 1,000–4,000 μg g⁻¹ stock solutions in 0.4 M HNO₃. All reagents used in this study were prepared using Optima™ high purity HNO₃ from Fisher Scientific and >18 MΩ⋅cm deionized Milli-Q water.

For laser ablation analysis, two 25 mm round epoxy mounts were prepared, each containing an aliquot of each of the four reference materials. Another mount was prepared containing sample NMC 12864. The mounts were polished to a 0.25 μm finish. The sample mounts were then rinsed with Milli-Q water and cleaned in an ultrasonic bath for 15 min in Milli-Q water and ethyl alcohol successively. The surface of the mount was cleaned using ethyl alcohol immediately before LA-MC-ICP-MS Cu isotope analysis.

Instrumentation and Data Acquisition

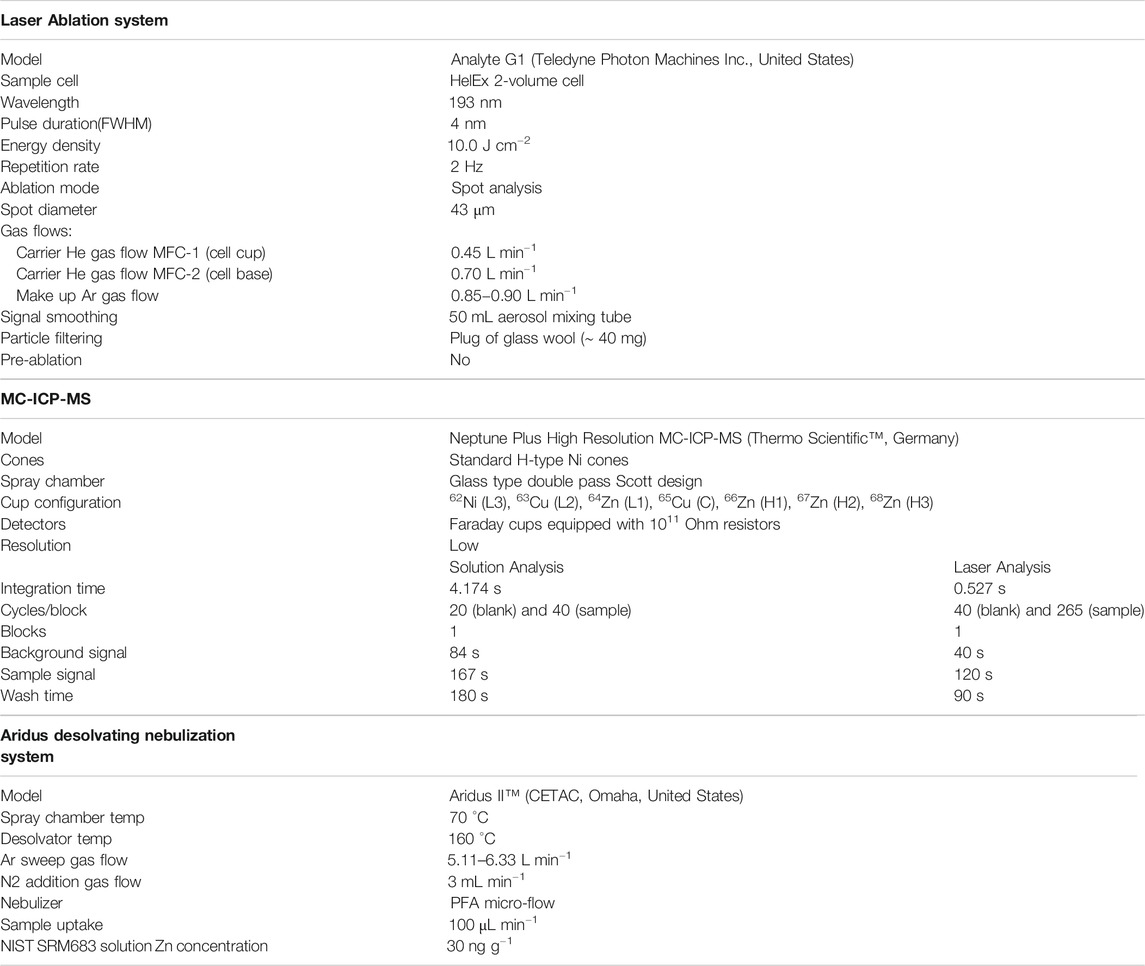
Copper isotope ratio measurements were performed using SN- and LA-MC-ICP-MS at the Geological Survey of Canada (GSC), Natural Resources Canada, in Ottawa, using a Thermo Scientific™ Neptune Plus™ High Resolution MC-ICP-MS. The SN Cu isotope measurements were performed in “wet plasma” mode. For laser ablation analysis, the MC-ICP-MS was coupled to a Teledyne Photon Machines Analyte G1 excimer laser ablation system (λ = 193 nm). Complete SN- and LA-MC-ICP-MS operating conditions are provided in Table 1. An electronic baseline measurement of 120 s and a Faraday collector gain calibration were performed daily before Cu isotope measurements started. The standard-sample-standard bracketing (SSB) external standardisation technique, in conjunction with Zn internal normalization (Maréchal et al., 1999; Maréchal and Albarède, 2002; Graham et al., 2004; Mason et al., 2004a; Maher, 2005; Yang L. et al., 2018), were used to correct for instrumental mass bias for both SN and LA analyses.

TABLE 1

TABLE 1. SN- and LA-MC-ICP-MS operating conditions.

For SN-MC-ICP-MS Cu isotope analysis, the bracketing calibrator and sample solutions were diluted to 100 ng g⁻¹ Cu in 2% HNO₃ and doped with Zn (NIST SRM683) to yield analysis solutions with 1:1 Cu:Zn concentration ratio. This ensures similar Cu and Zn ion signal intensities, and minimizes differences in isotope fractionation behaviour, which appears to be caused by space-charge effects in the interface region (Archer and Vance, 2004), and results in reduced errors in the mass bias estimation (Zhu et al., 2000; Archer and Vance, 2004). Each Cu isotope measurement consisted of 20 cycles and 40 cycles of 4.174 s integration time for the analyses of acid blank (2% HNO3) and Cu solutions, respectively. The analytical sequence was: blank, standard, blank, sample, blank, standard, blank, sample, etc. The instrument was flushed for 3 min using 2% HNO₃ after each analysis. The total Cu signal intensity for the acid blank background was 4–6 mV for ⁶³Cu and ⁶⁵Cu and total Zn signal intensity was 4.0–5.0 mV for ⁶⁴Zn and ⁶⁶Zn. The total Cu signal intensity was 6.0–10 V for 100 ng g⁻¹ Cu; the total Zn signal intensity was 2.7–3.5 V for 100 ng g⁻¹ Zn in different analytical sessions. Each of the SSC-1, SSC-3, SSC-4, and CUPD-1 reference materials, along with the quality control Cu isotope reference material ERM®-AE647, were measured repeatedly in multiple analytical sessions using either NIST SRM976 or ERM®-AE633 as the Cu isotope calibration standard. The Cu isotope reference materials ERM®-AE647 and Romil Cu were also measured in different analytical sessions using SSC-1, SSC-3, SSC-4 or CUPD-1 as the Cu isotope calibration standard. Analyses with values outside of the mean ± 2 s.d. were repeated. The solution analyses were conducted over a period of a month.

Laser ablation sampling for MC-ICP-MS Cu isotope analysis was performed in a He atmosphere, which reduces dramatically the condensation blanket of material around the ablation site (Guillong and Günther, 2002) and improves the transport efficiency. All analyses were accomplished using 43 µm spots, a low laser repetition rate (2 Hz) and high laser fluence (10 J cm⁻²) to minimise down-hole laser-induced isotopic fractionation (Jackson and Günther, 2003). The ablation aerosol was first passed through a 50 ml volume test tube to smooth the signal. The aerosol was then passed through approximately 40 mg of glass wool (“glass wool superfine,” No. 41408002, Hecht-Assistent, Sondheim/Rhön, Germany) that was inserted into the 3.2 mm i. d. sample delivery tubing, creating an approximately 1.5 cm long plug. The glass wool filter preferentially filters larger (> 0.5 μm) aerosol particles that are too large to be quantitatively volatilised in the ICP and can result in noisy signals and severe isotopic fractionation (Jackson and Günther, 2003). The glass wool filter was replaced regularly (∼ 5 h for a maximum of 76 spot analyses) in order to minimise any cross contamination of the aerosol from particulates of completed analyses. A “semi-dry” aerosol of NIST SRM683 Zn, generated by an Aridus II™ desolvating nebulization system (CETAC, Omaha, United States), was added to the filtered ablation aerosol via a Y-shape connector placed between the glass wool filter and the ICP torch. The instrument was flushed for 90 s after each analysis. The total signal intensity for the gas background during LA-MC-ICP-MS analysis was ca. 3–5 mV for ⁶³Cu and ⁶⁵Cu. The total Zn background signal intensity was ca. 2–6 mV for ⁶⁴Zn and ⁶⁶Zn, respectively. The total Zn signal for 30 ng g⁻¹ Zn was 4.6–5.4 V. The total Cu signal during ablation typically dropped from ca. 18–3.0 V during 120 s ablation time, for a mean of ca. 12 V. Each of SSC-1, SSC-3, SSC-4, and CUPD-1 was measured repeatedly using each of the other three materials, in turn, as a bracketing calibration standard. Analyses with values outside of the mean ± 2 s.d. were repeated. Laser ablation analyses were conducted over a period of two months.

Data Reduction

Processing of the SN- and LA-MC-ICP-MS Cu isotope analysis data was performed in MS Excel spreadsheets. The time-resolved laser ablation signals were examined and signal intervals for integration were carefully selected. In most cases, the absolute variation of ⁶⁵Cu/⁶³Cu ratios for the individual measurement cycles was < 0.0005 and almost all of the acquired data (ca. 115 s of 120 s) were integrated. The raw Zn isotope signals, together with the calculated ⁶⁶Zn/⁶⁴Zn ratios, were also examined to ensure that there were no anomalous variations that might indicate localised differences in Zn contribution from extraneous sources, such as the samples being tested. The effect of the interference of ⁶⁴Ni on ⁶⁴Zn was also evaluated. The acid blank (2% HNO₃)-corrected ⁶²Ni⁺ signals for SN-MC-ICP-MS analyses were < 0.09 mV; thus ⁶⁴Ni/⁶⁴Zn signal intensity ratios were < 0.0014%. The gas blank-corrected ⁶²Ni⁺ signals for LA-MC-ICP-MS were < 0.17 mV; thus ⁶⁴Ni/⁶⁴Zn signal intensity ratios were < 0.0022%. With ⁶⁴Zn⁺ signals 45,000 times higher than ⁶⁴Ni⁺ signal, the interference of ⁶⁴Ni⁺ on ⁶⁴Zn⁺ was deemed insignificant.

The acid or gas blank-corrected signal intensities for ⁶³Cu⁺ and ⁶⁵Cu⁺, along with signal intensities for ⁶⁴Zn⁺ and ⁶⁶Zn⁺, were used to determine the ⁶⁵Cu/⁶³Cu and ⁶⁶Zn/⁶⁴Zn isotope amount ratios for each acquisition cycle for the sample and bracketing calibrators. Then, the instrument mass bias factor (f^Zn) was calculated for both unknown samples and bracketing calibrators, using the exponential mass bias law, a ⁶⁶Zn/⁶⁴Zn isotope amount ratio of 0.56388 for NIST SRM683 and atomic mass data published by IUPAC (http://www.ciaaw.org/atomic-masses.htm, 2018). The determined f^Zn was then applied to exponentially correct the measured ⁶⁵Cu/⁶³Cu isotope amount ratios for each analytical cycle of respective samples and bracketing calibrators.

In order to develop the most robust data processing procedure for LA-MC-ICP-MS Cu isotope analysis, the effect of removing individual outliers was evaluated by processing the data with and without outlier removal. Outliers were defined as a ⁶⁵Cu/⁶³Cu amount ratio for an individual cycle of a single analysis that deviated from the range of mean ± 2 sigma. The results from 707 analyses of SSC-1, SSC-3, SSC-4, and CUPD-1 show that an absolute difference between measured ⁶⁵Cu/⁶³Cu isotope amount ratios with and without outlier removal are 0.000003 for 80.2% analyses, 0.000008 for 15% analyses and 0.000016 for 3.4% analyses respectively. This indicates that measured ⁶⁵Cu/⁶³Cu isotope amount ratios obtained with outlier removal are not significantly different from those without outlier removal in most instances. In contrast to the results described above, only 1.4% of total analyses display significant differences (ranging from 0.000029 to 0.0030 absolute difference) between outlier-filtered and unfiltered Cu isotope amount ratios. Inspection of these significant outliers reveals that they are all clearly related to spikes in ⁶⁵Cu/⁶³Cu that appeared randomly in analyses and affected one cycle to three cycles of data. These spikes, which are commonly observed in LA analyses, were most likely induced by large ablated particles, not other instrument noise. Such spikes are probably related to release from the glass wool of large particles that are incompletely vaporized in the ICP-MS, resulting in ionization of fractionated Cu vapour.

This study demonstrates that for 98.6% of the analyses, the approach of outlier removal yields insignificant difference in the measured ⁶⁵Cu/⁶³Cu isotope amount ratios from the values for the same analyses not subjected to outlier removal. However, outlier analysis is considered critical for detecting and removing significant, erratic, analytical spikes during the analyses and thus ensuring a robust procedure for accurate and precise Cu isotope ratio measurements. Therefore, any outliers at the 2 sigma confidence level in the mass bias-corrected cycle values were discarded, both for SN and LA analyses. This resulted in filtering of 0 cycles to 4 cycles of data for SN analyses (40 cycles) and 2 cycles to 15 cycles for LA analyses (maximum 221 cycles).

Finally, the mean of the outlier-filtered, mass bias-corrected Cu isotope ratios of an unknown sample was further corrected by application of the SSB procedure to account for additional analytically-induced isotopic fractionation (e.g., laser-induced). The Cu mass bias factor (f^Cu) was determined using the exponential mass bias law and the true ⁶⁵Cu/⁶³Cu isotope amount ratio of the bracketing calibrator. The mean of the factors f^Cu obtained for the two adjacent bracketing calibrators was then employed to achieve correction of additional isotopic fractionation and to calculate the mass bias-corrected ⁶⁵Cu/⁶³Cu amount ratio of an unknown sample. The determined absolute ⁶⁵Cu/⁶³Cu amount ratio of each unknown sample was then converted to standard delta notation (δ⁶⁵Cu units), where δ⁶⁵Cu is relative difference in per mil (‰) of the ⁶⁵Cu/⁶³Cu amount ratio of the sample and the NIST SRM976 standard (Shields et al., 1964; Shields et al., 1965) (Eq. 1), having a certified value of ⁶⁵Cu/⁶³Cu_SRM976 = 1/2.2440 = 0.44563.

${\delta }^{65}Cu = \left(\frac{{}^{65}Cu/{}^{63}C{u}_{sample}}{{}^{65}Cu/{}^{63}C{u}_{\mathit{standard}}} -1\right) (1)$

The uncertainties, contributed from the mass correction factor, the true Cu isotope amount ratio in the bracketing calibrator, and normalization standard NIST SRM976, were estimated using the rule for propagation of errors. The combined uncertainty associated with the reported δ⁶⁵Cu value was estimated according to JCGM 100, 2008 “GUM 1995 with minor corrections,” and in consideration of type A major uncertainty components associated with the measurement of δ⁶⁵Cu value, including the standard uncertainties from an unknown sample and the calibration standard. Considering Eq. 1 and using the rules for propagation of uncertainty for uncorrelated input quantities, the square of combined uncertainty U² can be expressed as Eq. 2:

$U^2\left({\mathrm{\delta }}^{65}\mathrm{Cu}\right)=\frac{1}{R_{standard}^2} \times U_{sample}^2+\frac{R_{sample}^2}{R_{standard}^4}\times U_{standard}^2 (2)$

where, U_sample and U_standard are standard measurement uncertainty (standard deviation) of ⁶⁵Cu/⁶³Cu amount ratios of an unknown sample and the calibration standard, respectively, and R_sample and R_standard are mass bias corrected ⁶⁵Cu/⁶³Cu amount ratios of an unknown sample and the calibration standard, respectively. The expanded uncertainty can then be obtained by multiplying U by a coverage factor k = 2, which gives a confidence level of 95% (JCGM 100: 2008).

A graphical assessment of normal distribution by quantile-quantile plots [the quantiles of the sample data (ordered by δ⁶⁵Cu values) vs. the theoretical quantile values for a normal distribution] and a Jarque-Bera statistic test for normality were completed in MS Excel spreadsheets. A Shapiro-Wilk test for normality and one-way analysis of variance (ANOVA) were conducted using the IBM SPSS Statistics software version 26. Calculation of the reduced chi-squared statistic, also known as mean square weighted deviation (MSWD), was performed using Isoplot 3.00: a Geochronological Toolkit for Microsoft Excel (Ludwig, 2003).

Results and Discussion

Effects of Matrix in SN-MC-ICP-MS

On the basis of the certified element concentrations in the Cu reference materials from previous studies (Malson, 1975; Malson, 2003; Cooper et al., 2008; Salley and Leaver, 2009), the determined concentrations of impurity trace elements such as S, Mn, Fe, Ni, Zn, Se, As, Ag, Cd, Sn, Sb, Te, Au, Pb, and Bi in the 100 ng g⁻¹ Cu solutions of the reference materials were in the range of ≤ 0.031 ng g⁻¹. The concentrations of other impurity elements in the same solutions determined in this study using a Thermo X-Series II quadrupole ICP-MS were < 6 ng g⁻¹ for Na, Mg, Al and Ca, and < 0.5 ng g⁻¹ Ti, V, Cr, and Ba. Therefore, the calculated concentration ratios of most impurity elements, relative to Cu, in the pure copper reference materials were in the range of < 0.0003; and in the range of < 0.1 for Mg, Al, S and Ca. These concentration ratios are in the range that are reported to produce insignificant matrix related biases according to studies on matrix effects in SN-MC-ICP-MS Cu isotope measurement. These include the effects of Fe (Zhu et al., 2002; Graham et al., 2004; Rouxel et al., 2004; Maher, 2005; Markl et al., 2006; Maher and Larson, 2007), S (Rouxel et al., 2004), Zn (Graham et al., 2004; Maher, 2005), Ni (Graham et al., 2004; Maher, 2005), Na (Zhu et al., 2002; Savage et al., 2015); Mg (Zhu et al., 2002). Furthermore, given the low levels of impurities and low production rate of polyatomic ion interferences in ICP-MS, potential polyatomic interferences from impurity elements in 100 ng g⁻¹ Cu solutions (e.g., ²³Na⁴⁰Ar⁺, ²⁷Al³⁶Ar⁺, ⁴⁷Ti¹⁶O⁺, ¹²⁶Te²⁺ on ⁶³Cu⁺; ²⁴Mg⁴⁰Ar⁺, ²⁸Si³⁶Ar⁺, ³²S₂⁺, ⁴⁸Ca¹⁶O⁺, ⁴⁸Ti¹⁶O⁺, ¹²⁸Te²⁺ on ⁶⁴Zn⁺; ²⁵Mg⁴⁰Ar⁺, ²⁷Al³⁸Ar⁺, ²⁹Si³⁶Ar⁺, ³²S³³S⁺, ⁴⁹Ti¹⁶O⁺, ¹³⁰Te⁺, and ¹³⁰Ba²⁺ on ⁶⁵Cu⁺; ²⁶Mg⁴⁰Ar⁺, ³⁰Si³⁶Ar⁺, ³²S³⁴S⁺, ⁵⁰Ti¹⁶O⁺, ⁵⁰Cr¹⁶O⁺, and ¹³²Ba²⁺ on ⁶⁶Zn⁺) are considered to have been negligible.

The effect of Zn present in SSC-1, SSC-3, SSC-4 and CUPD-1 (Malson, 1975; Malson, 2003; Cooper et al., 2008; Salley and Leaver, 2009) on the accuracy of Zn isotope ratio measurement of NIST SRM683 in Cu isotope analysis of these reference materials by SN-MC-ICP-MS was also assessed. There was ca. 0.0015–0.0033 ng g⁻¹ Zn impurity in the 100 ng g⁻¹ Cu solution of those isotope reference materials spiked with 100 ng g⁻¹ Zn NIST SRM683. Applying isotopic mixture theory (Faure and Mensing, 2005), the Zn isotopic composition in 100 ng g⁻¹ Cu and Zn solutions can be considered a mixture of two Zn isotope components, which have different ⁶⁶Zn/⁶⁴Zn isotope amount ratios and different Zn concentrations, including 100 ng g⁻¹ NIST SRM683 Zn solution, and impurity Zn in 100 ng g⁻¹ Cu solution of the Cu isotope reference materials. If it is assumed that the Zn isotope composition of the Zn impurity in each Cu isotope reference material has the most extreme value reported for terrestrial samples (δ⁶⁶Zn_JMC-Lyon = 2.0‰; Moynier et al., 2017), the calculated ⁶⁶Zn/⁶⁴Zn isotope composition of the Zn impurity in the Cu isotope reference materials would be 0.564934. Thus, the ⁶⁶Zn/⁶⁴Zn isotope composition of the Zn isotope mixture solution, calculated using the equation of isotope ratio mixtures (Faure and Mensing, 2005), is nominally identical to the 0.563880 value of NIST SRM683 with an absolute relative difference < 0.000007%. Therefore, Zn impurities in the reference materials are expected to have produced no measurable effect on the measured ⁶⁶Zn/⁶⁴Zn isotope composition of NIST SRM683 in SN analyses and subsequently no significant bias in the mass bias correction factors for the Cu isotope measurements.

Solution Nebulization MC-ICP-MS Measurements

The measured δ⁶⁵Cu_SRM976 value for Cu isotope standard solution ERM®-AE647, analysed for quality control by SN-MC-ICP-MS, was 0.20 ± 0.07‰ (U, k = 2, n = 26), whereas the measured δ⁶⁵Cu_AE633 value was 0.20 ± 0.06‰ (U, k = 2, n = 101). These results, shown in Figure 1; Table 2, are in excellent agreement with previously reported values of δ⁶⁵Cu_SRM976 = 0.21 ± 0.04‰ (2 s.d., n = 60) and δ⁶⁵Cu_AE633 = 0.20 ± 0.04‰ (2 s.d., n = 60) (Moeller et al., 2012).

FIGURE 1

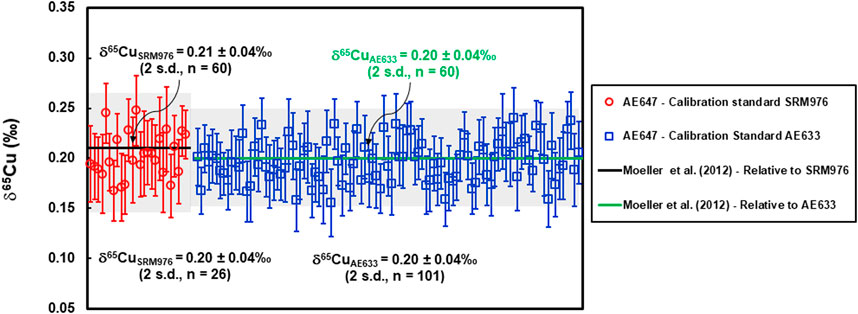
FIGURE 1. Cu isotope determinations of certified reference material ERM®-AE647. The determined δ⁶⁵Cu values, measured against NIST SRM976, were consistent during one analytical session in February 2019, and agree within uncertainty with the certified δ⁶⁵Cu_SRM976 value and precision from Moeller et al. (2012) (as indicated by a solid black line and shaded area). The determined δ⁶⁵Cu values against ERM®-AE633 were consistent over three analytical sessions, measured from September 2018 to February 2019 and also agree well within the uncertainty with the certified δ⁶⁵Cu_AE633 values and precision from Moeller et al. (2012) (as indicated by a solid green line and shaded area). Precision of a single measurement is shown at 2 standard errors (number of measurement cycles, n = 40).

TABLE 2

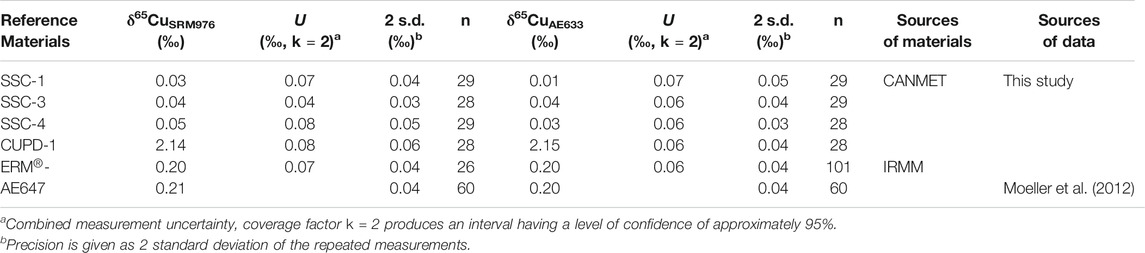
TABLE 2. δ⁶⁵Cu values of reference materials ERM®-AE647, SSC-1, SSC-3, SSC-4 and CUPD-1 determined by SN-MC-IC-MS using NIST SRM976 and ERM®-AE633 as calibration standards.

The measured δ⁶⁵Cu_SRM976 values of the SSC-1, SSC-3, SSC-4, and CUPD-1 solutions, determined with NIST976 as a calibration standard, were 0.03 ± 0.07‰ (U, k = 2, n = 29), 0.04 ± 0.04‰ (U, k = 2, n = 28), 0.05 ± 0.08‰ (U, k = 2, n = 29) and 2.14 ± 0.08‰ (U, k = 2, n = 28), respectively (Figure 2A; Table 2). The measured δ⁶⁵Cu_AE633 values of the same solutions, determined with solution ERM®-AE633 as a calibration standard, were 0.01 ± 0.07‰ (U, k = 2, n = 29), 0.04 ± 0.06‰ (U, k = 2, n = 29), 0.03 ± 0.06‰ (U, k = 2, n = 28) and 2.15 ± 0.06‰ (U, k = 2, n = 28), respectively (Figure 2B; Table 2).

FIGURE 2

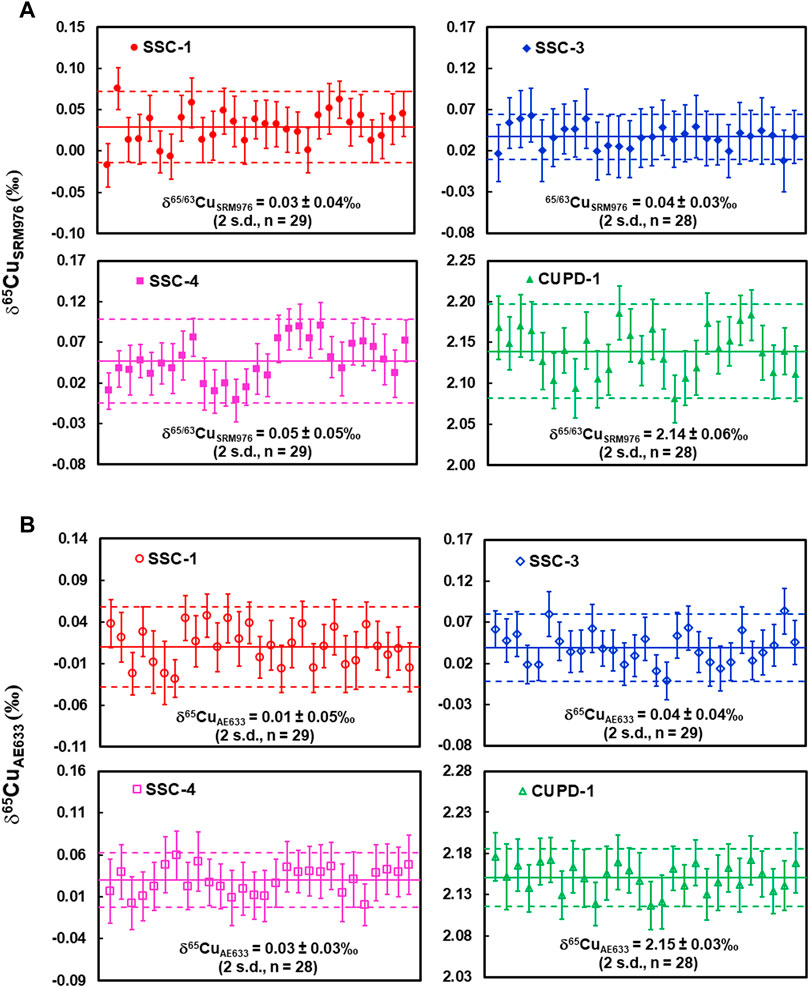
FIGURE 2. (A) SN-MC-ICP-MS Cu isotope determinations of reference material SSC-1, SSC-3, SSC-4 and CUPD-1 against NIST SRM976; (B) SN-MC-ICP-MS Cu isotope determinations of reference material SSC-1, SSC-3, SSC-4 and CUPD-1 against ERM®-AE633. Measurement precision is indicated by dashed lines. For a single measurement, the within-run precision was 0.03% (2 standard error; number of measurement cycles n = 40).

The measured δ⁶⁵Cu_AE633 values of the new Cu isotope reference materials and reference material ERM®-AE647, determined using ERM®-AE633 as a calibration standard, were recalculated as δ⁶⁵Cu_SRM976 values on the basis that the relative difference in δ⁶⁵Cu of NIST SRM976 and ERM®-AE633 = −0.01 ± 0.05‰ (2 sd., n = 40) (Moeller et al., 2012). These values were then plotted against the δ⁶⁵Cu_SRM976 values for the same materials using NIST SRM976 as a calibration standard (Figure 3). The Cu isotope data overlap the 1:1 relationship within uncertainty, suggesting that measured relative Cu isotope amount ratios for each of the new Cu isotope reference materials and reference material ERM®-AE647, using ERM®-AE633 as a calibration standard are identical at the 95% confidence level to the ratios determined using NIST SRM976 as a calibration standard. Moreover, the δ⁶⁵Cu values for Cu isotope standard solution ERM®-AE647 and Romil Cu, determined using each of the newly developed reference materials, SSC-1, SSC-3, SSC-4 and CUPD-1, as a calibration standard (Supplementary Figure S1A; Figure 1B; Supplementary Table S1), show that mean measured δ⁶⁵Cu of ERM®-AE647 was 0.18 ± 0.06‰ (2 s.d., n = 69), which is in good agreement with the certified δ⁶⁵Cu values 0.21 ± 0.04‰ (2 s.d., n = 60; Moeller et al., 2012). The mean measured δ⁶⁵Cu value of Romil Cu was 0.18 ± 0.07‰ (2 s.d., n = 68), and is also in good agreement with the reported δ⁶⁵Cu of 0.18 ± 0.06‰ (2 s.d., n = 19) and 0.21 ± 0.08‰ (2 s.d., n = 69) obtained in two different laboratories (Moeller et al., 2012).

FIGURE 3

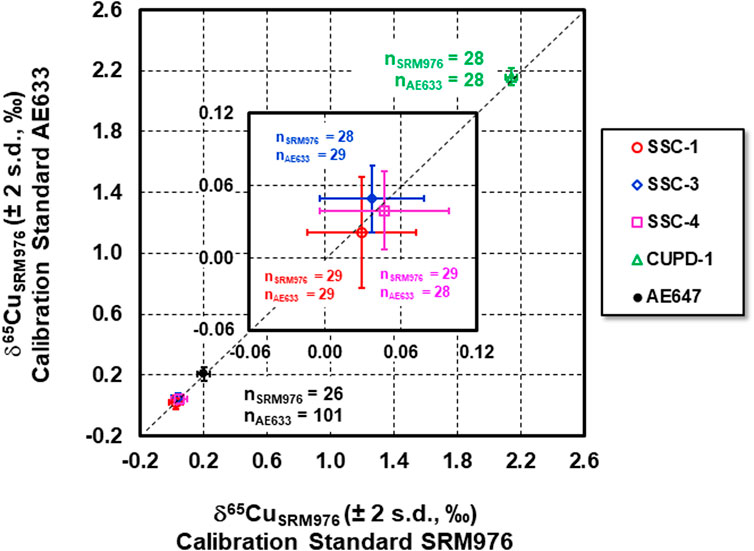
FIGURE 3. δ⁶⁵Cu_SRM976 values of reference materials SSC-1, SSC-3, SSC-4, CUPD-1 and ERM®-AE647 determined using calibration standard NIST SRM976 vs. using ERM®-AE633.

For relative Cu isotope amount ratio measurements by SN-MC-ICP-MS, a long term measurement precision was ± 0.04‰ (2 standard deviation, n = 101 over 1 month), estimated based on analyses of the certified reference materials ERM®-AE647. For a single measurement, the precision was ± 0.03‰ (2 standard error; number of cycles = 40), estimated based on analyses of reference material ERM®-AE647 in this study. The long term and single measurement precision achieved in this study are comparable to the reported precisions of ± 0.05‰ (n = 60) and ± 0.05‰ (n = 40), respectively (Moeller et al., 2012).

Effects of Laser-Induced Cu Isotopic Fractionation

During LA-MC-ICP-MS analysis, the raw and mass bias-corrected ⁶⁵Cu/⁶³Cu absolute isotope ratios were typically relatively stable during the 120 s data acquisition times (Figures 4A,B), whereas the ⁶³Cu⁺ and ⁶⁵Cu⁺ signals were typically stable for the first 35 s of ablation, following which signal strength decreased progressively during the subsequent 85 s (Figure 4B). These observations, which are consistent with the study of Jackson and Günther (2003), suggest that ablation time-dependent fractionation of Cu isotope ratios during the course of a ns laser ablation spot analysis was substantially avoided by aerosol filtering. The magnitude of Cu isotope variation during the whole data acquisition in the example shown in Figure 4 was 0.03‰.

FIGURE 4

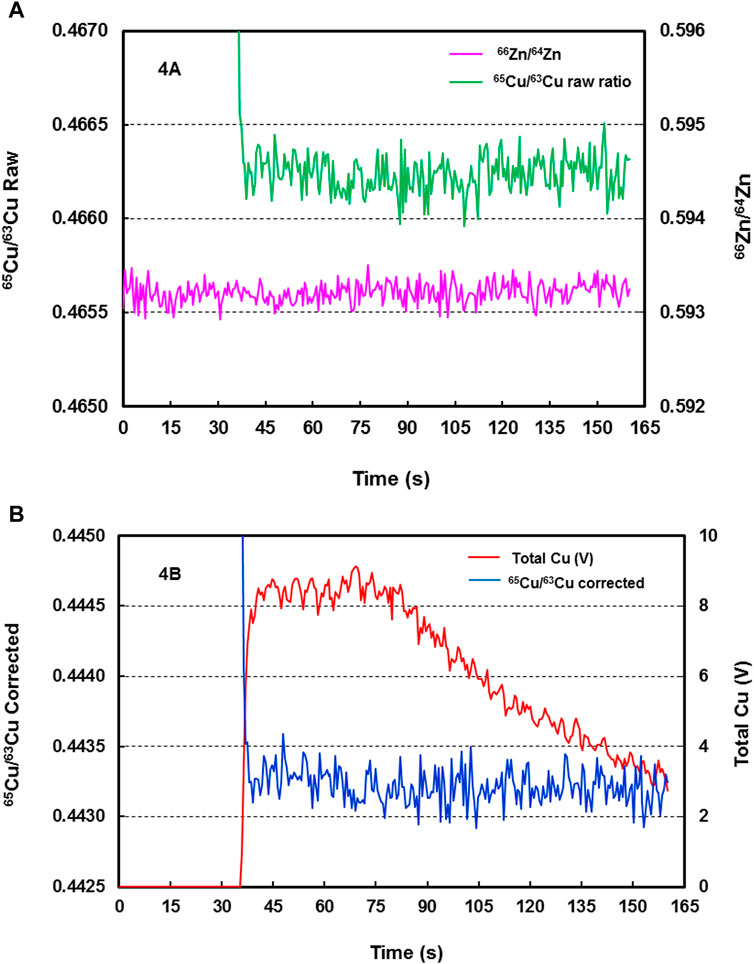
FIGURE 4. (A) Raw time-resolved ⁶⁵Cu/⁶³Cu isotope ratios (green line) and ⁶⁶Zn/⁶⁴Zn ratios (pink line) from LA-MC-ICP-MS analysis of reference material SSC-1 (DE06B22 analysis). Zn was added to the sample carrier gas as a dry aerosol. The relatively stable Cu isotope ratios indicate that substantial ablation time-dependent fractionation of Cu isotope ratios during the course of a ns laser ablation spot analysis was largely avoided by aerosol filtering. (B) Time resolved ⁶⁵Cu/⁶³Cu ratios corrected (blue line) for mass bias from LA-MC-ICP-MS analysis of reference material SSC-1 (DE06B22 analysis). The relatively stable Cu isotope ratios are entirely independent of the Cu signals (red line), and laser induced isotope fractionation was largely avoided by aerosol filtering.

It was also observed in our study that, under nominally identical laser ablation conditions, one analysis could display almost no ablation time-dependent fractionation of Cu isotope ratios during the whole period of ablation, whereas the next analysis could show obvious fractionation of Cu isotope ratios. These variations were consistently characterised by an increase of ∼ 0.08‰ in measured Cu isotope composition between the first and second 30 s of data. The cause(s) of this is not clear, but the consistent nature of the variations argues against isotopic heterogeneity of the sample. They are more likely associated with errors in laser focus position and/or fluctuations in laser output energy. Errors in the focus position would have resulted in a change of the pulse energy applied to the sample surface (Lazarov and Horn, 2015), which, combined with changes in laser output energy, could affect ns laser-copper interaction, and change the ablation efficiency due to the heat conduction losses and the plasma shielding effects (Semerok et al., 2002). It should be noted that even a within-run variation of 0.08‰ implies excellent performance when compared with Cu isotope variation of ca. 1.0‰ during a 90 s filtered ablation of pure copper using 213 ns LA analysis (Kuhn et al., 2007). Although this difference is not fully understood, it may be attributed to the entirely different LA and MC-ICP-MS hardware and settings employed in our study compared to those employed by Kuhn et al. (2007); for example, glass wool vs. centrifugal particle filtering, 2 vs. 5 Hz laser repetition rate and fluence of 10 vs. 17 J cm⁻², respectively. The latter two settings presumably resulted in large differences in crater aspect ratios. It should also be noted that, even analyses showing within run variations of up to 0.08‰ provided measured Cu isotopic compositions that were indistinguishable within analytical uncertainty from the composition of the SN analysis of the same reference material.

The relative difference between internally (Zn) normalised SN Cu isotope ratio measurements of NIST SRM976 and the true value were 1.1–1.7% (0.62–0.95‰ absolute positive offset, n = 74). For LA analysis of SSC-1 and SSC-4, this difference, which represents the bias not corrected using Zn internal normalization alone, were 11.2–12.4% (5.3–5.7‰ negative offset, n = 37). The magnitude of the internal (Zn) mass bias correction for LA analyses (2.5–2.7%) was similar to that for SN analyses (2.6–2.8%), implying that the mass discrimination taking place in the ICP-MS as a result of plasma load and space charge effects, was very similar for both sample introduction systems. Thus, we estimate that laser sampling-induced Cu isotopic fractionation represents ∼ 10% (absolute) of the total analytically-induced isotopic fractionation budget. The large discrepancy between the mass bias-corrected Cu isotope ratios in LA analysis and the target Cu isotopic composition observed in this study is consistent with the results of Jackson and Günther (2003), and highlights the inability of Zn internal normalization to correct for laser-induced isotopic fractionation. On the other hand, this study shows that the achievable measurement precision (2 s.d.) attained in LA Cu isotope analysis using the SSB mass bias correction only, is 5 times to 7 times worse than the 0.07‰ achievable measurement precision obtained for the same analyses (n = 35) using both internal normalisation and SSB mass bias corrections. This indicates that there were significant instrumental drift effects during LA Cu isotope analysis that cannot be corrected by SSB alone. Thus, the approach of combined internal Zn normalisation and SSB bracketing is demonstrated to provide a highly effective and robust technique for precise and accurate Cu isotope ratio measurement by ns pulse LA-MC-ICP-MS.

Homogeneity of the Reference Materials

Each of the reference materials was analyzed repeatedly using each of the three other reference materials as a calibrator. The Cu isotope homogeneity of the four new reference materials was assessed firstly by determining whether multiple individual in situ Cu isotope measurements made by LA-MC-ICP-MS analysis at 43 µm spot size approximated a single normal distribution. It was further assessed using one-way analysis of variance (ANOVA) to determine whether there are statistically significant differences between the mean δ⁶⁵Cu values of three independent data sets for each of the Cu isotope reference materials, SSC-1, SSC-3, SSC-4, and CUPD-1. The results of the homogeneity tests of the reference materials are summarized in Tables 3, 4.

TABLE 3

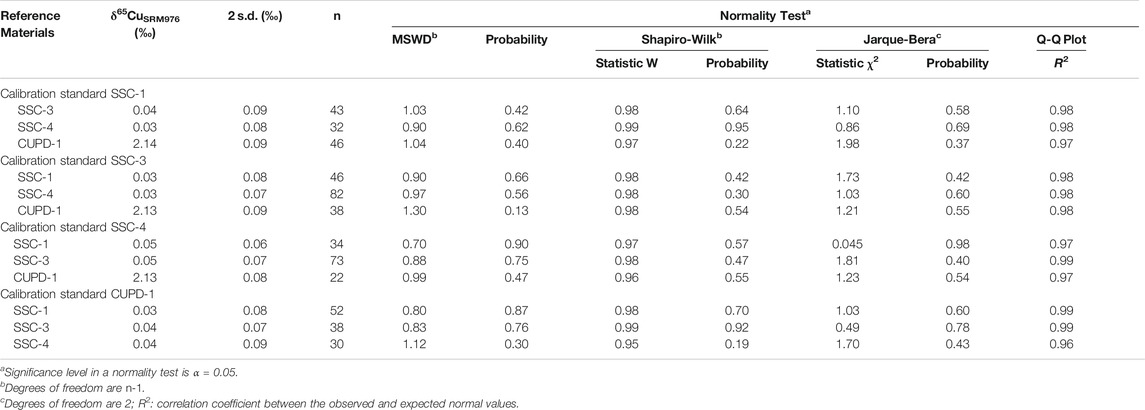
TABLE 3. Results of Cu isotope homogeneity tests for the reference materials SSC-1, SSC-3, SSC-4 and CUPD-1.

TABLE 4

TABLE 4. Results of one-way ANOVA.

Graphical assessment of normal distribution using quantile-quantile plots (Q-Q) in Figure 5 shows that the quantile data points for the determined relative Cu isotope amount ratios from each of the reference materials did not deviate seriously from the fitted straight 1:1 line, and have good linear correlation coefficients close to 1 (0.96–0.99).

FIGURE 5

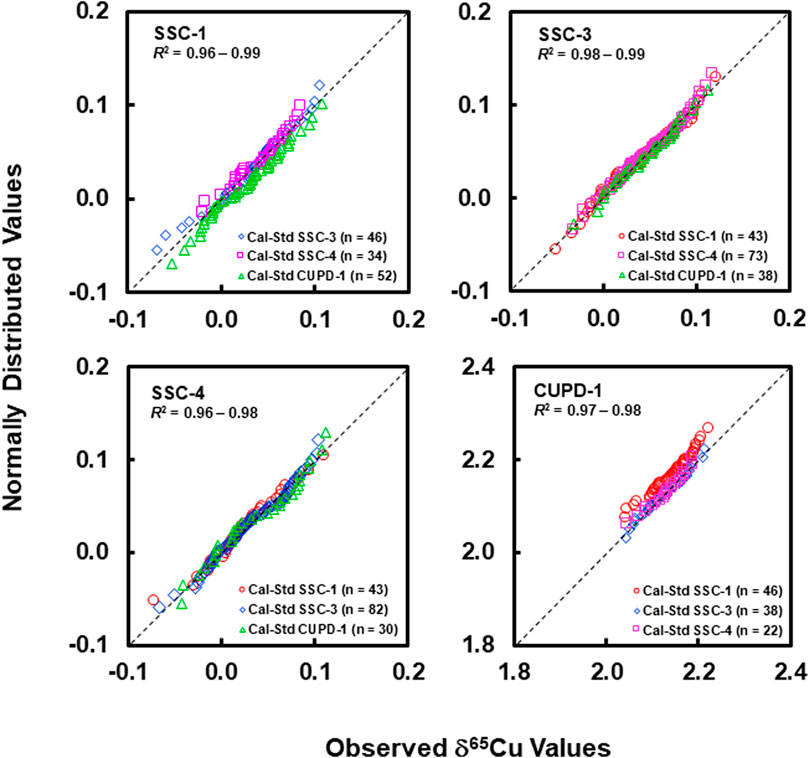
FIGURE 5. Normal quantile-quantile plots for SSC-1, SSC-3, SSC-4, and CUPD1 show that the quantile data points for the determined δ⁶⁵Cu values for each of the reference materials do not seriously deviate from the 1:1 line and have linear correlation coefficients close to 1.

Normality tests using the well-known Shapiro-Wilk test (Shapiro and Wilk, 1965, Shapiro and Wilk, 1968; Royston, 1982) yielded statistic W values in the range of 0.97–0.98 (probability: 0.42–0.70) for SSC-1, 0.98 to 0.99 (probability: 0.47–0.92) for SSC-3, 0.95 to 0.99 (probability: 0.19–0.95) for SSC-4 and 0.96 to 0.97 (probability: 0.22–0.55) for CUPD-1. Statistic W values close to 1, with corresponding probability values significantly greater than 0.05, indicate that the Cu isotope data acquired on each of SSC-1, SSC-3, SSC-4 and CUPD-1 reference materials did not significantly depart from normality with 95% confidence.

An alternative test of normality, the Jarque-Bera test (Jarque and Bera, 1980; Jarque and Bera, 1987), a procedure for skewness-kurtosis testing, gave χ² values in the range of 0.045–1.98 (probability: 0.37–0.78), which lies within the null hypothesis acceptance zone of less than the critical χ² value of 5.448 (for n < 100) of the chi-squared distribution with 2 degrees of freedom at significance level α = 0.05. This reveals that Cu isotope data acquired on each of the new reference materials do not deviate significantly from a normal distribution with 95% confidence.

The homogeneity of the reference materials was also evaluated using the reduced chi-squared statistic, or MSWD (Wendt and Carl, 1991; Ludwig, 2003), at the 95% confidence interval, a measure of goodness of fit that takes into account the effect of both the internal and external analytical uncertainties. An MSWD value of approximately 1 indicates that the scatter of the data for a reference material can be explained by analytical uncertainties alone, and that additional sources of uncertainty, such as heterogeneity of the sample material, do not exist within measurement uncertainty (Wendt and Carl, 1991; Gilbert et al., 2014). The determined MSWD values were in the range of 0.70–0.90 (probability: 0.66–0.90) for SSC-1, 0.83–1.03 (probability: 0.42–0.76) for SSC-3, 0.90–1.12 (probability: 0.30–0.56) for SSC-4, and 0.99–1.30 (probability: 0.13–0.47) for CUPD-1, respectively. The MSWD values are all close to 1 and within the expected acceptance ranges of 1 ± 2 (2/f)^1/2, where the degrees of freedom f = n–1 and n = the number of data points (Wendt and Carl, 1991), implying that the scatter of the Cu isotope data was due to the analytical uncertainty with 95% confidence, and no other significant sources of uncertainty were unaccounted for.

The homogeneity of the reference materials was further evaluated through one-way ANOVA to examine whether any of the three means of the relative Cu isotope ratios of a reference material measured using the other three reference materials as calibrators are different from each other with statistical significance. The results of one-way ANOVA (Table 4) illustrate that the mean difference for each of the reference materials SSC-1, SSC-3, SSC-4, and CUPD-1 is statistically not significant at the 0.05 level as determined with F_{(2, 131)} = 2.782, and p = 0.066 for SSC-1, F_{(2, 153)} = 1.746, and p = 0.178 for SSC-3, F_{(2, 143)} = 0.632, and p = 0.533 for SSC-4, and F_{(2, 105)} = 2.260 and p = 0.110 for CUPD-1.

The results of intensive homogeneity testing on two sections for each of the reference materials demonstrate that the new Cu isotope reference materials SSC-1, SSC-3, SSC-4 and CUPD-1 can be considered isotopically homogeneous at a spatial resolution of 43 µm with 95% confidence. It should also be noted that short ablations for some thin grains of CUPD-1, owing to rapid penetration of the laser through the grain, resulted in larger counting statistics-associated within-run measurement precision (2 standard error > 0.1‰). Therefore, LA analyses of CUPD-1 with less than 20 s laser ablation time were not considered in this study. Consequently, there are fewer Cu isotope data for CUPD-1 than for the other three reference materials. Further homogeneity tests should be conducted to unequivocally confirm isotopic homogeneity of CUPD-1.

Laser Ablation MC-ICP-MS Measurement

The δ⁶⁵Cu values and measurement precisions (2 s.d.) of SSC-1, SSC-3, SSC-4 and CUPD-1 determined by LA-MC-ICP-MS using each of the other three reference materials as a calibration standard are summarized in Figures 6A–D; Tables 3, 5.

FIGURE 6

FIGURE 6. Results of Cu isotope ratio determinations of reference materials SSC-1 (A), SSC-3 (B), SSC-4 (C), and CUPD-1 (D) by LA-MC-ICP-MS using each of the other three reference materials as a calibration standard. For a single measurement, the within-run precision is 2 standard error. For comparison, δ⁶⁵Cu values and measurement precision obtained by SN-MC-ICP-MS is indicated by solid black lines and shaded areas.

TABLE 5

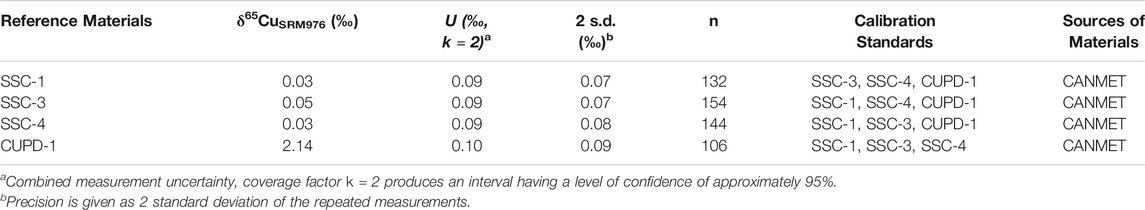
TABLE 5. δ⁶⁵Cu_SRM976 values of the reference materials SSC-1, SSC-3, SSC-4 and CUPD-1 determined by LA-MC-ICP-MS.

The LA Cu isotope data for SSC-1, SSC-3, SSC-4 and CUPD-1 are plotted against the SN Cu isotope data in Figure 7. The Cu isotope data overlap, within measurement precision (2 s.d.), a 1:1 reference line, indicating that precise and accurate Cu isotope measurements by LA-MC-ICP-MS were achieved using these reference materials as calibration standards. The achievable external measurement precision (2 s.d.) for our ns pulse LA-MC-ICP-MS, expressed as relative Cu isotope amount ratios, was ± 0.07–0.09‰. This is comparable to the reported external precision of better than 0.1‰ by Jackson and Günther (2003) and Hirata et al. (2003), using ns pulse LA analysis, and Lazarov and Horn (2015) using fs pulse LA analysis with similar spot size. The measurement precision (2 standard error, number of cycles = 121) for a single measurement was 0.07‰ for ∼110 s ablation acquisition time, which is 0.035–0.055‰ higher than the reported uncertainty using fs LA analysis (Lazarov and Horn, 2015). The mean Cu signal intensity for ns pulse LA Cu isotope measurement in this study was ∼ 12 V and the calculated Poisson counting statistics error on the ⁶⁵Cu/⁶³Cu ratio was 0.000004, which is ca. 1.4 times higher than that for fs pulse LA Cu isotope measurements reported by Lazarov and Horn (2015). In our ns LA study, ca. 26% of the overall uncertainty of the ⁶⁵Cu/⁶³Cu ratio came from counting statistics, while ca. 33% of the overall uncertainty was due to counting statistics in the fs LA study of Lazarov and Horn (2015). It is worth noting that within-run measurement precision obtained using ns pulse LA Cu isotope measurement in this study was a combined uncertainty, including the uncertainties from analyses of both bracketing calibration standard and the NIST SRM976 normalization standard. Uncertainties propagating from the normalizing standard resulted in degraded analytical precision. NIST SRM976 was used both for calibration and normalization in the fs pulse LA Cu isotope study (Lazarov and Horn, 2015).

FIGURE 7

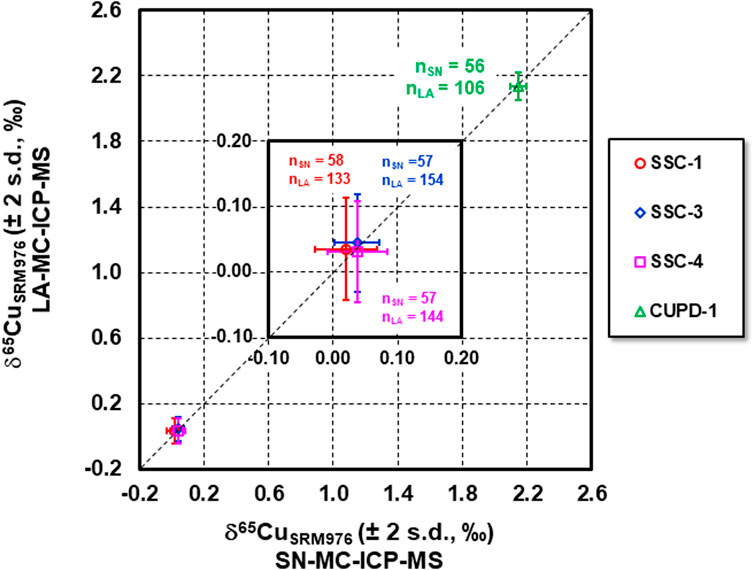
FIGURE 7. Cu isotope compositions of SSC-1, SSC-3, SSC-4 and CUPD-1 determined by LA-MC-ICP-MS vs. SN-MC-ICP-MS. The Cu isotope data overlap well the 1:1 relationship within the uncertainty, indicating that the measured Cu isotope composition of SSC-1, SSC-3, SSC-4 and CUPD-1 by LA analysis agree with those by SN analysis within the analytical uncertainties, and that precise and accurate Cu isotope measurements by LA-MC-ICP-MS using these reference materials as calibration standards were achieved.

Finally, the developed Cu isotope reference materials SSC-1, SSC-3, SSC-4 and CUPD-1 were employed as calibration standards in the measurement of the Cu isotope composition of a native copper sample, NMC 12864, by LA analysis. The results (Figure 8 and Supplementary Table S2) show that the mean Cu isotope composition of sample NMC 12864 was 0.54 ± 0.11‰ (U, k = 2, n = 50), and the Cu isotope ratios are remarkably consistent within the sample fragment (∼ 6 × 6 mm). This demonstrates that consistent Cu isotope ratio measurements were achieved using each of the developed Cu isotope reference materials as a calibration standard for LA-MC-ICP-MS Cu isotope analysis of native copper.

FIGURE 8

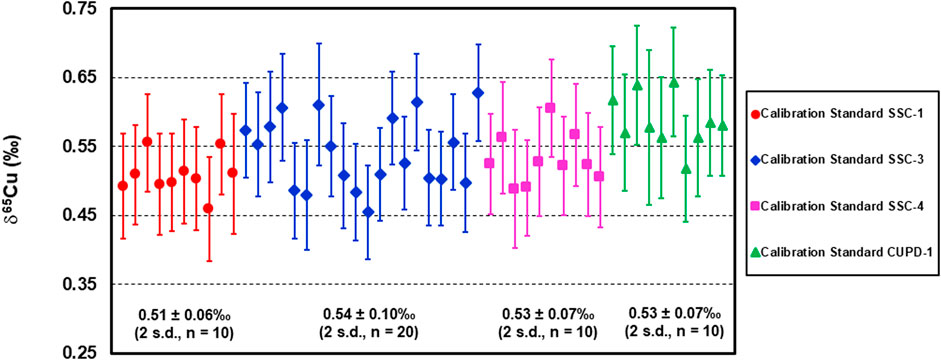
FIGURE 8. Cu isotope composition of native copper sample NMC 12864 determined by LA-MC-ICP-MS using reference materials SSC-1, SSC-3, SSC-4 and CUPD-1 as calibration standards.

Conclusions

The new Cu isotope reference materials SSC-1, SSC-3, SSC-4 and CUPD-1 can be considered isotopically homogeneous at a spatial resolution of 43 µm with 95% confidence. The Cu isotopic compositions (δ⁶⁵Cu) and measurement precisions of SSC-1, SSC-3, SSC-4, and CUPD-1, relative to Cu isotope standard NIST SRM976, are summarized in Tables 2, 5. The Cu isotope ratios for each of the reference materials determined by LA-MC-ICP-MS are in agreement with the SN-MC-ICP-MS data within analytical uncertainty. The achievable combined measurement uncertainty of our ns pulse LA-MC-ICP-MS Cu isotope analysis of native copper (U, k = 2) was 0.09–0.10‰, whereas it was 0.04–0.08‰ by SN-MC-ICP-MS analysis. The Cu isotope composition of SSC-1, SSC-3, and SSC-4 are identical to each other within analytical uncertainty, and they are only 0.03–0.05‰ heavier than the NIST SRM976. On the other hand, CUPD-1 displays a much heavier Cu isotope composition (2.14‰) than the NIST SRM976. The unique Cu isotope composition of CUPD-1 makes it more useful in LA Cu isotope measurements of those native copper samples that exhibit similar Cu isotope compositions; for example, Bornhorst and Mathur (2017) have reported a δ⁶⁵Cu value of 2.29‰ for a native Cu sample, which they attribute to near-surface supergene processes.

The results of this study demonstrate that SSC-1, SSC-3, SSC-4 and CUPD-1 are suitable reference materials for calibration and quality control of in situ LA-MC-ICP-MS Cu isotope analysis at spot size of 43 μm. These reference materials are also suitable reference materials for calibration and quality control of SN-MC-ICP-MS Cu isotope analysis. Further investigation of the homogeneity of CUPD-1 might be needed as a result of the smaller number of analyses of this sample. In addition, the influence of laser focus position along with the crater geometry should be investigated in the future for better understanding the causes of the inconsistency and relatively large variations in Cu isotope measurements for some analyses by ns pulse LA-MC-ICP-MS.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding authors.

Author Contributions

ZY conducted the method development and experiments, carried out the experiment data reduction, and reference materials homogeneity tests, wrote the article, and performed article revision. SJ provided the reference materials, participated in the method development and experimental design, and provided major article revision. TS contributed the native Cu sample, provided suggestions for the homogeneity test, and performed article revision.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

The authors would like to thank the Targeted Geoscience Initiative 5 (TGI-5) of the Geological Survey of Canada (GSC), Natural Resources Canada for financial support for this project. Don Chipley from Queen’s University, Canada is particularly acknowledged for generously providing the precious NIST SRM976 Cu isotope solution standard. We are grateful to Isabelle Girard, GSC, for technical assistance with the SN-ICP-MS analyses.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2021.617205/full#supplementary-material.

Footnotes

¹CUPD-1 was discontinued after submission of this manuscript. Please contact the authors regarding the distribution of the reference materials.

References

Archer, C., and Vance, D. (2004). Mass discrimination correction in multiple-collector plasma source mass spectrometry: an example using Cu and Zn isotopes. J. Anal. At. Spectrom. 19, 656–665. doi:10.1039/b315853e

CrossRef Full Text | Google Scholar

Baggio, S. B., Hartmann, L. A., Lazarov, M., Massonne, H.-J., Opitz, J., Theye, T., et al. (2018). Origin of native copper in the Paraná volcanic province, Brazil, integrating Cu stable isotopes in a multi-analytical approach. Miner. Depos. 53, 417–434. doi:10.1007/s00126-017-0748-2

CrossRef Full Text | Google Scholar

Bornhorst, T. J., and Mathur, R. (2017). Copper isotope constraints on the genesis of the Keweenaw Peninsula native copper district, Michigan, USA. Minerals 7 (185), 1–20. doi:10.3390/min7100185

CrossRef Full Text | Google Scholar

Braxton, D., and Mathur, R. (2011). Exploration applications of copper isotopes in the supergene environment: a case study of the Bayugo porphyry copper-gold deposit, southern Philippines. Econ. Geol. 106, 1447–1463. doi:10.2113/econgeo.106.8.1447

CrossRef Full Text | Google Scholar

Chapman, J. B., Mason, T. F. D., Weiss, D. J., Coles, B. J., and Wilkinson, J. J. (2006). Chemical separation and isotopic variations of Cu and Zn from five geological reference materials. Geostand. Geoanalyt. Res. 30, 5–16. doi:10.1111/j.1751-908x.2006.tb00907.x

CrossRef Full Text | Google Scholar

Cooper, H. K., Duke, M. J. M., Simonetti, A., Chen, G., and Chen, G. (2008). Trace element and Pb isotope provenance analyses of native copper in northwestern North America: results of a recent pilot study using INAA, ICP-MS, and LA-MC-ICP-MS. J. Archaeol. Sci. 35, 1732–1747. doi:10.1016/j.jas.2007.11.012

CrossRef Full Text | Google Scholar

Dekov, V. M., Rouxel, O., Asael, D., Hålenius, U., and Munnik, F. (2013). Native Cu from the oceanic crust: isotopic insights into native metal origin. Chem. Geol. 359, 136–149. doi:10.1016/j.chemgeo.2013.10.001

CrossRef Full Text | Google Scholar

G. Faure (Editor) (1991). “Chemical weathering of mineral deposits,” in Principles and applications of inorganic geochemistry. 2nd Edn (New York, NY: Macmillan Publishing Company), 476–626.

Google Scholar

Faure, G., and Mensing, T. M. (2005). Isotopes: principles and applications. 3rd Edn. New York, NY: John Wiley & Sons, Inc.

Gale, N. H., Woodhead, A. P., Stos-Gale, Z. A., Walder, A., and Bowen, I. (1999). Natural variations detected in the isotopic composition of copper: possible applications to archaeology and geochemistry. Int. J. Mass Spectrom. 184, 1–9. doi:10.1016/s1387-3806(98)14294-x

CrossRef Full Text | Google Scholar

Gilbert, S. E., Danyushevsky, L. V., Rodemann, T., Shimizu, N., Gurenko, A., and Meffre, S. (2014). Optimisation of laser parameters for the analysis of sulphur isotopes in sulphide minerals by laser ablation ICP-MS. J. Anal. At. Spectrom. 29, 1042–1051. doi:10.1039/c4ja00011k

CrossRef Full Text | Google Scholar

Graham, S., Pearson, N., Jackson, S., Griffin, W., and O’Reilly, S. Y. (2004). Tracing Cu and Fe from source to porphyry: in situ determination of Cu and Fe isotope ratios in sulfides from the Grasberg Cu-Au deposit. Chem. Geol. 207, 147–169. doi:10.1016/j.chemgeo.2004.02.009

CrossRef Full Text | Google Scholar

Guillong, M., and Günther, D. (2002). Effect of particle size distribution on ICP-induced elemental fractionation in laser ablation-inductively coupled plasma-mass spectrometry. J. Anal. At. Spectrom. 17, 831–837. doi:10.1039/b202988j

CrossRef Full Text | Google Scholar

Hirata, T., Hayano, Y., and Ohno, T. (2003). Improvements in precision of isotopic ratio measurements using laser ablation-multiple collector-ICP-mass spectrometry: reduction of changes in measured isotopic ratios. J. Anal. At. Spectrom. 18, 1283–1288. doi:10.1039/b305127g

CrossRef Full Text | Google Scholar

Horn, I., and von Blanckenburg, F. (2007). Investigation on elemental and isotopic fractionation during 196 nm femtosecond laser ablation multiple collector inductively coupled plasma mass spectrometry. Spectrochim. Acta B At. Spectrosc. 62, 410–422. doi:10.1016/j.sab.2007.03.034

CrossRef Full Text | Google Scholar

Ikehata, K., Chida, K., Bornhorst, T. J., Ishibashi, J., and Hirata, T. (2015). Using copper isotopic composition to distinguish native copper formation mechanisms. Geol. Soc. Am. Abstr. Prog. 47. 518.

Google Scholar

Ikehata, K., and Hirata, T. (2012). Copper isotope characteristics of copper-rich minerals from Horoman peridotite complex, Hokkaido, Northen Japan. Econ. Geol. 107, 1489–1497. doi:10.2113/econgeo.107.7.1489

CrossRef Full Text | Google Scholar

Ikehata, K., and Hirata, T. (2013). Evaluation of UV-fs-LA-MC-ICP-MS for precise in situ copper isotopic microanalysis of cubanite. Anal. Sci. 29, 1213–1217. doi:10.2116/analsci.29.1213

PubMed Abstract | CrossRef Full Text | Google Scholar

Ikehata, K., Notsu, K., and Hirata, T. (2008). In situ determination of Cu isotope ratios in copper-rich materials by NIR LA-MC-ICP-MS. J. Anal. At. Spectrom. 23, 1003–1008. doi:10.1039/b801044g

CrossRef Full Text | Google Scholar

Ikehata, K., Notsu, K., and Hirata, T. (2011). Copper isotope characteristics of copper-rich minerals from Besshi-type volcanogenic massive sulfide deposits, Japan, determined using a Femtosecond LA-MC-ICP-MS. Econ. Geol. 106, 307–316. doi:10.2113/econgeo.106.2.307

CrossRef Full Text | Google Scholar

Jackson, S. E., and Günther, D. (2003). The nature and sources of laser induced isotopic fractionation in laser ablation-multicollector-inductively coupled plasma-mass spectrometry. J. Anal. At. Spectrom. 18, 205–212. doi:10.1039/b209620j

CrossRef Full Text | Google Scholar

Jackson, S. E., Pearson, N. J., and Griffin, W. L. (2001). “In situ isotope ratio determination using LA-magnetic sector-ICP-MS,” in Laser ablation-mass spectrometry in the Earth sciences: principles and applications. Editor P. J. Sylvester (Quebec, Canada: Mineralogical Association of Canada (MAC) Short Course Series), 29, 105–119.

Google Scholar

Jarque, C., and Bera, A. (1980). Efficient tests for normality, homoscedasticity and serial independence of regression residuals. Econ. Lett. 6, 255–259. doi:10.1016/0165-1765(80)90024-5

CrossRef Full Text | Google Scholar

Jarque, C. M., and Bera, A. K. (1987). A test for normality of observations and regression residuals. Int. Stat. Rev. 55, 163–172. doi:10.2307/1403192

CrossRef Full Text | Google Scholar

JCGM 100 (2008). Evaluation of measurement data-guide to the expression of uncertainty in measurement. Geneva, Switzerland: International Organization for Standardization, 134.

Kuhn, H.-R., Pearson, N. J., and Jackson, S. E. (2007). The influence of the laser ablation process on isotopic fractionation of copper in LA-MC-ICP-MS. J. Anal. At. Spectrom. 22, 547–552. doi:10.1039/b616232k

CrossRef Full Text | Google Scholar

Larner, F., Rehkämper, M., Coles, B. J., Kreissig, K., Weiss, D. J., Sampson, B., et al. (2011). A new separation procedure for Cu prior to stable isotope analysis by MC-ICP-MS. J. Anal. At. Spectrom. 26, 1627–1632. doi:10.1039/c1ja10067j

CrossRef Full Text | Google Scholar

Larson, P. B., Maher, K., Ramos, F. C., Chang, Z., Gaspar, M., and Meinert, L. D. (2003). Copper isotope ratios in magmatic and hydrothermal ore-forming environments. Chem. Geol. 201, 337–350. doi:10.1016/j.chemgeo.2003.08.006

CrossRef Full Text | Google Scholar

Lazarov, M., and Horn, I. (2015). Matrix and energy effects during in-situ determination of Cu isotope ratios by ultraviolet-femtosecond laser ablation multicollector inductively coupled plasma mass spectrometry. Spectrochim. Acta Part B At. Spectrosc. 111, 64–73. doi:10.1016/j.sab.2015.06.013

CrossRef Full Text | Google Scholar

Li, W., Jackson, S. E., Pearson, N. J., and Graham, S. (2010). Copper isotopic zonation in the Northparkes porphyry Cu-Au deposit, SE Australia. Geochim. Cosmochim. Acta 74, 4078–4096. doi:10.1016/j.gca.2010.04.003

CrossRef Full Text | Google Scholar

Ludwig, K. R. (2003). Isoplot 3.00: a geochronological toolkit for Microsoft Excel. Berkeley, CA: Berkeley Geochronology Center, 70.

Maher, K. C. (2005). Analysis of copper isotope ratios by multic-collector inductively coupled plasma mass spectrometry and interpretation of copper isotope ratios from copper mineralization. PhD thesis. Pullman (WA): Department of Geology, Washington State University, 1–249.

Google Scholar

Maher, K. C., and Larson, P. B. (2007). Variation in copper isotope ratios and controls on fractionation in hypogene skarn mineralization at Coroccohuayco and Tintaya, Peru. Econ. Geol. 102, 225–237. doi:10.2113/gsecongeo.102.2.225

CrossRef Full Text | Google Scholar

Malson, G. L. (1975). Commercial-purity copper rods, SSC-1, SSC-2, SSC-3 and SSC-4: their preparation and certification as certified reference materials. The non-ferrous standards committee of the spectroscopy society of Canada and members of the staff of the former mines branches. Ottawa, Canada: Canada Center for Mineral and Energy Technology, 1–7.

Malson, G. L. (2003). SSC-1 to SSC-3 commercial-purity copper rods, certified reference materials. Ottawa, Canada: CANMET, 1–2.

Maréchal, C., and Albarède, F. (2002). Ion-exchange fractionation of copper and zinc isotopes. Geochim. Cosmochim. Acta 66, 1499–1509. doi:10.1016/s0016-7037(01)00815-8

CrossRef Full Text | Google Scholar

Maréchal, C. N., Télouk, P., and Albarède, F. (1999). Precise analysis of copper and zinc isotopic compositions by plasma-source mass spectrometry. Chem. Geol. 156, 251–273. doi:10.1016/s0009-2541(98)00191-0

CrossRef Full Text | Google Scholar

Markl, G., Lahaye, Y., and Schwinn, G. (2006). Copper isotopes as monitors of redox processes in hydrothermal mineralization. Geochim. Cosmochim. Acta 70, 4215–4228. doi:10.1016/j.gca.2006.06.1369

CrossRef Full Text | Google Scholar

Mason, T. F. D., Weiss, D. J., Horstwood, M. S. A., and Parrish, R. R. (2004a). High-precision Cu and Zn isotope analysis by plasma source mass spectrometry - Part 1. Spectral interferences and their correction. J. Anal. At. Spectrom. 19, 209–217. doi:10.1039/b306958c

CrossRef Full Text | Google Scholar

Mason, T. F. D., Weiss, D. J., Horstwood, M. S. A., and Parrish, R. R. (2004b). High-precision Cu and Zn isotope analysis by plasma source mass spectrometry - Part 2. correcting for mass discrimination effects. J. Anal. At. Spectrom. 19, 218–226. doi:10.1039/b306953b

CrossRef Full Text | Google Scholar

Mathur, R., Falck, H., Belogub, E., Milton, J., Wilson, M., Rose, A., et al. (2018). Origins of chalcocite defined by copper isotope values. Geofluids 2018, 1–9. doi:10.1155/2018/5854829

CrossRef Full Text | Google Scholar

Mathur, R., Munk, L., Nguyen, M., Gregory, M., Annell, H., and Lang, J. (2013). Modern and paleofluid pathways revealed by Cu isotope compositions in surface waters and ores of the pebble porphyry Cu-Au-Mo deposit, Alaska. Econ. Geol. 108, 529–541. doi:10.2113/econgeo.108.3.529

CrossRef Full Text | Google Scholar

Mathur, R., Titley, S., Barra, F., Brantley, S., Wilson, M., Phillips, A., et al. (2009a). Exploration potential of Cu isotope fractionation in porphyry copper deposits. J. Geochem. Explor. 102, 1–6. doi:10.1016/j.gexplo.2008.09.004

CrossRef Full Text | Google Scholar

Mathur, R., Titley, S., Hart, G., Wilson, M., Davignon, M., and Zlatos, C. (2009b). The history of the United States cent revealed through copper isotope fractionation. J. Archaeol. Sci. 36, 430–433. doi:10.1016/j.jas.2008.09.029

CrossRef Full Text | Google Scholar

Mathur, R., Wilson, M., and Parra, M. L. (2014). Challenges of using copper isotope ratios to trace the origin of native copper artifacts: an example from the Keweenaw Peninsula. Ann. Carnegie Mus. 82, 241–245. doi:10.2992/007.082.0304

CrossRef Full Text | Google Scholar

Moeller, K., Schoenberg, R., Pedersen, R., Weiss, D., and Dong, S. (2012). Calibration of the new certified reference materials ERM®-AE633 and ERM®-AE647 for copper and IRMM-3702 for zinc isotope amount ratio determinations. Geostand. Geoanal. Res. 36, 177–199. doi:10.1111/j.1751-908x.2011.00153.x

CrossRef Full Text | Google Scholar

Moynier, F., Vance, D., Fujii, T., and Savage, P. (2017). “The isotope geochemistry of zinc and copper,” in Review in mineralogy and geochemistry. Editor J. J. Rosso (Chantilly, VA: Mineralogical Society of America, Geochemical Society), Vol. 82, 543–600.

CrossRef Full Text | Google Scholar

Peel, K., Weiss, D., Chapman, J., Arnolda, T., and Colesa, B. (2008). A simple combined sample-standard bracketing and inter-element correction procedure for accurate mass bias correction and precise Zn and Cu isotope ratio measurements. J. Anal. At. Spectrom. 23, 103–110. doi:10.1039/b710977f

CrossRef Full Text | Google Scholar

Poitrasson, F., and d'Abzac, F. (2017). Femtosecond laser ablation inductively coupled plasma source mass spectrometry for elemental and isotopic analysis: are ultrafast lasers worthwhile? J. Anal. At. Spectrom. 32, 1075–1091. doi:10.1039/c7ja00084g

CrossRef Full Text | Google Scholar

Ponzevera, E., Quétel, C. R., Berglund, M., Taylor, P. D., Evans, P., Loss, R. D., et al. (2006). Mass discrimination during MC-ICPMS isotopic ratio measurements: investigation by means of synthetic isotopic mixtures (IRMM-007 series) and application to the calibration of natural-like zinc materials (including IRMM-3702 and IRMM-651). J. Am. Soc. Mass Spectrom. 17, 1412–1427. doi:10.1016/j.jasms.2006.06.001

CrossRef Full Text | Google Scholar

Rasberry, S. D. (1988). Standard reference material 683 zinc metal. Gaithersburg, MD: National Bureau of Standards Certificate of Analysis, National Institute of Standards and Technology.

Rouxel, O., Fouquet, Y., and Ludden, J. N. (2004). Copper isotope systematics of the lucky strike, Rainbow, and Logatchev sea-floor hydrothermal fields on the Mid-Atlantic ridge. Econ. Geol. 99, 585–600. doi:10.2113/gsecongeo.99.3.585

CrossRef Full Text | Google Scholar

Royston, J. P. (1982). An extension of Shapiro and Wilk W test for normality to large samples. Appl. Stat. 31, 115–124. doi:10.2307/2347973

CrossRef Full Text | Google Scholar

Salley, J., and Leaver, M. E. (2009). CUPD-1 copper anode certified reference materials, certificate of analysis. Ottawa, Canada: CANMET-MMSL, 1–2.

Savage, P. S., Moynier, F., Chen, H., Shofner, G., Siebert, J., Badro, J., et al. (2015). Copper isotope evidence for large-scale sulphide fractionation during Earth’s differentiation. Geochem. Perspect. Lett. 1, 53–64. doi:10.7185/geochemlet.1506

CrossRef Full Text | Google Scholar

Semerok, A., Sallé, B., Wanger, W-F., and Petite, G. (2002). Femtosecond, picosecond, and nanosecond laser microablation: laser plasma and crater investigation. Laser Part. Beams 20, 67–72. doi:10.1017/s0263034602201093

CrossRef Full Text | Google Scholar

Shapiro, S. S., and Wilk, M. B. (1965). An analysis of variance test for normality (complete samples). Biometrika 52, 591–611. doi:10.1093/biomet/52.3-4.591

CrossRef Full Text | Google Scholar

Shapiro, S. S., and Wilk, M. B. (1968). Approximations for the null distribution of the W statistic. Technometrics 10, 861–866. doi:10.2307/1267467

CrossRef Full Text | Google Scholar

Shields, W. R., Goldich, S. S., Garner, E. L., and Murphy, T. J. (1965). Natural variations in the abundance ratio and the atomic weight of copper. J. Geophys. Res. 70, 479–491. doi:10.1029/jz070i002p00479

CrossRef Full Text | Google Scholar

Shields, W. R., Murphy, T. J., and Garner, E. L. (1964). Absolute isotopic abundance ratio and the atomic weight of a reference sample of copper. J. Res. Natl. Bur. Stand. A Phys. Chem. 68, 589–592. doi:10.6028/jres.068a.056

CrossRef Full Text | Google Scholar

Siebert, J., Corgne, A., and Ryerson, F. J. (2011). Systematics of metal-silicate partitioning for many siderophile elements applied to Earth’s core formation. Geochim. Cosmochim. Acta 75, 1451–1489. doi:10.1016/j.gca.2010.12.013

CrossRef Full Text | Google Scholar

Sullivan, K., Layton‐Matthews, D., Leybourne, M., Kidder, J., Mester, Z., and Yang, L. (2020). Copper isotopic analysis in geological and biological reference materials by MC‐ICP‐MS. Geostand. Geoanal. Res. 44, 349–362. doi:10.1111/ggr.12315

CrossRef Full Text | Google Scholar

Thomas, E. G. (1994). Standard reference material SRM976 isotopic standard for copper, certificate of analysis. Gaithersburg, MD: National institute of standards and technology, 1.

Walker, E. C., Cuttittla, F., and Senftle, F. E. (1958). Some natural variations in the relative abundance of copper isotopes. Geochim. Cosmochim. Acta 15, 183–194. doi:10.1016/0016-7037(58)90056-5

CrossRef Full Text | Google Scholar

Wendt, I., and Carl, C. (1991). The statistical distribution of the mean squared weighted deviation. Chem. Geol. 86, 275–285. doi:10.1016/0168-9622(91)90010-t

CrossRef Full Text | Google Scholar

Wilson, M., Wilson, D. L., and Mathur, R. (2016). Tracing the source of native copper mineral specimens with copper isotope values. Rocks Miner. 91, 352–356. doi:10.1080/00357529.2016.1172176

CrossRef Full Text | Google Scholar

Yang, L., Tong, S., Zhou, L., Hu, Z., Mester, Z., and Meija, J. (2018). A critical review on isotopic fractionation correction methods for accurate isotope amount ratio measurements by MC-ICP-MS. J. Anal. At. Spectrom. 33, 1849–1861. doi:10.1039/c8ja00210j

CrossRef Full Text | Google Scholar

Yang, Y., Zhang, X., Liu, S.-A., Zhou, T., Fan, H., and Yu, H. (2018). Calibrating NIST SRM683 as a new international reference standard for Zn isotopes. J. Anal. At. Spectrom. 33, 1777–1783. doi:10.1039/c8ja00249e

CrossRef Full Text | Google Scholar

Yuan, H., Yuan, W., Bao, Z., Chen, K., Huang, F., and Liu, S. (2017). Development of two new copper isotope standard solutions and their copper isotopic compositions. Geostand. Geoanal. Res. 41, 77–84. doi:10.1111/ggr.12127

CrossRef Full Text | Google Scholar

Zhu, X. K., Guo, Y., Williams, R. J. P., O’Nions, R. K., Matthews, A., Belshaw, N. S., et al. (2002). Mass fractionation processes of transition metal isotopes. Earth Planet. Sci. Lett. 200, 47–62. doi:10.1016/s0012-821x(02)00615-5

CrossRef Full Text | Google Scholar

Zhu, X. K., O'Nions, R. K., Guo, Y., Belshaw, N. S., and Rickard, D. (2000). Determination of natural Cu-isotope variation by plasma-source mass spectrometry: implications for use as geochemical tracers. Chem. Geol. 163, 139–149. doi:10.1016/s0009-2541(99)00076-5

CrossRef Full Text | Google Scholar