Viscoelasticity Acts as a Marker for Tumor Extracellular Matrix Characteristics

Biological materials such as extracellular matrix scaffolds, cancer cells, and tissues are often assumed to respond elastically for simplicity; the viscoelastic response is quite commonly ignored. Extracellular matrix mechanics including the viscoelasticity has turned out to be a key feature of cellular behavior and the entire shape and function of healthy and diseased tissues, such as cancer. The interference of cells with their local microenvironment and the interaction among different cell types relies both on the mechanical phenotype of each involved element. However, there is still not yet clearly understood how viscoelasticity alters the functional phenotype of the tumor extracellular matrix environment. Especially the biophysical technologies are still under ongoing improvement and further development. In addition, the effect of matrix mechanics in the progression of cancer is the subject of discussion. Hence, the topic of this review is especially attractive to collect the existing endeavors to characterize the viscoelastic features of tumor extracellular matrices and to briefly highlight the present frontiers in cancer progression and escape of cancers from therapy. Finally, this review article illustrates the importance of the tumor extracellular matrix mechano-phenotype, including the phenomenon viscoelasticity in identifying, characterizing, and treating specific cancer types.

Introduction

The General Phenomenon of Viscoelasticity

In the nature, certain materials undergo deformations other than purely elastic ones, where the material will fully return to its original shape upon the removal of the external force. Among them are viscoelastic materials. Viscoelastic materials, according to their name, unite two distinct characteristics. The “viscous” part means that they deform gradually when subjected to an applied external force. The “elastic” part means that the material comes back to its original shape after a deforming force is eliminated. In contrary, in pure viscous fluids there is a deformation closely succeeded by a permanent reorganization of the molecules in the fluid. The mechanical characteristics of materials are generally measured in the form of their stress-strain (or load-deformation) response. Specifically, in purely elastic materials, the curves of stress and strain under load and unload are placed on top of each other. In general, the viscoelasticity represents a time-dependent inelastic characteristic of materials, including extracellular matrices frameworks, cells, cell clusters and entire tissues. In specific, the reaction of the material to a stimulus is lagged and a “hysteresis” loop is created, as well as energy is lost within the material. It usually dissipates as heat.

A multitude of inelastic characteristics of actual materials are known, among them are viscoelasticity, plasticity and fracture. However, the focus of this review is placed on viscoelasticity. The inelastic response can be witnessed as a slow or partial recovery of the material upon removal of the forces that induced the deformation. Moreover, it is reasonable that the deformation is a function of the history of imposed forces. In summary, viscoelastic materials exhibit three key characteristics: stress relaxation, which means the stress decrease with time (a response of a viscoelastic material to a constant strain step), creep (a constant stress with decreasing strain as a function of time), and hysteresis (a mismatch between loading and unloading processes) (Banks et al., 2011) (Figure 1). As the term “viscoelasticity” implies, this type of mechanical reaction brings together the reaction of elastic solids and viscous fluids. Therefore, it is not only solids but also liquids that are capable of displaying such a characteristic feature. However, the nature of how these materials answer varies greatly. Specifically, the reaction of a fluid to a specific deformation from any two conditions would be identical, whereas a solid, for instance, in its initial shape and after a deformation would react in a different way. Expressed in more general terms, for solids, pure strains can influence the response of the material, however, rotations can have no impact (Truesdell et al., 2004).

FIGURE 1

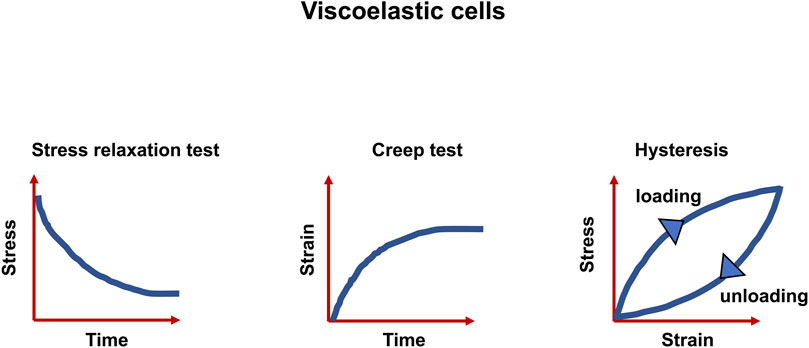
FIGURE 1. Viscoelastic matter are characterized by three features, the relaxation of stress, the response to creep test and the hysteresis between stress and strain.

Another characteristic of viscoelastic materials is that their mechanical behavior relies on the rate of deformation. The material stiffness rises according to the loading rate. Consequently, there is no single stress-strain curve, but a range of curves depicting the deformation response at various deformation rates. In more detail, viscoelastic properties usually arise on a variety of time scales (relaxation times) in the selfsame material. The stimulus responding at short or very short relaxation times (shorter than 1 s) needs to be examined in dynamic conditions using an oscillating excitation at a constant frequency or over a band of frequencies. The behavior at high relaxation times (from 1 s to hours) can be probed through creep experiments (a load is imposed and held constant throughout while the deformation is monitored) or relaxation experiments (the material is stretched and held at constant strain during which the stress is monitored in time). In this review article, an emphasis is placed on the viscoelastic behavior of the tumor extracellular matrix, which reacts as solids.

Concepts of Linear and Nonlinear Viscoelasticity

Although the traditional linear theories of solid mechanics can be extended to a larger class of materials, because many different nonlinear constitutive equations can in fact have the identical linear first approximation (Truesdell et al., 2004), the majority of natural phenomena are nonlinear. Consequently, nonlinear theories are capable of yielding far more precise answers to the actions of materials including living matter, such as extracellular matrices, cells and tissues.

Concept of Linear Viscoelasticity

A displacement is defined as elastic when the undeformed shape is fully restored after elimination of all external forces (Terzopoulos and Fleischer, 1988). The fundamental assumption underpinning the constitutive laws of conventional elasticity theory is that the stress-strain relationship is the same for both loading and unloading, and that the restoring force (stress) is a univalent function of the actual deformation (strain), not its antecedent. To quantity elastic restoring forces, it is feasible to utilize displacement potential energies, what is the characterization that is used in the formulation of those models. Similar to an ideal spring, an elastic model material accumulates potential energy while deforming itself and fully releases the energy when it resumes the former shape. In contrast, a perfect (Newtonian) fluid holds no deformation energy; therefore, it displays no elasticity. Hence, the interest is on models depicting this very common inelastic deformation phenomenon, which lies between fully elastic solids at one side and viscous fluids at the other side. Specifically, the case where the relationship between the stress, strain, and strain rate is nonlinear is a matter of interest in future approaches and thus represents an important Frontier in the field of cancer research. For extracellular matrix scaffolds, cells, clusters of cells and entire tissues, the pure linear elastic behavior cannot be detected. However, the majority of the material can be inferred to be linearly viscoelastic in response to small deformations.

Classical Concept of Nonlinear Viscoelasticity

Even though definitions differ, viscoelasticity is a common characteristic of materials that, when deformed, exhibit both viscous (dashpot-like) and elastic (spring-like) features (Figures 2A,B) (Şengül, 2021). Corresponding to the influence of time on their mechanical response, viscoelastic materials can also be designated as time-dependent materials (Banks et al., 2011). The experimental analysis of this kind of materials is basically more complicated in relation to time-independent materials, since it is not feasible to hold time constant or to remove it in the course of an experiment (Findley et al., 1976). Moreover, the nonlinear viscoelastic behavior of cells has been attributed to cytoskeletal tension (Kollmannsberger et al., 2011). Although observational evidence for cell viscoelasticity has been noted since earlier biomechanical efforts (Y. Fung, 1967; Y.-C. Fung, 1993; Levin and Wyman, 1927), it has been disregarded in benefit of solely hyperelastic approaches because of the emphasis on quasi-static analyses, limited existing experimental data, and mathematical considerations. In fact, whereas hyperelastic modulations have dominated the investigation of biomaterials, viscoelastic effects including stress relaxation, creep, hysteresis, and variable frequency behavior are frequently overlooked (Zhang et al., 2021). Viscoelastic modeling has been first explored by merging linear rheological elements that possess strictly elastic or strictly viscous behavior-springs and dashpots (Levin and Wyman, 1927). In the most basic and oldest versions, these two elements are connected in series, termed Maxwell model (Figure 2C), or in parallel, termed Kelvin-Voigt model (Figure 2D) (Christensen, 1980). To account for physiological effects, these models are augmented by a broader range of rheological elements, such as the standard linear model (Figure 2E) (synonymously referred to the Zener model) or the generalized Maxwell model (Brazel et al., 2012). The application of these linear models to nonlinear viscoelasticity has also been conducted by applying different generalizations (Monsia, 2011; Balbi et al., 2018). These viscoelastic based formulations afford a basis for precisely determining the velocity-dependent characteristics of living tissues.

FIGURE 2

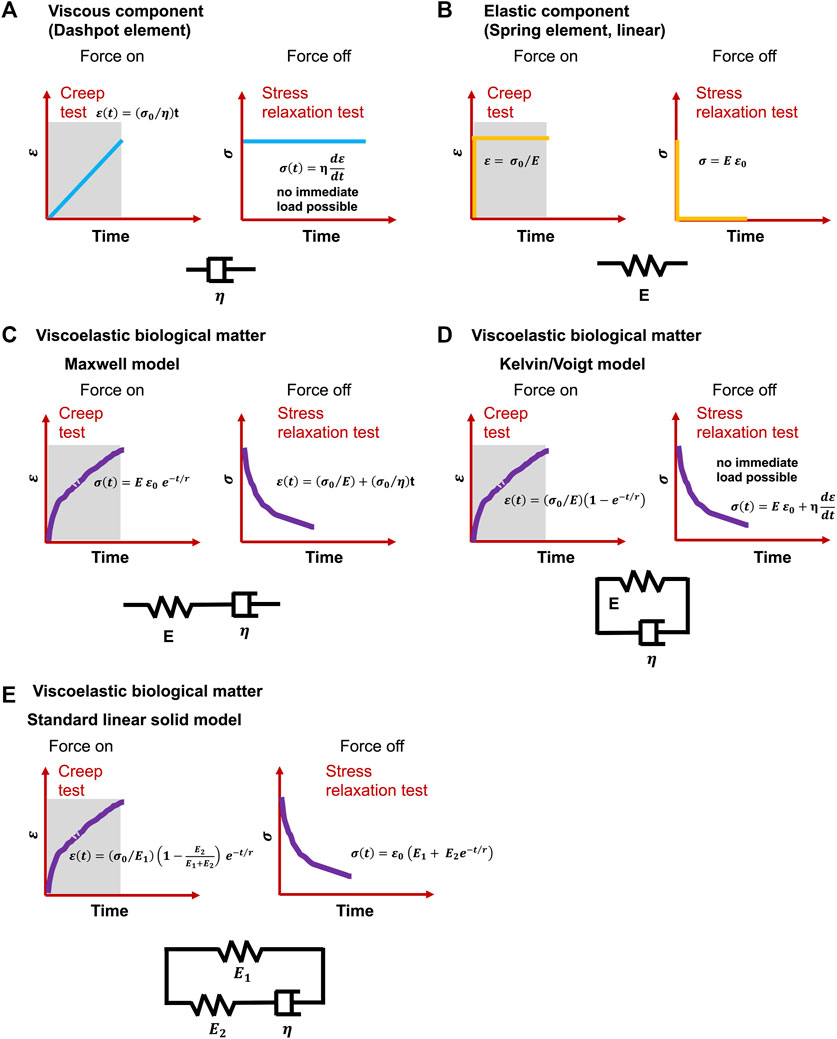
FIGURE 2. Classical viscoelastic models for creep and stress relaxation testing caused by immediate and constant stress ${\sigma }_0$ and strain ${\epsilon }_0$. (A) Spring element, (B) dashpot element, C-E) Cells undergo a material response when they are mechanically reshaped (deformed): (C) Maxwell model, (D) Kelvin or Voigt model and (E) Standard linear solid model. t is time, E represents the elastic modulus, $\eta$ is the viscosity of dashpots and r denotes relaxation time.

New Concept of Nonlinear Viscoelasticity Model

The mechanical properties of materials, such as stiffness, alter with temperature. Therefore, it stands to reason that the deformation of viscoelastic materials also changes with temperature. As a matter of fact, the deformation of viscoelastic matter relies on the temperature with the occurrence of a thermal transition. Alterations of the free volume or relaxation time are employed to explore this transition characteristics (Q. Xu et al., 2020). The viscoelastic deformation has been explained in terms of two atomistic phenomena (Roylance et al., 2001): on the one hand, the distortion of the lengths and angles of chemical bonds linking atoms in a small and fast agitation, and on the other hand, the large-scale redistributions of atoms and molecules. Thermal transitions at increased temperatures can encompass a handful of steps, such as for polymers, γ, which involves the local movement of molecular bonds, and β, which denotes the bending and stretching events of molecular bonds, glass, which encompasses the transition from the glassy to the rubbery phase, and terminal transitions that occur from melts into liquids (McCrum et al., 1991). Various transitions exist for diverse materials, but the glass transition is the principal type of deformation for viscoelasticity, which becomes the subject of attention in several respects. The viscoelasticity of materials can be denoted through the relaxation modulus E(t) or the dynamic modulus E*(ω) = E′(ω) + iE′′(ω). E(t) and E*(ω) rely both on temperature which can be transferred into a time or frequency equivalency based on the time-temperature superposition concept. Several biophysical models have been proposed to grasp the linear viscoelastic characteristics. For example, the Rouse model (Rouse, 1953) is built on molecular dynamics theory and employs Brownian motion theory to predict single-chain diffusion of beads joined together through harmonic springs. Moreover, the Kremer-Grest model (Kremer and Grest, 1990) utilizes up to several hundreds of chains (Likhtman et al., 2007) for probing the individual polymer entities. The single-chain theories including the tube theory (de Gennes, 1971) and the arm retraction model incorporating arm launch (de Gennes, 1979) (Figure 2) have also been employed to characterize the linear viscoelastic performance of entangled polymers, such as hydrogels composed of extracellular matrix molecules. The generalized Maxwell model seems to be the most frequently used model for characterizing the glass transfer of linear viscoelastic solids that can also be applied to tumor extracellular matrix scaffolds. For the unwrapped polymers, the spring-pot row of the generalized Maxwell model physically depicts various molecular chains possessing different lengths with time distributions (Roylance et al., 2001) (Figure 3). E(t) of generalized Maxwell model can be denoted as provided in the following Equation 1:

$E\left(t\right)= E_{\infty }+{\displaystyle \sum _{i=1}^n{E}_i{e}^{-\frac{E_i}{{\eta }_i}t}}(1)$

where $E_{\infty }$ represents the modulus at infinite time, $E_i$ stands for the elastic modulus of the spring, ${\eta }_i$ is referred to as viscosity of the linear dashpot in series, and n denotes the number of spring-dashpot terms. This merged exponential term in a single discrete spectrum is also known as the Prony series. It merges toward a standard solid model when n is equal to 1. The Maxwell or generalized Maxwell-based model along with its Prony series formula are widely adopted to match the modulus of linear viscoelastic materials (Soussou et al., 1970). These materials encompass polymers (Krairi and Doghri, 2014), dielectric elastomers (O’Brien et al., 2009; Bai et al., 2014), glasses (Koontz et al., 2012), silicon (Norris, 2012), tissue (Eastwood et al., 2015), blood vessels (Singh et al., 2003), brain (Elkin et al., 2011), ligament (Provenzano et al., 2002), worm (Backholm et al., 2013), and asphalt concrete (AC) (Q. Xu and Solaimanian, 2009). The generalized Maxwell model has also been employed as a foundation that can be broadened to plasticity models which comprise the viscoplastic Bingham-Maxwell model (Prior et al., 2016). Additional models similar to the generalized Maxwell model encompass the so-called generalized Kelvin model and the Burgers model (Chaudhuri et al., 2015). The Prony series is mathematically elegant with its exponential time-integration equation (Slanik et al., 2000), and has therefore been adopted as the standard material model in mainstream numerical programs, such as ANSYS and ABAQUS, for the purpose of modeling material and structural behaviors. The nonlinear viscoelastic models have also been advocated for the modeling of materials with high deformations or characteristics that evolve with deformation or time (Kim, 2011; Xu and Engquist, 2018). One of them is the model of Schapery that regarded the elastic modulus of the spring as a nonlinear function of time (Schapery, 1969), and several other models have estimated the stress of the dashpot in a nonlinear function to be dependent on either strain rate (Monsia, 2011; Chung and Buist, 2012) or relaxation time (Xu and Engquist, 2018). However, liquid models are usually not included.

FIGURE 3

FIGURE 3. Four models for viscoelasticity. (A) Classical arm-retraction model, (B) Classical Prony series model, (C) New model and (D) Nonlinear spring-dashpot model.

Nevertheless, there are some critical concerns that have emerged. The models based on the theory of multiple molecular chains have been deduced and confirmed principally for polymers, and they are less appropriate to characterize the physics of other compounds with a dissimilar morphology for instance, materials with an amorphous structure. For the generalized Maxwell model or Prony series: one issue is that the Prony series formula can generate instability in fitting experimental acquisition data. A second issue is that it is quite hard to specify a huge number of model inputs based on experimental observations. A third issue is that with a large number of model inputs, the precision for fitting experimental data has increased in mathematical terms. But the physical explanation of this large spring and dashpot arrangement turns out to be less obvious and more complicated (Q. Xu et al., 2020). These inadequacies of available models are the motivation to strive for the creation of a new viscoelastic material model that more precisely depicts a broad spectrum of materials (Q. Xu et al., 2020). In specific detail, a theoretical model and a mathematical solve have been formulated. Experimental confirmations have been carried out for a variety of materials that span from inorganic materials to biomaterials.

In briefly, the model has been found to increase precision both in fitting experimental data and in forecasting out-of-experimental-range moduli. The model is both numerically stable and will not slow down the computational process. It employs fewer model variables than the generalized Maxwell model or the Prony series. In the proposed model, the nonlinear strain-hardening characteristics are also taken into account. Hence, the novel Xu’s model (Q. Xu et al., 2020) is debated in the following. An innovative nonlinear viscoelastic model for describing the glass transition of solid matter has been introduced to surmount the inadequacies of available models. The model characterizes the modulus with merely five to six variables, but without an additional variable to account for nonlinear hardening, model variables in a continuous range. Experimental validations on several kinds of materials have revealed that the new model has improved fidelity both in fitting the experimental measurements and in forecasting the relaxation modulus beyond the experimental regime, compared to the generalized Maxwell model or the Prony series, which is the most commonly employed model for solid matters. Accurate forecasting of the modulus might be extremely beneficial, since laboratory assays can only cover a narrow band of decreased frequency or time. Moreover, the new model of Xu can predict E(t) beyond the experimental region and is more precise with a smoother curve compared to the Prony series. The forecasted curve records the glass transition more obviously and uniformly than the Prony series. In contrast, the Prony series attempts to provide an exact fit just to existing experimental data, but it can either overestimate or underestimate modulus levels beyond the experimental region, probably leading to erroneous E₀ or E_∞ values. The competition between the new model and the Prony series (or the generalized Maxwell model) is equitable, since the same optimization scheme has been adopted to define/adjust the modelling variables. Using the identical number of model variables, the suggested model obtained a more definite solution compared to the Prony series. With a fairly very high number of the terms of the generalized Maxwell model obtained equal or more than 30, its accuracy for data fitting can be enhanced, whereas it remains elusive whether the data prediction beyond the experimental limit is accurate or not. However, a pre-smooth method can be employed to elevate the fitting precision of the Prony series (Park and Kim, 2001). The new model might be considered superior to the generalized Maxwell model or the Prony series if the following issues are taken into account. Firstly, a model, such as the new one, with fewer model variables that meets the fitting precision is frequently favored for simplicity. Secondly, a high number of model inputs in the Prony series (generalized Maxwell model) can generate more uncertainty with non-unique solutions for the determination of the model inputs utilizing mathematical optimization techniques. Thirdly, it is harder to account for the physical phenomenon for the model with a comparatively much higher number of model variables, apart from improving the precision for fitting the experimental results. Fourthly, the presented new model provides higher flexibility, such as it acts a standard solid-model when α = 1, and fifthly the nonlinear strain-hardening phenomenon can be included in it.

Consequently, the new model can be employed as an alternative technique to account for the viscoelastic properties of a huge spectrum of solid materials to further elevate the accuracy of the prediction of data. The generalized Maxwell model cannot model the specific or abnormal physical characteristics, such as a stress overshot of distinct materials covering structural glasses (Wisitsorasak and Wolynes, 2017) and amorphous solids (Jiang et al., 2015).

Application of the Viscoelasticity on Cancer

Living matter, including (cancer) cells, tumor extracellular matrices, and entire tissues, represent soft and complex biomaterials. It is therefore a great challenge to characterize them mechanically. However, it has turned out that viscoelasticity is an inherent characteristic of tissues, as with all polymers and elastomers, and can also be impacted through fluid flow across the porous matrix architecture of tissues. Due to this fact, hemodynamics and mechanical properties have to be investigated at the same time, when examining whole tissues. In this regard, even on larger length scales, such as the organic level, viscoelasticity can be detected, since the components of organs exhibit viscoelastic responses. Apart from physiological cell migration, tissue morphogenesis and organ development, the viscoelasticity of extracellular matrices plays a fundamental role in the progression of cancer (Rozario et al., 2009; Tetley et al., 2019). Therefore, the viscoelasticity of tumor extracellular matrices can be modeled firstly, by cell-based and energy minimization in Vertex models (Manning et al., 2010; Staple et al., 2010; Bi et al., 2014) or cellular Potts models (Vroomans et al., 2015) and secondly, by topological models based on cell contact networks of non-confluent tissues, including embryonic and cancerous tissues (Douezan et al., 2011; Mongera et al., 2018, 2021; Petridou et al., 2019, 2021).

However, viscoelasticity in biological environments, including heathy and diseased states, has only recently begun to be considered important. Specifically, the cell mechanics have been linked to multiple human diseases or disease stages (Huang and Ingber, 2005; Lim, 2006; Lee and Lim, 2007; Fuhrmann et al., 2011; Maciaszek et al., 2011; Prabhune et al., 2012; Efremov et al., 2014). For instance, the red blood cells that are infected with malaria raise their stiffness and increase their stickiness, both of which is rather not supportive for the transport of oxygen, and may cause subsequently severe anemia, coma or finally organismic death (Lim, 2006). A similar phenomenon can be seen in solid cancers, where the oxygen transport is often severely impacted by an altered mechanical microenvironment (Le Maout et al., 2020). For this reason, the accurate measurement of the mechanical features of the tumor extracellular matrix (see below) can be very decisive for the diagnosis of human diseases and the improved comprehension of biological processes in cancer, such as metabolism.

In cancer, it is particularly difficult to determine the beginning of a malignant course and to predict it at all. Therefore, the different stages of malignant progression must be characterized by structural, molecular, or mechanical markers. However, there are not many such markers which are then also generally valid. Therefore, the different stages of malignant progression of cancer have been described by general suggestions. Specifically, in cancer disease, the initiation, growth and progression of solid cancers relies on specific hallmarks that had been identified over 2 decades ago in 2000 (Hanahan and Weinberg, 2000) and even refined a decade later then in 2011 (Hanahan and Weinberg, 2011). These milestones still disregard the mechanical properties of the cancer (Mierke, 2014). Specifically, the mechanical properties of cancer cells and their microenvironment should be included as milestones. Solid cancers and cancer cells cannot be treated as isolated entities that only feel their cellular neighbors without any contact to extracellular matrix molecules, embedded factors or embedded other cell type or structural and mechanical cues. Cancer is not just a collection of specific cells that divide, invade, and spread in a random manner. Instead, cancer is a multi-layered accurately fine-tuned event that demands the entire organism, which acts on the process of cancer development and progression. The malignant course of tumor diseases should also be included in the analysis of mechanical characterization. The transformation of a cancer cell from a benign phenotype to an invasive or metastatic entity entails both biological factors, such as up- or down-regulation or inhibition of the expression of certain genes and cancer markers (Vogelstein and Kinzler, 2004; Simpson et al., 2005), and physical elements, such as modifications of cell and tissue architecture (A. Hall, 2009; The Physical Sciences - Oncology Centers Network, 2013; Wirtz et al., 2011). Lately, mechanical alterations of cancer cell phenotype have been conceived as an important part, with changes in cell forces playing a crucial role (Kumar and Weaver, 2009). Hence, it can be deduced that specific hallmarks, termed systemic hallmarks, addressing these points needs to be postulated (Paul, 2020). The organismic level is excluded here and the focus is set towards the structural and mechanical interplay between the tumor extracellular matrix microenvironment and cancer cells. However, tissue-level characteristics, especially those addressing mechanical characteristics of tumor extracellular matrices, are still underrepresented and need special attention in relation to the success of tumor treatments.

Several biophysical analyses have been performed to identify the mechanical properties of individual cells. In most of the studies, the viscoelastic properties of cancer cells have been compared to healthy counterparts or treated with pharmacological substances that impair cytoskeletal component assembly/disassembly or functions. For example, viscoelastic properties of human cancer cells, such as lung, skin, breast and liver, and normal cells have been investigated using micropipette aspiration technique (Xie et al., 2019). It turned out that the cancer cells are more deformable and their viscoelastic parameters are decreased compared to normal cancer cells (Xie et al., 2019). Atomic force microscopy (AFM) has been employed to benchmark the viscoelastic characteristics of human mammary epithelial cells of varying metastatic capacity in both their adherent and suspended contexts (Nematbakhsh et al., 2017). Notably, cell elasticity has been determined by spatial mapping of the elastic modulus with the force indentation technique, and cell viscosity has been measured based on stress relaxation (Nematbakhsh et al., 2017). The dynamic change in cell mechanical properties, such as the elevation in cell deformability, is directly connected to the development of a transformed phenotype from a non-cancerous, benign cell to a cancerous, malignant cell (Ketene et al., 2012). The reduction in the quantity of actin in the cytoskeleton and its organization is linked in a direct way to the alterations in the biomechanical characteristics of the cells.

In cancer disease, the viscoelastic characteristics of mammalian cells seem to rely on the biological state, such as whether the cells are in a more epithelial or mesenchymal state or on the transition from epithelial-to-mesenchymal in the malignant progression of cancer (Y. Yang et al., 2019). Therefore, certain viscoelastic characteristics can turn out to become a reliable and useful physical biomarker for diseases, such as cancer or others, and age-specific changes (Agyapong-Badu et al., 2021; Efremov et al., 2020; K.; Park et al., 2019). All of which can be impacted by the viscoelastic characteristics of the tumor extracellular matrix scaffold or age-associated changes of the extracellular matrix scaffold. Moreover, the viscoelastic properties of cells have evolved as critical biomarkers of disease condition and progression (Bao and Suresh, 2003). The simplest attempt to specify viscoelastic characteristics of cells explores two main parameters: Stiffness and viscosity, which typify the elastic and dissipative nature of a cell’s reaction to stress (Moeendarbary and Harris, 2014). Elastic response has been implicated as a marker of cancer cells (Cross et al., 2008) or the metastatic potency of cancers (Xu et al., 2012), and has been strongly connected to cell migration in embryogenesis (Barriga et al., 2018). Cell viscosity has been associated with several biological events, such as the porosity and deformability of erythrocytes (Lim et al., 2006), diffusion (Einstein, 1905; Wojcieszyn et al., 1981), and the condition of cells in disease (Eze, 1992; Zakim et al., 1992). In addition, the viscoelastic properties of the tumor extracellular matrix environment may on top alter the viscoelastic response of cancer cells, since these cells are in direct interplay with the tumor microenvironment.

Specific advances have been made in the study of cancer cell migration and invasion: The simple investigation of isolated of cancer cells has been overcome by advancing cellular assays for analyzing cancer cell behavior and function in vitro models from simple 2D models without a suitable tumor microenvironment to a more sophisticated 3D microenvironment. The behavior indicates that the tumor extracellular matrix is considered important and therefore, in addition to structural, mechanical characterization seems to play a role. In this regard, the tumor extracellular matrix is changed at the different levels, such as biochemical, architectural, biomechanical and topographical length scales, and therefore, there is an exponential raise in studies that incorporate the matrix in solid tumors (Bissell et al., 1982; Herbison et al., 2019; Cox, 2021).

Another step in the right direction is the analysis of the dynamic performance of cells rather than just endpoints that further advances these 3D assays to 4D assays. For the dynamic analysis of the mechanical properties, the viscoelastic behavior of the tumor environment and that of the cancer cells is of special relevance. Moreover, the mutual interaction between the tumor microenvironment and cancer cells must be considered, which requires simultaneous analysis of structural and mechanical phenotypes (Mierke, 2019, 2020). By characterizing the tumor extracellular matrix environment, an emphasis is on the phenomenon of viscoelasticity and hence an introduction to it is provided in the following section.

From a physical point-of-view, growth, migration and invasion, intravasation, blood or lymphoid circulation, arrest/adhesion, and extravasation of cancer cells demand distinct cell-mechanical characteristics that contribute to the survival of cancer cells and subsequently full execution of the metastatic cascade. In this regard, metastatic cancer cells are generally softer than their non-malignant equivalents (Guck et al., 2005; Fischer et al., 2017; Lv et al., 2021), and high deformability of both the cell and the nucleus is hypothesized to confer a substantial benefit in terms of the metastatic status (Fischer et al., 2020). Nevertheless, it remains ambiguous whether there is a more fine-tuned but steady mechanical state that accounts for all the mechanical characteristics necessary for survival across the cascade, or whether cancer cells must dynamically fine-tune their characteristics and intracellular constituents at every new stage.

Viscoelastic Properties of the Tumor Extracellular Matrix

The viscoelasticity of the tumor extracellular matrix arises due to covalent nature of crosslinking that considers the extracellular matrix as an elastic-like network (Muiznieks and Keeley, 2013) and the strain-stiffening response of collagen scaffolds, which emerges from the network level and specifically its connectivity (Wen and Janmey, 2013; Han et al., 2018; Jansen et al., 2018). The nonlinear characteristics of tumor extracellular matrices emerges from strain of only 10% increase where stiffness increased by 100 times before a rupture of the scaffold occurs (Sharma et al., 2016).

However, the tumor microenvironment is not simply a pure matrix scaffold. Instead, the tumor microenvironment represents a dynamic tapestry of cancer cells enclosed by the extracellular matrix and a plethora of stromal cells, among them fibroblasts, hematopoietic and lymphoid cells, immune cells, and multiple tissue-specific cells, including adipocytes, endothelial cells and pericytes. Due to the malignant transformation of the normal tissues to cancerous tissues, such as the progression of the primary solid tumor to cancer cell invasion, cancer dissemination, and consequently metastasis, the mechanical characteristics of the tumor are largely impacted (Kumar and Weaver, 2009; Gensbittel et al., 2021). These tumor microenvironment alterations are driven by increased contractility of cancer cells, the enlargement of the expanding tumor mass, and changes of the material characteristics of the local tumor extracellular matrix components including viscoelastic properties. Consequently, the physical characteristics of a tissue, such as the stiffness and structure of the extracellular matrix, potentially exert a pervasive impact on cell performance and, in the end, on tissue organization and function. Simple experiments with substrates of different stiffness to which normal thyroid cells and thyroid cancer cells have been attached yielded different results. Normal thyroid cells adjusted their mechanical characteristics to substrates with varying stiffness, while cancer cells were influenced less by the stiffness of the microenvironment (Rianna and Radmacher, 2017). Therefore, it seems important to select not pure elastic substrates but viscoelastic substrates that better represent the natural tumor environment in order to study the cancer cell response to them.

The characterization of viscoelastic materials can be performed in multiple ways that depends on the different exertion of stimuli, such as stepwise testing (creep and stress relaxation), ramp-type testing or sinusoidal testing.

Stepwise Testing (Creep and Stress Relaxation)

In the past decades, various biophysical approaches have been designed to quantitatively examine the viscoelastic properties of cells (Addae-Mensah and Wikswo, 2008), encompassing mechanical micropipette aspiration (Hochmuth, 2000), optical tweezers (Hoffman et al., 2006), magnetic tweezing cytometry (Fabry et al., 2003; Hoffman et al., 2006), magnetic tweezers (Kollmannsberger et al., 2011; Mierke et al., 2011) and AFM (Efremov et al., 2020; Fischer et al., 2017; B.; Wang et al., 2013). Compared to a number of these approaches, AFM provides the major benefit of being able to directly probe living cells under their physiological constraints with a force and spatial resolution on the scale of piconewtons and nanometers, respectively. The simplest way to describe the mechanical properties of viscoelastic materials is stepwise stimulation, such as creep and stress relaxation experiments. The steps can be performed as single measures or they could be performed as multiple measures with the same force or varying force, such as descending or ascending.

Sinusoidal Testing

Apart from a single step or multiple step probing, a range of frequencies can be applied. The deformation of the material is recorded in reaction to a sinusoidal load at a specific frequency, where the range of amenable frequencies is delineated by the capacity of the instrument employed, ranging characteristically from 0.1 to 200 Hz for most standard commercial AFMs (Alcaraz et al., 2003; Lu et al., 2006). Typically, measurements of viscoelasticity with AFM can be classified crudely into vibrational (oscillatory) frequency measurements (Chyasnavichyus et al., 2014) and time-dependent measurements of penetration depth, such as stress relaxation (Chyasnavichyus et al., 2014). In particular, the measurement of oscillatory frequencies is the most widely employed, but measurements in liquids are subject to hydrodynamic forces that are heavily impacted by the experimental conditions (Radmacher et al., 1996; Alcaraz et al., 2002).

More recently developed high-speed AFMs possess up to 100 kHz (Nia et al., 2013; Rigato et al., 2017). In distinction, time-dependent mechanical AFM experiments utilize a quasi-steady-state stress-relaxation approach, but this necessitates a fit of the force-impact curves with a predetermined phenomenological model to identify the “pseudo” material properties that delineate the viscoelastic reaction of the cells (Fischer-Cripps, 2004; Darling et al., 2007). In principle, these variables vary depending on the experimental procedure, such as holding time, and are subject to fitting mistakes related to the selected models and the estimation of the unknown variables (B. Wang et al., 2013). Recently, a novel AFM microrheology method has been developed to identify the linear viscoelastic characteristics of complex materials and living cells across five continuous frequency decades, such as 0.005–200 Hz, based on a simple stress relaxation nanoindentation sensing with a standard AFM instrument (Chim et al., 2018). Additionally, the experimental measurements can be immediately analyzed avoiding the requirement to interpret the experimental measurements with any pre-conceived viscoelastic model. These findings are in perfect accordance with traditional oscillatory bulk rheology tests in hydrogels. Apart from AFM, the optical tweezer can be employed to determine oscillatory measurements of intracellular components within cells and a single-cell parallel plates rheometer to probe overall cellular mechanics including viscoelasticity in an oscillatory manner (Mathieu and Manneville, 2019; Alibert et al., 2021).

Bulk (Tissue) or Local (Cellular) Probing

In addition to the mechanical probing method, the measurements can be performed as bulk or local analysis. An interesting experimental finding is that the mechanical characteristics of the microscale elements of cell and tissue viscoelasticity, such as the components of the cytoskeleton and the cells, do not generally correspond to the macroscale mechanical characteristics of cells and tissues (MacKintosh et al., 1995; Broedersz and MacKintosh, 2014; Petridou et al., 2021). In this way, macroscopic viscoelasticity, such as scaffold geometry and local topology of filaments, often displays nonlinear alterations that are not evident at the microscopic level, such as mechanical characteristics of the biopolymer filaments creating the cytoskeleton. (MacKintosh et al., 1995; Broedersz and MacKintosh, 2014; Mongera et al., 2021). Experimental evidence has been provided for those examples, such as the stiffening response of the cytoskeletal scaffolds (Gardel et al., 2004, 2006; Mierke et al., 2011; Pritchard et al., 2014), phase transitions in the energetic expense of cellular motility (Mongera et al., 2018) or sudden alterations in the viscosity of tissues (Petridou et al., 2019). Therefore, the mechanical loading capacity of individual microscopic elements cannot explain the macroscopic viscoelastic changes. Consequently, the pattern of interaction between the elements must be analyzed. There are at least three theoretical approaches that can be employed for this discrepancy (Table 1).

TABLE 1

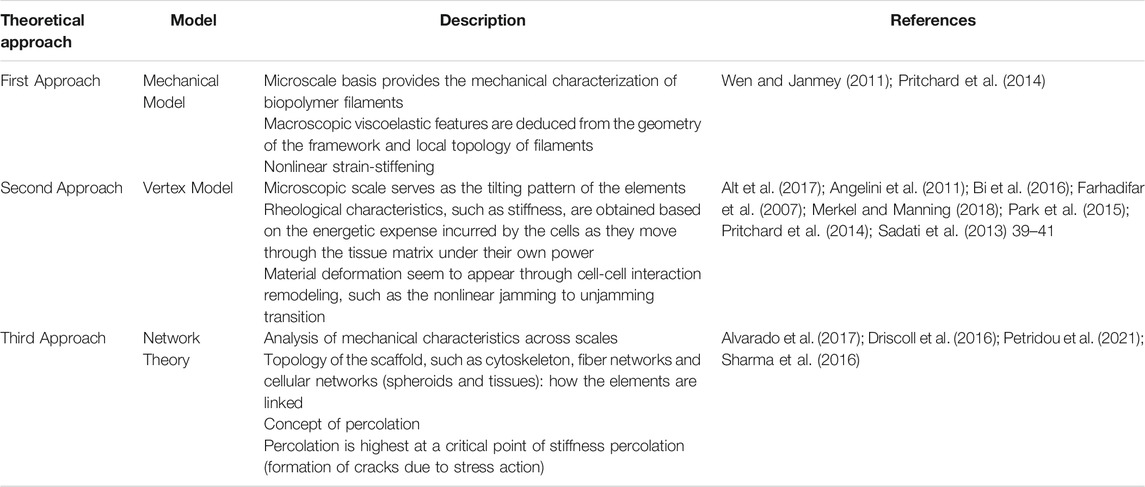
TABLE 1. Three theoretical approaches to describe the discrepancy between macroscopic and microscopic mechanical characteristics of tissues.

Moreover, the surrounding extracellular matrix cannot simply be considered separately from the embedded cancer cells. In the following, the influence of the viscoelastic tumor environment on the structural and mechanical properties of the cells is discussed.

Environmental Matrix Viscoelasticity Acts on Cells

Cell and tissue reactions result from forces created by the cell on its own that are opposed by the viscoelastic or active characteristics of the extracellular matrix or ambient cells. Moreover, the extracellular matrix environment can impose mechanical cues on cells and tissues. The focus is placed here on the effect of the environmental matrix on cancer cells. Specifically, the following question is raised: How will cells react when they adhere to surfaces and matrices that withstand deformation, when cells tug or push on them? However, the structural, molecular and mechanical properties of cells seem to be determined by the microenvironment of cells. The response of living cells is well-known to be influenced by both elastic (Discher, 2005; A. J.; Engler et al., 2009) and inelastic (Chaudhuri et al., 2015, 2016) mechanical characteristics of the microenvironment. The inelastic mechanical characteristics of the extracellular matrices can be either viscoelastic or plastic (Wang H. et al., 2014; Liu et al., 2016), where the latter results in long-term non-reversible deformations. Because the architecture and stiffness of the extracellular matrix affect cell spreading, movement, and differentiation (Discher, 2005; A. J.; Engler et al., 2009), the extent of matrix plasticity upon mechanical rearrangement is probably an influential factor in defining cell performance. For instance, the persistent alignment of fibers in the extracellular matrix leads to the persistence of mechanical evidence that can affect the alignment and migration of normal control cells (Dickinson et al., 1994) and cancer cells (W. Han et al., 2016; Provenzano et al., 2008).

Over the course of the last 2 decades, substantial scientific evidence has established that the elasticity or stiffness of the extracellular matrix governs essential cellular processes, among them spreading of cells, cell growth, proliferation, migration, differentiation, and assembly of organoids (Discher, 2005). Linear elastic polyacrylamide hydrogels and polydimethylsiloxane (PDMS) elastomers layered with extracellular matrix proteins are frequently employed to evaluate the effects of stiffness, and it is commonly hypothesized that the outcomes of these types of investigations will mimic the impacts of the mechanical environment encountered within cells in vivo. However, tissues and extracellular matrices typically have no linear elasticity (Elosegui-Artola et al., 2014), instead they display much more complicated mechanical characteristics, incorporating viscoelasticity, which is a time-dependent response to strain or deformation, mechanical plasticity and nonlinear elasticity. In the following the intricate mechanical characteristics of tissues and extracellular matrices is presented, the impact of extracellular matrix viscoelasticity on cells is debated, and the possible impact of viscoelastic tumor extracellular matrix scaffolds in cancer treatment is discussed.

Extracellular matrices and entire tissues and cannot be treated as linearly elastic materials because they manifest far more complexity in mechanical response, involving viscoelasticity (Abidine et al., 2021; Martinez-Garcia et al., 2021) mechanical plasticity (Buchmann et al., 2021) and nonlinear elasticity (Elosegui-Artola et al., 2014). Hence, matrix viscoelasticity seems to govern essential cellular processes and can foster types of behaviors not evident in cells that are cultured in purely elastic hydrogels in both two- and three-dimensional culture surroundings (Charbonier et al., 2021). Matrix viscoelasticity seems to be important in revealing the complex interplay between cells and their environment at cell-matrix interaction sites and how these interactions variably impact mechanosensitive molecular signaling paths in cells (Tan and Song, 2021). Specifically, the collagen density can foster the progression of cancer (Provenzano et al., 2008).

In this context, hydrogels with dynamic characteristics, which are accomplished either through the integration of degradable structural compounds or reversible dynamic cross-links, permit efficient accommodation of cells to the matrix and aid in the achievement of the connected cellular specific functions (B. Yang et al., 2021). Since, it is well known that cancer cells can alter their extracellular matrix environment by secreting of molecules (Dhar et al., 2018; Kano, 2015), release of exosomes (Hoshino et al., 2015), degrading (Stephens et al., 2019), re-orientating (aligning) (B. Lee et al., 2017; Levental et al., 2009; Provenzano et al., 2002; Vader et al., 2009) or cross-linking the matrix scaffold (Levental et al., 2009), it seems to be quite obvious that in turn the tumor microenvironment modifies the properties of cancer cells, including their structural, morphological and mechanical properties. Consequently, many tissues have nonlinear elasticity and do not exhibit the straightforward linear relationship between stress and strain that is typical of most conventional Hookean solid materials, such as concrete or steel. Similar to a nonlinear elastic material, a coiled tether is relatively simple to stretch at first, but gradually tends to get more challenging as it is fully stretched. In particular, networks of cross-linked collagen fibers are assumed to be accountable not merely for tissue viscoelasticity, but also for nonlinear elasticity (Figure 4). In both shear and tensile strains, collagen meshes act similarly to linear elastic materials up to a certain limit of strain; beyond this point, they stiffen as the fibers orient themselves in the direction of peak tensile stress (M. S. Hall et al., 2016; Licup et al., 2015; Steinwachs et al., 2016; Storm et al., 2005; Vader et al., 2009; Wang H. et al., 2014). The alignment of the fibers, such as collagen fibers, can facilitate force transfer over hundreds of micrometers, leading to improved long-range cell communication (Y. L. Han et al., 2018; Wang H. et al., 2014). A theoretical fiber mesh model of collagen has revealed that tight coupling between deformation modes can lead to much higher stiffening of the meshes under triaxial and biaxial tensile loading relative to uniaxial loading (Eckes et al., 2000).

FIGURE 4

FIGURE 4. Matrix features of tumor extracellular matrices and biological tissues.

For example, the tumor extracellular matrix environment impacts the cytoskeletons of cells that undergo continuous structural remodeling due to highly dynamic perturbations and fluctuations in their entire life cycle and during the development and progression of cancer. The tumor microenvironment induced cell mechanical characteristics have been attributed to multiple cellular physiological tasks, encompassing cell movement (Lautenschläger et al., 2009; Mierke et al., 2011), differentiation of cells and tissues (Nelson et al., 2006), cell adhesion (Kumar et al., 2006; Qian et al., 2008) and the take-up of nanoparticles through a process termed endocytosis (C. Huang et al., 2013; Wang J. et al., 2014; Wang and Li, 2015). All of which can be severely de-regulated and promote the malignant progression of cancer.

Effect of the Tumor Microenvironment on the Cell’s Cytoskeleton and Focal Adhesions

Cell proliferation, differentiation and migration rely strongly on the extracellular matrix mechanical stiffness. Natural extracellular matrices are also known to possess dissipative, including plastic and viscoelastic, characteristics that can in turn modify cellular response. There is growing support for the idea that cells can perceive and respond to the physical characteristics of the extracellular matrix, a capability that is key to events such as spreading of cells (Nisenholz et al., 2014; Chaudhuri et al., 2015), cell migration (Trichet et al., 2012; Shenoy et al., 2016; Sunyer et al., 2016), and cell proliferation (Klein et al., 2009; Trappmann et al., 2012; Swift et al., 2013). Focal adhesions, which tether the cell to the extracellular matrix and act as nodes for the replacement of biological and mechanical cues (Parsons et al., 2000; Cao et al., 2015), are generally believed to be responsible for the cellular mechanosensitivity. Consequently, these physiological boundaries between the tumor microenvironment and cancer cells enable the process of mechanotransduction between these two compartments (Boyle and Samuel, 2016). Thereby, the regulation of cells by tumor microenvironments is feasible, such as through signaling via Rho-associated protein kinase (Boyle and Samuel, 2016). Through the continuous interaction between the extracellular matrix scaffold a matrisome is created (Naba et al., 2012). Although the composition, size and remodeling of focal adhesions is altered in 2D and 3D environments (Cukierman et al., 2001), an emerging concept, which is equally amenable to 2D and 3D extracellular matrices, is that stress relaxation of an extracellular matrix can modify the dynamics of adhesion. When a material is subjected to uniform strain, the relative stress reduces with time, potentially a fast or slow acting phenomenon. Focal adhesions are able to react to either rapid or low-speed stress relaxation of hydrogels (Adebowale et al., 2021). Specifically, cells travel at a minimum on substrates with a modulus of elasticity of 2 kPa, which are elastic or display slow stress relaxation, while traveling extensively on 2 kPa substrates, which display rapid stress relaxation (Adebowale et al., 2021). The arrangement and orientation of the cytoskeleton are highly sensitive to the mechanical and structural characteristics of the matrix, such as Young’s modulus, Poisson’s ratio, and roughness (R. De et al., 2007; Wei et al., 2008). Thus, depending on its physical and mechanical properties, a substrate displays various types of characteristics in reaction to cell aggregation (H.-B. Wang et al., 2000). To put it another way: When a substrate is stiff and inelastic, focal adhesions act as structural connections between the extracellular matrix and the actin cytoskeleton (Guo et al., 2006). A focal adhesion provides a stable physical connection that conveys cell adhesion to the substrate (Iwanaga et al., 2001). In contrary, soft and elastic substrates afford a temporary retention in the cellular matrix (Qian and Gao, 2010).

When interfacing with the substrate, cellular reactions, involving relaxation time and adaptation through changes in fibrous structures, are governed through the local deformability of the matrix (De and Safran, 2008; Hsu et al., 2009). The adaptation of the cell cytoskeleton to the mechanical characteristics of the substrate relies on the polymerization and depolymerization of actin fibers (Nekouzadeh et al., 2008), which operate through focal adhesion proteins located at the cell-substrate interface (De and Safran, 2008).

These insights have yielded knowledge of cell-matrix linkages and how these linkages variously modify mechano-sensitive molecular signaling transduction cascades in cells. Beyond that, these findings propose design directions for the next evolution of biomaterials to mimic the tumor extracellular matrix network, with the aim of tuning the mechanics of tissue and extracellular matrix for in vitro tissue models for cancer metastasis research.

Tumor Extracellular Matrix Environment Is Sensed by Cancer Cells

Cells, such as cancer cells, can sense the mechanical stiffness of their microenvironments through by probing the resistance of focal adhesions toward a retrograde flow of actin that is evoked by intracellular contractions based on myosin filaments (Cao et al., 2017; Case and Waterman, 2015; Plotnikov et al., 2012; Shemesh et al., 2005). Focal adhesions, which function as molecular clutches, impact the motion of intracellular structures, such as actin filaments, through offering an adaptable linkages toward the surrounding extracellular matrix (Chan and Odde, 2008; Case and Waterman, 2015). Consequently, the classical motor-clutch model (Chan and Odde, 2008; Bangasser et al., 2013; Bangasser and Odde, 2013) has been proposed that forecasts a biphasic reliance on the cell adhesion-based traction (and subsequently on the spreading of cells) and on the rigidity of the extracellular matrix. Consistent with this, emerging experiments have identified a monotonic enhancement of cell spreading rates along with extracellular matrix stiffness (Ghassemi et al., 2012; Étienne et al., 2015) and this may be linked to reinforcement mechanisms that involve, for instance, activation of adhesion proteins under high environmental stiffness/stress or retention of integrins within the focal adhesions (Elosegui-Artola et al., 2014, 2016).

Besides substrate stiffness, the majority of natural extracellular matrix matters that include biomaterials such as collagen, and fibrin (Roberts et al., 1974), and living tissues (Alcaraz et al., 2003; Deng et al., 2006; Verdier et al., 2009) are inherently viscoelastic and manifest a robust frequency-dependent mechanical responsiveness. Moreover, the spreading of cells can be enhanced through stress relaxation of a cell culture substrate, such as alginate and polyacrylamide, an effect that seemed to rely on the elastic modulus of the substrate (Cameron et al., 2011; Chaudhuri et al., 2015). A local redistribution (causing enhanced ligand density) of the matrix occurred upon deformation to account for this (Chaudhuri et al., 2015), which is consistent with a plastic rather than a viscous reaction. Conversely, experiments have also led to the hypothesis that viscosity has a marginal impact on cell spreading (Chaudhuri et al., 2020). Nonetheless, it is unsure how a merely viscoelastic substrate can have divergent effects on cell spreading, in large part because few theoretical models (Chaudhuri et al., 2015; Gong et al., 2018; De and De, 2019) are in place to clarify the physical mechanisms that direct the cellular reaction to viscoelasticity. To further resolve this crucial concern, a systematic approach to investigate how cell spreading is governed by the viscoelastic constituents of the extracellular matrix has been undertaken by means of analytical mean-field analysis and direct Monte Carlo computational simulations (Van Liedekerke et al., 2015; Chaudhuri et al., 2020). In specifically, by considering the tumor extracellular matrix as a standard linear viscoelastic solid, there is evidence that an intermediate level of viscosity is capable of facilitating cancer cell spreading when the stiffness of the extracellular matrix is fairly weak, which mirrors the circumstance that the substrate relaxation time under such conditions is somewhere between the coupling bond time scale and its typical bond lifetime. In other words, viscosity acts to rigidify soft substrates, which encourages cell adhesion to the extracellular matrix and facilitates consequently cell spreading. As with high stiffness, the large stress carried by the couplings elicits an enhancement of their binding levels as well as an augmentation of integrin tightness (clutch amplification), thereby rendering the cell contribution to substrate stiffness to become a saturated response, and viscosity no longer to be an issue (Figure 5). These datasets can be displayed in heat maps of the propagation response in the parameter volume defined by the substrate and cell time scales.

FIGURE 5

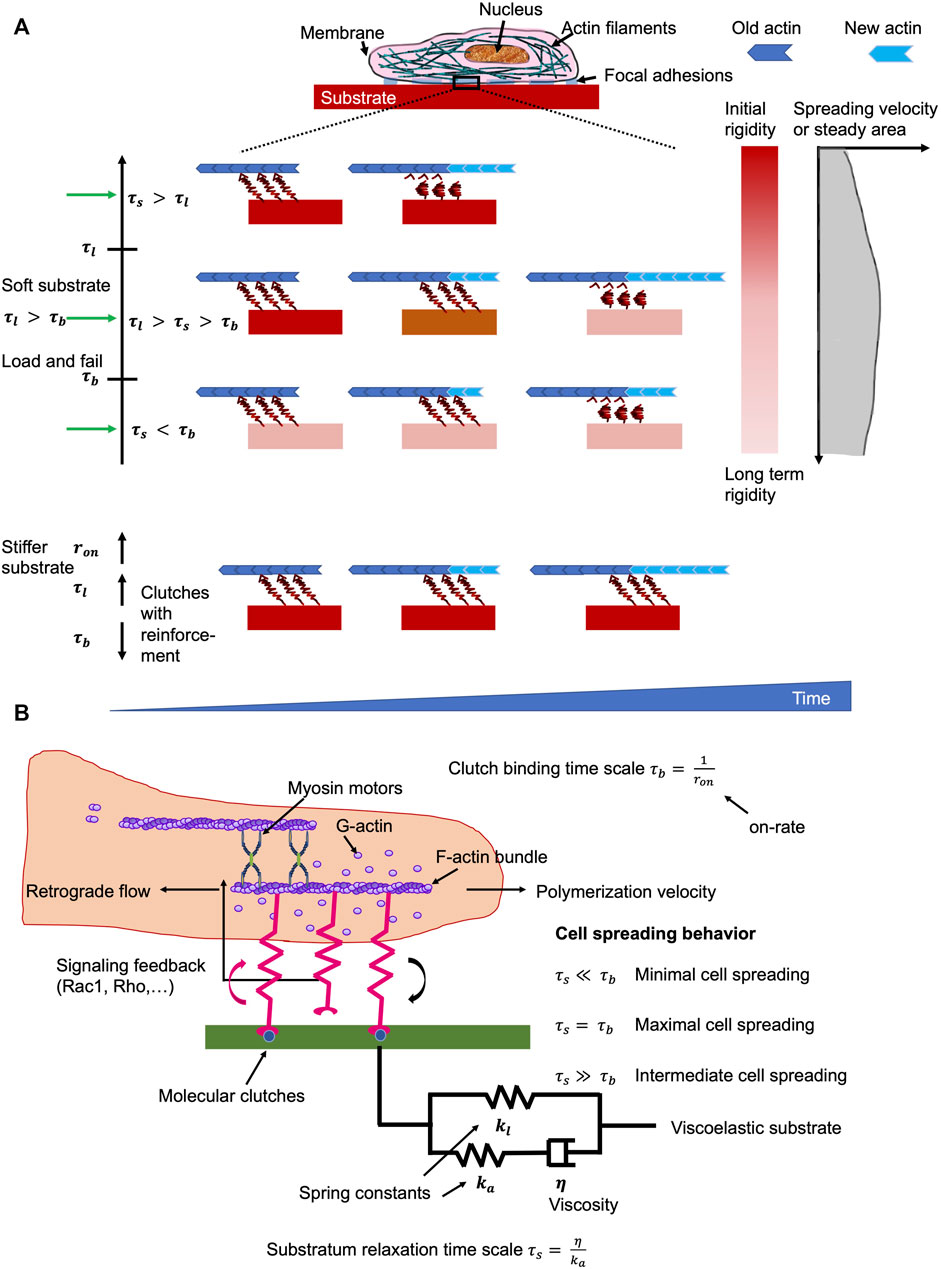
FIGURE 5. Matrix environments impacts cell mechanics. The ideal viscosity minimizes the retrograde flow of actin whereby the turnover of focal adhesions is prolonged on soft materials. (A) Effects if the substrate viscoelasticity toward the characteristics of cells in relation to clutch binding, ${\tau }_b$, substrate relaxation, ${\tau }_s$, and cellular life timescales ${\tau }_l.$ In the load and fail domain with ${\tau }_l> {\tau }_b$, the spreading of cells is maximal when ${\tau }_l> {\tau }_s >{\tau }_b$. It means that the cells sense a surrounding material with high initial stiffness, which vastly relaxes after the clutch engagement. However, when the viscosity of the material is low, such as ${\tau }_s< {\tau }_b$, cells can merely perceive long-term rigidity that elevates the lifetime of focal adhesions, but did not impair the retrograde flow of actin. When the viscosity of the substrate is relatively elevated, such as ${\tau }_s> {\tau }_l$, the cells perceive solely the starting rigidity, which did not alter the lifetime of focal adhesions, but lead to premature clutches that cannot fulfill their function. In contrast to rigid extracellular matrices, a high number of clutches is created evoked by reinforcement of clutches that cause subsequently prolonged lifetimes of focal adhesions, which restricts the retrograde flow of actin and improves the rate of spreading. (B) Sketch of the molecular clutch model that is employed for viscoelastic materials. Optimal cell spreading is gained when the timescale for stress relaxation is close or equal to that of the clutch binding timescale. Out of this balanced state, the cell spreading is impaired.

Design of Hydrogel Scaffolds That Mimic Tumor Microenvironments

Mimicking Viscoelastic Characteristics of Tumor Extracellular Matrix Environments With Hydrogels

The extracellular matrix not only offers structural sustenance and governs functional characteristics, but also performs an essential part in tissue physiology through interaction with cells and trafficking of interstitial fluid. The simplest way to mimic the tumor microenvironment appears to be cell culture assays using an extracellular matrix scaffold with the aid of hydrogels. Hydrogels are soft, water-based polymer gels that are increasingly used to fabricate free-standing fluidic devices for tissue and biological engineering applications. Specifically, hydrogel materials and other biopolymer scaffolds can be produced that created almost weak linkages, such as the dynamics of physical cross-links between polymers. For instance, viscoelastic poly ethylene glycol (PEG) hydrogels form dynamic covalent hydrazone bonds, borate bonds or thioester exchange (McKinnon et al., 2014; Brown et al., 2018; Tang et al., 2018). In natural alginate gels, weak ionic cross-linking creates viscoelastic gels (Zhao et al., 2010). Moreover, viscoelastic hyaluronic acid-based hydrogels can be generated through employing hydrazone bonds or guest-host cross-links (Lou et al., 2018; Loebel et al., 2019). Alternatively, weak-bonds can be produced by engineering, namely, the so-called programmed peptide-based hydrogels (Dooling et al., 2016). Weak-bonds within these matrices can be altered in their viscoelasticity independently of their initial elastic modules through the combination of the following elements: molecular weight of the constituent polymer, coupling of inert molecules toward the constituent polymer, which functions as spacers, affinity of weak bonds, relationship between weak and covalent bonds and the total amount of bonds (Chaudhuri et al., 2016; Dooling et al., 2016; Lou et al., 2018; Loebel et al., 2019; Nam et al., 2019; Richardson et al., 2019; Vining et al., 2019). These weak bonds have been reported to be possibly viscoplastic and explore a viscoelastic transition (Müller et al., 1995; Wang and Zocchi, 2011). Single or double networks generated by a combination of covalent and weak cross-links can possibly display viscoplasticity at the bulk scale due to the molecular structure and degradation-evoked alteration of these hydrogels can alter their viscoelasticity (Narasimhan et al., 2021). Specifically, these hydrogels are made of different scaffold structures that offer the accurate guidance and fine-tuning of the dissipation of hydrogen bonding. The hydrogels with adjustable dissipative characteristics are achieved by photopolymerization of a second polymer contained within a preshaped crosslinked hydrogel grid of poly (acrylamide). Specifically, the second networks are prepared with distinct structures and capacities for hydrogen bonding to the first network, which are linear poly (acrylic acid) for the first network and branched poly (tannic acid) for the second network. For example, gels with a second network composed of poly (tannic acid) displayed increased stiffness (0.35 ± 0.035 MPa) and elevated toughness (1.64 ± 0.26 MJ m⁻³) compared to the poly (acrylic acid) counterparts. Moreover, a strategy was outlined for the preparation of hydrogels in which dissipation (loss modulus) can be adjusted separately from elasticity (storage modulus) and which are convenient for cell culture purposes. It can be envisioned that this modular approach to hydrogel fabrication will find uses in customized substrates for cell culture assays and in load-bearing tissue engineering implementations.

Since both the viscoelastic and viscoplastic characteristics of hydrogels can be impacted by poroelastic effects, they have to be taken into account. Tumor extracellular matrix scaffolds display physical interactions of water with other phases that are inevitably to characterize these systems. In specific, the theory of poroelasticity affords a means of delineating the mechanical response based on these interactions, by modeling a porous material that possesses an elastic solid skeleton with fluid-saturated interconnected pores. Using this model, the microstructural variables, phases and interactions, can be compared to scaled-up continuum mechanical characteristics that can be experimentally determined. In fact, their high water retention accounts for this, as the extensive migration of water molecules through their porous matrix permits the stress to relax under a constant load, a phenomenon commonly referred to as poroelasticity (Chaudhuri, 2017; Caccavo et al., 2018). Nevertheless, in most investigations dealing with the role of time-dependent mechanical characteristics on cell response, hydrogels are treated as simple viscoelastic systems, while poroelastic properties are not incorporated. This simplification is tolerable provided that the solvent diffusion time is much larger than the time scales of viscoelastic relaxation and cellular processes under investigation (Caccavo et al., 2018).

Theoretical Predictions of Tunable Viscoelasticity and Their Impact on Cellular Microenvironments

The theoretical predictions involving analysis of the viscoelastic relaxation time scale are in outstanding accord with previous monitoring and furnish the groundwork for interpreting experiments in which extracellular matrices with tunable viscoelastic characteristics have been constructed by two distinct techniques (Chaudhuri et al., 2020). For example, to simulate focal adhesion binding and cell spreading characteristics, there are both analytical and Monte Carlo methods that can be employed. Instead of utilizing a stochastic lattice model (Figure 6) (Chaudhuri et al., 2015), a simple linear viscoelastic standard substrate has been combined with the motor-coupling model to illustrate how multiple viscoelastic material variables, including long-term stiffness, additional stiffness, and viscosity, adjust cell spreading. More specifically, viscosity at a low long-term and additional stiffness encourages cell spreading, and peak cell spreading is accomplished at an intermediate viscosity level. In contrary, the viscosity has a marginal influence on the spreading when the long-term stiffness is high. This unresponsiveness of cell spreading toward viscosity in this regime is due to the coupling enhancement phenomenon, which results in saturation of the limited couplings that can be built on stiff extracellular matrices. Such a strengthening mechanism (under high coupling load), nevertheless, may not be available in specific cell types such as neurons (Koch et al., 2012; Swaminathan and Waterman, 2016), eventually causing repressed cell spreading when the substrate turns very stiff.

FIGURE 6

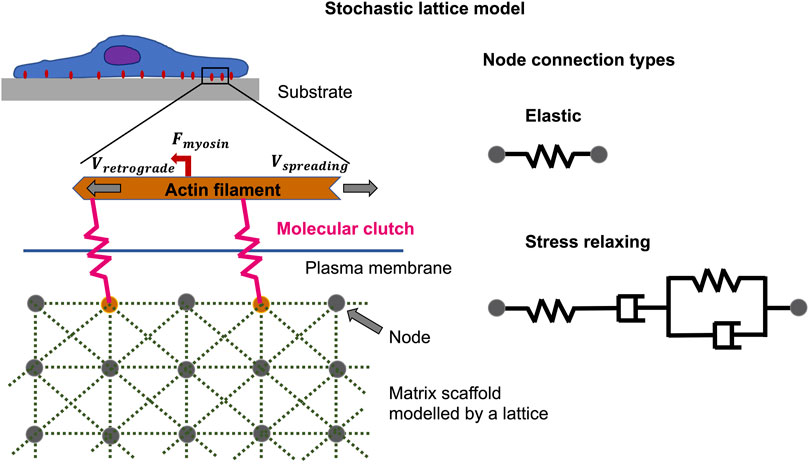
FIGURE 6. Non-equilibrium phenomena can be described by the stochastic lattice model, such as cell spreading on elastic or viscoelastic tumor microenvironments with low stiffness. The polymerization of actin at the cell front edge is tethered to the underlying substrate by molecular clutches that impede the retrograde flow of myosin motors acting on actin filaments. The elastic substrate is modelled by multiple nodes coupled by Hookean springs, whereas the viscoelastic substrate, which exhibits stress relaxation, is modelled by Burgers model elements.

Through the detection of the mechanism through which extracellular matrix viscoelasticity impacts cell spreading over a wide range of material properties, the analytical model by Chaudhuri seems to provide a valuable resource for the designing of biomaterials that maximize cellular adhesion and mechanosensing. Most notably, intermediate viscosity is determined to actually optimize cell spreading on soft substrates, whereas cell spreading on stiff substrates is not affected by viscosity. This knowledge could then be exploited to engineer dissipative biomaterials for optimized management of cellular performance (Gong et al., 2018). In parallel with stiffness, the viscosity of the extracellular matrix decisively effects the performance and functioning of cells. But the mechanism underlying such mechanosensitivity to viscoelasticity still is elusive. Thus, the evolution of motor coupling dynamics, such as focal adhesions, occurring between the cell and a viscoelastic substrate, has been systematically explored through analytical techniques and a direct Monte Carlo simulation. When the stiffness of the extracellular matrix is less, maximum cell spreading is obtained at an ideal viscosity level where the substrate relaxation time is intermediate between the coupling bond time scale and the characteristic bond lifetime. Specifically, viscosity acts to rigidify soft substrates on a time scale more rapid than the disengagement rate, which promotes cell adhesion to the extracellular matrix and increases cell spreading. Alternatively, for substrates that are rigid, this model predicts that viscosity has no effect on cell spreading because the bound couplings are at saturation due to the increased stiffness. The model has been validated and verified by experimental testing on three distinct material systems, and provides an interpretation of the varying observed implications of viscosity for each substrate. By grasping the mechanism through which substrate viscoelasticity governs how cells spread over a broad array of material properties, this analytical model offers a valuable guide for the construction of biomaterials for cancer research that maximize adhesion and mechanosensing of cells.

Fine-Tuning of Tumor Extracellular Matrix’s Structure, Architecture and Mechanics, Including Viscoelasticity

The easiest way to fine-tune extracellular matrix models, such as collagen hydrogels, is to increase the concentration of collagen type I monomers. More intricate fine-tuning can be performed by adding specific cross-linkers that can be simply chemical cross-linkers, biomolecule-based cross-linkers or cell-derived cross-linkers. All of which varies the overall mechanical properties of tumor extracellular matrix scaffolds employed to explore cancer cell behaviors, such as adhesion and motility. For example, the nature of collective migration of cells has been seen to rely to varying degrees on the density (Gudipaty et al., 2017; Tlili et al., 2018) and motility (Bi et al., 2016; Hayer et al., 2016) of individual cells and intercellular adhesion (Benjamin et al., 2010; Vedula et al., 2012). For instance, in a confluent cell monolayer, an augmentation of cell motility can lead to a transition from a solid to liquid state (Hayer et al., 2016; Malinverno et al., 2017), whereas a breakdown of intercellular links can result in random uncorrelated cell movements (Benjamin et al., 2010; Vedula et al., 2012).

Apart from intrinsic characteristics of cells, extrinsic signals, including geometric confinement of the tumor microenvironment (Tanner et al., 2012; Vedula et al., 2012; Doxzen et al., 2013; Camley et al., 2014; Li and Sun, 2014; Segerer et al., 2015; Lin et al., 2017), chemical factors (Harris et al., 2012) and electric field (Cohen et al., 2014) can additionally impact dynamic properties of cells. Migrating cancer cells in vivo are frequently constrained geometrically by the tumor surrounding environment, such as extracellular matrix or other tumor stroma embedded cells. A typical case is the invasion of cancer cells within the porous peritumoral stroma (Friedl et al., 2012).

The main physical constraints faced by migrating cells in vivo are adhesion (friction), boundary, rigidity of migrating substrates, shear flow of extracellular liquids, topology and density of the ambient tissue or extracellular matrix scaffold (Charras and Sahai, 2014). To address these physical constraints of a 3D microenvironment, individual cells quickly change their viscoelasticity to recontour and “squeeze” or withstand deformation (Petrie and Yamada, 2012; Mueller et al., 2017). Nonetheless, to modify their viscoelastic characteristics and face their physical migratory microenvironment as a supracellular entity, cells within migratory aggregates must orchestrate the machinery that accomplishes such transformations.

Apart from the cell migratory aspect, the fine-tuning of the tumor extracellular matrix seems to be necessary to mimic the process of matrix alteration during cancer disease progression. In particular, the viscoelastic characteristics of hydrogels can be tailored in the course of time by agents that influence the forming or breaking down of cross-links. In addition, enzymatic crosslinkers use the reaction kinetics of the enzymes to change the viscoelastic characteristics of the gel with time (Mattei et al., 2020; Cacopardo and Ahluwalia, 2021). Slow kinetics chemical reactions have also been applied to produce gels with time-varying viscoelasticity (Guvendiren and Burdick, 2012; Rodell et al., 2015; Arkenberg et al., 2019; Carberry et al., 2020). Stem cell engagement switch that can be leveraged to stiffen hydrogels (Das et al., 2016). Consequently, the use of reactive materials is an interesting tactic to modify the viscoelasticity of gels as needed (Abdeen et al., 2016; Chen et al., 2021; Tran et al., 2021). For this purpose, these reactive materials have been shown to affect, for instance, the adhesion and spreading behavior of mesenchymal stem cells on magneto-responsive gels when the gel transitions from an elastic to a liquid-like characteristic (Abdeen et al., 2016). Most promising appear time evolving hydrogels. However, there is still too little research activity there. Therefore, further endeavors in this pursuit are required to develop mechano-mimetic approaches capable of replicating pathophysiological processes in vitro.

Effect of Time Scales on Extracellular Matrix Scaffold’s Viscoelasticity

Special attention will be paid to the behavioral links between the various time scales concerned, such as mechanical, cellular, and observational, and to the principles of scaling that must be taken into account when developing viscoelastic materials and conducting tests for biomechanical or mechanobiological engineering purposes. The observational time scale is often not addressed and not included here. Time scale analysis identified that extracellular matrix viscoelasticity controls cell spreading, on the basis of the extent of the substrate relaxation time scale in comparison to the time scales of motor coupling binding and the focal adhesion lifespan. The coupling (clutch) binding time, τ_b, is entirely due to the stochastic binding signature of the focal adhesion molecules, such as integrins, talin, paxillin and vinculin, with value of around 1 s (Chan and Odde, 2008; Bangasser and Odde, 2013). The interplay of myosin motor traction and substrate rigidity results in a focal adhesion lifetime timescale, τ_l, ranging from 10^–1 s to 10³ s. When no strengthening is acting, the focal adhesion lifetime is linear proportional to its lifetime time scale, τ_l (Bangasser and Odde, 2013). Average lifetimes vary from approximately 10 s–100 s, consistent with nascent focal adhesions within the lamellipodium, relying on stiffness (Choi et al., 2008). But once the enhancement is in effect, the focal adhesion lifetime could be in minutes or possibly beyond (a divergent condition), which is in agreement with a large number of experiments demonstrating robust focal adhesions for stiffer carriers (Walcott et al., 2011; Trichet et al., 2012; Cao et al., 2015). The substrate relaxation time scale, τ_s, would encompass a broad spectrum from 10^–2 s to 10² s, which matches the viscoelastic character of diverse substrates. The behavioral association between three timescales, such as τ_l, τ_b, and τ_s, distinctly accounts for how viscosity governs cell spreading. In particular, when τ_l > τ_b, the spreading of cells is largest when the relaxation timescale τ_s lies between the binding timescale τ_b and the lifetime timescale τ_l (τ_b <τ_s <τ_l) (Figure 5). However, when τ_l < τ_b, the coupling reinforcement occurs and causes a saturation of the cell spreading area. Under this circumstance, the viscosity is not altering the spreading behavior of cells. The relaxation timescales, which are shorter than the coupling (binding) timescale (τ_s < τ_b) exhibit a negligible impact on the spreading of cells.

Convenient way to identify the effective relaxation time scale of viscoelastic substrate in terms of its impact on cell spreading has been elaborated. Therefore, firstly, the relaxation time spectra need to be captured from stress relaxation data of viscoelastic materials. Secondly, the most prominent timescale, such as the highest peak for τ_s ≥ τ_b, has been figured out of the relaxation spectrum as the so-called effective timescale. Computer simulations using multiple timescales revealed that the effective timescale perfectly mirrors the dynamical nature of the process of cell spreading on viscoelastic substrates. However, in instances where there are multiple prominent relaxation times past the binding time scale, the simulations indicate that the final outcome cell spreading is roughly a weighted average of the response for every time scale.

Earlier investigations proposed that the impact of substrate viscoelasticity on cell spreading is related to local substrate compaction and plastic flow (Chaudhuri et al., 2015; Bauer et al., 2017). Nevertheless, these experimental findings can be replicated by the newly proposed model (Chaudhuri et al., 2020), demonstrating that viscoelasticity by itself is fully adequate to account for the results obtained. Importantly, it is worth noting that this model is able to adequately clarify the viscoelastic control of cell spreading for three completely distinct types of hydrogels, including alginate, hyaluronic acid (HA) and polyacrylamide, with various manners of imparting viscoelasticity, such as supramolecular interactions, semi-interpenetrating entanglements of the scaffold and ionic-based cross-linking, distinct stiffnesses in the spectrum from 10^–1 pN/nm to 10¹ pN/nm, and various cell types, encompassing human MSCs, 3T3 fibroblasts and the U2OS osteosarcoma cell line. In addition, it is also possible to use this model for various cancer cell types. Moreover, experiments with substrates produced in various manners and displaying virtually no plasticity are also covered by the model predictions. These findings show that viscoelasticity by itself possesses a strong impact on cell spreading, plasticity and extracellular matrix restructuring that all seem to be equally critical. A more sophisticated model that encompasses the plastic theory may be addressed for future applications.

In summary, an analytical model incorporating the viscoelastic relaxation time range has successfully elucidated the implications of substrate viscoelasticity on cell spreading of various cell types and collagen matrices (Gautieri et al., 2013; Nam et al., 2016). This model can be employed as a theoretical framework for continued study of viscoelastic control of cell performance for multiple cancer cell types and tissues. It is also able to aid in forecasting cell spreading over the entire parameter range for viscoelastic substrates to be used in cancer research, thereby permitting the streamlined design of biomaterials. Finally, this result offers both physical glimpses and a practical approach to examine how cellular and material time scales intersect to adjust cancer cell performance.

Separation of Mechanical Characteristics From Other Matrix Parameters

During the design of tumor microenvironments for mechanobiological investigations, it is essential to ensure that cells have appropriate topographical and biochemical cues in addition to mechanical constraints, and, especially in 3D, to ensure adequate room for cell growth. Yet, there has been limited emphasis on accounting for these interacting elements, potentially causing a false interpretation of the findings. Typical investigations involve benchmarking the performance of cells on 2D plastic substrates versus cells on 2D or 3D gels. Not merely the mechanical characteristics are distinct, but also features such as surface roughness, surface chemistry, and haptotactic information are to be distinguished (Alenghat and Ingber, 2002; Bettinger et al., 2009; Trappmann and Chen, 2013; Woods et al., 2017). For example, a tension platform for the analysis of the interplay between cancer cell phenotype and tumor extracellular matrix stiffness has been developed (Cassereau et al., 2015). Notably, the precise mechanical tailoring of the stiffness of collagen hydrogels while retaining a constant composition and porosity.

Numerous efforts are devoted to the decoupling of stiffness and ligand density (A. Engler et al., 2004; Grevesse et al., 2013; S. J. Han et al., 2012), whereas fewer efforts are directed to stiffness and topography (Ahmed et al., 2021) or mineral grade (Mattei et al., 2020). The problem of decoupling interacting effects in mechotransduction is amplified in 3D gels because raising the polymer concentration and crosslinking not only modifies the mechanical response of the gels, but also impacts oxygen and nutrient diffusion as well as cell volume (García, 2014; Xue et al., 2021). Consequently, alterations in cell responsiveness can be affected or sometimes even obscured by issues other than the mechanical characteristics of the cell surrounding environment (Vandrangi et al., 2014). Currently, a particular difficulty in vitro research is to isolate or decouple mechanical characteristics from other quantities of parameters to completely comprehend and control cell performance by tailoring environmental factors that can be engineered and monitored.

Final Remarks and Future Perspectives

How the tumor extracellular matrix shields, protects and nourish the primary tumor is important to fully understand the progression of cancer and the failure of cancer therapies in specific cancer types or distinct patients. A reason for this may be the diverse extracellular matrices of tumors. Multiple time, it has been shown that the extracellular matrix in tumors is pronouncedly altered in composition and structure compared to normal healthy tissue. As for their physical characteristics, the extracellular matrix of the tumor is richer, denser and stiffer. These modified characteristics may adversely impact the responsiveness to therapy in several respects. Apparently, exaggerated clustering of dense and rigid extracellular matrix, which histologically frequently envelops clusters of cancer cells, is able to function as a border barrier, insulating the cells from therapeutic compounds.

This specific behavior is directly related to a decreased overall perfusion, as this barrier also impedes the diffusion of oxygen, nutrients, and metabolites. Therefore, the tumor microenvironment limits the trafficking of oxygen and other nutrients that subsequently leads to immunosuppression in the primary tumor and later in the whole organism. A major reason for this is that the poorly functioning blood vessel of tumors are leaky and compressed, and some endothelial cells are replaced by cancer cells, which take over the role of endothelial cells. All of this must be reversed by potentially altering the mechanical factors in the tumor extracellular matrix to improve oxygenation of primary tumors. Therefore, immunotherapies should be combined with therapies that normalize the tumor microenvironment to synergistically augment oxygen transport and treatment outcomes. Elevated hypoxia and metabolic stress cause activation of anti-apoptotic and drug-resistant signaling cascades. Consequently, cell-extracellular matrix contacts and augmented tissue stiffness may participate in direct support of tumor chemoresistance through integrin and FAK signal transduction pathways. For the progress in the development of viscoelastic tumor microenvironment mimicking hydrogels, the time-evolving viscoelasticity needs to be addressed. Time-evolving viscoelasticity, which means how viscoelasticity alters during various stages of cancer, is a key aspect in mimicking the tumor microenvironment (Mattei et al., 2020).

Viscoelasticity of the migratory microenvironment is crucial to induce cell migration, engineer a material that enables the efficient migration of cells, and/or to regulate migration through the mechano-sensing-based process of durotaxis. Consistent with this, new advances have been explored that highlight possible mechanisms facilitating the transfer of mechanical cues from the tumor extracellular matrix environment into cells, their impact on the expression of traditional transcriptional controllers of epithelial-to-mesenchymal transition (EMT) of individual cancer cells, and their implications for altering the viscoelastic phenotype of migrating cells and their local tumor microenvironment. In this regard, the time-evolving viscoelastic alterations of hydrogels seem to be most promising and need future research effort.

Tissue interferences occur, including that the EMT, and offer the integration of morphogenesis as a mechano-molecular feedback circuit that coordinates the timing of cellular redistributions and gene expression patterns that are needed for cancer progression. Moreover, it has to be accounted for that molecular signal transduction causes cellular remodeling events, which alter the tissue including its viscoelastic characteristics, and this new viscoelasticity of the tissue environment can then act on a long-range timescale to alter the cellular, molecular, and viscoelastic characteristics of a neighbor tissue. This behavior is similar to a mechano-molecular feedback circuit that governs the process of morphogenesis. Since tissue interferences during morphogenesis are seen at a chemical scale through the secretion of molecules, it would be promising to investigate the interaction of viscoelasticity and secreted molecules in the regulation of collective movement of cancer cells, as an element of this integrative approach in cancer research. The involvement of these types of mechano-molecular feedback interferences seem to be highly crucial in the advancement for the forming and engineering of organ-analogous structures, such as organoids, for investigating the malignant progression of cancer and its mutual interplay with the tumor extracellular matrix scaffold. Thus, it is even more important to combine the analysis of local tumor microenvironment with molecular elements, viscoelastic variables of cells and gene expression patterns (Poh et al., 2014; Wrighton and Kiessling, 2015; Mierke, 2019).

Moreover, if it is known how these feedback loops act on the progression of cancer, the knowledge could be used to perform effective cancer therapies. Employing in vivo rat cancer metastasis models, the mechanical stress generated through gastric cancers toward their microenvironment has been seen to cause severe molecular impacts that are associated with a poor prognosis (Fernández-Sánchez et al., 2015). Identification of viscoelastic characteristics of wounds and inflammatory tissues together with the knowledge how these alterations impact the collective migration and cellular fate, may be beneficial in developing new therapies targeting these kinds of processes during the progression of cancer. Consequently, viscoelasticity represents a general characteristic feature for the vast majority of biological substances and the majority of cells and tissues that experience one or even another mechanical force. Lastly, the requirement of multidisciplinary studies combining biophysical and biochemical variables seem to be critical to obtain a knowledge of growing intricate living biological systems under diseased conditions, such as cancer.

Finally, it is important not only to address the stiffness of the tumor extracellular matrix network, but also to explore the viscoelastic characteristics of these networks that then act on the other mechanical characteristics, such as the aforementioned stiffness.

Author Contributions

The author confirms being the sole contributor of this work and has approved it for publication.

Conflict of Interest

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

The author acknowledges support from the German Research Foundation (DFG) and Universität Leipzig within the program of Open Access Publishing, and Thomas M.L. Mierke for critical proof reading of the manuscript.

References

Abdeen, A. A., Lee, J., Bharadwaj, N. A., Ewoldt, R. H., and Kilian, K. A. (2016). Temporal Modulation of Stem Cell Activity Using Magnetoactive Hydrogels. Adv. Healthc. Mater. 5 (19), 2536–2544. doi:10.1002/adhm.201600349

PubMed Abstract | CrossRef Full Text | Google Scholar

Abidine, Y., Giannetti, A., Revilloud, J., Laurent, V. M., and Verdier, C. (2021). Viscoelastic Properties in Cancer: From Cells to Spheroids. Cells 10 (7), 1704. doi:10.3390/cells10071704

PubMed Abstract | CrossRef Full Text | Google Scholar

Addae-Mensah, K. A., and Wikswo, J. P. (2008). Measurement Techniques for Cellular Biomechanics In Vitro. Exp. Biol. Med. (Maywood) 233 (7), 792–809. doi:10.3181/0710-MR-278

PubMed Abstract | CrossRef Full Text | Google Scholar

Adebowale, K., Gong, Z., Hou, J. C., Wisdom, K. M., Garbett, D., Lee, H.-p., et al. (2021). Enhanced Substrate Stress Relaxation Promotes Filopodia-Mediated Cell Migration. Nat. Mater. 20 (9), 1290–1299. doi:10.1038/s41563-021-00981-w

PubMed Abstract | CrossRef Full Text | Google Scholar

Agyapong-Badu, S., Warner, M. B., Samuel, D., Koutra, V., and Stokes, M. (2021). Non-Invasive Biomarkers of Musculoskeletal Health with High Discriminant Ability for Age and Gender. Jcm 10 (7), 1352. doi:10.3390/jcm10071352

PubMed Abstract | CrossRef Full Text | Google Scholar

Ahmed, A., Joshi, I. M., Mansouri, M., Ahamed, N. N. N., Hsu, M.-C., Gaborski, T. R., et al. (2021). Engineering Fiber Anisotropy within Natural Collagen Hydrogels. Am. J. Physiology-Cell Physiol. 320 (6), C1112–C1124. doi:10.1152/ajpcell.00036.2021

CrossRef Full Text | Google Scholar

Alcaraz, J., Buscemi, L., Grabulosa, M., Trepat, X., Fabry, B., Farré, R., et al. (2003). Microrheology of Human Lung Epithelial Cells Measured by Atomic Force Microscopy. Biophysical J. 84 (3), 2071–2079. doi:10.1016/S0006-3495(03)75014-0

CrossRef Full Text | Google Scholar

Alcaraz, J., Buscemi, L., Puig-de-Morales, M., Colchero, J., Baró, A., and Navajas, D. (2002). Correction of Microrheological Measurements of Soft Samples with Atomic Force Microscopy for the Hydrodynamic Drag on the Cantilever. Langmuir 18 (3), 716–721. doi:10.1021/la0110850

CrossRef Full Text | Google Scholar

Alenghat, F. J., and Ingber, D. E. (2002). Mechanotransduction: All Signals Point to Cytoskeleton, Matrix, and Integrins. Sci. STKE 2002 (119), 2002. doi:10.1126/stke.2002.119.pe6

PubMed Abstract | CrossRef Full Text | Google Scholar

Alibert, C., Pereira, D., Lardier, N., Etienne-Manneville, S., Goud, B., Asnacios, A., et al. (2021). Multiscale Rheology of Glioma Cells. Biomaterials 275, 120903. doi:10.1016/j.biomaterials.2021.120903

PubMed Abstract | CrossRef Full Text | Google Scholar

Alt, S., Ganguly, P., and Salbreux, G. (2017). Vertex Models: From Cell Mechanics to Tissue Morphogenesis. Phil. Trans. R. Soc. B 372 (1720), 20150520. doi:10.1098/rstb.2015.0520

PubMed Abstract | CrossRef Full Text | Google Scholar

Alvarado, J., Sheinman, M., Sharma, A., MacKintosh, F. C., and Koenderink, G. H. (2017). Force Percolation of Contractile Active Gels. Soft Matter 13 (34), 5624–5644. doi:10.1039/C7SM00834A

PubMed Abstract | CrossRef Full Text | Google Scholar

Angelini, T. E., Hannezo, E., Trepat, X., Marquez, M., Fredberg, J. J., and Weitz, D. A. (2011). Glass-like Dynamics of Collective Cell Migration. Proc. Natl. Acad. Sci. 108 (12), 4714–4719. doi:10.1073/pnas.1010059108

PubMed Abstract | CrossRef Full Text | Google Scholar

Arkenberg, M. R., Moore, D. M., and Lin, C.-C. (2019). Dynamic Control of Hydrogel Crosslinking via Sortase-Mediated Reversible Transpeptidation. Acta Biomater. 83, 83–95. doi:10.1016/j.actbio.2018.11.011

PubMed Abstract | CrossRef Full Text | Google Scholar

Backholm, M., Ryu, W. S., and Dalnoki-Veress, K. (2013). Viscoelastic Properties of the Nematode Caenorhabditis elegans, a Self-Similar, Shear-Thinning Worm. Proc. Natl. Acad. Sci. 110 (12), 4528–4533. doi:10.1073/pnas.1219965110

PubMed Abstract | CrossRef Full Text | Google Scholar

Bai, Y., Jiang, Y., Chen, B., Chiang Foo, C., Zhou, Y., Xiang, F., et al. (2014). Cyclic Performance of Viscoelastic Dielectric Elastomers with Solid Hydrogel Electrodes. Appl. Phys. Lett. 104 (6), 062902. doi:10.1063/1.4865200

CrossRef Full Text | Google Scholar

Balbi, V., Shearer, T., and Parnell, W. J. (2018). A Modified Formulation of Quasi-Linear Viscoelasticity for Transversely Isotropic Materials under Finite Deformation. Proc. R. Soc. A. 474 (2217), 20180231. doi:10.1098/rspa.2018.0231

PubMed Abstract | CrossRef Full Text | Google Scholar

Bangasser, B. L., and Odde, D. J. (2013). Master Equation-Based Analysis of a Motor-Clutch Model for Cell Traction Force. Cel. Mol. Bioeng. 6 (4), 449–459. doi:10.1007/s12195-013-0296-5

PubMed Abstract | CrossRef Full Text | Google Scholar

Bangasser, B. L., Rosenfeld, S. S., and Odde, D. J. (2013). Determinants of Maximal Force Transmission in a Motor-Clutch Model of Cell Traction in a Compliant Microenvironment. Biophysical J. 105 (3), 581–592. doi:10.1016/j.bpj.2013.06.027

CrossRef Full Text | Google Scholar

Banks, H. T., Hu, S., and Kenz, Z. R. (2011). A Brief Review of Elasticity and Viscoelasticity for Solids. Adv. Appl. Math. Mech. 3 (1), 1–51. doi:10.4208/aamm.10-m1030

CrossRef Full Text | Google Scholar

Bao, G., and Suresh, S. (2003). Cell and Molecular Mechanics of Biological Materials. Nat. Mater 2 (11), 715–725. doi:10.1038/nmat1001

PubMed Abstract | CrossRef Full Text | Google Scholar

Barriga, E. H., Franze, K., Charras, G., and Mayor, R. (2018). Tissue Stiffening Coordinates Morphogenesis by Triggering Collective Cell Migration In Vivo. Nature 554 (7693), 523–527. doi:10.1038/nature25742

PubMed Abstract | CrossRef Full Text | Google Scholar

Bauer, A., Gu, L., Kwee, B., Li, W. A., Dellacherie, M., Celiz, A. D., et al. (2017). Hydrogel Substrate Stress-Relaxation Regulates the Spreading and Proliferation of Mouse Myoblasts. Acta Biomater. 62, 82–90. doi:10.1016/j.actbio.2017.08.041

PubMed Abstract | CrossRef Full Text | Google Scholar

Benjamin, J. M., Kwiatkowski, A. V., Yang, C., Korobova, F., Pokutta, S., Svitkina, T., et al. (2010). Αe-Catenin Regulates Actin Dynamics Independently of Cadherin-Mediated Cell-Cell Adhesion. J. Cell Biol. 189 (2), 339–352. doi:10.1083/jcb.200910041

CrossRef Full Text | Google Scholar

Bettinger, C. J., Langer, R., and Borenstein, J. T. (2009). Engineering Substrate Topography at the Micro- and Nanoscale to Control Cell Function. Angew. Chem. Int. Ed. 48 (30), 5406–5415. doi:10.1002/anie.200805179

CrossRef Full Text | Google Scholar

Bi, D., Lopez, J. H., Schwarz, J. M., and Manning, M. L. (2014). Energy Barriers and Cell Migration in Densely Packed Tissues. Soft Matter 10 (12), 1885. doi:10.1039/c3sm52893f

PubMed Abstract | CrossRef Full Text | Google Scholar

Bi, D., Yang, X., Marchetti, M. C., and Manning, M. L. (2016). Motility-Driven Glass and Jamming Transitions in Biological Tissues. Phys. Rev. X 6 (2), 021011. doi:10.1103/PhysRevX.6.021011

PubMed Abstract | CrossRef Full Text | Google Scholar

Bissell, M. J., Hall, H. G., and Parry, G. (1982). How Does the Extracellular Matrix Direct Gene Expression? J. Theor. Biol. 99 (1), 31–68. doi:10.1016/0022-5193(82)90388-5

CrossRef Full Text | Google Scholar

Boyle, S. T., and Samuel, M. S. (2016). Mechano-reciprocity Is Maintained between Physiological Boundaries by Tuning Signal Flux through the Rho-Associated Protein Kinase. Small GTPases 7 (3), 139–146. doi:10.1080/21541248.2016.1173771

PubMed Abstract | CrossRef Full Text | Google Scholar

Brazel, C. S., Rosen, S. L., and Rosen, S. L. (2012). Fundamental Principles of Polymeric Materials. Third edition. Wiley.

Google Scholar

Broedersz, C. P., and MacKintosh, F. C. (2014). Modeling Semiflexible Polymer Networks. Rev. Mod. Phys. 86 (3), 995–1036. doi:10.1103/RevModPhys.86.995

CrossRef Full Text | Google Scholar

Brown, T. E., Carberry, B. J., Worrell, B. T., Dudaryeva, O. Y., McBride, M. K., Bowman, C. N., et al. (2018). Photopolymerized Dynamic Hydrogels with Tunable Viscoelastic Properties through Thioester Exchange. Biomaterials 178, 496–503. doi:10.1016/j.biomaterials.2018.03.060

PubMed Abstract | CrossRef Full Text | Google Scholar

Buchmann, B., Engelbrecht, L. K., Fernandez, P., Hutterer, F. P., Raich, M. K., Scheel, C. H., et al. (2021). Mechanical Plasticity of Collagen Directs branch Elongation in Human Mammary Gland Organoids. Nat. Commun. 12 (1), 2759. doi:10.1038/s41467-021-22988-2

PubMed Abstract | CrossRef Full Text | Google Scholar

Caccavo, D., Cascone, S., Lamberti, G., and Barba, A. A. (2018). Hydrogels: Experimental Characterization and Mathematical Modelling of Their Mechanical and Diffusive Behaviour. Chem. Soc. Rev. 47 (7), 2357–2373. doi:10.1039/C7CS00638A

PubMed Abstract | CrossRef Full Text | Google Scholar

Cacopardo, L., and Ahluwalia, A. (2021). Engineering and Monitoring 3D Cell Constructs with Time-Evolving Viscoelasticity for the Study of Liver Fibrosis In Vitro. Bioengineering 8 (8), 106. doi:10.3390/bioengineering8080106

PubMed Abstract | CrossRef Full Text | Google Scholar

Cameron, A. R., Frith, J. E., and Cooper-White, J. J. (2011). The Influence of Substrate Creep on Mesenchymal Stem Cell Behaviour and Phenotype. Biomaterials 32 (26), 5979–5993. doi:10.1016/j.biomaterials.2011.04.003

PubMed Abstract | CrossRef Full Text | Google Scholar

Camley, B. A., Zhang, Y., Zhao, Y., Li, B., Ben-Jacob, E., Levine, H., et al. (2014). Polarity Mechanisms Such as Contact Inhibition of Locomotion Regulate Persistent Rotational Motion of Mammalian Cells on Micropatterns. Proc. Natl. Acad. Sci. 111 (41), 14770–14775. doi:10.1073/pnas.1414498111

PubMed Abstract | CrossRef Full Text | Google Scholar

Cao, X., Lin, Y., Driscoll, T. P., Franco-Barraza, J., Cukierman, E., Mauck, R. L., et al. (2015). A Chemomechanical Model of Matrix and Nuclear Rigidity Regulation of Focal Adhesion Size. Biophysical J. 109 (9), 1807–1817. doi:10.1016/j.bpj.2015.08.048

PubMed Abstract | CrossRef Full Text | Google Scholar

Carberry, B. J., Rao, V. V., and Anseth, K. S. (2020). Phototunable Viscoelasticity in Hydrogels through Thioester Exchange. Ann. Biomed. Eng. 48 (7), 2053–2063. doi:10.1007/s10439-020-02460-w

PubMed Abstract | CrossRef Full Text | Google Scholar

Case, L. B., and Waterman, C. M. (2015). Integration of Actin Dynamics and Cell Adhesion by a Three-Dimensional, Mechanosensitive Molecular Clutch. Nat. Cell Biol 17 (8), 955–963. doi:10.1038/ncb3191

PubMed Abstract | CrossRef Full Text | Google Scholar

Cassereau, L., Miroshnikova, Y. A., Ou, G., Lakins, J., and Weaver, V. M. (2015). A 3D Tension Bioreactor Platform to Study the Interplay between ECM Stiffness and Tumor Phenotype. J. Biotechnol. 193, 66–69. doi:10.1016/j.jbiotec.2014.11.008

CrossRef Full Text | Google Scholar

Chan, C. E., and Odde, D. J. (2008). Traction Dynamics of Filopodia on Compliant Substrates. Science 322 (5908), 1687–1691. doi:10.1126/science.1163595

PubMed Abstract | CrossRef Full Text | Google Scholar

Charbonier, F., Indana, D., and Chaudhuri, O. (2021). Tuning Viscoelasticity in Alginate Hydrogels for 3D Cell Culture Studies. Curr. Protoc. 1 (5). doi:10.1002/cpz1.124

CrossRef Full Text | Google Scholar

Charras, G., and Sahai, E. (2014). Physical Influences of the Extracellular Environment on Cell Migration. Nat. Rev. Mol. Cell Biol 15 (12), 813–824. doi:10.1038/nrm3897

PubMed Abstract | CrossRef Full Text | Google Scholar

Chaudhuri, O., Cooper-White, J., Janmey, P. A., Mooney, D. J., and Shenoy, V. B. (2020). Effects of Extracellular Matrix Viscoelasticity on Cellular Behaviour. Nature 584 (7822), 535–546. doi:10.1038/s41586-020-2612-2

PubMed Abstract | CrossRef Full Text | Google Scholar

Chaudhuri, O., Gu, L., Darnell, M., Klumpers, D., Bencherif, S. A., Weaver, J. C., et al. (2015). Substrate Stress Relaxation Regulates Cell Spreading. Nat. Commun. 6 (1), 6365. doi:10.1038/ncomms7365

CrossRef Full Text | Google Scholar

Chaudhuri, O., Gu, L., Klumpers, D., Darnell, M., Bencherif, S. A., Weaver, J. C., et al. (2016). Hydrogels with Tunable Stress Relaxation Regulate Stem Cell Fate and Activity. Nat. Mater 15 (3), 326–334. doi:10.1038/nmat4489

PubMed Abstract | CrossRef Full Text | Google Scholar

Chaudhuri, O. (2017). Viscoelastic Hydrogels for 3D Cell Culture. Biomater. Sci. 5 (8), 1480–1490. doi:10.1039/C7BM00261K

PubMed Abstract | CrossRef Full Text | Google Scholar

Chen, W., Zhang, Y., Kumari, J., Engelkamp, H., and Kouwer, P. H. J. (2021). Magnetic Stiffening in 3D Cell Culture Matrices. Nano Lett. 21 (16), 6740–6747. doi:10.1021/acs.nanolett.1c00371

PubMed Abstract | CrossRef Full Text | Google Scholar

Chim, Y. H., Mason, L. M., Rath, N., Olson, M. F., Tassieri, M., and Yin, H. (2018). A One-step Procedure to Probe the Viscoelastic Properties of Cells by Atomic Force Microscopy. Sci. Rep. 8 (1), 14462. doi:10.1038/s41598-018-32704-8

PubMed Abstract | CrossRef Full Text | Google Scholar

Choi, C. K., Vicente-Manzanares, M., Zareno, J., Whitmore, L. A., Mogilner, A., and Horwitz, A. R. (2008). Actin and α-actinin Orchestrate the Assembly and Maturation of Nascent Adhesions in a Myosin II Motor-independent Manner. Nat. Cell Biol 10 (9), 1039–1050. doi:10.1038/ncb1763

PubMed Abstract | CrossRef Full Text | Google Scholar

Christensen, R. M. (1980). A Nonlinear Theory of Viscoelasticity for Application to Elastomers. J. Appl. Mech. 47 (4), 762–768. doi:10.1115/1.3153787

CrossRef Full Text | Google Scholar

Chung, C. W., and Buist, M. L. (2012). A Novel Nonlinear Viscoelastic Solid Model. Nonlinear Anal. Real World Appl. 13 (3), 1480–1488. doi:10.1016/j.nonrwa.2011.11.011

CrossRef Full Text | Google Scholar

Chyasnavichyus, M., Young, S. L., and Tsukruk, V. V. (2014). Probing of Polymer Surfaces in the Viscoelastic Regime. Langmuir 30 (35), 10566–10582. doi:10.1021/la404925h

PubMed Abstract | CrossRef Full Text | Google Scholar

Cohen, D. J., James Nelson, W., and Maharbiz, M. M. (2014). Galvanotactic Control of Collective Cell Migration in Epithelial Monolayers. Nat. Mater 13 (4), 409–417. doi:10.1038/nmat3891

PubMed Abstract | CrossRef Full Text | Google Scholar

Cox, T. R. (2021). The Matrix in Cancer. Nat. Rev. Cancer 21 (4), 217–238. doi:10.1038/s41568-020-00329-7

PubMed Abstract | CrossRef Full Text | Google Scholar

Cross, S. E., Jin, Y.-S., Tondre, J., Wong, R., Rao, J., and Gimzewski, J. K. (2008). AFM-based Analysis of Human Metastatic Cancer Cells. Nanotechnology 19 (38), 384003. doi:10.1088/0957-4484/19/38/384003

PubMed Abstract | CrossRef Full Text | Google Scholar

Cukierman, E., Pankov, R., Stevens, D. R., and Yamada, K. M. (2001). Taking Cell-Matrix Adhesions to the Third Dimension. Science 294 (5547), 1708–1712. doi:10.1126/science.1064829

PubMed Abstract | CrossRef Full Text | Google Scholar

Darling, E. M., Zauscher, S., Block, J. A., and Guilak, F. (2007). A Thin-Layer Model for Viscoelastic, Stress-Relaxation Testing of Cells Using Atomic Force Microscopy: Do Cell Properties Reflect Metastatic Potential? Biophysical J. 92 (5), 1784–1791. doi:10.1529/biophysj.106.083097

CrossRef Full Text | Google Scholar

Das, R. K., Gocheva, V., Hammink, R., Zouani, O. F., and Rowan, A. E. (2016). Stress-stiffening-mediated Stem-Cell Commitment Switch in Soft Responsive Hydrogels. Nat. Mater. 15 (3), 318–325. doi:10.1038/nmat4483

PubMed Abstract | CrossRef Full Text | Google Scholar

de Gennes, P. G. (1979). Brownian Motions of Flexible Polymer Chains. Nature 282 (5737), 367–370. doi:10.1038/282367a0

CrossRef Full Text | Google Scholar

de Gennes, P. G. (1971). Reptation of a Polymer Chain in the Presence of Fixed Obstacles. J. Chem. Phys. 55 (2), 572–579. doi:10.1063/1.1675789

CrossRef Full Text | Google Scholar

De, P. S., and De, R. (2019). Stick-slip Dynamics of Migrating Cells on Viscoelastic Substrates. Phys. Rev. E 100 (1), 012409. doi:10.1103/PhysRevE.100.012409

PubMed Abstract | CrossRef Full Text | Google Scholar

De, R., and Safran, S. A. (2008). Dynamical Theory of Active Cellular Response to External Stress. Phys. Rev. E 78 (3), 031923. doi:10.1103/PhysRevE.78.031923

PubMed Abstract | CrossRef Full Text | Google Scholar

De, R., Zemel, A., and Safran, S. A. (2007). Dynamics of Cell Orientation. Nat. Phys. 3 (9), 655–659. doi:10.1038/nphys680

CrossRef Full Text | Google Scholar

Deng, L., Trepat, X., Butler, J. P., Millet, E., Morgan, K. G., Weitz, D. A., et al. (2006). Fast and Slow Dynamics of the Cytoskeleton. Nat. Mater. 5 (8), 636–640. doi:10.1038/nmat1685

PubMed Abstract | CrossRef Full Text | Google Scholar

Dhar, M., Lam, J. N., Walser, T., Dubinett, S. M., Rettig, M. B., and Di Carlo, D. (2018). Functional Profiling of Circulating Tumor Cells with an Integrated Vortex Capture and Single-Cell Protease Activity Assay. Proc. Natl. Acad. Sci. 115 (40), 9986–9991. doi:10.1073/pnas.1803884115

PubMed Abstract | CrossRef Full Text | Google Scholar

Dickinson, R. B., Guido, S., and Tranquillo, R. T. (1994). Biased Cell Migration of Fibroblasts Exhibiting Contact Guidance in Oriented Collagen Gels. Ann. Biomed. Eng. 22 (4), 342–356. doi:10.1007/BF02368241

PubMed Abstract | CrossRef Full Text | Google Scholar

Discher, D. E. (2005). Tissue Cells Feel and Respond to the Stiffness of Their Substrate. Science 310 (5751), 1139–1143. doi:10.1126/science.1116995

PubMed Abstract | CrossRef Full Text | Google Scholar

Dooling, L. J., Buck, M. E., Zhang, W.-B., and Tirrell, D. A. (2016). Programming Molecular Association and Viscoelastic Behavior in Protein Networks. Adv. Mater. 28 (23), 4651–4657. doi:10.1002/adma.201506216

PubMed Abstract | CrossRef Full Text | Google Scholar

Douezan, S., Guevorkian, K., Naouar, R., Dufour, S., Cuvelier, D., and Brochard-Wyart, F. (2011). Spreading Dynamics and Wetting Transition of Cellular Aggregates. Proc. Natl. Acad. Sci. 108 (18), 7315–7320. doi:10.1073/pnas.1018057108

PubMed Abstract | CrossRef Full Text | Google Scholar

Doxzen, K., Vedula, S. R. K., Leong, M. C., Hirata, H., Gov, N. S., Kabla, A. J., et al. (2013). Guidance of Collective Cell Migration by Substrate Geometry. Integr. Biol. 5 (8), 1026. doi:10.1039/c3ib40054a

CrossRef Full Text | Google Scholar

Driscoll, M. M., Chen, B. G., Beuman, T. H., Ulrich, S., Nagel, S. R., and Vitelli, V. (2016). The Role of Rigidity in Controlling Material Failure. Proc. Natl. Acad. Sci. 113 (39), 10813–10817. doi:10.1073/pnas.1501169113

PubMed Abstract | CrossRef Full Text | Google Scholar

Eastwood, A. L., Sanzeni, A., Petzold, B. C., Park, S.-J., Vergassola, M., Pruitt, B. L., et al. (2015). Tissue Mechanics Govern the Rapidly Adapting and Symmetrical Response to Touch. Proc. Natl. Acad. Sci. 112 (50), E6955–E6963. doi:10.1073/pnas.1514138112

PubMed Abstract | CrossRef Full Text | Google Scholar

Eckes, B., Colucci-Guyon, E., Smola, H., Nodder, S., Babinet, C., Krieg, T., et al. (2000). Impaired Wound Healing in Embryonic and Adult Mice Lacking Vimentin. J. Cell Sci. 113 (Pt 13), 2455–2462. doi:10.1242/jcs.113.13.2455

CrossRef Full Text | Google Scholar

Efremov, Y. M., Okajima, T., and Raman, A. (2020). Measuring Viscoelasticity of Soft Biological Samples Using Atomic Force Microscopy. Soft Matter 16 (1), 64–81. doi:10.1039/C9SM01020C

PubMed Abstract | CrossRef Full Text | Google Scholar

Efremov, Yu. M., Lomakina, M. E., Bagrov, D. V., Makhnovskiy, P. I., Alexandrova, A. Y., Kirpichnikov, M. P., et al. (2014). Mechanical Properties of Fibroblasts Depend on Level of Cancer Transformation. Biochim. Biophys. Acta (Bba) - Mol. Cell Res. 1843 (5), 1013–1019. doi:10.1016/j.bbamcr.2014.01.032

CrossRef Full Text | Google Scholar

Einstein, A. (1905). Zur Elektrodynamik Bewegter Körper. Annalen der Physik 322 (10), 891–921. doi:10.1002/andp.19053221004

CrossRef Full Text | Google Scholar

Elkin, B. S., Ilankovan, A. I., and Morrison, B. (2011). A Detailed Viscoelastic Characterization of the P17 and Adult Rat Brain. J. Neurotrauma 28 (11), 2235–2244. doi:10.1089/neu.2010.1604

CrossRef Full Text | Google Scholar

Elosegui-Artola, A., Bazellières, E., Allen, M. D., Andreu, I., Oria, R., Sunyer, R., et al. (2014). Rigidity Sensing and Adaptation through Regulation of Integrin Types. Nat. Mater. 13 (6), 631–637. doi:10.1038/nmat3960

PubMed Abstract | CrossRef Full Text | Google Scholar

Elosegui-Artola, A., Oria, R., Chen, Y., Kosmalska, A., Pérez-González, C., Castro, N., et al. (2016). Mechanical Regulation of a Molecular Clutch Defines Force Transmission and Transduction in Response to Matrix Rigidity. Nat. Cell Biol. 18 (5), 540–548. doi:10.1038/ncb3336

PubMed Abstract | CrossRef Full Text | Google Scholar

Engler, A., Bacakova, L., Newman, C., Hategan, A., Griffin, M., and Discher, D. (2004). Substrate Compliance versus Ligand Density in Cell on Gel Responses. Biophysical J. 86 (1), 617–628. doi:10.1016/S0006-3495(04)74140-5

PubMed Abstract | CrossRef Full Text | Google Scholar

Engler, A. J., Humbert, P. O., Wehrle-Haller, B., and Weaver, V. M. (2009). Multiscale Modeling of Form and Function. Science 324 (5924), 208–212. doi:10.1126/science.1170107

PubMed Abstract | CrossRef Full Text | Google Scholar

Étienne, J., Fouchard, J., Mitrossilis, D., Bufi, N., Durand-Smet, P., and Asnacios, A. (2015). Cells as Liquid Motors: Mechanosensitivity Emerges from Collective Dynamics of Actomyosin Cortex. Proc. Natl. Acad. Sci. 112 (9), 2740–2745. doi:10.1073/pnas.1417113112

CrossRef Full Text | Google Scholar

Eze, M. O. (1992). Membrane Fluidity, Reactive Oxygen Species, and Cell-Mediated Immunity: Implications in Nutrition and Disease. Med. Hypotheses 37 (4), 220–224. doi:10.1016/0306-9877(92)90191-E

PubMed Abstract | CrossRef Full Text | Google Scholar

Fabry, B., Maksym, G. N., Butler, J. P., Glogauer, M., Navajas, D., Taback, N. A., et al. (2003). Time Scale and Other Invariants of Integrative Mechanical Behavior in Living Cells. Phys. Rev. E 68 (4), 041914. doi:10.1103/PhysRevE.68.041914

CrossRef Full Text | Google Scholar

Farhadifar, R., Röper, J.-C., Aigouy, B., Eaton, S., and Jülicher, F. (2007). The Influence of Cell Mechanics, Cell-Cell Interactions, and Proliferation on Epithelial Packing. Curr. Biol. 17 (24), 2095–2104. doi:10.1016/j.cub.2007.11.049

PubMed Abstract | CrossRef Full Text | Google Scholar

Fernández-Sánchez, M. E., Barbier, S., Whitehead, J., Béalle, G., Michel, A., Latorre-Ossa, H., et al. (2015). Mechanical Induction of the Tumorigenic β-catenin Pathway by Tumour Growth Pressure. Nature 523 (7558), 92–95. doi:10.1038/nature14329

PubMed Abstract | CrossRef Full Text | Google Scholar

Findley, W. N., Lai, J. S., and Onaran, K. (1976). Creep and Relaxation of Nonlinear Viscoelastic Materials, with an Introduction to Linear Viscoelasticity [recurso Electrónico. North-Holland Pub. Co Sole distributors for the U.S.A. and Canada Elsevier/North Holland.

Google Scholar

Fischer, T., Hayn, A., and Mierke, C. T. (2020). Effect of Nuclear Stiffness on Cell Mechanics and Migration of Human Breast Cancer Cells. Front. Cell Developmental Biol. 8, 393. doi:10.3389/fcell.2020.00393

PubMed Abstract | CrossRef Full Text | Google Scholar

Fischer, T., Wilharm, N., Hayn, A., and Mierke, C. T. (2017). Matrix and Cellular Mechanical Properties Are the Driving Factors for Facilitating Human Cancer Cell Motility into 3D Engineered Matrices. Convergent Sci. Phys. Oncol. 3 (4), 044003. doi:10.1088/2057-1739/aa8bbb

CrossRef Full Text | Google Scholar

Fischer-Cripps, A. C. (2004). A Simple Phenomenological Approach to Nanoindentation Creep. Mater. Sci. Eng. A 385 (1–2), 74–82. doi:10.1016/j.msea.2004.04.070

CrossRef Full Text | Google Scholar

Friedl, P., Locker, J., Sahai, E., and Segall, J. E. (2012). Classifying Collective Cancer Cell Invasion. Nat. Cell Biol. 14 (8), 777–783. doi:10.1038/ncb2548

PubMed Abstract | CrossRef Full Text | Google Scholar

Fuhrmann, A., Staunton, J. R., Nandakumar, V., Banyai, N., Davies, P. C. W., and Ros, R. (2011). AFM Stiffness Nanotomography of normal, Metaplastic and Dysplastic Human Esophageal Cells. Phys. Biol. 8 (1), 015007. doi:10.1088/1478-3975/8/1/015007

PubMed Abstract | CrossRef Full Text | Google Scholar

Fung, Y.-C. (1993). Biomechanics Mechanical Properties of Living Tissues. doi:10.1007/978-1-4757-2257-4

CrossRef Full Text | Google Scholar

Fung, Y. (1967). Elasticity of Soft Tissues in Simple Elongation. Am. J. Physiology-Legacy Content 213 (6), 1532–1544. doi:10.1152/ajplegacy.1967.213.6.1532

CrossRef Full Text | Google Scholar

García, A. J. (2014). PEG–Maleimide Hydrogels for Protein and Cell Delivery in Regenerative Medicine. Ann. Biomed. Eng. 42 (2), 312–322. doi:10.1007/s10439-013-0870-y

PubMed Abstract | CrossRef Full Text | Google Scholar

Gardel, M. L., Nakamura, F., Hartwig, J. H., Crocker, J. C., Stossel, T. P., and Weitz, D. A. (2006). Prestressed F-Actin Networks Cross-Linked by Hinged Filamins Replicate Mechanical Properties of Cells. Proc. Natl. Acad. Sci. 103 (6), 1762–1767. doi:10.1073/pnas.0504777103

CrossRef Full Text | Google Scholar

Gardel, M. L., Shin, J. H., MacKintosh, F. C., Mahadevan, L., Matsudaira, P., and Weitz, D. A. (2004). Elastic Behavior of Cross-Linked and Bundled Actin Networks. Science 304 (5675), 1301–1305. doi:10.1126/science.1095087

PubMed Abstract | CrossRef Full Text | Google Scholar

Gautieri, A., Vesentini, S., Redaelli, A., and Ballarini, R. (2013). Modeling and Measuring Visco-Elastic Properties: From Collagen Molecules to Collagen Fibrils. Int. J. Non-Linear Mech. 56, 25–33. doi:10.1016/j.ijnonlinmec.2013.03.012

CrossRef Full Text | Google Scholar

Gensbittel, V., Kräter, M., Harlepp, S., Busnelli, I., Guck, J., and Goetz, J. G. (2021). Mechanical Adaptability of Tumor Cells in Metastasis. Developmental Cell 56 (2), 164–179. doi:10.1016/j.devcel.2020.10.011

PubMed Abstract | CrossRef Full Text | Google Scholar

Ghassemi, S., Meacci, G., Liu, S., Gondarenko, A. A., Mathur, A., Roca-Cusachs, P., et al. (2012). Cells Test Substrate Rigidity by Local Contractions on Submicrometer Pillars. Proc. Natl. Acad. Sci. 109 (14), 5328–5333. doi:10.1073/pnas.1119886109

PubMed Abstract | CrossRef Full Text | Google Scholar

Gong, Z., Szczesny, S. E., Caliari, S. R., Charrier, E. E., Chaudhuri, O., Cao, X., et al. (2018). Matching Material and Cellular Timescales Maximizes Cell Spreading on Viscoelastic Substrates. Proc. Natl. Acad. Sci. 115 (12), E2686–E2695. doi:10.1073/pnas.1716620115

PubMed Abstract | CrossRef Full Text | Google Scholar

Grevesse, T., Versaevel, M., Circelli, G., Desprez, S., and Gabriele, S. (2013). A Simple Route to Functionalize Polyacrylamide Hydrogels for the Independent Tuning of Mechanotransduction Cues. Lab. A Chip 13 (5), 777. doi:10.1039/c2lc41168g

PubMed Abstract | CrossRef Full Text | Google Scholar

Guck, J., Schinkinger, S., Lincoln, B., Wottawah, F., Ebert, S., Romeyke, M., et al. (2005). Optical Deformability as an Inherent Cell Marker for Testing Malignant Transformation and Metastatic Competence. Biophysical J. 88 (5), 3689–3698. doi:10.1529/biophysj.104.045476

PubMed Abstract | CrossRef Full Text | Google Scholar

Gudipaty, S. A., Lindblom, J., Loftus, P. D., Redd, M. J., Edes, K., Davey, C. F., et al. (2017). Mechanical Stretch Triggers Rapid Epithelial Cell Division through Piezo1. Nature 543 (7643), 118–121. doi:10.1038/nature21407

PubMed Abstract | CrossRef Full Text | Google Scholar

Guo, W., Frey, M. T., Burnham, N. A., and Wang, Y. (2006). Substrate Rigidity Regulates the Formation and Maintenance of Tissues. Biophysical J. 90 (6), 2213–2220. doi:10.1529/biophysj.105.070144

PubMed Abstract | CrossRef Full Text | Google Scholar

Guvendiren, M., and Burdick, J. A. (2012). Stiffening Hydrogels to Probe Short- and Long-Term Cellular Responses to Dynamic Mechanics. Nat. Commun. 3 (1), 792. doi:10.1038/ncomms1792

PubMed Abstract | CrossRef Full Text | Google Scholar

Hall, A. (2009). The Cytoskeleton and Cancer. Cancer Metastasis Rev. 28 (1–2), 5–14. doi:10.1007/s10555-008-9166-3

PubMed Abstract | CrossRef Full Text | Google Scholar

Hall, M. S., Alisafaei, F., Ban, E., Feng, X., Hui, C.-Y., Shenoy, V. B., et al. (2016). Fibrous Nonlinear Elasticity Enables Positive Mechanical Feedback between Cells and ECMs. Proc. Natl. Acad. Sci. 113 (49), 14043–14048. doi:10.1073/pnas.1613058113

PubMed Abstract | CrossRef Full Text | Google Scholar

Han, S. J., Bielawski, K. S., Ting, L. H., Rodriguez, M. L., and Sniadecki, N. J. (2012). Decoupling Substrate Stiffness, Spread Area, and Micropost Density: A Close Spatial Relationship between Traction Forces and Focal Adhesions. Biophysical J. 103 (4), 640–648. doi:10.1016/j.bpj.2012.07.023

CrossRef Full Text | Google Scholar

Han, W., Chen, S., Yuan, W., Fan, Q., Tian, J., Wang, X., et al. (2016). Oriented Collagen Fibers Direct Tumor Cell Intravasation. Proc. Natl. Acad. Sci. 113 (40), 11208–11213. doi:10.1073/pnas.1610347113

PubMed Abstract | CrossRef Full Text | Google Scholar

Han, Y. L., Ronceray, P., Xu, G., Malandrino, A., Kamm, R. D., Lenz, M., et al. (2018). Cell Contraction Induces Long-Ranged Stress Stiffening in the Extracellular Matrix. Proc. Natl. Acad. Sci. 115 (16), 4075–4080. doi:10.1073/pnas.1722619115

PubMed Abstract | CrossRef Full Text | Google Scholar

Hanahan, D., and Weinberg, R. A. (2011). Hallmarks of Cancer: The Next Generation. Cell 144 (5), 646–674. doi:10.1016/j.cell.2011.02.013

PubMed Abstract | CrossRef Full Text | Google Scholar

Hanahan, D., and Weinberg, R. A. (2000). The Hallmarks of Cancer. Cell 100 (1), 57–70. doi:10.1016/S0092-8674(00)81683-9

PubMed Abstract | CrossRef Full Text | Google Scholar

Harris, T. H., Banigan, E. J., Christian, D. A., Konradt, C., Tait Wojno, E. D., Norose, K., et al. (2012). Generalized Lévy Walks and the Role of Chemokines in Migration of Effector CD8+ T Cells. Nature 486 (7404), 545–548. doi:10.1038/nature11098

PubMed Abstract | CrossRef Full Text | Google Scholar

Hayer, A., Shao, L., Chung, M., Joubert, L.-M., Yang, H. W., Tsai, F.-C., et al. (2016). Engulfed Cadherin Fingers Are Polarized Junctional Structures between Collectively Migrating Endothelial Cells. Nat. Cell Biol. 18 (12), 1311–1323. doi:10.1038/ncb3438

PubMed Abstract | CrossRef Full Text | Google Scholar

Herbison, R., Evans, S., Doherty, J.-F., Algie, M., Kleffmann, T., and Poulin, R. (2019). A Molecular War: Convergent and Ontogenetic Evidence for Adaptive Host Manipulation in Related Parasites Infecting Divergent Hosts. Proc. R. Soc. B: Biol. Sci. 286 (1915), 20191827. doi:10.1098/rspb.2019.1827

CrossRef Full Text | Google Scholar

Hochmuth, R. M. (2000). Micropipette Aspiration of Living Cells. J. Biomech. 33 (1), 15–22. doi:10.1016/S0021-9290(99)00175-X

CrossRef Full Text | Google Scholar

Hoffman, B. D., Massiera, G., Van Citters, K. M., and Crocker, J. C. (2006). The Consensus Mechanics of Cultured Mammalian Cells. Proc. Natl. Acad. Sci. 103 (27), 10259–10264. doi:10.1073/pnas.0510348103

PubMed Abstract | CrossRef Full Text | Google Scholar

Hoshino, A., Costa-Silva, B., Shen, T.-L., Rodrigues, G., Hashimoto, A., Tesic Mark, M., et al. (2015). Tumour Exosome Integrins Determine Organotropic Metastasis. Nature 527 (7578), 329–335. doi:10.1038/nature15756

PubMed Abstract | CrossRef Full Text | Google Scholar

Hsu, H.-J., Lee, C.-F., and Kaunas, R. (2009). A Dynamic Stochastic Model of Frequency-dependent Stress Fiber Alignment Induced by Cyclic Stretch. PLoS ONE 4 (3), e4853. doi:10.1371/journal.pone.0004853

PubMed Abstract | CrossRef Full Text | Google Scholar

Huang, C., Butler, P. J., Tong, S., Muddana, H. S., Bao, G., and Zhang, S. (2013). Substrate Stiffness Regulates Cellular Uptake of Nanoparticles. Nano Lett. 13 (4), 1611–1615. doi:10.1021/nl400033h

PubMed Abstract | CrossRef Full Text | Google Scholar

Huang, S., and Ingber, D. E. (2005). Cell Tension, Matrix Mechanics, and Cancer Development. Cancer Cell 8 (3), 175–176. doi:10.1016/j.ccr.2005.08.009

PubMed Abstract | CrossRef Full Text | Google Scholar

Iwanaga, Y., Braun, D., and Fromherz, P. (2001). No Correlation of Focal Contacts and Close Adhesion by Comparing GFP-Vinculin and Fluorescence Interference of DiI. Eur. Biophys. J. 30 (1), 17–26. doi:10.1007/s002490000119

PubMed Abstract | CrossRef Full Text | Google Scholar

Jansen, K. A., Licup, A. J., Sharma, A., Rens, R., MacKintosh, F. C., and Koenderink, G. H. (2018). The Role of Network Architecture in Collagen Mechanics. Biophysical J. 114 (11), 2665–2678. doi:10.1016/j.bpj.2018.04.043

PubMed Abstract | CrossRef Full Text | Google Scholar

Jiang, M. Q., Wilde, G., and Dai, L. H. (2015). Origin of Stress Overshoot in Amorphous Solids. Mech. Mater. 81, 72–83. doi:10.1016/j.mechmat.2014.10.002

CrossRef Full Text | Google Scholar

Kano, A. (2015). Tumor Cell Secretion of Soluble Factor(s) for Specific Immunosuppression. Scientific Rep. 5 (1), 8913. doi:10.1038/srep08913

PubMed Abstract | CrossRef Full Text | Google Scholar

Ketene, A. N., Schmelz, E. M., Roberts, P. C., and Agah, M. (2012). The Effects of Cancer Progression on the Viscoelasticity of Ovarian Cell Cytoskeleton Structures. Nanomedicine: Nanotechnology, Biol. Med. 8 (1), 93–102. doi:10.1016/j.nano.2011.05.012

CrossRef Full Text | Google Scholar

Kim, S. (2011). Viscoelastic Behaviors in Polymeric Nanodroplet Collisions. Phys. Rev. E 83 (4), 041302. doi:10.1103/PhysRevE.83.041302

PubMed Abstract | CrossRef Full Text | Google Scholar

Klein, E. A., Yin, L., Kothapalli, D., Castagnino, P., Byfield, F. J., Xu, T., et al. (2009). Cell-Cycle Control by Physiological Matrix Elasticity and In Vivo Tissue Stiffening. Curr. Biol. 19 (18), 1511–1518. doi:10.1016/j.cub.2009.07.069

PubMed Abstract | CrossRef Full Text | Google Scholar

Koch, D., Rosoff, W. J., Jiang, J., Geller, H. M., and Urbach, J. S. (2012). Strength in the Periphery: Growth Cone Biomechanics and Substrate Rigidity Response in Peripheral and Central Nervous System Neurons. Biophysical J. 102 (3), 452–460. doi:10.1016/j.bpj.2011.12.025

CrossRef Full Text | Google Scholar

Kollmannsberger, P., Mierke, C. T., and Fabry, B. (2011). Nonlinear Viscoelasticity of Adherent Cells Is Controlled by Cytoskeletal Tension. Soft Matter 7 (7), 3127–3132. doi:10.1039/C0SM00833H

CrossRef Full Text | Google Scholar

Koontz, E., Blouin, V., Wachtel, P., Musgraves, J. D., and Richardson, K. (2012). Prony Series Spectra of Structural Relaxation in N-BK7 for Finite Element Modeling. The J. Phys. Chem. A 116 (50), 12198–12205. doi:10.1021/jp307717q

CrossRef Full Text | Google Scholar

Krairi, A., and Doghri, I. (2014). A Thermodynamically-Based Constitutive Model for Thermoplastic Polymers Coupling Viscoelasticity, Viscoplasticity and Ductile Damage. Int. J. Plasticity 60, 163–181. doi:10.1016/j.ijplas.2014.04.010

CrossRef Full Text | Google Scholar

Kremer, K., and Grest, G. S. (1990). Dynamics of Entangled Linear Polymer Melts: A Molecular‐dynamics Simulation. J. Chem. Phys. 92 (8), 5057–5086. doi:10.1063/1.458541

CrossRef Full Text | Google Scholar

Kumar, S., Maxwell, I. Z., Heisterkamp, A., Polte, T. R., Lele, T. P., Salanga, M., et al. (2006). Viscoelastic Retraction of Single Living Stress Fibers and its Impact on Cell Shape, Cytoskeletal Organization, and Extracellular Matrix Mechanics. Biophysical J. 90 (10), 3762–3773. doi:10.1529/biophysj.105.071506

PubMed Abstract | CrossRef Full Text | Google Scholar

Kumar, S., and Weaver, V. M. (2009). Mechanics, Malignancy, and Metastasis: The Force Journey of a Tumor Cell. Cancer Metastasis Rev. 28 (1–2), 113–127. doi:10.1007/s10555-008-9173-4

PubMed Abstract | CrossRef Full Text | Google Scholar

Lautenschläger, F., Paschke, S., Schinkinger, S., Bruel, A., Beil, M., and Guck, J. (2009). The Regulatory Role of Cell Mechanics for Migration of Differentiating Myeloid Cells. Proc. Natl. Acad. Sci. 106 (37), 15696–15701. doi:10.1073/pnas.0811261106

CrossRef Full Text | Google Scholar

Le Maout, V., Alessandri, K., Gurchenkov, B., Bertin, H., Nassoy, P., and Sciumè, G. (2020). Role of Mechanical Cues and Hypoxia on the Growth of Tumor Cells in strong and Weak Confinement: A Dual In Vitro–In Silico Approach. Sci. Adv. 6 (13), eaaz7130. doi:10.1126/sciadv.aaz7130

PubMed Abstract | CrossRef Full Text | Google Scholar

Lee, B., Konen, J., Wilkinson, S., Marcus, A. I., and Jiang, Y. (2017). Local Alignment Vectors Reveal Cancer Cell-Induced ECM Fiber Remodeling Dynamics. Scientific Rep. 7 (1), 39498. doi:10.1038/srep39498

PubMed Abstract | CrossRef Full Text | Google Scholar

Lee, G. Y. H., and Lim, C. T. (2007). Biomechanics Approaches to Studying Human Diseases. Trends Biotechnol. 25 (3), 111–118. doi:10.1016/j.tibtech.2007.01.005

PubMed Abstract | CrossRef Full Text | Google Scholar

Levental, K. R., Yu, H., Kass, L., Lakins, J. N., Egeblad, M., Erler, J. T., et al. (2009). Matrix Crosslinking Forces Tumor Progression by Enhancing Integrin Signaling. Cell 139 (5), 891–906. doi:10.1016/j.cell.2009.10.027

PubMed Abstract | CrossRef Full Text | Google Scholar

Levin, A., and Wyman, J. (1927). The Viscous Elastic Properties of Muscle. Proc. R. Soc. Lond. Ser. B, Containing Pap. a Biol. Character 101 (709), 218–243. doi:10.1098/rspb.1927.0014

CrossRef Full Text | Google Scholar

Li, B., and Sun, S. X. (2014). Coherent Motions in Confluent Cell Monolayer Sheets. Biophysical J. 107 (7), 1532–1541. doi:10.1016/j.bpj.2014.08.006

PubMed Abstract | CrossRef Full Text | Google Scholar

Licup, A. J., Münster, S., Sharma, A., Sheinman, M., Jawerth, L. M., Fabry, B., et al. (2015). Stress Controls the Mechanics of Collagen Networks. Proc. Natl. Acad. Sci. 112 (31), 9573–9578. doi:10.1073/pnas.1504258112

PubMed Abstract | CrossRef Full Text | Google Scholar

Likhtman, A. E., Sukumaran, S. K., and Ramirez, J. (2007). Linear Viscoelasticity from Molecular Dynamics Simulation of Entangled Polymers. Macromolecules 40 (18), 6748–6757. doi:10.1021/ma070843b

CrossRef Full Text | Google Scholar

Lim, C. T. (2006). Single Cell Mechanics Study of the Human Disease Malaria. J. Biomechanical Sci. Eng. 1 (1), 82–92. doi:10.1299/jbse.1.82

CrossRef Full Text | Google Scholar

Lim, C. T., Zhou, E. H., and Quek, S. T. (2006). Mechanical Models for Living Cells—A Review. J. Biomech. 39 (2), 195–216. doi:10.1016/j.jbiomech.2004.12.008

CrossRef Full Text | Google Scholar

Lin, S.-Z., Li, B., Xu, G.-K., and Feng, X.-Q. (2017). Collective Dynamics of Cancer Cells Confined in a Confluent Monolayer of normal Cells. J. Biomech. 52, 140–147. doi:10.1016/j.jbiomech.2016.12.035

CrossRef Full Text | Google Scholar

Liu, A. S., Wang, H., Copeland, C. R., Chen, C. S., Shenoy, V. B., and Reich, D. H. (2016). Matrix Viscoplasticity and its Shielding by Active Mechanics in Microtissue Models: Experiments and Mathematical Modeling. Scientific Rep. 6 (1), 33919. doi:10.1038/srep33919

PubMed Abstract | CrossRef Full Text | Google Scholar

Loebel, C., Mauck, R. L., and Burdick, J. A. (2019). Local Nascent Protein Deposition and Remodelling Guide Mesenchymal Stromal Cell Mechanosensing and Fate in Three-Dimensional Hydrogels. Nat. Mater. 18 (8), 883–891. doi:10.1038/s41563-019-0307-6

PubMed Abstract | CrossRef Full Text | Google Scholar

Lou, J., Stowers, R., Nam, S., Xia, Y., and Chaudhuri, O. (2018). Stress Relaxing Hyaluronic Acid-Collagen Hydrogels Promote Cell Spreading, Fiber Remodeling, and Focal Adhesion Formation in 3D Cell Culture. Biomaterials 154, 213–222. doi:10.1016/j.biomaterials.2017.11.004

PubMed Abstract | CrossRef Full Text | Google Scholar

Lu, Y.-B., Franze, K., Seifert, G., Steinhauser, C., Kirchhoff, F., Wolburg, H., et al. (2006). Viscoelastic Properties of Individual Glial Cells and Neurons in the CNS. Proc. Natl. Acad. Sci. 103 (47), 17759–17764. doi:10.1073/pnas.0606150103

PubMed Abstract | CrossRef Full Text | Google Scholar

Lv, J., Liu, Y., Cheng, F., Li, J., Zhou, Y., Zhang, T., et al. (2021). Cell Softness Regulates Tumorigenicity and Stemness of Cancer Cells. EMBO J. 40 (2). doi:10.15252/embj.2020106123

CrossRef Full Text | Google Scholar

Maciaszek, J. L., Andemariam, B., and Lykotrafitis, G. (2011). Microelasticity of Red Blood Cells in Sickle Cell Disease. J. Strain Anal. Eng. Des. 46 (5), 368–379. doi:10.1177/0309324711398809

CrossRef Full Text | Google Scholar

MacKintosh, F. C., Käs, J., and Janmey, P. A. (1995). Elasticity of Semiflexible Biopolymer Networks. Phys. Rev. Lett. 75 (24), 4425–4428. doi:10.1103/PhysRevLett.75.4425

PubMed Abstract | CrossRef Full Text | Google Scholar

Malinverno, C., Corallino, S., Giavazzi, F., Bergert, M., Li, Q., Leoni, M., et al. (2017). Endocytic Reawakening of Motility in Jammed Epithelia. Nat. Mater. 16 (5), 587–596. doi:10.1038/nmat4848

PubMed Abstract | CrossRef Full Text | Google Scholar

Manning, M. L., Foty, R. A., Steinberg, M. S., and Schoetz, E.-M. (2010). Coaction of Intercellular Adhesion and Cortical Tension Specifies Tissue Surface Tension. Proc. Natl. Acad. Sci. 107 (28), 12517–12522. doi:10.1073/pnas.1003743107

PubMed Abstract | CrossRef Full Text | Google Scholar

Martinez-Garcia, F. D., de Hilster, R. H. J., Sharma, P. K., Borghuis, T., Hylkema, M. N., Burgess, J. K., et al. (2021). Architecture and Composition Dictate Viscoelastic Properties of Organ-Derived Extracellular Matrix Hydrogels. Polymers 13 (18), 3113. doi:10.3390/polym13183113

PubMed Abstract | CrossRef Full Text | Google Scholar

Mathieu, S., and Manneville, J.-B. (2019). Intracellular Mechanics: Connecting Rheology and Mechanotransduction. Curr. Opin. Cell Biol. 56, 34–44. doi:10.1016/j.ceb.2018.08.007

PubMed Abstract | CrossRef Full Text | Google Scholar

Mattei, G., Cacopardo, L., and Ahluwalia, A. (2020). Engineering Gels with Time-Evolving Viscoelasticity. Materials 13 (2), 438. doi:10.3390/ma13020438

PubMed Abstract | CrossRef Full Text | Google Scholar

McCrum, N. G., Read, B. E., and Williams, G. (1991). Anelastic and Dielectric Effects in Polymeric Solids. Dover ed. Dover Publications.

Google Scholar

McKinnon, D. D., Domaille, D. W., Cha, J. N., and Anseth, K. S. (2014). Biophysically Defined and Cytocompatible Covalently Adaptable Networks as Viscoelastic 3D Cell Culture Systems. Adv. Mater. (Deerfield Beach, Fla.) 26 (6), 865–872. doi:10.1002/adma.201303680

CrossRef Full Text | Google Scholar

Merkel, M., and Manning, M. L. (2018). A Geometrically Controlled Rigidity Transition in a Model for Confluent 3D Tissues. New J. Phys. 20 (2), 022002. doi:10.1088/1367-2630/aaaa13

CrossRef Full Text | Google Scholar

Mierke, C. T., Frey, B., Fellner, M., Herrmann, M., and Fabry, B. (2011). Integrin α5β1 Facilitates Cancer Cell Invasion through Enhanced Contractile Forces. J. Cell Sci. 124 (3), 369–383. doi:10.1242/jcs.071985

CrossRef Full Text | Google Scholar

Mierke, C. T. (2020). Mechanical Cues Affect Migration and Invasion of Cells from Three Different Directions. Front. Cell Developmental Biol. 8, 583226. doi:10.3389/fcell.2020.583226

PubMed Abstract | CrossRef Full Text | Google Scholar

Mierke, C. T. (2014). The Fundamental Role of Mechanical Properties in the Progression of Cancer Disease and Inflammation. Rep. Prog. Phys. 77 (7), 076602. doi:10.1088/0034-4885/77/7/076602

PubMed Abstract | CrossRef Full Text | Google Scholar

Mierke, C. T. (2019). The Matrix Environmental and Cell Mechanical Properties Regulate Cell Migration and Contribute to the Invasive Phenotype of Cancer Cells. Rep. Prog. Phys. 82 (6), 064602. doi:10.1088/1361-6633/ab1628

PubMed Abstract | CrossRef Full Text | Google Scholar

Moeendarbary, E., and Harris, A. R. (2014). Cell Mechanics: Principles, Practices, and Prospects: Cell Mechanics. Wiley Interdiscip. Rev. Syst. Biol. Med. 6 (5), 371–388. doi:10.1002/wsbm.1275

PubMed Abstract | CrossRef Full Text | Google Scholar

Mongera, A., Pochitaloff, M., Gustafson, H. J., Stooke-Vaughan, G. A., Rowghanian, P., and Campàs, O. (2021). Mechanics Of the Cellular Microenvironment as Perceived by Cells In Vivo [Preprint]. Biophysics. doi:10.1101/2021.01.04.425259

CrossRef Full Text | Google Scholar

Mongera, A., Rowghanian, P., Gustafson, H. J., Shelton, E., Kealhofer, D. A., Carn, E. K., et al. (2018). A Fluid-To-Solid Jamming Transition Underlies Vertebrate Body axis Elongation. Nature 561 (7723), 401–405. doi:10.1038/s41586-018-0479-2

PubMed Abstract | CrossRef Full Text | Google Scholar

Monsia, M. D. (2011). A Simplified Nonlinear Generalized Maxwell Model for Predicting the Time Dependent Behavior of Viscoelastic Materials. World J. Mech. 01 (03), 158–167. doi:10.4236/wjm.2011.13021

CrossRef Full Text | Google Scholar

Mueller, J., Szep, G., Nemethova, M., de Vries, I., Lieber, A. D., Winkler, C., et al. (2017). Load Adaptation of Lamellipodial Actin Networks. Cell 171 (1), 188–200. e16. doi:10.1016/j.cell.2017.07.051

PubMed Abstract | CrossRef Full Text | Google Scholar

Muiznieks, L. D., and Keeley, F. W. (2013). Molecular Assembly and Mechanical Properties of the Extracellular Matrix: A Fibrous Protein Perspective. Biochim. Biophys. Acta (Bba) - Mol. Basis Dis. 1832 (7), 866–875. doi:10.1016/j.bbadis.2012.11.022

CrossRef Full Text | Google Scholar

Müller, M., Seidel, U., and Stadler, R. (1995). Influence of Hydrogen Bonding on the Viscoelastic Properties of Thermoreversible Networks: Analysis of the Local Complex Dynamics. Polymer 36 (16), 3143–3150. doi:10.1016/0032-3861(95)97877-I

CrossRef Full Text | Google Scholar

Naba, A., Clauser, K. R., Hoersch, S., Liu, H., Carr, S. A., and Hynes, R. O. (2012). The Matrisome: In Silico Definition and In Vivo Characterization by Proteomics of Normal and Tumor Extracellular Matrices. Mol. Cell Proteomics 11 (4), M111014647. doi:10.1074/mcp.M111.014647

CrossRef Full Text | Google Scholar

Nam, S., Hu, K. H., Butte, M. J., and Chaudhuri, O. (2016). Strain-enhanced Stress Relaxation Impacts Nonlinear Elasticity in Collagen Gels. Proc. Natl. Acad. Sci. 113 (20), 5492–5497. doi:10.1073/pnas.1523906113

PubMed Abstract | CrossRef Full Text | Google Scholar

Nam, S., Stowers, R., Lou, J., Xia, Y., and Chaudhuri, O. (2019). Varying PEG Density to Control Stress Relaxation in Alginate-PEG Hydrogels for 3D Cell Culture Studies. Biomaterials 200, 15–24. doi:10.1016/j.biomaterials.2019.02.004

PubMed Abstract | CrossRef Full Text | Google Scholar

Narasimhan, B. N., Deijs, G. S., Manuguri, S., Ting, M. S. H., Williams, M. A. K., and Malmström, J. (2021). A Comparative Study of Tough Hydrogen Bonding Dissipating Hydrogels Made with Different Network Structures. Nanoscale Adv. 3 (10), 2934–2947. doi:10.1039/D1NA00103E

CrossRef Full Text | Google Scholar

Nekouzadeh, A., Pryse, K. M., Elson, E. L., and Genin, G. M. (2008). Stretch-activated Force Shedding, Force Recovery, and Cytoskeletal Remodeling in Contractile Fibroblasts. J. Biomech. 41 (14), 2964–2971. doi:10.1016/j.jbiomech.2008.07.033

CrossRef Full Text | Google Scholar

Nelson, C. M., VanDuijn, M. M., Inman, J. L., Fletcher, D. A., and Bissell, M. J. (2006). Tissue Geometry Determines Sites of Mammary Branching Morphogenesis in Organotypic Cultures. Science 314 (5797), 298–300. doi:10.1126/science.1131000

PubMed Abstract | CrossRef Full Text | Google Scholar

Nematbakhsh, Y., Pang, K. T., and Lim, C. T. (2017). Correlating the Viscoelasticity of Breast Cancer Cells with Their Malignancy. Convergent Sci. Phys. Oncol. 3 (3), 034003. doi:10.1088/2057-1739/aa7ffb

CrossRef Full Text | Google Scholar

Nia, H. T., Bozchalooi, I. S., Li, Y., Han, L., Hung, H.-H., Frank, E., et al. (2013). High-Bandwidth AFM-Based Rheology Reveals that Cartilage Is Most Sensitive to High Loading Rates at Early Stages of Impairment. Biophysical J. 104 (7), 1529–1537. doi:10.1016/j.bpj.2013.02.048

PubMed Abstract | CrossRef Full Text | Google Scholar

Nisenholz, N., Rajendran, K., Dang, Q., Chen, H., Kemkemer, R., Krishnan, R., et al. (2014). Active Mechanics and Dynamics of Cell Spreading on Elastic Substrates. Soft Matter 10 (37), 7234. doi:10.1039/C4SM00780H

PubMed Abstract | CrossRef Full Text | Google Scholar

Norris, S. A. (2012). Stability Analysis of a Viscoelastic Model for Ion-Irradiated Silicon. Phys. Rev. B 85 (15), 155325. doi:10.1103/PhysRevB.85.155325

CrossRef Full Text | Google Scholar

O’Brien, B., McKay, T., Calius, E., Xie, S., and Anderson, I. (2009). Finite Element Modelling of Dielectric Elastomer Minimum Energy Structures. Appl. Phys. A 94 (3), 507–514. doi:10.1007/s00339-008-4946-8

CrossRef Full Text | Google Scholar

Park, J.-A., Kim, J. H., Bi, D., Mitchel, J. A., Qazvini, N. T., Tantisira, K., et al. (2015). Unjamming and Cell Shape in the Asthmatic Airway Epithelium. Nat. Mater. 14 (10), 1040–1048. doi:10.1038/nmat4357

PubMed Abstract | CrossRef Full Text | Google Scholar

Park, K., Lonsberry, G. E., Gearing, M., Levey, A. I., and Desai, J. P. (2019). Viscoelastic Properties of Human Autopsy Brain Tissues as Biomarkers for Alzheimer’s Diseases. IEEE Trans. Biomed. Eng. 66 (6), 1705–1713. doi:10.1109/TBME.2018.2878555

PubMed Abstract | CrossRef Full Text | Google Scholar

Park, S. W., and Kim, Y. R. (2001). Fitting Prony-Series Viscoelastic Models with Power-Law Presmoothing. J. Mater. Civil Eng. 13 (1), 26–32. doi:10.1061/(asce)0899-1561(2001)13:1(26)

CrossRef Full Text | Google Scholar

Parsons, J. T., Martin, K. H., Slack, J. K., Taylor, J. M., and Weed, S. A. (2000). Focal Adhesion Kinase: A Regulator of Focal Adhesion Dynamics and Cell Movement. Oncogene 19 (49), 5606–5613. doi:10.1038/sj.onc.1203877

PubMed Abstract | CrossRef Full Text | Google Scholar

Paul, D. (2020). The Systemic Hallmarks of Cancer. J. Cancer Metastasis Treat. 2020. doi:10.20517/2394-4722.2020.63

CrossRef Full Text | Google Scholar

Petridou, N. I., Corominas-Murtra, B., Heisenberg, C.-P., and Hannezo, E. (2021). Rigidity Percolation Uncovers a Structural Basis for Embryonic Tissue Phase Transitions. Cell 184 (7), 1914–1928. e19. doi:10.1016/j.cell.2021.02.017

PubMed Abstract | CrossRef Full Text | Google Scholar

Petridou, N. I., Grigolon, S., Salbreux, G., Hannezo, E., and Heisenberg, C.-P. (2019). Fluidization-mediated Tissue Spreading by Mitotic Cell Rounding and Non-canonical Wnt Signalling. Nat. Cell Biol. 21 (2), 169–178. doi:10.1038/s41556-018-0247-4

PubMed Abstract | CrossRef Full Text | Google Scholar

Petrie, R. J., and Yamada, K. M. (2012). At the Leading Edge of Three-Dimensional Cell Migration. J. Cell Sci. 125, 093732. doi:10.1242/jcs.093732

CrossRef Full Text | Google Scholar

Poh, Y.-C., Chen, J., Hong, Y., Yi, H., Zhang, S., Chen, J., et al. (2014). Generation of Organized Germ Layers from a Single Mouse Embryonic Stem Cell. Nat. Commun. 5 (1), 4000. doi:10.1038/ncomms5000

PubMed Abstract | CrossRef Full Text | Google Scholar

Prabhune, M., Belge, G., Dotzauer, A., Bullerdiek, J., and Radmacher, M. (2012). Comparison of Mechanical Properties of normal and Malignant Thyroid Cells. Micron 43 (12), 1267–1272. doi:10.1016/j.micron.2012.03.023

PubMed Abstract | CrossRef Full Text | Google Scholar

Prior, C., Moussou, J., Chakrabarti, B., Jensen, O. E., and Juel, A. (2016). Ribbon Curling via Stress Relaxation in Thin Polymer Films. Proc. Natl. Acad. Sci. 113 (7), 1719–1724. doi:10.1073/pnas.1514626113

PubMed Abstract | CrossRef Full Text | Google Scholar

Pritchard, R. H., Shery Huang, Y. Y., and Terentjev, E. M. (2014). Mechanics of Biological Networks: From the Cell Cytoskeleton to Connective Tissue. Soft Matter 10 (12), 1864. doi:10.1039/c3sm52769g

PubMed Abstract | CrossRef Full Text | Google Scholar

Provenzano, P. P., Inman, D. R., Eliceiri, K. W., Knittel, J. G., Yan, L., Rueden, C. T., et al. (2008). Collagen Density Promotes Mammary Tumor Initiation and Progression. BMC Med. 6 (1), 11. doi:10.1186/1741-7015-6-11

PubMed Abstract | CrossRef Full Text | Google Scholar

Provenzano, P. P., Lakes, R. S., Corr, D. T., and Vanderby, R. (2002). Application of Nonlinear Viscoelastic Models to Describe Ligament Behavior. Biomech. Model. Mechanobiology 1 (1), 45–57. doi:10.1007/s10237-002-0004-1

PubMed Abstract | CrossRef Full Text | Google Scholar

Qian, J., and Gao, H. (2010). Soft Matrices Suppress Cooperative Behaviors Among Receptor-Ligand Bonds in Cell Adhesion. PLoS ONE 5 (8), e12342. doi:10.1371/journal.pone.0012342

PubMed Abstract | CrossRef Full Text | Google Scholar

Qian, J., Wang, J., and Gao, H. (2008). Lifetime and Strength of Adhesive Molecular Bond Clusters between Elastic Media. Langmuir 24 (4), 1262–1270. doi:10.1021/la702401b

PubMed Abstract | CrossRef Full Text | Google Scholar

Radmacher, M., Fritz, M., Kacher, C. M., Cleveland, J. P., and Hansma, P. K. (1996). Measuring the Viscoelastic Properties of Human Platelets with the Atomic Force Microscope. Biophysical J. 70 (1), 556–567. doi:10.1016/S0006-3495(96)79602-9

CrossRef Full Text | Google Scholar

Rianna, C., and Radmacher, M. (2017). Comparison of Viscoelastic Properties of Cancer and normal Thyroid Cells on Different Stiffness Substrates. Eur. Biophys. J. 46 (4), 309–324. doi:10.1007/s00249-016-1168-4

PubMed Abstract | CrossRef Full Text | Google Scholar

Richardson, B. M., Wilcox, D. G., Randolph, M. A., and Anseth, K. S. (2019). Hydrazone Covalent Adaptable Networks Modulate Extracellular Matrix Deposition for Cartilage Tissue Engineering. Acta Biomater. 83, 71–82. doi:10.1016/j.actbio.2018.11.014

PubMed Abstract | CrossRef Full Text | Google Scholar

Rigato, A., Miyagi, A., Scheuring, S., and Rico, F. (2017). High-frequency Microrheology Reveals Cytoskeleton Dynamics in Living Cells. Nat. Phys. 13 (8), 771–775. doi:10.1038/nphys4104

PubMed Abstract | CrossRef Full Text | Google Scholar

Roberts, W. W., Kramer, O., Rosser, R. W., Nestler, F. H. M., and Ferry, J. D. (1974). Rheology of Fibrin Clots. I. Biophysical Chem. 1 (3), 152–160. doi:10.1016/0301-4622(74)80002-5

CrossRef Full Text | Google Scholar

Rodell, C. B., MacArthur, J. W., Dorsey, S. M., Wade, R. J., Wang, L. L., Woo, Y. J., et al. (2015). Shear-Thinning Supramolecular Hydrogels with Secondary Autonomous Covalent Crosslinking to Modulate Viscoelastic Properties In Vivo. Adv. Funct. Mater. 25 (4), 636–644. doi:10.1002/adfm.201403550

PubMed Abstract | CrossRef Full Text | Google Scholar

Rouse, P. E. (1953). A Theory of the Linear Viscoelastic Properties of Dilute Solutions of Coiling Polymers. J. Chem. Phys. 21 (7), 1272–1280. doi:10.1063/1.1699180

CrossRef Full Text | Google Scholar

Roylance, D., Cohen, K. C., Jenkins, C. H., and Khanna, S. K. (2001). Mechanics of Materials: A Materials Science Perspective. Proc. Inst. Mech. Eng. L: J. Mater. Des. Appl. 215 (3), 141–145. doi:10.1243/1464420011544987

CrossRef Full Text | Google Scholar

Rozario, T., Dzamba, B., Weber, G. F., Davidson, L. A., and DeSimone, D. W. (2009). The Physical State of Fibronectin Matrix Differentially Regulates Morphogenetic Movements In Vivo. Developmental Biol. 327 (2), 386–398. doi:10.1016/j.ydbio.2008.12.025

CrossRef Full Text | Google Scholar

Sadati, M., Taheri Qazvini, N., Krishnan, R., Park, C. Y., and Fredberg, J. J. (2013). Collective Migration and Cell Jamming. Differentiation 86 (3), 121–125. doi:10.1016/j.diff.2013.02.005

PubMed Abstract | CrossRef Full Text | Google Scholar

Schapery, R. A. (1969). On the Characterization of Nonlinear Viscoelastic Materials. Polym. Eng. Sci. 9 (4), 295–310. doi:10.1002/pen.760090410

CrossRef Full Text | Google Scholar

Segerer, F. J., Thüroff, F., Piera Alberola, A., Frey, E., and Rädler, J. O. (2015). Emergence and Persistence of Collective Cell Migration on Small Circular Micropatterns. Phys. Rev. Lett. 114 (22), 228102. doi:10.1103/PhysRevLett.114.228102

PubMed Abstract | CrossRef Full Text | Google Scholar

Şengül, Y. (2021). Nonlinear Viscoelasticity of Strain Rate Type: An Overview. Proc. R. Soc. A: Math. Phys. Eng. Sci. 477 (2245), 20200715. doi:10.1098/rspa.2020.0715

CrossRef Full Text | Google Scholar

Sharma, A., Licup, A. J., Jansen, K. A., Rens, R., Sheinman, M., Koenderink, G. H., et al. (2016). Strain-controlled Criticality Governs the Nonlinear Mechanics of Fibre Networks. Nat. Phys. 12 (6), 584–587. doi:10.1038/nphys3628

CrossRef Full Text | Google Scholar

Shenoy, V. B., Wang, H., and Wang, X. (2016). A Chemo-Mechanical Free-Energy-Based Approach to Model Durotaxis and Extracellular Stiffness-dependent Contraction and Polarization of Cells. Interf. Focus 6 (1), 20150067. doi:10.1098/rsfs.2015.0067

PubMed Abstract | CrossRef Full Text | Google Scholar

Simpson, P. T., Reis-Filho, J. S., Gale, T., and Lakhani, S. R. (2005). Molecular Evolution of Breast Cancer: Molecular Evolution of Breast Cancer. J. Pathol. 205 (2), 248–254. doi:10.1002/path.1691

CrossRef Full Text | Google Scholar

Singh, R., Davies, P., and Bajaj, A. K. (2003). Identification of Nonlinear and Viscoelastic Properties of Flexible Polyurethane Foam. Nonlinear Dyn. 34 (3/4), 319–346. doi:10.1023/B:NODY.0000013511.07097.87

CrossRef Full Text | Google Scholar

Slanik, M. L., Nemes, J. A., Potvin, M.-J., and Piedboeuf, J.-C. (2000). Time Domain Finite Element Simulations of Damped Multilayered Beams Using a Prony Series Representation. Mechanics of Time-dependent Materials. Mech. Time-Dependent Mater. 4 (3), 211–230. doi:10.1023/A:1009826923983

CrossRef Full Text | Google Scholar

Soussou, J. E., Moavenzadeh, F., and Gradowczyk, M. H. (1970). Application of Prony Series to Linear Viscoelasticity. Trans. Soc. Rheology 14 (4), 573–584. doi:10.1122/1.549179

CrossRef Full Text | Google Scholar

Staple, D. B., Farhadifar, R., Röper, J.-C., Aigouy, B., Eaton, S., and Jülicher, F. (2010). Mechanics and Remodelling of Cell Packings in Epithelia. The Eur. Phys. J. E 33 (2), 117–127. doi:10.1140/epje/i2010-10677-0

CrossRef Full Text | Google Scholar

Steinwachs, J., Metzner, C., Skodzek, K., Lang, N., Thievessen, I., Mark, C., et al. (2016). Three-dimensional Force Microscopy of Cells in Biopolymer Networks. Nat. Methods 13 (2), 171–176. doi:10.1038/nmeth.3685

PubMed Abstract | CrossRef Full Text | Google Scholar

Stephens, D. C., Osunsanmi, N., Sochacki, K. A., Powell, T. W., Taraska, J. W., and Harris, D. A. (2019). Spatiotemporal Organization and Protein Dynamics Involved in Regulated Exocytosis of MMP-9 in Breast Cancer Cells. J. Gen. Physiol. 151 (12), 1386–1403. doi:10.1085/jgp.201812299

CrossRef Full Text | Google Scholar

Storm, C., Pastore, J. J., MacKintosh, F. C., Lubensky, T. C., and Janmey, P. A. (2005). Nonlinear Elasticity in Biological Gels. Nature 435 (7039), 191–194. doi:10.1038/nature03521

PubMed Abstract | CrossRef Full Text | Google Scholar

Sunyer, R., Conte, V., Escribano, J., Elosegui-Artola, A., Labernadie, A., Valon, L., et al. (2016). Collective Cell Durotaxis Emerges from Long-Range Intercellular Force Transmission. Science 353 (6304), 1157–1161. doi:10.1126/science.aaf7119

PubMed Abstract | CrossRef Full Text | Google Scholar

Swaminathan, V., and Waterman, C. M. (2016). The Molecular Clutch Model for Mechanotransduction Evolves. Nat. Cell Biol. 18 (5), 459–461. doi:10.1038/ncb3350

PubMed Abstract | CrossRef Full Text | Google Scholar

Swift, J., Ivanovska, I. L., Buxboim, A., Harada, T., Dingal, P. C. D. P., Pinter, J., et al. (2013). Nuclear Lamin-A Scales with Tissue Stiffness and Enhances Matrix-Directed Differentiation. Science 341 (6149), 1240104. doi:10.1126/science.1240104

PubMed Abstract | CrossRef Full Text | Google Scholar

Tan, Y., and Song, J. (2021). Independent and Synergistic Modulations of Viscoelasticity and Stiffness of Dynamically Cross-Linked Cell-Encapsulating ClickGels by Covalently Tethered Polymer Brushes. Biomacromolecules 22 (8), 3408–3415. doi:10.1021/acs.biomac.1c00477

PubMed Abstract | CrossRef Full Text | Google Scholar

Tang, S., Ma, H., Tu, H., Wang, H., Lin, P., and Anseth, K. S. (2018). Adaptable Fast Relaxing Boronate‐Based Hydrogels for Probing Cell–Matrix Interactions. Adv. Sci. 5 (9), 1800638. doi:10.1002/advs.201800638

PubMed Abstract | CrossRef Full Text | Google Scholar

Tanner, K., Mori, H., Mroue, R., Bruni-Cardoso, A., and Bissell, M. J. (2012). Coherent Angular Motion in the Establishment of Multicellular Architecture of Glandular Tissues. Proc. Natl. Acad. Sci. 109 (6), 1973–1978. doi:10.1073/pnas.1119578109

CrossRef Full Text | Google Scholar

Terzopoulos, D., and Fleischer, K. (1988). Modeling Inelastic Deformation: Viscolelasticity, Plasticity, Fracture. ACM SIGGRAPH Computer Graphics 22 (4), 269–278. doi:10.1145/378456.378522

CrossRef Full Text | Google Scholar

Tetley, R. J., Staddon, M. F., Heller, D., Hoppe, A., Banerjee, S., and Mao, Y. (2019). Tissue Fluidity Promotes Epithelial Wound Healing. Nat. Phys. 15 (11), 1195–1203. doi:10.1038/s41567-019-0618-1

PubMed Abstract | CrossRef Full Text | Google Scholar

The Physical Sciences - Oncology Centers Network (2013). A Physical Sciences Network Characterization of Non-tumorigenic and Metastatic Cells. Scientific Rep. 3 (1), 1449. doi:10.1038/srep01449

CrossRef Full Text | Google Scholar

Tlili, S., Gauquelin, E., Li, B., Cardoso, O., Ladoux, B., Delanoë-Ayari, H., et al. (2018). Collective Cell Migration without Proliferation: Density Determines Cell Velocity and Wave Velocity. R. Soc. Open Sci. 5 (5), 172421. doi:10.1098/rsos.172421

PubMed Abstract | CrossRef Full Text | Google Scholar

Tran, K. A., Kraus, E., Clark, A. T., Bennett, A., Pogoda, K., Cheng, X., et al. (2021). Dynamic Tuning of Viscoelastic Hydrogels with Carbonyl Iron Microparticles Reveals the Rapid Response of Cells to Three-Dimensional Substrate Mechanics. ACS Appl. Mater. Inter. 13 (18), 20947–20959. doi:10.1021/acsami.0c21868

CrossRef Full Text | Google Scholar

Trappmann, B., and Chen, C. S. (2013). How Cells Sense Extracellular Matrix Stiffness: A Material’s Perspective. Curr. Opin. Biotechnol. 24 (5), 948–953. doi:10.1016/j.copbio.2013.03.020

PubMed Abstract | CrossRef Full Text | Google Scholar

Trappmann, B., Gautrot, J. E., Connelly, J. T., Strange, D. G. T., Li, Y., Oyen, M. L., et al. (2012). Extracellular-matrix Tethering Regulates Stem-Cell Fate. Nat. Mater. 11 (7), 642–649. doi:10.1038/nmat3339

PubMed Abstract | CrossRef Full Text | Google Scholar

Trichet, L., Le Digabel, J., Hawkins, R. J., Vedula, S. R. K., Gupta, M., Ribrault, C., et al. (2012). Evidence of a Large-Scale Mechanosensing Mechanism for Cellular Adaptation to Substrate Stiffness. Proc. Natl. Acad. Sci. 109 (18), 6933–6938. doi:10.1073/pnas.1117810109

PubMed Abstract | CrossRef Full Text | Google Scholar

Truesdell, C., Noll, W., and Antman, S. S. (2004). The Non-linear Field Theories of Mechanics. 3rd ed. Springer.

Google Scholar

Vader, D., Kabla, A., Weitz, D., and Mahadevan, L. (2009). Strain-Induced Alignment in Collagen Gels. PLoS ONE 4 (6), e5902. doi:10.1371/journal.pone.0005902

PubMed Abstract | CrossRef Full Text | Google Scholar

Van Liedekerke, P., Palm, M. M., Jagiella, N., and Drasdo, D. (2015). Simulating Tissue Mechanics with Agent-Based Models: Concepts, Perspectives and Some Novel Results. Comput. Part. Mech. 2 (4), 401–444. doi:10.1007/s40571-015-0082-3

CrossRef Full Text | Google Scholar

Vandrangi, P., Gott, S. C., Kozaka, R., Rodgers, V. G. J., and Rao, M. P. (2014). Comparative Endothelial Cell Response on Topographically Patterned Titanium and Silicon Substrates with Micrometer to Sub-micrometer Feature Sizes. PLoS ONE 9 (10), e111465. doi:10.1371/journal.pone.0111465

PubMed Abstract | CrossRef Full Text | Google Scholar

Vedula, S. R. K., Leong, M. C., Lai, T. L., Hersen, P., Kabla, A. J., Lim, C. T., et al. (2012). Emerging Modes of Collective Cell Migration Induced by Geometrical Constraints. Proc. Natl. Acad. Sci. 109 (32), 12974–12979. doi:10.1073/pnas.1119313109

PubMed Abstract | CrossRef Full Text | Google Scholar

Verdier, C., Etienne, J., Duperray, A., and Preziosi, L. (2009). Review: Rheological Properties of Biological Materials. Comptes Rendus Physique 10 (8), 790–811. doi:10.1016/j.crhy.2009.10.003

CrossRef Full Text | Google Scholar

Vining, K. H., Stafford, A., and Mooney, D. J. (2019). Sequential Modes of Crosslinking Tune Viscoelasticity of Cell-Instructive Hydrogels. Biomaterials 188, 187–197. doi:10.1016/j.biomaterials.2018.10.013

PubMed Abstract | CrossRef Full Text | Google Scholar

Vogelstein, B., and Kinzler, K. W. (2004). Cancer Genes and the Pathways They Control. Nat. Med. 10 (8), 789–799. doi:10.1038/nm1087

PubMed Abstract | CrossRef Full Text | Google Scholar

Vroomans, R. M. A., Hogeweg, P., and ten Tusscher, K. H. W. J. (2015). Segment-Specific Adhesion as a Driver of Convergent Extension. PLOS Comput. Biol. 11 (2), e1004092. doi:10.1371/journal.pcbi.1004092

PubMed Abstract | CrossRef Full Text | Google Scholar

Walcott, S., Kim, D.-H., Wirtz, D., and Sun, S. X. (2011). Nucleation and Decay Initiation Are the Stiffness-Sensitive Phases of Focal Adhesion Maturation. Biophysical J. 101 (12), 2919–2928. doi:10.1016/j.bpj.2011.11.010

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, B., Lançon, P., Bienvenu, C., Vierling, P., Di Giorgio, C., and Bossis, G. (2013). A General Approach for the Microrheology of Cancer Cells by Atomic Force Microscopy. Micron 44, 287–297. doi:10.1016/j.micron.2012.07.006

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, H.-B., Dembo, M., and Wang, Y.-L. (2000). Substrate Flexibility Regulates Growth and Apoptosis of normal but Not Transformed Cells. Am. J. Physiology-Cell Physiol. 279 (5), C1345–C1350. doi:10.1152/ajpcell.2000.279.5.C1345

CrossRef Full Text | Google Scholar

Wang, H., Abhilash, A. S., Chen, C. S., Wells, R. G., and Shenoy, V. B. (2014a). Long-Range Force Transmission in Fibrous Matrices Enabled by Tension-Driven Alignment of Fibers. Biophysical J. 107 (11), 2592–2603. doi:10.1016/j.bpj.2014.09.044

CrossRef Full Text | Google Scholar

Wang, J., and Li, L. (2015). Coupled Elasticity–Diffusion Model for the Effects of Cytoskeleton Deformation on Cellular Uptake of Cylindrical Nanoparticles. J. R. Soc. Interf. 12 (102), 20141023. doi:10.1098/rsif.2014.1023

CrossRef Full Text | Google Scholar

Wang, J., Li, L., and Zhou, Y. (2014b). Creep Effect on Cellular Uptake of Viral Particles. Chin. Sci. Bull. 59 (19), 2277–2281. doi:10.1007/s11434-014-0207-8

CrossRef Full Text | Google Scholar

Wang, Y., and Zocchi, G. (2011). Viscoelastic Transition and Yield Strain of the Folded Protein. PLoS ONE 6 (12), e28097. doi:10.1371/journal.pone.0028097

PubMed Abstract | CrossRef Full Text | Google Scholar

Wei, Z., Deshpande, V. S., McMeeking, R. M., and Evans, A. G. (2008). Analysis and Interpretation of Stress Fiber Organization in Cells Subject to Cyclic Stretch. J. Biomechanical Eng. 130 (3), 031009. doi:10.1115/1.2907745

CrossRef Full Text | Google Scholar

Wen, Q., and Janmey, P. A. (2013). Effects of Non-linearity on Cell–ECM Interactions. Exp. Cell Res. 319 (16), 2481–2489. doi:10.1016/j.yexcr.2013.05.017

PubMed Abstract | CrossRef Full Text | Google Scholar

Wen, Q., and Janmey, P. A. (2011). Polymer Physics of the Cytoskeleton. Curr. Opin. Solid State. Mater. Sci. 15 (5), 177–182. doi:10.1016/j.cossms.2011.05.002

PubMed Abstract | CrossRef Full Text | Google Scholar

Wirtz, D., Konstantopoulos, K., and Searson, P. C. (2011). The Physics of Cancer: The Role of Physical Interactions and Mechanical Forces in Metastasis. Nat. Rev. Cancer 11 (7), 512–522. doi:10.1038/nrc3080

PubMed Abstract | CrossRef Full Text | Google Scholar

Wisitsorasak, A., and Wolynes, P. G. (2017). Dynamical Theory of Shear Bands in Structural Glasses. Proc. Natl. Acad. Sci. 114 (6), 1287–1292. doi:10.1073/pnas.1620399114

PubMed Abstract | CrossRef Full Text | Google Scholar

Wojcieszyn, J. W., Schlegel, R. A., Wu, E. S., and Jacobson, K. A. (1981). Diffusion of Injected Macromolecules within the Cytoplasm of Living Cells. Proc. Natl. Acad. Sci. 78 (7), 4407–4410. doi:10.1073/pnas.78.7.4407

PubMed Abstract | CrossRef Full Text | Google Scholar

Woods, K., Thigpen, C., Wang, J. P., Park, H., and Hielscher, A. (2017). Mechanically Tuned 3 Dimensional Hydrogels Support Human Mammary Fibroblast Growth and Viability. BMC Cell Biol. 18 (1), 35. doi:10.1186/s12860-017-0151-y

PubMed Abstract | CrossRef Full Text | Google Scholar

Wrighton, P. J., and Kiessling, L. L. (2015). Forces of Change: Mechanics Underlying Formation of Functional 3D Organ Buds. Cell Stem Cell 16 (5), 453–454. doi:10.1016/j.stem.2015.04.018

PubMed Abstract | CrossRef Full Text | Google Scholar

Xu, Q., and Engquist, B. (2018). A Mathematical Model for Fitting and Predicting Relaxation Modulus and Simulating Viscoelastic Responses. Proc. R. Soc. A: Math. Phys. Eng. Sci. 474 (2213), 20170540. doi:10.1098/rspa.2017.0540

PubMed Abstract | CrossRef Full Text | Google Scholar

Xu, Q., Engquist, B., Solaimanian, M., and Yan, K. (2020). A New Nonlinear Viscoelastic Model and Mathematical Solution of Solids for Improving Prediction Accuracy. Scientific Rep. 10 (1), 2202. doi:10.1038/s41598-020-58240-y

CrossRef Full Text | Google Scholar

Xu, Q., and Solaimanian, M. (2009). Modelling Linear Viscoelastic Properties of Asphalt concrete by the Huet–Sayegh Model. Int. J. Pavement Eng. 10 (6), 401–422. doi:10.1080/10298430802524784

CrossRef Full Text | Google Scholar

Xu, W., Mezencev, R., Kim, B., Wang, L., McDonald, J., and Sulchek, T. (2012). Cell Stiffness Is a Biomarker of the Metastatic Potential of Ovarian Cancer Cells. PLoS ONE 7 (10), e46609. doi:10.1371/journal.pone.0046609

PubMed Abstract | CrossRef Full Text | Google Scholar

Xue, B., Tang, D., Wu, X., Xu, Z., Gu, J., Han, Y., et al. (2021). Engineering Hydrogels with Homogeneous Mechanical Properties for Controlling Stem Cell Lineage Specification. Proc. Natl. Acad. Sci. 118 (37), e2110961118. doi:10.1073/pnas.2110961118

PubMed Abstract | CrossRef Full Text | Google Scholar

Yang, B., Wei, K., Loebel, C., Zhang, K., Feng, Q., Li, R., et al. (2021). Enhanced Mechanosensing of Cells in Synthetic 3D Matrix with Controlled Biophysical Dynamics. Nat. Commun. 12 (1), 3514. doi:10.1038/s41467-021-23120-0

PubMed Abstract | CrossRef Full Text | Google Scholar

Yang, Y., Chen, B., Meng, S., Li, S., and Yang, X. (2019). “Electrical and Viscoelastic Measurement of Cancer Cells in Epithelial-Mesenchymal Transition Process on a Microfluidic Device,” in 2019 8th International Symposium on Next Generation Electronics (ISNE), 1–3. doi:10.1109/ISNE.2019.8896395

CrossRef Full Text | Google Scholar

Zakim, D., Kavecansky, J., and Scarlata, S. (1992). Are Membrane Enzymes Regulated by the Viscosity of the Membrane Environment. Biochemistry 31 (46), 11589–11594. doi:10.1021/bi00161a043

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhang, W., Capilnasiu, A., and Nordsletten, D. (2021). Comparative Analysis of Nonlinear Viscoelastic Models across Common Biomechanical Experiments. J. Elasticity 145, 117–152. doi:10.1007/s10659-021-09827-7

CrossRef Full Text | Google Scholar

Zhao, X., Huebsch, N., Mooney, D. J., and Suo, Z. (2010). Stress-relaxation Behavior in Gels with Ionic and Covalent Crosslinks. J. Appl. Phys. 107 (6), 063509. doi:10.1063/1.3343265

CrossRef Full Text | Google Scholar