An Experimental Study on Scale Effect in Dynamic Shear Properties of High-Damping Rubber Bearings

High-damping rubber bearing (HDRB) is one of the most popular devices used for seismic isolation of structures. In order to clarify the mechanical characteristics of HDRB, various loading tests have been conducted on the bearings and obtained data have been applied to practical design of isolation systems. In this study, in order to investigate the scale effect on the physical characteristics of HDRB, dynamic loading tests were conducted with full scale and scaled model isolators, which have diameters of 1,000 and 225 mm, respectively. The test program covers shear strain dependence tests, frequency dependence tests, and repeated loading dependence tests. Special attention is paid in the differences of shear characteristics caused by the specimen scale. Repeated loading test was conducted only with a scaled model, and the relationship of temperature increase of the specimen and shear characteristics was evaluated. In parallel, finite element analysis (FEA) of the isolator under repeated loading was conducted. After the constitutive model of FEA was identified by the results, the FEA was extrapolated to simulate repeated loading of 1,000- and 1,600-mm-diameter isolators, which cannot be tested realistically by dynamic loading. Change of properties along the increasing number of cycles and temperature distribution of full scale and scaled down isolators were investigated. Necessity of consideration for the scale effect in the evaluation of HDRB properties by dynamic testing is discussed.

Introduction

Seismic isolation technology has gained popularity in the recent decades as one of the measures for seismic protection of structures (Murota, 2009; Nishi and Murota, 2012). Seismic isolation is an aseismic design concept to reduce the seismic force transmitted to the structure by supporting it with a flexible support member to elongate the natural period of the structure and thereby decouples it from the ground effects. Basically, seismic isolation systems provide functions of restoring force and energy dissipation. The seismic isolation bearing (SIB), made up with layers of alternating rubber and steel plates, is the most popular device for providing restoring force and damping characteristics. In addition to conventional SIB, innovation of new types of SIB has been progressed by many researchers (Losanno et al., 2019; Madera Sierra et al., 2019).

Many kinds of SIB testing, such as shear strain dependence, temperature dependence, frequency dependence, vertical loading force dependence (Tubaldi et al., 2016; Kalfas et al., 2017), aging effect (Hamaguchi et al., 2009), and ultimate properties (Nishi et al., 2019), have been conducted and useful data have been obtained for the design of the isolation systems. In order to evaluate realistic isolator characteristics, full scale isolator testing under dynamic loading is desired (Infanti et al., 2004; Yamamoto et al., 2012; Kato et al., 2014). However, in the case of dynamic loading of such specimens, a substantial capacity of hydraulic systems including the number of accumulators, high-level control devices, and high-precision measurement systems are required for the test setup. Therefore, full scale testing is generally conducted under quasi-static loading conditions, and rate-dependent characteristics are evaluated using a scaled model by a relatively small capacity dynamic testing machine. As an example, in ASCE 7–16, chapter 17 (American Society of Civil Engineers [ASCE], 2017), it is permitted to use a scaled model for the test of isolators that has rate-dependent properties, considering practical reasons caused by the limitation of the number of facilities, which provides sufficient capacity for dynamic tests of full scale specimens. In ISO 22762 “Elastomeric seismic-protection isolators” (ISO 22762, 2018a, b), the allowable scaling for each test type is specified. Basically, rubber material itself has frequency dependency in its restoring force characteristics. High-damping rubber bearing (HDRB) is one of the types of SIB that has relatively large frequency dependence in terms of shear properties. Generally, the rubber material of HDRB is filled with carbon or other ingredients, and interaction occurring between the polymer and the filler under stretching condition dissipates kinetic energy as heat builds up. Increasing temperature of rubber results in the decrease in shear stiffness and damping ratio. The thermal conductivity of rubber is much lower than that of metals, and the build-up heat in rubber radiates from the surface of SIB. Therefore, the accumulation of heat inside rubber is affected by the scale of length, which is considered as the main reason of scale effect on the physical properties of SIB.

In this study, firstly, the scale effect on the fundamental shear properties of HDRB is investigated by dynamic loading tests with two types of specimen: full scale and scaled-down specimens with a scale ratio of 1/4.44. Specimen diameters are 1,000 and 225 mm. The measured properties are shear strain dependence and frequency dependence. Secondly, dynamic repeated loading test with 200 cycles is conducted on isolator specimens with a diameter of 225 mm.

After the 2003 Tokachi-Oki earthquake, long-period and long duration ground motions have become one of the most highlighted topics in earthquake engineering in Japan. A long duration ground motion may continue for over 5 min. and in the case of a seismically isolated structure, isolation devices are subjected to vibration for a long period (Building Research Institute Japan, 2016). On this background, this study deals with the subject of scale effect on isolator characteristics under repeated cyclic loading. During the repeated loading, the properties of isolators are changed according to the increasing number of cycles. This property change firstly occurred by loading history (Tubaldi et al., 2017) for first several cycles, and then the increase in temperature is mostly influenced by the large number of cycles. During the tests, the surface temperature of the specimens is measured, and the relationship between the change of mechanical properties and the temperature according to the increase in the number of cycles is investigated. Additionally, finite element analyses (FEAs) are carried out under identical conditions with the tests, where parameter identification is conducted. After confirming the accuracy of the FEA model, the dynamic repeated loading characteristics with 1,000- and 1,600-mm-diameter isolators are investigated with this model and compared with the test results.

Dynamic Loading Test

Definition of Shear Properties

The shear characteristics, such as effective shear stiffness K_eq, equivalent shear modulus G_eq, equivalent damping ratio H_eq, and dissipated energy E_d, of HDRB are defined in Figure 1. K_eq is defined as the slope of the straight line from the point of (maximum load, maximum displacement) to the point of (minimum load, minimum displacement). Under sinusoidal loading, the force at maximum displacement has generally small difference from the maximum force. In the definition of the viscoelastic characteristics, the force at maximum displacement is generally used for the estimation of effective stiffness. However, the maximum displacement point, where the shear force drops sharply, is very difficult to identify and sometimes causes loss of accuracy in the calculation of characteristics when the time step for data acquisition is not precise enough. For this reason, effective stiffness is defined as mentioned above in this study. In frequency dependence test and shear strain dependence test, shear properties at the third cyclic loop are used for evaluation.

FIGURE 1

Figure 1. Definition of shear properties of HDR.

Test Specimens

The dimensional characteristics, performance specifications, and material properties of full scale and scaled-down models are shown in Table 1 and Figure 2. The full scale and scaled model isolators have consistency of important parameters such as unit rubber layer thickness, number of layers, and first and second shape factors. However, because of the manufacturing process, there are slight differences for each parameter, which are indicated in Table 1. For reinforcing plates and flanges, it is challenging to follow an exact scale. Furthermore, full scale isolator has an inner hole with a diameter of 25 mm for manufacturing purpose. The authors considered these differences in the scale as negligible in this study. Full scale and scaled model isolators are identified as scale-I and scale-II, respectively. Two different types of high-damping rubber materials are used in the specimens. They are identified as rubber-A and -B, which have equivalent shear modulus G_eq of 0.392 and 0.620 MPa at 100% shear strain, respectively. The equivalent damping ratio H_eq of both rubber materials is 24% at 100% shear strain. These two high-damping rubber materials had been improved in terms of load history dependence. A stress softening behavior, known as the Mullins effect, is improved compared with conventional high-damping rubber materials (Murota et al., 2007).

TABLE 1

Table 1. Dimensional characteristics and material properties of test specimens.

FIGURE 2

Figure 2. Test specimens.

Each test specimen is named according to size, rubber material, and specimen no. as follows: [size: I or II]-[rubber: A or B]-[No.: 1, 2, or 3].

Shear strain γ is defined as X_max/h_r, where X_max is the maximum horizontal displacement at a cycle of shear loading and h_r is the total rubber height in an isolator unit. The shear strain of 100% corresponds to the shear displacement equivalent to the total rubber height. Each test specimen was manufactured from a different lot of rubber material and vulcanization conditions. Therefore, the variation of the modulus of used rubber material and the cure state of rubber by vulcanization may affect the shear properties of isolators. Especially, the cure state of scale-I and scale-II may have significant difference in network structures of polymer, sulfur, and fillers. This may be also considered as a scale effect.

Test Facility

The dynamic loading tests of the scale-I specimen were carried out by “Seismic Response Modification Device (SRMD) Test Machine” (Seible et al., 2000) in the University of California at San Diego. The loading plate of the test device, where the isolator is installed, slides over low-friction hydrostatic bearings. The generated friction force during dynamic loading was deducted from the raw data during data analysis. The friction force was measured by the UCSD laboratory in advance. The inertia force is calculated by acceleration and weight of mobile parts of the test device and was also deducted from the raw data. All test procedures were operated by the staff of the SRMD test facility in the UCSD.

The tests of scale-II were carried out by dynamic loading test machine in Bridgestone Corporation Technical Center, Yokohama, Japan. The maximum vertical load is 1,000 kN, and the maximum horizontal load and maximum strokes of the horizontal actuator is 200 kN and ± 0.3 m, respectively. The load cell, for measurement of shear and compression load, was installed just beneath the specimen. Therefore, the shear force obtained by the load cell does not include the friction force generated in the slide guide of the test machine. The inertia force is included but it is considered as negligibly small.

Test Conditions

Correspondence between the specimen number and the test type is shown in Table 2 along with test frequency and no. of cycles incorporated. Benchmark test was conducted to investigate the fundamental performance of isolators at a shear strain of 100% under a compressive stress of 13 MPa for material A and 15 MPa for material B, which is the test condition of the isolator used for determining the nominal shear modulus and the damping ratio by the manufacturer (Table 2). In frequency dependence tests, the benchmark tests were conducted repeatedly between each test at specific frequency in order to evaluate the influence of loading history on the results, which should be properly considered at evaluation. The change of the properties measured by the benchmark tests is an indicator of the fatigue by numerous loadings to the specimen. Ambient temperature during the testing of the scaled model was controlled at 20 ± 5 degree Celsius. The temperature of scale-I in the UCSD was not controlled, but the measured temperature ranges between 20 and 26 degrees Celsius during the test. Therefore, the authors consider the influence of ambient temperature to the properties as insignificant and negligible.

TABLE 2

Table 2. Summary of test conditions.

In frequency dependence test, the shear strain is 100%, the wave form is sinusoidal, and the number of cycles is three. A frequency of 0.33 Hz is considered as the standard vibration frequency in this study. The series of frequency is 0.01, 0.033, 0.1, and 0.3 Hz. Benchmark test was conducted between each test at a specific frequency as prescribed. In this study, frequency dependence is defined as the ratio of G_eq, H_eq, and E_d at each frequency level to those of 0.33-Hz frequency.

In the shear strain dependence test, its series is 10, 25, 50, 100, 150, 200, and 270%. The test frequency is fixed as 0.33 Hz, and the wave form is sinusoidal. The benchmark test is conducted at the beginning and end of the test series. In this study, shear strain dependence is defined as the ratio of G_eq, H_eq, and E_d at each shear strain to those of 100% shear strain.

In the repeated loading test, the specimen was subjected to a compressive load according to the corresponding nominal stress of 13 and 15 MPa for materials A and B, respectively. Under the controlled compressive load, the cyclic loading was conducted in the shear direction for a shear strain of 200%, with a frequency of 0.33 Hz. The total number of cycles was 200. The cumulative displacement was 72 m, which corresponds to 320 m for a full scale isolator unit assuming a total rubber height of 200 mm. The ratio of shear properties, G_eq and H_eq, at each cycle was computed by normalizing each value with that of the third cyclic loading. During testing, the surface temperature of the specimen was measured by a radiation thermometer.

Verification of Initial Properties of Test Specimens

Initial properties of test specimens were evaluated by the first set of benchmark tests. Comparing the nominal value of each property, all results were within the range of ± 20%, concluding that all test specimens were properly manufactured within designated margins. In the tests, scale-I specimens gave higher stiffness and a lower damping ratio than those of scale-II specimens. Figure 3 shows a comparison of the shear stress-strain relationship of I-A-1 and II-A-1, I-B-1, and I-B-1, for all three cycles. The factors that have possible influence on the difference in properties between scale-I and -II are the difference in the rubber material lot, vulcanization conditions, and the test device. Here, the difference in stiffness may be caused by the rubber material. The difference in the dissipated energy is quite small between scale-I and scale-II specimens, while the difference in stiffness is larger, which is attributed to the difference of the material lot and/or vulcanization conditions.

FIGURE 3

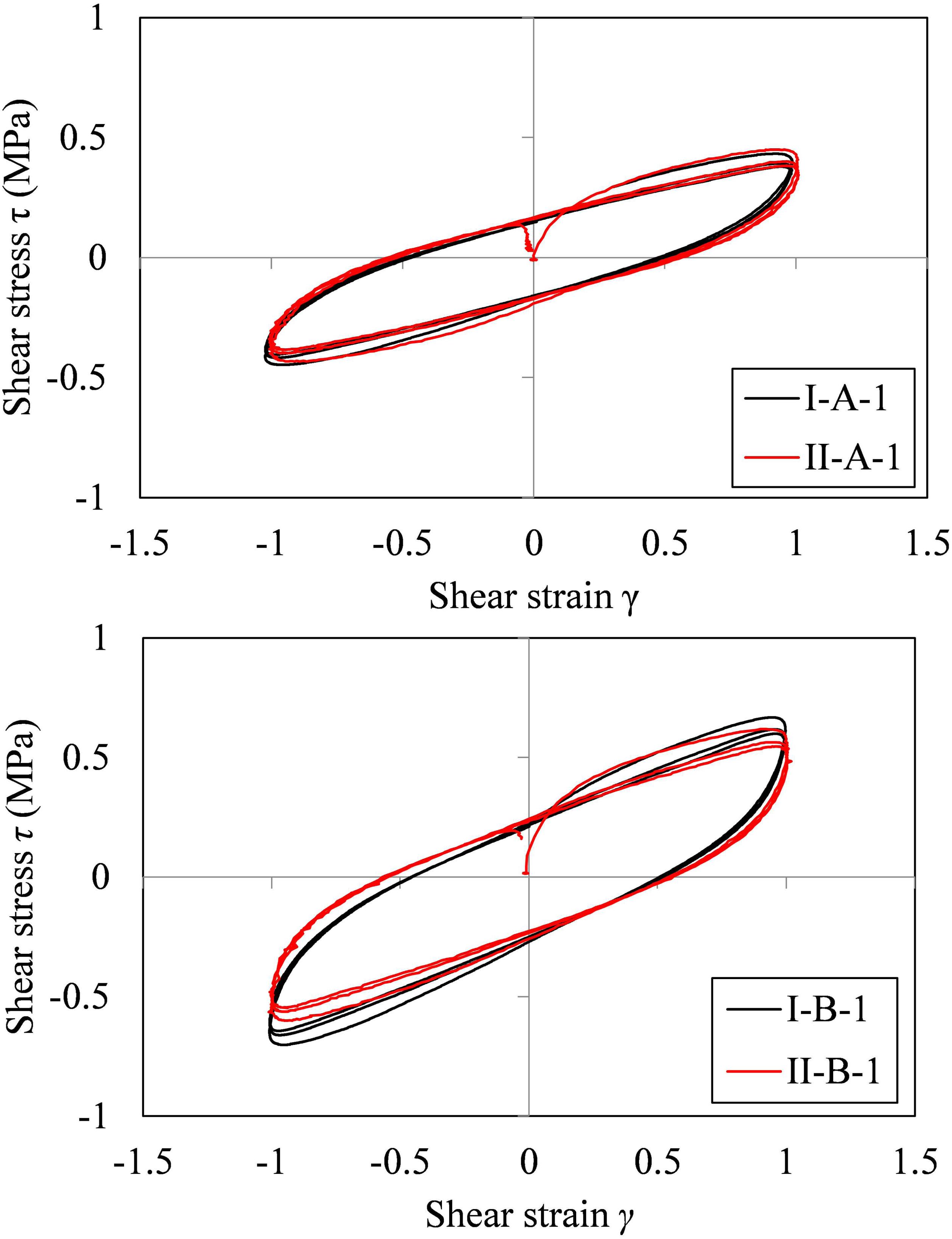
Figure 3. Shear stress-strain relationship of scale-I and-II of rubber-A and -B for all three cycles.

Although there is some difference in measured properties between scale-I and -II specimens, all specimens satisfy the standard deviation, ± 15% of the design value, which is generally considered as the acceptance criterion of isolators in practical use.

Results of Frequency Dependence and Shear Strain Dependence Tests

The comparison of shear stress–strain relationships under frequency of 0.01, 0.033, 0.1, and 0.33Hz for scale-I and -II with rubber-A and -B at the third cycle is shown in Figure 4. Shear properties, G_eq, H_eq, and E_d at each frequency, normalized by the value at 0.33 Hz are shown in Figure 5. As it was expected, the shear modulus becomes higher for increasing values of the frequency. The shear modulus at 0.01-Hz frequency, which is considered as quasi-static, is more than 25% lower than that at the 0.33-Hz level. The damping ratio and dissipated energy values also show similar tendency. The difference in the frequency dependence between scale-I and -II is relatively small. Especially, for E_d, results almost agree with each other. These results indicate that the test results of the scale-I isolator conducted by quasi-static test conditions can be corrected to the results of dynamic test conditions by applying frequency dependence obtained by dynamic test of the scaled model.

FIGURE 4

Figure 4. Shear stress-strain relationship of scale-I and -II in frequency dependence test at 3rd cycle.

FIGURE 5

Figure 5. Comparison of shear properties in frequency dependency of-Scale-I and -II at 3rd cycle.

Figure 6 shows the transition of each property in benchmark tests conducted before each test at each frequency. The first benchmark test [BT(1), number in () : set number of the test] is conducted for verification of the initial performance of the specimens as prescribed in 2.5. The total number of sets was 5, and their sequence is

FIGURE 6

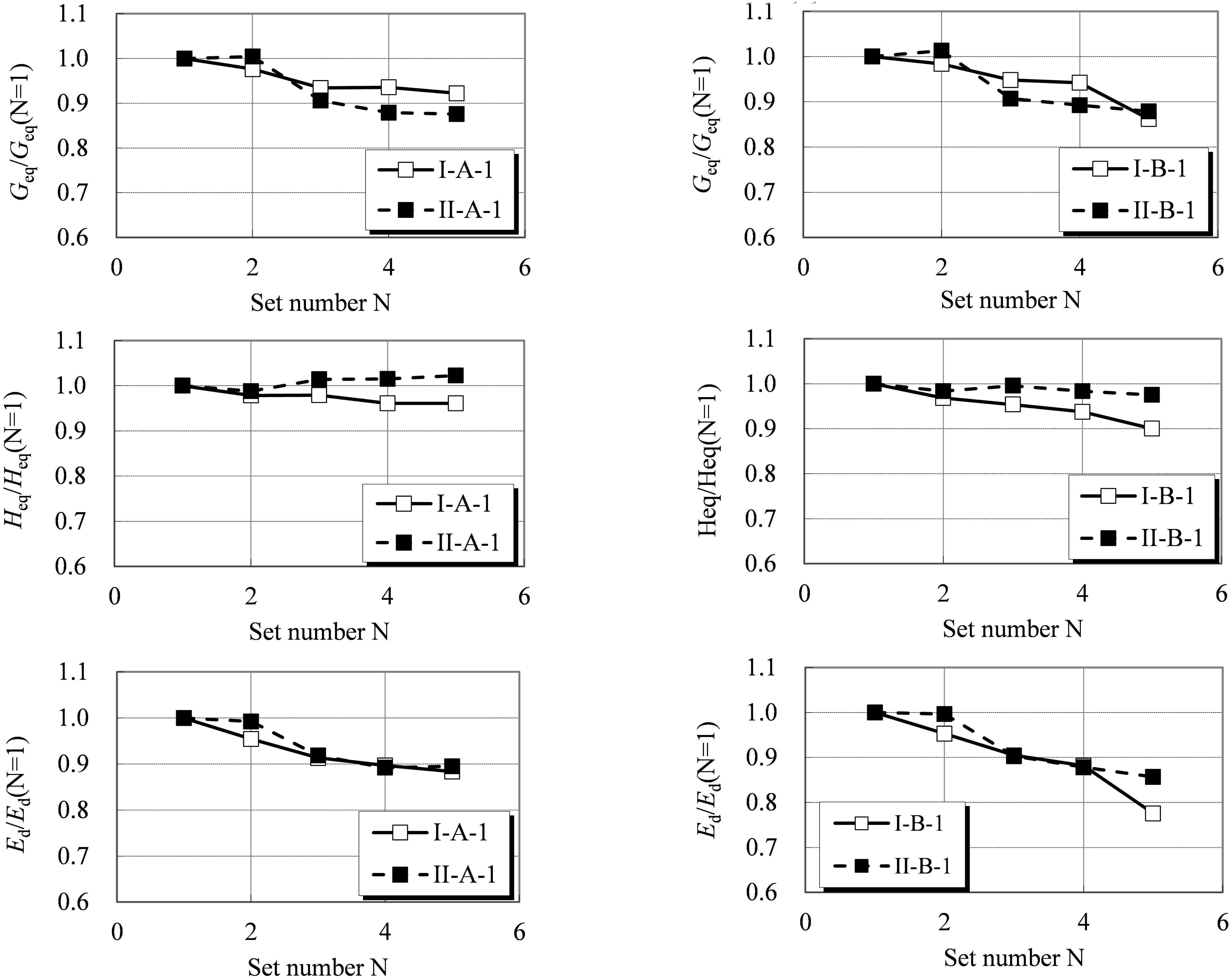
Figure 6. Change of characteristics in benchmark test [BT(1) to BT(5)] of scale-I and -II at 3rd cvcle.

$$\begin{array}{c}\mathrm{BT}(1)\to \mathrm{BT}(2)\to 0.01\mathrm{Hz\; test}\to \mathrm{BT}(3)\to 0.033\mathrm{Hz}\to \mathrm{BT}(4)\hfill \\ \to 0.1\mathrm{Hz\; test}\to 0.33\mathrm{Hz\; test}(=\mathrm{BT}(5))\hfill \end{array}$$

The absolute value of each BT was normalized by the value at BT(1). All properties in each test specimen decrease as the number of sets increases. Also, it can be observed that scale-II shows more reduction of stiffness and constant reduction in dissipated energy. However, correlation with scaling is not obvious. The change ratio varies approximately between −10 and −20%. The results indicate that during the prototype test of isolators, effects of fatigue by accumulated loading should be adequately considered when making judgments according to design criteria. It is suggested that when the frequency dependence test will be conducted, fatigue effect should be measured by the benchmark test between each test as conducted in this study, and adequate correction of the results should be carried out such as deduction of the decreased amount of properties by fatigue from the results.

The shear stress–strain relationships for all cycles and each property normalized by the value at a shear strain of 100% for both rubber-A and -B with scale-I and II at the third cycle of each shear strain are shown in Figures 7, 8. Significant difference in the absolute value of shear stress is observed in the shear stress–strain relationship for scale-I and II, which is shown in Figure 7. The authors consider that the difference in stress is caused by the variation of rubber material and vulcanization process in scale-I and II specimens. However, it is noteworthy that the normalized value shows good agreement in scale-I and -II as indicated in Figure 8. Deviation in shear modulus, ± 10 to 20% for example, does not affect the shear strain dependence. This result suggests that the shear strain dependence can be effectively evaluated using scaled models instead of full scale similar to frequency dependence.

FIGURE 7

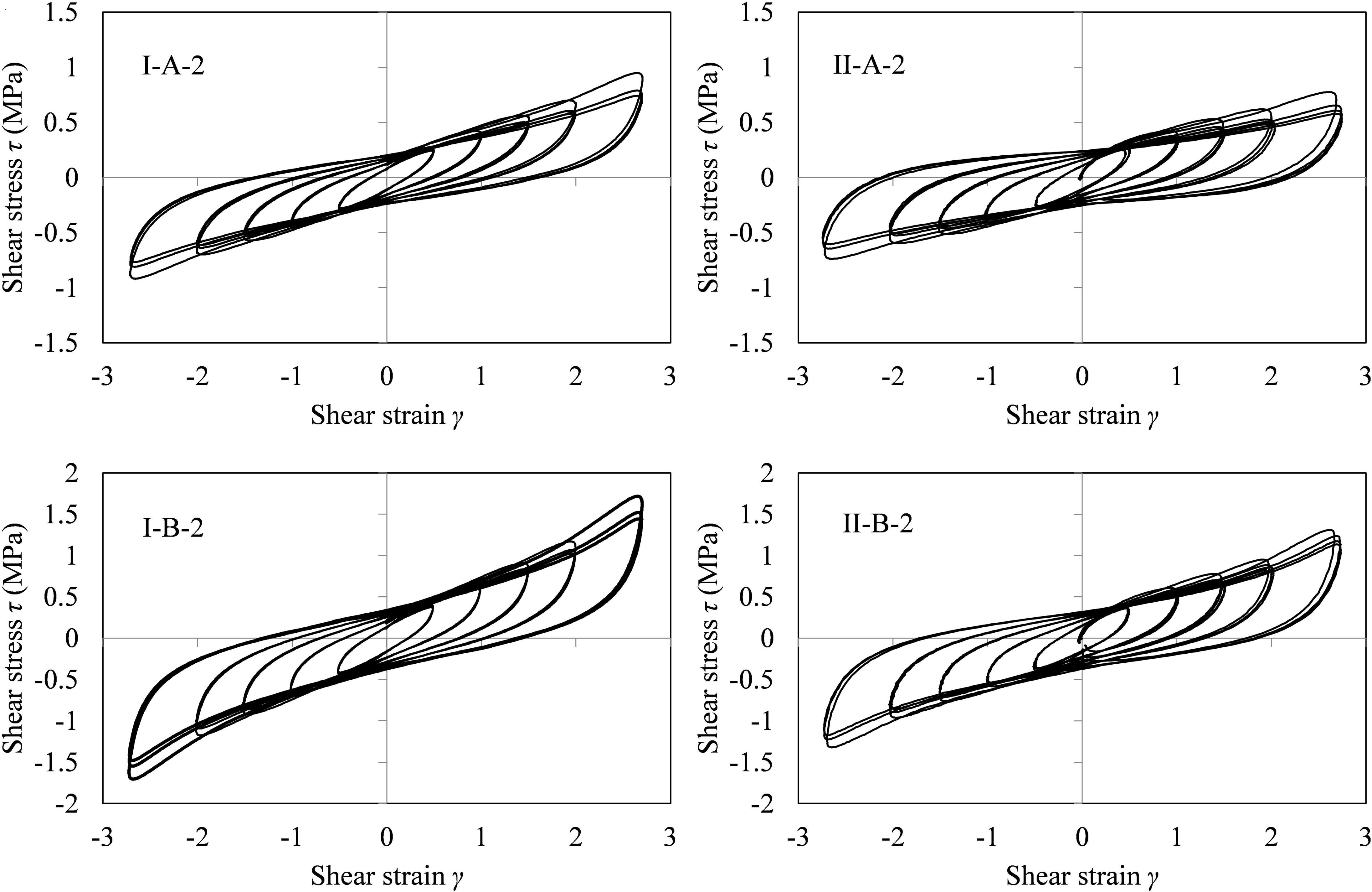
Figure 7. Comparison of shear stress-strain relationship in shear strain dependence test of scale-I and -II for all three cycles.

FIGURE 8

Figure 8. Comparison of shear strain dependency of scale-I and -II at 3rd cycle.

The results in frequency and shear strain dependence tests show that even when there is significant difference in the absolute value of shear properties in scale-I and -II, the normalized trends with frequency and shear strain amplitude were not affected by the scale. In both tests, loading was conducted in three cycles. The temperature on the rubber surface on scale-I isolators increased only 4 to 5 degrees Celsius at the end of the test compared to the initial condition. Benchmark test results indicate effective stiffness and dissipated energy decrease as loading experience increases. Appropriate consideration should be made in the evaluation of the results in continuous sets of loading.

Results of Repeated Loading Test

Figure 9 shows the shear force–displacement relationship of each specimen in repeated loading tests for the entire cyclic loading protocol. It is observed that the shear force and E_d are decreased by the increased number of cycles. The change of G_eq, H_eq, and E_d under repeated loading is indicated in Figure 10. The change of properties by repeated loading is considered as a combination of fatigue and temperature effect. For the first few cycles, fatigue effect, also called as “Mullins’s effect” (Mullins, 1969) is dominant in the change of properties. After a few cycles, properties are majorly affected by temperature increase. At the 200th cycle, G_eq decreased for approximately 40% in II-A-3 and 30% in II-B-3. There is no significant difference in the change ratio of G_eq and E_d for both rubber-A and -B. The increase in the surface temperature along the increase in the number of loading cycle, and the relationship between volumetric E_d of rubber in specimens (V_r) and surface temperature for both rubber materials are shown in Figure 11. The temperature increases from 20 degrees to a maximum of 80 degrees Celsius in the case of specimen B, which has higher shear modulus and energy dissipation. The result indicates that the specific heat capacities of rubber-A and -B have no significant difference. Therefore, the difference of temperature increase in A and B is simply caused by the difference in accumulated energy dissipation during repeated loading.

FIGURE 9

Figure 9. Shear stress-strain relationship of scale- II-A and -II-B under repeated loading for shear strain 200% × 200 cycles.

FIGURE 10

Figure 10. Relationship of change of properties and number of cycles in scale-II-A and -II-B.

FIGURE 11

Figure 11. Change of temperature by increase of loading cycle and E_d/V_r in scale-II-A, and -II-B.

Finite Element Analysis for Evaluation of Scale Effect in Repeated Loading

As repeated loading tests on full scale specimens are quite challenging in existing test machines, FEA was conducted to predict the scale effect on the repeated loading by extrapolation to a large size isolator test case. FEA code named as the “Deformation History Integral Type (DHI)” model (Mori et al., 2012; Masaki et al., 2017) was implemented for the study. The function of the code is a heat-mechanics coupled analysis, which consists of hyper elastic stress–strain analysis and heat-transfer analysis. The constitutive law of the mathematical model (Mori et al., 2012) involves parameters for temperature affect and fatigue affect. Using both parameters, the properties of isolators during repeated loading are reproduced. The conceptual flow of the heat-mechanics coupled FEA and the comparison of the test results and FEA results for scaled model II-B-3 are shown in Figure 12.

FIGURE 12

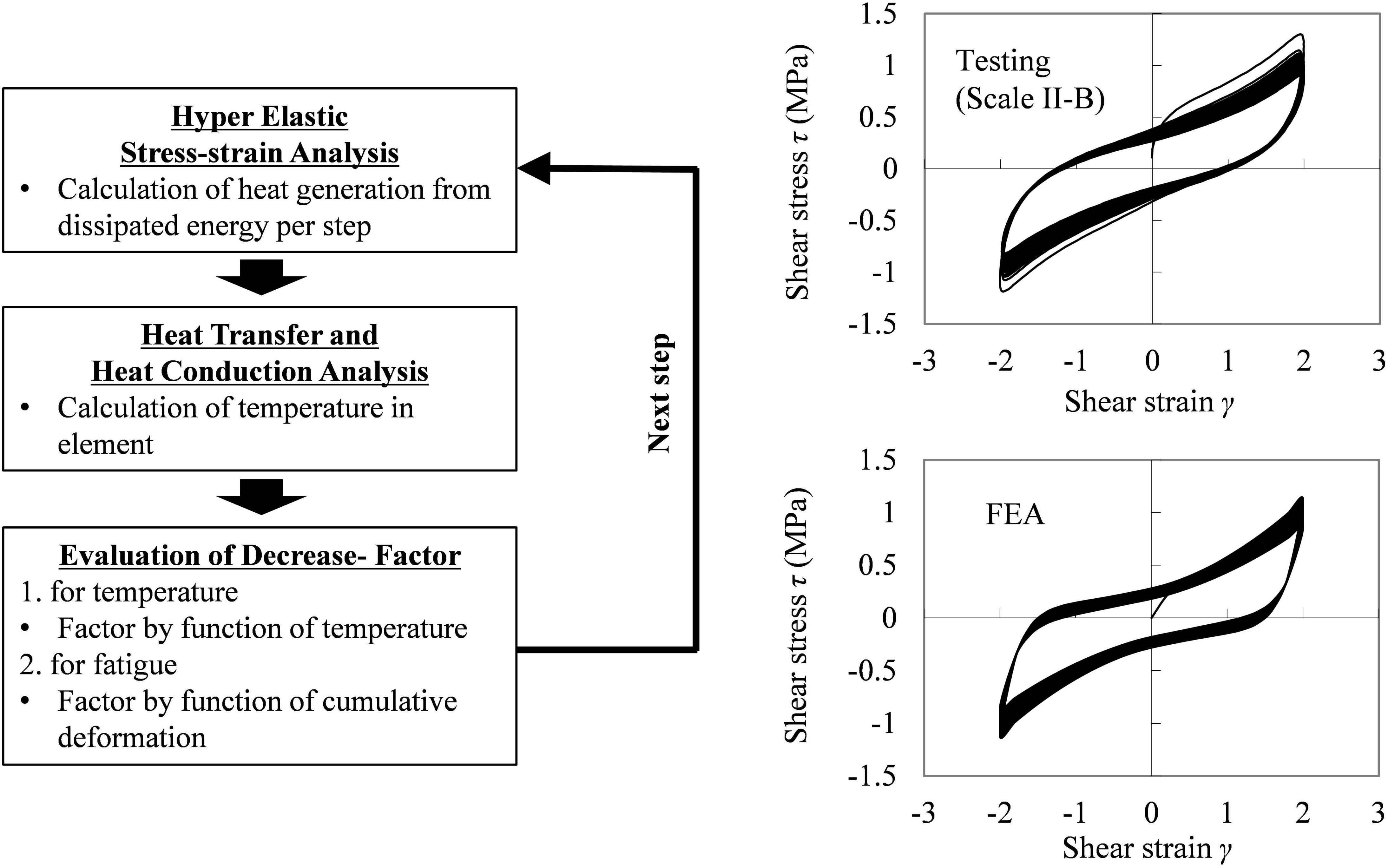
Figure 12. Concept of heat-mechanics coupled FEA and comparison of shear stress-strain relationship of Testing (Scale II-B) and FEA results.

Heat transfer boundary is set surrounded by isolators, and the coefficient of heat transfer was identified so as to agree with the surface temperature of the model. As explained in a previous publication (Mori et al., 2012), parameters were identified by the results of scaled models and shear-block specimens. Firstly, the parameters for temperature effect on the change of properties were identified by the test conducted with shear-block specimen, which was conditioned in constant temperature under −10, 0, 10, 20, 30, and 40 degrees Celsius. The number of loading cycles was three, and the properties at the third cycle was measured and recorded. Property change in the temperature range over 40 degrees Celsius was assumed by extrapolation with curve fitting. Parameters related to fatigue were identified using data of property change obtained during repeated loading dependency tests with 200 cycles.

Using these parameters, the repeated loading for full scale isolators with a diameter of 1,000 mm, which was used in this study as specimen I-B, and 1,600-mm-diameter was simulated by an FEA model, and the results were compared with II-3. The analysis was conducted based on 50 cycles. The dimensional characteristics of 1,600-mm isolators are as follows:

• outer diameter = 1,600 mm, inner diameter = 80 mm

• thickness of unit rubber layer = 10.4 mm, number of lamination = 19, total rubber thickness = 198 mm

• thickness of reinforcing plate = 5.8 mm, rubber material = B.

Figure 13 shows a comparison of normalized analysis results for G_eq, H_eq, and E_d with respect to the number of loading cycles, where values are normalized according to the results of the third cycle. The temperature distribution in all three isolator models at the final step is also shown in Figure 13. The results show the scale effect on the dynamic characteristics under repeated loading, which was not observed in frequency and shear strain dependence tests conducted for the three cycles. Analyses have shown that the effect of increasing repeated cycles on the shear modulus is almost the same for each diameter. However, in terms of dissipated energy E_d, there are significant differences between scaled model II-B and the largest size of the 1,600-mm-diameter isolator as the number of cycles increases. Obviously, the difference comes from the different temperature levels inside isolators, as the heat generation by high-damping rubber is proportional to the cube of the size (volume), whereas heat dissipation from the surface of the isolator is proportional to the square of the size (surface area).

FIGURE 13

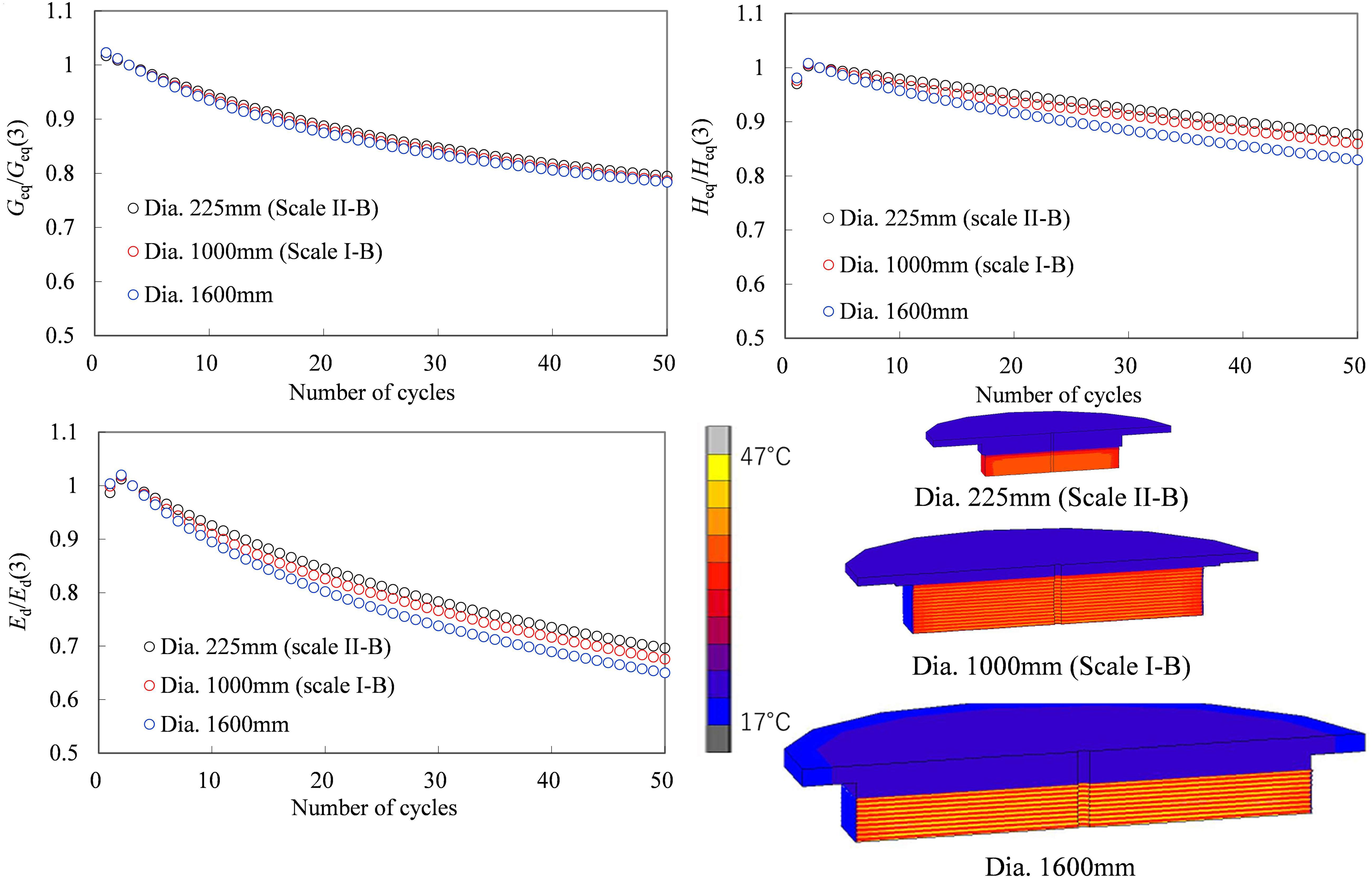
Figure 13. Comparison of change in G_eq, H_eq, and E_d of isolators with diameter of 225 mm (scale-II-B). 1000 mm (scale I-B), and 1600 mm in repeated loading by FEA.

Conclusion

The scale effect on the dynamic shear properties of HDRB was investigated by dynamic loading tests on full scale specimens with a diameter of 1,000 mm, and scaled model with 225 mm, using two different types of rubber material with soft and hard shear moduli.

Firstly, the nominal shear properties at 100% shear strain were measured and the manufacturing conformity of all specimens was verified. Then, dynamic loading tests of frequency and shear strain dependence were conducted. In both types of rubber materials, although there is some degree of difference between shear stress–strain relationship of full scale and scaled specimens, no significant difference was observed in neither dependences.

During the frequency dependence test, the change of fundamental properties was evaluated by the benchmark test, which was conducted between each test at a specific frequency. The stiffness and dissipated energy vales were decreased as the number of test cases increased. This fact suggests that when the continuous test is conducted, such as the prototype test of an actual project, the fatigue condition of the specimen should be appropriately considered in the evaluation of the results.

Next, repeated dynamic loading for 200 cycles of 200% shear strain with a scaled isolator specimen was conducted, and the relationship between the change of shear properties, temperature increase, and the number of loading cycles was investigated. As the number of cycles increases, the temperature of the isolator increased and stiffness and dissipated energy decreased.

The FEA model, which was developed in a previous study by authors for heat-mechanics coupled analysis, was implemented in order to investigate the cyclic characteristics of 1,000- and 1,600-mm-diameter isolators. The parameters in the constitutive law of the FEA was identified with test results of the scaled model with the diameter of 225 mm. The results show a significant difference in the change of shear properties by the number of repeated cycles. The results indicate that when the isolator is subjected to repeated loading over three cycles, the scale effect on shear properties is significant.

It is concluded that the scale effect on shear properties under a limited number of cycles, such as fewer than 10 cycles, can be neglected. It can be fed back to the practical case of the isolator test. In the prototype tests, if the frequency dependence is evaluated in advance, testing with a scaled model can be accepted. However, when long duration seismic input is considered, which has been a current issue since recent major earthquakes in Japan, the isolators may be subjected to an extreme repeated number of shear loading cycles. Considering these cases, the scale effect on the dynamic properties should be properly considered.

In this study, investigation of the scale effect is limited to the properties between 100 and 200% shear strain. The scale effect of HDRB isolators on ultimate properties such as shear breaking or buckling is under consideration as future subjects. Furthermore, investigation of the scale effect on other types of isolators, especially lead-core rubber bearing (LRB), is also considered in the next step. Energy dissipation is concentrated in the lead core where the thermal diffusion is considered to be affected by the dimension of the isolator.

Data Availability Statement

The datasets generated for this study are available on request to the corresponding author.

Author Contributions

All authors listed have made a substantial, direct and intellectual contribution to the work, and approved it for publication.

Conflict of Interest

NM and TM were employed by the company Bridgestone Corporation.

Acknowledgments

The authors would like to express great appreciation to all the members of the University of California at San Diego for their contribution to the dynamic loading test of full scale isolators.

References

American Society of Civil Engineers [ASCE], (2017). “Chapter 17 seismic design requirements for seismically isolated structures,” in Minimum Design Loads and Associated Criteria for Buildings and Other Structures American Society of Civil Engineering, Bombai: American Society of Civil Engineers.

Google Scholar

Building Research Institute Japan, (2016). “Study on repeated loading characteristics of seismic isolation devices and its influence to seismically isolated structures,” in Building Research Report, No.170, 2016.4, Japanese.

Google Scholar

Hamaguchi, H., Samejima, Y., and Kani, N. (2009). “A study of aging effect on rubber bearings after about twenty years in use,” in 11the World Conference on Seismic Isolation, Energy Dissipation and Active Vibration Control of Structures, Guangzou, 7–21.

Google Scholar

Infanti, S., Papanikolas, P., Benzoni, G., and Castellano, M. G. (2004). “Rion-antirion bridge: design and full scale testing of the seismic protection devices,” in 13th World Conference on Earthquake Engineering, Vancouver.

Google Scholar

Iso 22762, (2018a). “Elastomeric seismic-protection isolators,” in Part-1 Test methods International Organization for Standardization, Geneva: ISO.

Google Scholar

Iso 22762, (2018b). “Elastomeric seismic-protection isolators,” in Part-3 Applications of buildings - specifications, International Organization for Standardization, Geneva: ISO.

Google Scholar

Kalfas, K., Mitoulis, S., and Katakalos, K. (2017). Numerical study on the response of steel-laminated elastomeric bearings subjected to variable axial loads and development of local tensile stresses. Eng. Struct. Col. 134, 346–357. doi: 10.1016/j.engstruct.2016.12.015

CrossRef Full Text | Google Scholar

Kato, H., Akutsu, S., Mori, T., Nakamura, M., and Murota, N. (2014). “Development of large-scale elastomeric seismic isolation bearings for buildings,” in 5th Asia Conference on Earthquake Engineering, Taiwan.

Google Scholar

Losanno, D., Dierra, I. E., Spizzuoco, M., Marulanda, J., and Thomson, P. (2019). Experimental assessment and analytical modeling of novel fiber-reinforced isolators in unbounded configuration. Composize Struct. 212, 66–82. doi: 10.1016/j.compstruct.2019.01.026

CrossRef Full Text | Google Scholar

Madera Sierra, I. E., Losanno, D., Srano, S., Marulanda, J., and Thomson, P. (2019). Development and experimental behavior of HDR seismic isolators for low-rise residential buildings. Eng. Struct. 183, 894–906. doi: 10.1016/j.engstruct.2019.01.037

CrossRef Full Text | Google Scholar

Masaki, N., Mori, T., Murota, N., and Kasai, K. (2017). “Validation of hysteresis model of deformation-integral type of high damping rubber bearings,” in 16WCEE, proceedings of 12th World Conference of Earthquake Engineering, Santiago.

Google Scholar

Mori, T., Kato, H., and Murota, N. (2012). Modeling for analysis of high damping rubber bearings with effects of fatigue and temperature increase due to repeated deformation. Struct. Constr. Eng. 77, 1517–1526. doi: 10.3130/aijs.77.1517

CrossRef Full Text | Google Scholar

Mullins, L. (1969). Softening of rubber by deformation. Rubber Chem. Technol. 42, 339–362. doi: 10.5254/1.3539210

CrossRef Full Text | Google Scholar

Murota, N. (2009). Earthquake protection materials – reviews and future directions of elastomeric isolators. Soc. Polym. Sci. 58,

Google Scholar

Murota, N., Nishimura, K., Kikuchi, T., Okutsu, N., Kuwamoto, K., and Suzuki, S. (2007). “Development of new high-damping rubber bearings with improved material for load-history dependence,” in Summaries of Technical Papers of Annual Meeting Architectural Institute of Japan, Japanese, 879–880.

Google Scholar

Nishi, T., and Murota, N. (2012). Elastomeric seismic-protection isolators for buildings and bridges. Chin. J. Polym. Sci. 31, 50–57.

Google Scholar

Nishi, T., Suzuki, S., Aoki, M., Sawada, T., and Fukuda, S. (2019). International investigation of shear displacement capacity of various elastomeric seismic-protection isolators for buildings. J. Rubber Res. 22, 33–41. doi: 10.1007/s42464-019-00006-x

CrossRef Full Text | Google Scholar

Seible, F., Benzoni, G., Filiatrault, A., Post, T., and Mellon, D. (2000). “Performance validation of large seismic response modification devices,” in Proceedings of 12th World Conference of Earthquake Engineering, Auckland.

Google Scholar

Tubaldi, E., Mitoulis, S., Ahmadi, H., and Muhr, A. (2016). A parametric study on the axial behaviour of elastomeric isolators in multi-span bridges subjected to horizontal excitation. Bull. Earthqu. Eng. 14, 1285–1310. doi: 10.1007/s10518-016-9876-9

CrossRef Full Text | Google Scholar

Tubaldi, E., Ragni, L., Dall’Asta, A., Ahmadi, H., and Muhr, A. (2017). stress softening behavior of HDNR bearings: modelling and influence on the seismic response of isolated structures. Earthq. Eng. Struct. Dyn. 46, 2033–2054.

Google Scholar

Yamamoto, M., Minewaki, S., Yoneda, H., and Higashino, M. (2012). Nonlinear behavior of high-damping rubber bearings under horizontal bidirectional loading: full scale tests and analytical modeling. Earthq. Eng. Struct. Dyn. 41, 1845–1860. doi: 10.1002/eqe.2161

CrossRef Full Text | Google Scholar