TDHF Theory and Its Extensions for the Multinucleon Transfer Reaction: A Mini Review

Sekizawa, Kazuyuki

doi:10.3389/fphy.2019.00020

MINI REVIEW article

Front. Phys., 12 March 2019

Sec. Nuclear Physics

Volume 7 - 2019 | https://doi.org/10.3389/fphy.2019.00020

This article is part of the Research Topic Structure, Reaction and Production Mechanism of Extremely Neutron-Rich Nuclei View all 4 articles

TDHF Theory and Its Extensions for the Multinucleon Transfer Reaction: A Mini Review

$\r\nKazuyuki Sekizawa*$ Kazuyuki Sekizawa^*

Center for Transdisciplinary Research, Institute for Research Promotion, Niigata University, Niigata, Japan

Time-dependent Hartree-Fock (TDHF) theory has been a powerful tool in describing a variety of complex nuclear dynamics microscopically without empirical parameters. In this contribution, recent advances in nuclear dynamics studies employing TDHF and its extensions are briefly reviewed, in line with the study of multinucleon transfer (MNT) reactions. The latter lies at the core of this Research Topic, whose application for the production of extremely neutron-rich nuclei has been extensively discussed in recent years. Having in mind the ongoing theoretical developments, it is envisaged how microscopic theories may contribute to the future MNT study.

1. Introduction

Multinucleon transfer (MNT) reactions may be a promising means to produce yet-unknown, neutron-rich unstable nuclei, whose production is difficult by other methods. This possibility makes the study of MNT both fascinating and important. For instance, besides the fundamental interest in nuclear structures such as shell evolution [1] and shape transitions [2, 3], properties of extremely neutron-rich nuclei are crucial to fully understanding the detailed scenario of r-process nucleosynthesis [4]. Needless to say, the historic detection of gravitational waves from a merger of two neutron stars, GW170817 [5], together with radiation from a short γ-ray burst, GRB 170817A [6], followed by a kilonova [7] makes it a timely and imperative task to unveil inherent properties of the nuclei far away from stability. Furthermore, the superheavy nuclei produced so far are neutron-deficient ones, and the predicted island of stability has not yet been reached [8, 9]. The production of neutron-rich superheavy nuclei in the island is highly desired, as it would provide a new stringent constraint for microscopic theories. Therefore, the study of MNT reactions is listed as one of the key subjects at the current and future RI beam facilities, such as RIBF (RIKEN, Japan) [10], HIRFL-CSR and HIAF (IMP, China)¹, RAON (RISP, Korea) [11], DRIB (FLNR, Russia)², SPIRAL2 (GANIL, France) [12], FAIR (GSI, Germany)³, SPES (INFN, Italy)⁴, FRIB (MSU, USA) [13], and so on.

This review briefly digests recent advances of microscopic reaction theories to stimulate new ideas for the future study of MNT reactions. In section 2, the theoretical framework of TDHF is succinctly recalled. In section 3, the current status of the MNT study with TDHF is given. In section 4, recent advances of theoretical approaches are outlined, along with a discussion on possible future applications. The article is concluded in section 5. The readers are intended to refer to other articles in this Research Topic for discussions on experimental as well as other theoretical studies.

2. The TDHF Theory

The TDHF theory was first proposed by Dirac [14] and its application to nuclear physics started in the 1970s [15–22]. The TDHF theory can be formally derived based on the time-dependent variational principle (various derivations can be found in a recent review [23]). In TDHF, the wave function is a Slater determinant for all times, and the Pauli principle is thus automatically ensured. The TDHF equations read as follows:

\begin{matrix} i ℏ \frac{\partial ϕ_{i} (r σ q, t)}{\partial t} = \hat{h} [ρ (t)] ϕ_{i} (r σ q, t), & (1) \end{matrix}

where ϕ_i(rσq, t) is the single-particle wave function of ith nucleon at position r with spin σ and isospin q. $ĥ [ρ (t)] = \frac{δ E}{δ ρ}$ is the single-particle Hamiltonian with ρ being the one-body density. E[ρ] is the total energy, which may be regarded as an energy density functional (EDF) in the context of time-dependent density functional theory (TDDFT)⁵ [27–31]. The EDF is constructed so as to reproduce static properties of finite nuclei in a wide mass region and also the basic nuclear matter properties (see e.g., [32–35] and references therein). There is no adjustable parameter in TDHF, once an EDF is given. The non-linearity arises because ĥ[ρ(t)] contains the mean-field potential which depends on densities generated by all the nucleons, e.g., $ρ (r, t) = \sum_{i, σ, q} | ϕ_{i} (r σ q, t) |^{2}$ . Such non-linear couplings between single-particle and collective degrees of freedom give rise to the so-called one-body dissipation mechanism (known as wall-and-window formulas [36]). Note that as is evident from a derivation of TDHF from the lowest order truncation of the Bogoliubov-Born-Green-Kirkwood-Yvon (BBGKY) hierarchy [23], where two-body correlations are neglected, two-body dissipations associated with nucleon-nucleon collisions are absent in TDHF. They are, however, expected to play a minor role at low energy due to the Pauli exclusion principle.

TDHF is notably versatile—by changing initial condition, external potential, boundary condition, etc., one can study a wide variety of phenomena: not only collective excitations in the linear response regime [37–46], where TDHF is formally equivalent to the random phase approximation (RPA), but also induced fission [47–52], dynamics of exotic configurations like α-chain structure [53], toroidal nucleus [54, 55], nuclear pasta formation [56–59], as well as heavy-ion reactions, such as nucleon transfer [60–74], quasifission (QF) [75–87], and fusion [88–104], and so on (see [22, 23, 26, 31, 105, 106], for review papers).

In the case of heavy-ion reactions, the initial wave function is composed of projectile and target nuclei in their ground state, which is obtained by self-consistently solving the static Hartree-Fock equations [iℏ∂/∂t → ε_i in Equation 1]. Those wave functions are placed in a computational box, with a sufficiently large relative distance, boosted with a proper relative momentum. The time evolution according to Equation (1) then allows us to follow reaction dynamics in real space and real time. As the theory deals with the single-particle wave functions of nucleons, not only dynamic effects such as nucleon transfer and internal excitations, but also structural effects such as static/dynamic shell effects and shape evolution are naturally incorporated into the description. Spin-orbit coupling is known to play an important role in energy dissipation processes in heavy-ion reactions [107–110]. The effects of the tensor term on nuclear dynamics were also investigated recently [101, 111–114]. TDHF enables us to study rich and complex physics of low-energy heavy-ion reactions from nucleonic degrees of freedom.

3. TDHF Theory for Multinucleon Transfer Reactions

3.1. Multinucleon Transfer Reactions

The MNT reaction may be regarded as a non-equilibrium quantum transport process of nucleons during a collision. With the help of the particle-number projection technique [64], transfer probabilities can be deduced from a TDHF wave function after collision. In Sekizawa and Yabana [65, 66], a range of reactions at energies around the Coulomb barrier were studied within TDHF, for which precise experimental data are available [115–118]. Those reactions are characterized by different neutron-to-proton ratios, N/Z, and charge product, Z_PZ_T. TDHF identified two distinct transfer mechanisms: (1) a fast isospin equilibration process and (2) transfer of many nucleons associated with dynamics of neck formation and its breaking. The neck breaking dynamics emerge at small impact parameters, especially in reactions with a large Z_PZ_T (≳ 1,600). The latter may be regarded as a precursor to QF, where the system starts evolving toward the mass equilibrium. Comparisons with measured cross sections showed that TDHF works fairly well in accuracy comparable to other model predictions. However, effects of secondary neutron evaporation become substantial, when many protons are transferred. Combining TDHF with a statistical compound-nucleus deexcitation model, GEMINI++ [119], it became possible to compute production cross sections after secondary deexcitation processes. With the method, dubbed TDHF+GEMINI, it was shown that the inclusion of deexcitation effects substantially improves agreement with the experimental data [72] (see Figures 1A,B). (For more details, see, e.g., [69, 72]).

FIGURE 1

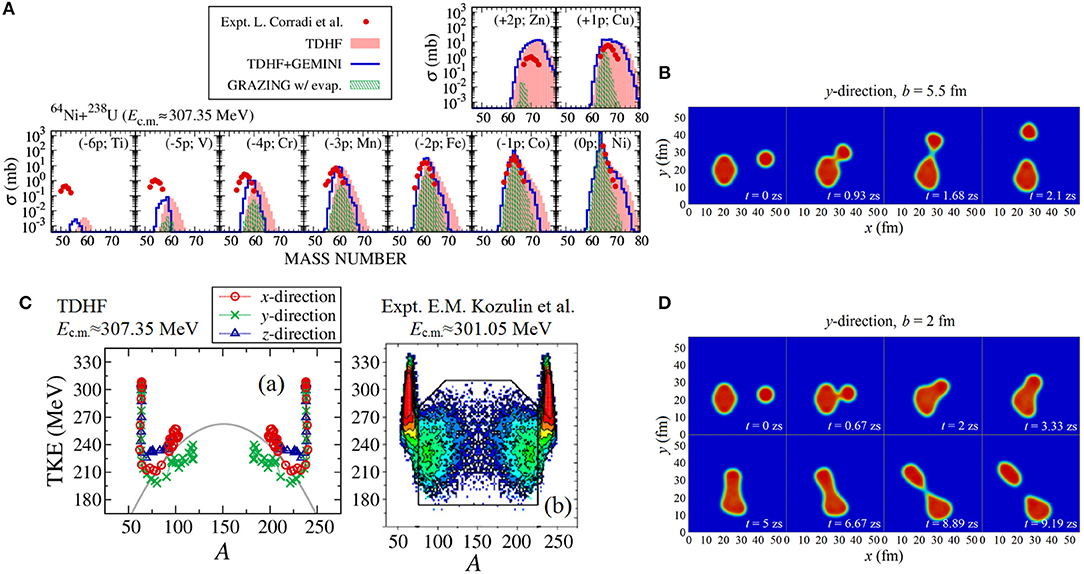
Figure 1. Results of TDHF calculations for the ⁶⁴Ni+²³⁸U reaction at E_c.m. ≈ 307 MeV are shown as an illustrative example. (A) Production cross sections for various proton transfer channels [indicated by (±xp), where plus (minus) sign corresponds to transfer from ²³⁸U to ⁶⁴Ni (vise versa)] as a function of the mass number of the reaction products. Red points are the experimental data [120], red-filled histograms are cross sections for primary products by TDHF [70], and blue histograms are cross sections for secondary products by TDHF+GEMINI [72]. For comparison, cross sections by a semi-classical model, GRAZING⁶, including neutron evaporation effects, are also shown by green shaded histograms. (B) This picture exhibits the time evolution of the density in the reaction plane in a peripheral collision (b = 5.5 fm), where MNT processes shown in (A) take place. Elapsed time in the simulation is indicated in zeptoseconds (1 zs = 10⁻²¹ s). (C) Correlation between the total kinetic energy (TKE) and the mass number A of the outgoing fragments. The left figure (a) shows results of TDHF calculations [70], while the right figure (b) shows the experimental data [121]. (D) Same as (B), but for a smaller impact parameter (b =2 fm), where QF processes shown in (C) take place. In the figure, x-, y-, and z-direction indicate the orientation of deformed ²³⁸U [70]. In this way, TDHF can describe both peripheral and damped collisions in a unified way. Figures were reprinted from Sekizawa and Yabana [70] and Sekizawa [72].

The description is still, however, not perfect, especially for channels where both neutrons and protons are removed. It would be improved when one includes missing one-body fluctuations into the description (cf. section 4.2). Nevertheless, TDHF may be used, taking advantage of its non-empirical nature, to explore the optimal or novel reaction mechanism for producing new neutron-rich unstable nuclei, at least qualitatively. Recently, other groups have also implemented TDHF+GEMINI: e.g. production of N = 126 neutron-rich nuclei was investigated for the ¹³²Sn+²⁰⁸Pb reaction [122].

3.2. Quasifission Processes

To synthesize the heaviest elements and also to explore the predicted island of stability, it is crucial to establish deep understanding of the main competitive process, the quasifission. The QF process is characterized by a large amount of mass transfer, full energy dissipation, and a long contact time (typically several to tens of zeptoseconds). The characteristic observables of QF are correlations between fragment masses with scattering angles, known as mass-angle distribution (MAD), or with the total kinetic energy (TKE) of outgoing fragments, sometimes called mass-energy distribution (MED) (see e.g., [121, 123–126]). In recent applications of TDHF, it has been shown that TDHF can quantitatively describe main QF dynamics, consistent with experimental observations [70, 78, 80, 81] (see Figures 1C,D). An intriguing indication is that in collisions involving an actinide nucleus, TKE becomes larger (smaller energy dissipation) for tip collisions as compared to side collisions [70, 84], perhaps due to shell effects of ²⁰⁸Pb [127]. This may be in contrast to the naive consideration, where one expects a compact shape at scission for side collisions, which would result in larger TKE for side collisions.

The revival of interest in the MNT reaction was initiated by the seminal work by Zagrebaev and Greiner [128–137]. In their work, the importance of shell effects in MNT processes was emphasized. For example, in the ²³⁸U+²⁴⁸Cm reaction, ²³⁸U tends to evolve toward doubly-magic ²⁰⁸Pb, giving the rest of the 30 nucleons to the heavier partner, resulting in the production of transcurium nuclei as primary reaction products. The latter process is called inverse (anti-symmetrizing) QF (IQF), since the ordinary QF drives the system toward the mass equilibrium. Clearly, it is essential to correctly include static and dynamic effects of nuclear shells to describe IQF. The IQF was also observed in TDHF. In Kedziora and Simenel [76], a typical “tip-on-side" orientation in collisions of two actinide nuclei, ²³²Th+²⁵⁰Cf, was found to result in IQF, where about 15 nucleons in the tip of ²³²Th were transferred to the side of ²⁵⁰Cf. Another type of IQF was reported in Sekizawa [73], where complex surface vibration modes are induced in tip collisions of ²³⁸U+¹²⁴Sn at energies well above the Coulomb barrier, which resulted in an abrupt development of a neck, forming a smaller subsystem, leading to transfer of around 27 nucleons from ¹²⁴Sn to ²³⁸U.

An important message here is that those IQF processes emerge as a main reaction outcome in TDHF. Since larger effects of fluctuations and correlations, that give rise to a wider fragment mass distribution, are expected in such damped collisions [105, 138–140], novel IQF processes may pave new pathways to unexplored territories in the nuclear chat far away from stability.

4. New Techniques and Extensions

4.1. Extraction of Macroscopic Physical Ingredients

The potential energy surface in a colliding nuclear system is often a key ingredient in phenomenological approaches. With the constrained Hartree-Fock technique [141], one can compute the adiabatic potential energy in a composite system. However, it differs, in general, from the potential in the entrance channel of a collision, where incident-energy-dependent dynamic effects come into play. Such a dynamic potential should be derived from microscopic theories, e.g., TDHF, where dynamic effects such as shape deformation (or necking), nucleon transfer, and inelastic excitations through the one-body dissipation mechanism are naturally taken into account. A method named dissipative-dynamics TDHF (DD-TDHF) [82, 142, 143] allows us to extract from TDHF not only a dynamic potential, but also a friction coefficient, based on a mapping of TDHF trajectories onto a set of classical equations of motion. Density-constrained TDHF (DC-TDHF) [88] is another way to extract a potential. In the latter approach, the density distribution obtained from TDHF is used as a constraint for Hartree-Fock calculations, leading to an adiabatic potential along the TDHF dynamical trajectory (see e.g., [53, 89–101], for various applications). Energy dependence as well as transition from dynamic to adiabatic potentials may be important as well. Later, the idea of the density constraint was also applied to extract a bare (without density distortion) potential taking full account of the Pauli exclusion principle, which is named density-constrained frozen Hartree-Fock (DC-FHF) [144]. Such a bare potential can be applied to, e.g., coupled-channel calculations for fusion reactions [145, 146]. Indeed, such a connection was established, where excitation energies of low-lying collective excitations were also determined by TDHF [147]. This is a remarkable example of building a bridge between elaborated macroscopic or phenomenological frameworks and those rooted with microscopic degrees of freedom, that may enhance predictability of the former.

4.2. Balian-Vénéroni Variational Principle and TDRPA

While there are, of course, limitations inherent in TDHF (e.g., the absence of many-body correlations, deterministic nature as well as spurious cross-channel couplings due to the single mean-field description [141]), some of them can nowadays be overcome with extended approaches. One such approach can be obtained from the variational principle of Balian and Vénéroni [148, 149]. The Balian-Vénéroni variational principle enables us to control the variational space according to not only the state in the Schrödinger picture, but also to the observable in the Heisenberg picture. For instance, a variation optimized for a Slater determinant and a one-body observable derives the TDHF equations [105]. By extending the variational space for fluctuations of a one-body observable, one obtains a formula that includes effects of one-body fluctuations on top of the TDHF mean-field trajectory, which may be regarded as time-dependent RPA (TDRPA). It has recently been shown that the description of the width of fragment mass distributions in deep-inelastic collisions, which is severely underestimated in TDHF [17, 18, 150], is substantially improved [151, 152], or even quantitatively agrees with experimental data [153], in TDRPA. It implies that one-body dissipation and fluctuations described by TDHF and TDRPA, respectively, are the predominant mechanisms in deep-inelastic collisions. Here, caution is required: the TDRPA formula in the present form can only be applied to symmetric reactions [153]. To overcome this difficulty, one may extend the derivation based on the Balian-Vénéroni variational principle to include higher-order corrections, or derive a formula generalized for asymmetric reactions from, e.g., the stochastic mean-field (SMF) theory [154, 155], from which one can derive the TDRPA formula in the small fluctuation limit [154]. Note that effects of two-body correlations were also indicated by the time-dependent density-matrix (TDDM) approach [156–160].

4.3. Stochastic Extensions

Another limitation of TDHF lies in its deterministic nature. To correctly describe abundant reaction outcomes as observed experimentally, one may need to introduce quantal, collisional, or thermal fluctuations that induce bifurcations of dynamics, leading to, in the end, minor processes apart from the TDHF mean-field trajectory. In the SMF approach, extensively developed by Ayik and his coworkers [138–140, 154, 155, 161–169], initial fluctuations are introduced in a stochastic manner, which gives rise to an ensemble of final states after dynamic evolutions. For the MNT study, the SMF description can be cast into the Fokker-Planck equations, where transport (drift and diffusion) coefficients are determined by the single-particle orbitals in TDHF. In Yilmaz et al. [169], the SMF approach was also applied to the Ni+Ni reaction, showing quantitative agreement with experimental data [153], comparable to TDRPA. Recently, Bulgac et al. [170] proposed another way to introduce stochasticity by means of external potentials which are random in both space and time. The method was applied to describe induced fission processes, with a simplified orbital-free approach, getting a sufficient width of the fission fragment mass distribution [170]. Further applications of those approaches may be promising for exploring optimal reactions, especially for producing the most exotic isotopes, where correct description of rare processes may be essential.

4.4. Inclusion of Pairing

Despite the known importance of pairing in nuclear structure studies, the effect of pairing on nuclear reaction dynamics has rarely been investigated thus far. While the BCS approximation has been employed to date [171–174], inclusion of dynamic effects of pairing became possible only quite recently with top-tier supercomputers. One may naively expect that pairing in the nucleus is so fragile and it would only affect tunneling phenomena below the Coulomb barrier, where clear effects of nucleon-nucleon correlations were indeed observed experimentally [62, 175–177]. To the contrary, Magierski et al. [178–180] found unexpectedly large effects associated with the relative phase (or gauge angles) of colliding superfluid nuclei on the reaction outcomes, such as TKE and fusion cross section. In this context, experimental fusion cross sections were analyzed, which indicates fusion barrier width may be increased by the superfluid effect [181]. In the work by Magierski et al., a local formulation of superfluid TDDFT, known as time-dependent superfluid local density approximation (TDSLDA) (see e.g., [182–185]), was employed, which is formally similar to the time-dependent Hartree-Fock-Bogoliubov (TDHFB) approach [141]. The TDSLDA has been extensively developed by Bulgac and his coworkers, which was successfully applied to the unitary Fermi gas [186–191], finite nuclei [178, 192–196], as well as interiors of neutron stars [197]. Hashimoto has developed a Gogny TDHFB code that works with a 2D harmonic oscillator and 1D-Lagrange-mesh hybrid basis [198], which could be applied for head-on collisions [199, 200]. Qualitatively, the same effects of the relative phase as reported in Magierski et al. [178] were observed with their code. We have just entered a new era in which dynamic effects of pairing can be fully incorporated with microscopic nuclear dynamics studies. With the aforementioned sophisticated approaches with the usage of top-tier supercomputers, the dynamic effects of pairing in MNT and QF processes will be unveiled in the near future.

4.5. Remarks on Other Theoretical Approaches

There are many models that have been developed so far for the study of MNT, such as the semi-classical model, like GRAZING [201– 205]⁴,⁷ or CWKB [116–118, 206], the dinuclear system (DNS) model [207–221], the improved quantum molecular dynamics (ImQMD) model [222–225], and the Langevin model [128–137, 226, 227]. There are pros and cons in each of those approaches. For instance, on the one hand, the semi-classical model can describe successive transfer processes at peripheral collisions, while on the other hand it misses deep-inelastic components at small impact parameters. In contrast, the DNS model is capable of describing the dynamic evolution of a composite system, according to a master equation with a potential energy (including shell effects) for mass asymmetry and deformation of the subsystem, that gives rise to a probability distribution for massive nucleon transfer as well as fusion. By construction, however, the latter model assumes a formation of a “di-nucleus” in a potential pocket, and thus it misses (quasi)elastic components at large impact parameters. A simple addition of those model predictions, DNS+GRAZING, was considered in the literature [228, 229]. The ImQMD model is applicable for both peripheral and damped collisions. By taking into account stochastic nucleon-nucleon collisions, it provides distributions of observables accumulated in a number of simulations. To the author's knowledge, however, the spin-orbit interaction has been neglected in ImQMD, which prevents a proper description of shell effects in MNT and QF processes. The elaborated Langevin model [226] may be promising in describing complex processes of MNT, QF and fusion, in a unified way. The model contains, however, various parameters that have to be tuned carefully to reproduce available experimental data [226].

A possible future task is to non-empirically determine model ingredients based on microscopic theories: potential energy surface with respect to an arbitrary set of variables, drift, diffusion, and friction coefficients, etc., can be derived from the microscopic approaches, which may lead to, e.g., kind of a TDHF+Langevin approach.

5. Summary and Prospect

Production of neutron-rich heavy nuclei is listed as one of the high-priority subjects of nuclear science today. In this contribution, recent advances in the MNT study, especially those based on microscopic dynamic theories, have been briefly reviewed. The traditional TDHF approach has shown remarkable successes in describing a variety of phenomena in nuclear systems. It has been shown that TDHF is capable of describing the main reaction channels, not only MNT processes in peripheral collisions but also deep-inelastic and QF processes in damped collisions, positioning it as a good starting point for building a fully microscopic theory for low-energy heavy-ion reactions. Moreover, the inclusion of one-body fluctuations significantly improves quantitative agreements of the width of fragment mass distributions with experimental data. With the use of top-tier supercomputers, studies of dynamic effects of pairing have just been started. One of the great advantages of microscopic approaches is their non-empirical nature—one may explore novel pathways toward the dreamed-of production of yet-unknown neutron-rich nuclei. Another possible future direction is to use microscopic approaches to determine phenomenological parameters in other theoretical models. It may be the time to combine knowledge obtained with different approaches to construct the most reliable framework to lead future experiments to the successful production of extremely neutron-rich nuclei, the farthest from the continent of stability.

Author Contributions

The author confirms being the sole contributor of this work and has approved it for publication.

Conflict of Interest Statement

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

The author acknowledges P. D. Stevenson, J. A. Maruhn, C. Simenel, Lu Guo, and A. S. Umar for careful reading of the manuscript and for providing valuable comments on this article.

Footnotes

1. ^http://english.imp.cas.cn/Home2017/

2. ^http://flerovlab.jinr.ru/flnr/dribs.html

3. ^https://fair-center.eu/

4. ^https://web.infn.it/spes/

5. ^Here a local EDF (like Skyrme) has been assumed, which makes the TDHF Equation (1) local in space, as it is currently used in most practical applications. In general, $E = \int E (r) d r$ is composed of various local densities, $\vec{ξ} \equiv {ρ, τ, \dots}$ , and the TDHF Equation (1) should read: $i ℏ \frac{\partial ϕ_{i} (r σ q, t)}{\partial t} = \int \sum_{k} \frac{δ E [\vec{ξ} (r^{'}, t)]}{δ ξ_{k} (r^{'}, t)} \frac{δ ξ_{k} (r^{'}, t)}{δ ϕ_{i}^{*} (r σ q, t)} d r^{'} = \sum_{σ^{'}} ĥ_{σ σ^{'}}^{(q)} (r, t) ϕ_{i} (r σ^{'} q, t)$ . In such a case, the single-particle Hamiltonian can have spin dependence as well as differential operators (for the explicit form, see, e.g., [24–26]).

6. ^Website of Nuclear Reactions Video (NRV) Project. Available online at: http://nrv.jinr.ru/nrv/webnrv/grazing/

7. ^http://personalpages.to.infn.it/~nanni/grazing/

References

1. Otsuka T, Gade A, Sorlin O, Suzuki T, Utsuno Y. Evolution of nuclear structure in exotic nuclei and nuclear forces. arXiv:1805.06501 [nucl-th]. (2018).

Google Scholar

2. Cejnar P, Jolie J, Casten RF. Quantum phase transitions in the shapes of atomic nuclei. Rev Mod Phys. (2010) 82:2155. doi: 10.1103/RevModPhys.82.2155