- ASL VT Medical Physics Laboratory, Viterbo, Italy
Developing an approach defined in previous papers a correspondence between elementary particles and elements of a particular set of directed graphs said “glyphs” is made explicit. These can, in turn, be put in correspondence with a particular set of biquaternions. Charge conjugation and weak isospin inversion then become both topological (on glyphs) and algebraic (on biquaternions) symmetries. The formalism describes leptons and quarks, the latter successfully combined into mesons and baryons. The introduction is possible of both a de Sitter interior spacetime of particles and the usual external Minkowski spacetime. The biquaternions can be related to creation and annihilation operators of the corresponding fermionic fields in Quantum Field Theory (QFT). The base states of QFT and their mutual interactions are thus constrained by their common emergence from logically antecedent structures. This result appears relevant for the understanding of the elementary particles spectrum.
Introduction
The Quantum Field Theory (QFT) is currently the most successful theory in the description of the fundamental interactions between elementary particles. Nevertheless, it still presents several open problems. The most formidable of these is, in our opinion, the following: the QFT formalism does not constraints the base states (that is, the spectrum of “elementary particles”) nor the form of their mutual interactions. The fields and their Hamiltonians (or Lagrangian), both free and of interaction, are introduced by incorporating data or principles borrowed from experience, so to account for the observed phenomena. As a result of this state of affairs there is not a well definite QFT, but different QFT descriptions of fundamental interactions (standard model, supersymmetric models and so on). In this work, which develops results already shown in previous articles [1, 2], is considered a scenario in which the QFT level of description is emerging from more fundamental structures, which determine both base states and their interactions. This attempt of QFT “logicization” builds on the basic concepts of microevent and “elementary particle.”
In this perspective, the physical world consists of events (the so called interaction vertices) where some elementary particles are annihilated and new sets of particles are created. So, let us define an elementary particle (lepton, meson or baryon) as a connection between two interaction vertices where it is created and successively annihilated. We recall here that only leptons seem really elementary, while mesons and baryons contain two and three quarks respectively. Gauge bosons can be considered as operators exchanging leptons or quarks in a given vertex, as will be shown later. The interaction vertices considered in this work are those that connect real (asymptotic) incoming and outgoing, leptonic, mesonic or baryonic states, while the quarks exchanged among hadrons within the interaction vertex do not appear as asymptotic states. The idea examined in preceding works [1, 2] and that we intend to develop further here is that the causation of such events is of a formative, rather than efficient, type. In other words, we assume that the time evolution of particle states is described by the conventional QFT formalism based on unitary evolution operators, which thus constitute the formal representation of a diachronic efficient causality. We interpret instead the appearance or disappearance of such states in an interaction vertex as a non-unitary process (connected with the reduction of the wave packet [3] through which certain physical observables emerge from an unobservable background breaking into time, or are reabsorbed in it disappearing from time. Such a process is necessarily synchronic and adynamic, as it connects an atemporal background with the time domain; this connection manifests itself in the interaction vertices.
The exposition will proceed as follows. In section Fragmentation of the void we present the fundamental assumptions regarding this process, attempting to elucidate the relations with well-known notions of particle physics such as color, generation, weak isospin. In section Directed glyphs and hypercomplex numbers we describe a particular representation of the process by means of graphs which we shall call glyphs; we also suggest a correspondence between glyphs associated with the various particles and certain specific hypercomplex numbers. We introduce the key concept of “universal oscillator,” a graph from which all the glyphs can be obtained by means of operations of distinction or identification of the various edges. In section Field operators we argue that a correspondence exists between these operations and QFT creation and annihilation operators. In section Classification of elementary interactions the fundamental interactions are re-interpreted in this perspective. section Connection to the Standard Model elucidates some connections with the Lagrangian formulation of the Standard Model of elementary particles and their interactions. Section Some reflections on the nature of the Higgs field is devoted to some reflections about the nature of the Higgs field from the perspective presented in this article. Comparison with works available in current literature is discussed in section Are elementary interactions a form of computation? The conclusions are reported in section Conclusions.
Fragmentation of the Void
In [1, 2] we have characterized the background as the set of algebraic operators which satisfy the conditions:
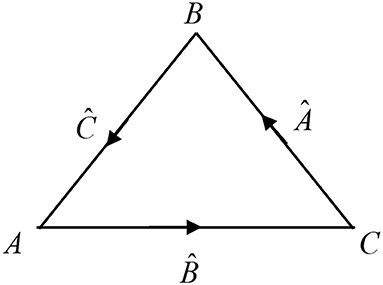
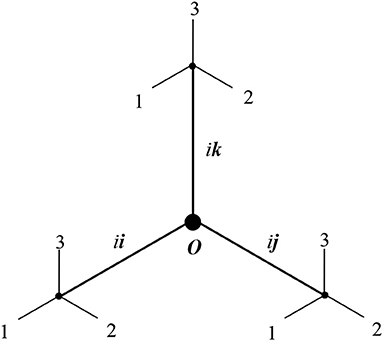
where Î denotes the identity operator. It is possible to put these operators in correspondence with the sides of the oriented triangle ABC in Figure 1. The operator  represents the side C→ B, while its inverse Â−1represents the side B→ C of the triangle obtained by inverting the directions of sides in Figure 1. The operators and Ĉ can be put in an analogous correspondence with the other two sides of the triangle, by means of a cyclic permutation of the letters A, B, C in the previous definition.
It is possible to obtain a simple geometric representation of these operators and their product by imagining the points A, B, C of Figure 1 as the vertices of a spherical triangle (on an ordinary two-dimensional spherical surface of center O) whose inner angles and sides are all right angles. Let us consider the rotation of a straight (flat) angle of the spherical surface around an axis perpendicular to the plane containing B, C and the center of the sphere and passing through the latter. This rotation transforms the sphere into itself by bringing each point to coincide with its previous symmetric respect to the axis OA, while the point of the sphere initially coinciding with C is moved in the direction of B. We associate the operator  to the quaternion representing this rotation. The operator Â−1 is then associated with the quaternion representing the inverse rotation. The other operators are represented in a similar way.
The product of two operators in a certain order is then represented by the quaternion associated with the product of the corresponding spherical rotations executed in the same order. This representation makes clear the meaning of the relationships (1) and (2a): the relations (1) state that the execution of the rotations corresponding to the three operators considered in anticyclic order sends each point of the spherical surface into itself; the relations (2a) state that the same effect is obtained by subsequently performing a rotation and its inverse.
The equation (2b) implies for each pair of operators ,Ŷ. The product ŶŶcommutes with each operator converting it into its own inverse. It is not possible to interpret this product as the identity, because this identification would involve , against the geometrical interpretation of operators illustrated in Figure 1. We denote the universal action of ŶŶ through the symbol −Î = ŶŶ, thus obtaining .
Based on what we said we can represent the operators and their inverses as quaternionic units, by letting . The product between operators is mapped on the product between quaternionic units. In this way, we obtain the usual relations occurring between quaternionic units:
We remark that ŶŶ = −Î⇒−1, so that the symbol minus in operators algebra is mapped on the symbol minus of quaternionic units. This mapping reveals the meaning of Equation (2b): the inverse can be also interpreted as the opposite (rotating of two flat angles in the same direction is different from rotate of a flat angle in one direction and then in the opposite direction; in the first case, the total arc is 2π, in the second case it is 0).
The relevance of the structure made up of the quaternionic units and their product is justified by the self-duality of the graph in Figure 1. This is the only self-dual graph in which every possible connection between vertices is a graph edge and corresponds to vertex under a duality operation (mutual conversion of vertices and sides). It constitutes the only set of objects that coincide with all and only their mutual transformations, a property that we postulate as essential to describe something that is self-generated, spontaneous [1, 2]. The relation XY = Z, where (X,Y,Z) is a cyclic permutation of (i, j, k) [or, alternatively, an anticyclic permutation of (–i, –j, –k)], means “Z emerges as the result of the action of X on Y”; we are therefore dealing with the co-emergence of the elements i,j,k (or –i, –j, –k) or their mutual co-creation.
This motivation can also be read in systemic terms. In the graph of a system we have knots and arrows. Nodes represent events or “individual” objects, arrows or edges represent their relations. The laws of formation of the nodes can be distinguished from those of the edges; we can say, using a reductionist language, that the fundamental laws can be distinguished from the boundary conditions. All this is very clear, for example, in molecular structures, whose representative graphs are the structural formulas. As is well known, much chemistry can be done simply by reasoning on connection laws and completely ignoring the laws of node formation (i.e., focusing on chemical bonds and ignoring the underlying quantum mechanics).
In the case of the timeless background constituted by the quaternionic units, the latter form the only set of objects that coincide with their relations (by virtue of self-duality). In this sense we can say that at the level of the background the structural laws and those of relationship coincide and cannot be distinguished. It is no longer possible to distinguish between “laws” and “conditions” (both represented by the algebraic rules described above) and we are faced with a level of irreducible complexity, which cannot be further analyzed. This level corresponds to the maximum possible “elementarity” and it is from this that the “elementary particles” emerge. The timeless background level thus represents the (unreachable) limit of the reductionist analysis of matter, corresponding to a stage in which the bottom-up description (from nodes to edges) coincides with the top-down one (from edges to nodes). This is the lowest possible level of processes in the physical world.
In the geometric representation described above, the vertices A, B, C of the spherical triangle can be chosen arbitrarily on the spherical surface. In other words, the triad of orthogonal axes OA, OB, OC (where O is the center of the sphere) can be arbitrarily chosen provided that these axes are orthogonal, have the same length as the radius of the sphere and the chirality of the system remains unchanged (we say right-hand). This means that this triad is defined unless of a rotation in three-dimensional space (∞3 possibilities) and a homotetia that multiplies the sphere radius for a factor exp(t) ∈ (0,∞). This homotetia generates additional ∞1 possibilities, each associated with a point t ∈ (–∞, +∞) of the real axis. The arbitrary rotation can be described by a unitary quaternion, and by multiplying this quaternion for exp(t) we obtain a general quaternion O. In this sense we can say that ∞4 distinct instances of the representation we introduced are possible, each associated with a value of O. The instantiation operation consists therefore of the passage from a single abstract set V = {1, i, j, k, –1, –i, –j, –k} to one of ∞4 distinct sets V(O) = {1(O), i(O), j(O), k(O), –1(O), –i(O), –j(O), –k(O)}, where O is a real quaternion. The algebras of the elements of V(O), V(O') are different if O and O' are different.
As we'll see, the elements of V(O) define physical quantities that are localized, following an interaction between elementary particles, in the point-event O. Each element of V(O) appears together with its opposite, so we are at a stage where O is still empty. The set of V(O) sets, where O runs on the set of real quaternions, represents therefore, in this description, the empty spacetime.
It is possible, at this level, to introduce the concept of the possibility of causal connection between two possible instantiations. We will say that two instantiations may be causally connected if there is, on the space of real quaternions {O}, a sequence of N steps (+1,+i), N steps (+1, –i), N steps (+1,+j), N steps (+1, –j), N steps (+1,+k), N steps (+1, –k), taken in any possible order, leading from one to another. Each step consists of a “spatial” translation represented by the imaginary unity ± i, ±j, ± k accomplished in an unitary “time” interval +1. Since the ratio between the modulus of the spatial translation and this time interval is 1, each single step is in effect a jump to the limit speed c = 1. Posing N = (N + N) + (N + N) + (N + N); Ni = (Ni+-Ni−) with i = 1,2,3 one has:
In general, we can consider jumps of arbitrary duration T and use arbitrary units for c. Then, posing NcT = ct, N1cT = x, N2cT = y, N3cT = z:
The inequality (3) represents the interior of the light cone in a Minkowski space {(ct, x, y, z)}, whose vertex corresponds to the start or end point of the succession of steps. In the following we will use the coordinates of the Minkowski space, instead of the real quaternions, to distinguish the various possible instantiations.
Let us now examine what we think takes place during the actual instantiation of a particle in an interaction vertex, bearing in mind that two different levels of explication are involved in this process: one that corresponds to the creation or annihilation of the particle state outgoing from the vertex or ingoing to the vertex, respectively; the other relating to the definition of the properties of the individual centers of charge within the vertex. Leptons contain only one center of charge, hadrons two or three (quarks).
It is therefore necessary to introduce a distinction between the internal spacetime of the particle, that is the spacetime “perceived” by the centers of charge in their mutual interactions without relation to the outside world, and the external spacetime where the particle propagates as a whole; interactions with other particles or external probes “perceive” this external spacetime. While the external spacetime is a Minkowski space, the internal spacetime must correspond to a portion of Minkowski space whose geometry is perceived by the centers of charge in a different way. This different “perception” mode must correspond to the confinement of the internal processes of the particle and thus to the presence of a horizon. In this paper, we consider elementary particles not as objects in the sense of the ontology of classical physics, but as events or instances. The horizon we are talking about must therefore be a time horizon.
Let us redefine the point in four-dimensional Minkowski space that corresponds to the actual instantiation of the particle (that we assume corresponding to an eigenvector of particle position, thus neglecting the spatial delocalization of the particle over its de Broglie scale) as the origin (0,0,0,0) and consider the region made of up the points (λ0, λ1, λ2, λ3) with:
where θ is dependent on the type of instantiated particle, while θ0 is a universal constant that we shall call “chronon.” This constant establishes the scale of the temporal localization of the particle in the interaction vertex, and elsewhere we have considered the identification of cθ0 with the classical radius of the electron [3].
From the outside, the (4a) is only a region of Minkowski spacetime. We postulate that the dynamics of internal particle processes sees instead (4a) as an invariant. To give a formal expression to this postulate, we reinterpret the Minkowski spacetime to which (4a) belongs as tangent to the five-dimensional quadrics:
in the point (λ0, λ1, λ2, λ3, λ4) = (0,0,0,0,cθ). In the five-dimensional space, this point belongs to the subspace λ4 = cθ which is precisely the Minkowski space. The five-dimensional light cone (λ0)2 − (λ1)2 − (λ2)2 − (λ3)2 − (λ4)2 = 0 intersects this space in two sheets forming the edge of (4a). On the other hand, it does not intersect the (4b) of which it constitutes an asymptote. Therefore, the straight segment having the five-dimensional origin (0,0,0,0,0) and a generic point of (4b) as its ends will intersect Minkowski tangent space at a point within (4a). The (4b) can therefore be projected onto (4a). We will assume that the internal space of the particle is the de Sitter space (4b), whose bounded projection on the Minkowski external space is given by (4a). The edges of (4a) are three-dimensional hyperbolic spaces.
The set of coordinates transformations leaving the (4b) unchanged is the de Sitter group [4], so our request translates into assuming that geometry “perceived” by internal processes is the geometry of a de Sitter space of radius cθ. To understand the difference with the usual geometry, let us consider the very simple case of a temporal translation from the point O = (0,0,0,0) to the point (cT,0,0,0) with θ > T > 0. In Minkowski geometry (perceived by external processes that link the particle to the outside) this translation approaches the observer to the future sheet of the hyperboloid that forms the boundary of (4a). At the same time, the observer moves away from the past sheet of the same hyperboloid. In de Sitter's geometry (perceived by internal processes), the observer's chronological distance from the two sheets of the hyperboloid remains unchanged and equal to θ, whatever the value of T. This is possible because in the kinematics of de Sitter space a rule of composition of durations holds that has the same form as that of speeds in Special Relativity. As the speed of light is unattainable by composition of sub-c speeds, so it is impossible to exceed the duration θ by composing durations smaller than θ [5]. In other words, the two sheets of the hyperboloid appear to the observer as a horizon: the de Sitter horizon. The parameter θ measures the (chronological, in Beltrami time) distance of each internal observer from the horizon. It is called “de Sitter time.” The unattainability of the horizon becomes, from an external point of view, a limit on the maximum duration of every internal process related to the particle instantiation, that does not exceed θ. For this reason, the constant θ0, which sets an upper limit on the de Sitter time, fixes the scale of the extension of a particle on the time domain, when it is “instantiated” on Minkowski space by interaction with other particles.
Let us thus consider three vectors (cθ, vl1θ, vl2θ, vl3θ), with l = 1,2,3, in the internal space (4a) and two of which (or even all three) can be coincident. Let us also consider their temporal conjugates (–cθ, vl1θ, vl2θ, vl3θ), which will have the same projections Ol = (0, vl1θ, vl2θ, vl3θ) onto the contemporaneousness space of O = (0,0,0,0). We shall identify these projections with the spatial positions of the centers of charge at the time of the instantiation in O. Such positions are defined by the velocity vectors (vl1, vl2, vl3) applied in O.
We postulate that the first step of the instantiation of the particle in O actually consists of the separation of the elements of V(O) = {1(O), i(O), j(O), k(O), −1(O), −i(O), −j(O), −k(O)} (we denote these particular elements in bold font) in two different sets V+(O) = {1(O), +i(O), +j(O), +k(O)} and V−(O) = {−1(O), −i(O), −j(O), −k(O)} or, alternatively, V+(O) = {1(O), −i(O), −j(O), −k(O)} and V−(O) = {−1(O), +i(O), +j(O), +k(O)}. The first possibility is related to the creation of a particle, while the second one is related to the creation of an antiparticle. In the following we only consider the set V+(O), because the set V−(O) undergoes the same process.
The second step consists in the separation of the elements of V+(O)−{1(O)} = {i(O), j(O), k(O)} or (in a disjunctive sense) {−i(O), −j(O), −k(O)} in one, two or three partitions according to the number of centers of charge (respectively one, two or three). Each partition becomes a center of charge, so that the correspondence between partitions and centers of charge is biunivocal. The elements of the same partition Ai (i = 1,…, imax; imax ≤ 3) are mapped on one and the same element of V(Ol)-{−1(Ol), +1(Ol)} where Ol (l = 1,2,3) denotes the position of l-th center of charge, defined by one of the three velocity vectors applied in O. The image of a given partition Ai is assumed to be independent on the value of Ol (l = 1,2,3).
These postulates are equivalent to stating that from the set V, which contains any element and its inverse, an unbalanced situation is reached in which: (1) a temporal localization occurs with the separation of the “retarded” version V+(O) of V(O) from the “advanced” one V−(O); this separation admits an obvious interpretation in transactional approach to quantum mechanics, as we will see in a successive section; (2) each partition of the triad {i(O), j(O), k(O)} (or alternatively of the antitriad {–i(O), –j(O), –k(O)}) is transformed in a single element of {i(Ol), j(Ol), k(Ol), –i(Ol), –j(Ol), –k(Ol)} and the mutual co-creation of its elements is then halted. The result is a single, “fixed,” center of charge whose “internal” properties are represented by that element. This is equivalent to the exit from a state of self-regenerating “balanced” vacuum and to the genesis of a particle state or to the inverse process of reabsorption of this state in the vacuum.
The elements of {i(O), j(O), k(O), –i(O), –j(O), –k(O)}, multiplied by the ordinary imaginary unit i, correspond to what in the standard model is the color degree of freedom through the following correspondences (unless cyclic permutations):
where R = red, G = green, B = blue. The color of a specific center of charge is defined as the product of the colors of the elements of the partition mapped on that center, according to the rules: RG , GB , BR = Ḡ, B, R, G, GBR = white. These relations can be seen as derived by the product of terms (5), associated with colors (anticolors), taken in cyclic (anti-cyclic) order with an additional i factor. The symbol ±i represents the white color. We remark that, as a consequence of these rules, the global color of a given particle (lepton-antilepton, meson, baryon-antibaryon) is ever white.
As we shall see, the selected element of {i(Ol), j(Ol), k(Ol), –i(Ol), –j(Ol), –k(Ol)} instead represents the generation of the elementary fermion (quark or lepton) associated with the center of charge in Ol, while its sign corresponds to the electric charge of the fermion.
Directed Glyphs and Hypercomplex Numbers
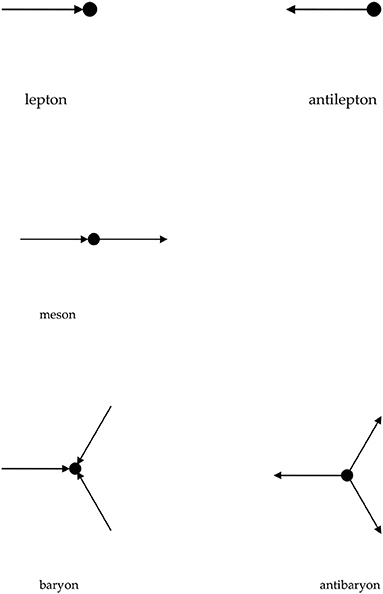
Glyphs have been introduced in Chiatti [2] as graphs that represent the second and third level of explication of the process described in the preceding section. Here, however, we shall consider directed glyphs while in Chiatti [2] the direction was not taken into consideration. A glyph is a tree graph with a root called “major vertex,” first-level branches called “internal edges” each of which connects the major vertex to a “minor vertex” and second-level branches that start from the minor vertices, called “external edges.” The major vertex represents an instantiation in O while each minor vertex represents the instantiation of a center of charge. The internal edge which connects the major vertex O to the minor vertex Ol represents the mapping of a partition Ai of {i(O), j(O), k(O)} (or alternatively {–i(O), –j(O), –k(O)}) on a single element of {i(Ol), j(Ol), k(Ol), –i(Ol), –j(Ol), –k(Ol)}. The product of the colors associated with the elements of Ai defines the color of the center of charge placed in Ol. Equation (5) associates this color (or anticolor) with a single element of {ii(O), ij(O), ik(O), –ii(O), –ij(O), –ik(O)}, which thus becomes a property of the internal edge. Since we start from a triad {ii(O), ij(O), ik(O)} = (R,G, B) or from an antitriad {–ii(O), –ij(O), –ik(O)} = (,Ḡ,) and successively two or three elements can be substituted by their product, the only possibilities are: three colors (baryon), one color, and one anticolor (meson), white (lepton, antilepton), three anticolors (antibaryon). Only one major vertex and one, two or three minor vertices exist, therefore, according to whether one is considering a lepton (antilepton), a meson or a baryon (antibaryon), respectively. We represent colored internal edge as coming in the major vertex and anticolored internal edge as coming out from the major vertex. The center of charge represented by the minor vertex at the other end of the internal edge is a quark in the first case, an antiquark in the second case. We assume coherently internal edges coming in the major vertex for leptons, coming out the major vertex for antileptons.
In conclusion, we have three possible configurations of internal edges, represented in Figure 2.
The incoming edges are elementary fermions (electron, u quark, d quark, neutrino and their analogs of the second and third generation); outgoing edges represent the corresponding antifermions. It is rather singular that graphs of Figure 2 relating to hadrons are the same as those obtained in a completely different context (that of the so-called S matrix topological expansion) by Capra in 1979 [6], apart from the opposite direction of the edges.
Let us now consider the external edges associated with the minor vertex Ol. They represent the image of the partition Ai, which is an element of {i(Ol), j(Ol), k(Ol), –i(Ol), –j(Ol), –k(Ol)}. We represent this element by means of one, two or three external edges having the same direction. The number of external edges defines the generation of the elementary fermion corresponding to the minor vertex (one edge = first generation, two edges = second generation, three edges = third generation). The direction of the external edges (which is the same for all the external edges connected to the same minor vertex) instead defines the fermion charge. A minor vertex with incoming external edges will be associated with a charged lepton (or antilepton) or with an u, c, t type quark (or antiquark). Instead, outgoing edges will denote a neutrino (or antineutrino) or a d, s, b type quark (or antiquark).
In other words, in order to reproduce a single element of {i(Ol), j(Ol), k(Ol), –i(Ol), –j(Ol), –k(Ol)} the external edges connected to Ol can all be distinct (third generation fermion), two identified and one distinct (second generation fermion) or all three identified (first generation fermion). Their common direction, as we have said, determines the charge of the fermion. The identification rules are therefore as follows (incoming/outgoing direction has been distinguished through the sign):
Obviously, if the glyph contains a single internal edge and therefore only one minor vertex, the fermion associated with this latter will be a lepton or an antilepton depending on the direction of the internal edge. Otherwise it will be a quark or antiquark, depending on the direction of its internal edge. Let us consider two examples.
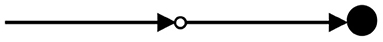
There is only one internal edge entering the major vertex of glyph reported in Figure 3; then this glyph represents a lepton. Moreover, there is only one external edge entering the minor vertex; therefore this vertex is associated with a first generation charged lepton. The glyph illustrated in Figure 3 represents therefore the electron.
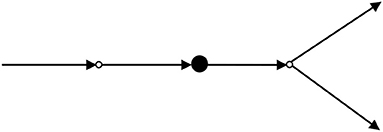
The glyph reported in Figure 4 contains two internal edges, one coming in the major vertex, the other coming out from it. We therefore have a meson made of two quarks. The first quark has only one external edge entering its minor vertex; it is a first generation u-type quark. Its internal edge enters the major vertex; this quark is then actually a u quark.
The internal edge of the second quark comes out from the major vertex; it is therefore related to an anti-quark in the strict sense. The minor vertex has two outgoing external edges; it is therefore an anti-s quark. The represented particle is thus an (anti-s, u) meson of unspecified spin and parity; some dynamical state of the positive kaon.
The directed glyph of a single center of charge Ol can be represented by means of a suitable biquaternion (a, b), where a = ± i(Ol), ± j(Ol), ± k(Ol) represents its external edges and b = ± i1(O), ± ii(O), ± ij(O), ± ik(O) its color. We define the following correspondence between elementary fermions of the standard model and the ordered pairs (a, b) (below we omit the imaginary unit i in b, because implicit in the pair order, and the dependence on O, Ol; internal parentheses mean the alternative “…or…”):
Ist generation:
(i, –1) = ν1; (i, (i, j, k)) = d(R, G, B);
(i, +1) = anti-ν1; (i, (–i, –j, –k)) = anti-d(anti−R, anti−G, anti−B);
(–i, (–i, –j, –k)) = anti-u(anti−R, anti−G, anti−B); (–i, –1) = e−;(–i, (i, j, k)) = u(R, G, B); (–i, +1) = e+;
IInd generation:
(j, –1) = ν2; (j, (i, j, k)) = s(R, G, B);
(j, +1) = anti-ν2; (j, (–i, –j, –k)) = anti-s(anti−R, anti−G, anti−B);
(–j, (–i, –j, –k)) = anti-c(anti−R, anti−G, anti−B); (–j, –1) = μ−;(–j, (i, j, k)) = c(R, G, B); (–j, +1) = μ+;
IIIrd generation:
(k, –1) = ν3; (k, (i, j, k)) = b(R, G, B);
(k, +1) = anti-ν3; (k, (–i, –j, –k)) = anti-b(anti−R, anti−G, anti−B);
(–k, (–i, –j, –k)) = anti-t(anti−R, anti−G, anti−B); (–k, –1) = τ−;(–k, (i, j, k)) = t(R, G, B); (–k, +1) = τ+;
We observe immediately that:
1) The inversion of the “real” part of (a, b), that is the operation a → –a, corresponds to the weak isospin inversion;
2) The inversion of the “imaginary” part of (a, b), that is the operation b → –b, corresponds to the passage to the corresponding antiparticle;
3) Moving from left to right along each row of the diagram, the electric charge changes from 0 to ± 1, respectively in equal steps of ± 1/3;
4) There may be only three states of color (or anti-color) and only three generations of fermions.
5) A “natural” chirality exists: that of the right-handed triad (i, j, k). The weak isospin inversion reverses this chirality.
6) The set of axes (i, j, k, ii, ij, ik) admits two physically relevant subsets: that of flavors (i, j, k) and that of colors (ii, ij, ik).
We note that the here considered correspondence is similar (but different) to that discussed by Rowlands in its “two spaces” formalism [7]. The further correspondence between this set of biquaternions and directed glyphs is very natural. For quarks, an internal edge will be represented by the element b of the pair, regarded with a plus sign if the edge enters the major vertex, with the minus sign if it comes out. It may be ii, ij, ik depending on its color (or anticolor) state. For leptons we have b = –i1; for antileptons b = +i1; the internal edge is respectively coming in and out the major vertex. The configuration of the external edges will be represented, for both leptons and quarks, from the element a of the pair. The first, second and third generation (respectively one, two or three external edges connected to the minor vertex) will correspond respectively to i, j, k, considered with a minus sign if the edges enter the minor vertex, with a plus sign if they come out.
Let us consider, for example, the only elementary fermion in Figure 3. Its internal edge enters the major vertex so that b = –i1; the only external edge enters the minor vertex, it is therefore a = –i. The fermion is thus represented by the pair (a, b) = (–i, –1); it is therefore an electron.
Let us pass now to consider the two elementary fermions represented in the glyph of Figure 4. We have two internal edges, the one incoming and the other outgoing; b is therefore respectively equal to (i, j, k) and (–i, –j, –k). We have in addition a single external edge coming in the minor vertex in the first case, and two external edges coming out from it in the second case; then a is respectively –i and j. The fermions will therefore be represented respectively by the pairs (a, b) = (–i, (i, j, k)) and (a, b) = (j, (–i, –j, –k)). They are then identified respectively with an ordinary u quark and a strange (s) antiquark.
All possible glyphs are generated, through certain operations, from the graph illustrated in Figure 5.
It is necessary to select the direction of each edge, internal as well as external. For an internal edge this selection determines whether the corresponding center of charge is a fermion or an antifermion. For an external edge it defines the electric charge of the fermion (antifermion). All external edges connected to the same vertex have the same direction, thus it has to be selected for only one of them. Furthermore, two or three internal edges can be mutually identified (in which case they all have the same direction) and the same holds for the concurrent external edges in the same minor vertex.
When the edges (respectively internal and external) are all identified and their directions are not fixed we have a configuration which is equivalent to the vacuum. As an effect of the action of certain distinction/identification operators the various particles (glyphs) emerge from the vacuum or come back to it. In a sense, the graph that we are considering is a kind of “universal oscillator” whose ground state is the vacuum, and whose “excitations” are the known elementary particles (leptons, mesons, baryons). It is therefore natural to ask whether the distinction/identification operators expressed in the language of the universal oscillator do not correspond to the different operators of creation/annihilation of the various fields of QFT. The answer is affirmative, as we will check in the next section. More precisely: the structure represented by the universal oscillator selects the possible field operators of QFT. We will see later that it also constrains the interactions.
Field Operators
Several algebraic formalisms can be proposed to represent the states of the universal oscillator and the transition from one of these states to the vacuum or vice versa. For the internal edges (or the external edges associated with a given minor vertex) we have three different degrees of distinction: all three edges are distinct, one is distinct and the other two are not, no edges are distinguished. Even with regard to their direction, we have three different degrees of distinction: the edge enters the vertex (the major vertex for an internal edge, the minor vertex for an external edge), the edge leaves the vertex, the edge has no defined direction. Both of these situations can be described by two-element column vectors, respectively v1i = δi1, v2i = δi2, v3i = 1; i = 1,2. According to this convention, the projectors Mik = δil δkl (l = 1,2) transform v3i in vli while δlk transform vli in v3i. In addition we have the rising operator δi1δj2 that transforms v2i in v1i and the lowering operator δi2δj1 that transforms v1i in v2i. It is therefore possible to describe algebraically the operations of distinguishing the elements of the oscillator, as well as removing such distinctions and returning to the vacuum, through the successive action of suitably defined operators. In the following, however, we will not explicitly use such representations for distinctions. Instead, we will consider the transition from vacuum to a specific elementary fermion (center of charge) and the inverse passage as terms of a binary logic.
Let us consider the set Γ = (∅, M) whose elements are projection operators respectively on the vacuum (ground state of the universal oscillator) denoted with ∅ and the elementary fermion represented by biquaternion M. We consider the structure (Γ, ·, ()) where · is a binary operation on the elements of Γ defined by the composition law:
which is the same of the conjunction “and” in conventional logic, if the Γ elements are taken as “true” and “false.” There is in addition the unary operation () defined by the law of composition:
which corresponds to negative. Naturally:
and these results can be summarized in the abstract expression (()) =, the so called Law of Crossing. Furthermore:
and these results can be summarized in the abstract expression ()·() = (), the so called Law of Calling. The structure (Γ, ·, ()) thus satisfies the Laws of Calling and Crossing as indicated by Spencer-Brown in his “Laws of Form” [8, 9]. In the language proposed by this author, the operator () is both the act of “distinguish” the element of Γ on which the operator acts (which appears inside the parenthesis) on the other (which is left “out,” as a result) and the result of such action. Physically, (∅) = M represents the creation of elementary fermion M from the vacuum, through all the acts of distinction of edges and their orientations that define it with respect to this background. The reverse operation (M) = ∅ represents the annihilation of that elementary fermion in the vacuum, which coincides with the removal of such distinctions.
The algebra of distinctions can be immediately connected to that of the (fermionic) operators which create/annihilate M on QFT states represented by the kets | 0 >, | 1 > or their conjugate bras, through a suitable definition scheme. We pose:
where λ is the number of elementary fermions created by operation (X), X ϵ Γ and λ′ is the final number of fermions after that operation. We obtain:
Let us define the operator A+ through the rather intuitive relations:
These definitions connect the concept of vacuum in the sense of ground state of universal oscillator with that of “vacuum” in the sense of QFT field associated with the elementary fermion M. It results:
Now let:
where λ is the number of elementary fermions annihilated by operation (X), X ϵ Γ and λ' is the final number of fermions after that operation. We obtain:
Let us define the operator B through the relations:
It results:
These relationships are exactly the same fulfilled from A+, when states | 0 >, | 1 > are exchanged. This is equivalent to say that B is the transpose of A+ or, in other words, that B = A. The obtained relationships can then be rewritten as:
And:
The operators A, A+ are not yet the creation/annihilation operators of QFT, although they are connected to them. The difference lies in the fact that the common QFT operators create and annihilate the centers of charge associated with the elementary fermions, while the operators introduced here create and annihilate only the biquaternion M associated with a center of charge. It is therefore necessary to integrate the insofar neglected dependence of the fermionic state on the spacetime coordinates. This can be done by introducing in the definition of A, A+ suitable factors depending on these coordinates.
First of all, we will have a multiplicity of dynamical states that, under reasonable conditions, will be labeled by an appropriate state index i = 0, 1, 2…, to which an occupation number (number of M fermions in that state) ni = 0, 1 will be related. Posing:
and:
The correct anticommutation rules between different states will be guaranteed:
Secondly, it is necessary to decompose the dependence of the state on the spacetime coordinates (that is, the wavefunction) in its Fourier components. With this choice, the creation/annihilation operators become operators acting on dynamical states represented by these components. If we redefine the operators simply multiplying them by these components:
then the square modulus of the integral on the coordinates of the scalar product of states coming in and out from an interaction vertex gives the probability of that vertex, as prescribed by the Born rule. According to the transactional reading of quantum formalism, this is the fraction of “quanta” of energy E, pulse p and spin s that mediate the causal relationship between that vertex and the vertex corresponding to the preparation of the initial state [10]. The operators redefined in this way are the QFT creation/annihilation operators of the center of charge associated with the elementary fermion M.
We remark that each Fourier component is an eigenfunction of both the energy operator iℏ∂t with eigenvalue E, and three momentum operators –iℏ∂x, –iℏ∂y, –iℏ∂z. On the quaternionic version {O} of the Minkowski spacetime the quadrimpulse is defined as (we denote here the quaternionic elements in bold font to avoid confusion with the ordinary imaginary unit i):
This operator is relevant in Dirac theory of spin particles. For centers of charge which freely propagate on spacetime (leptons), the operator γ0 P*c, with
represents the particle rest energy (this statement is the Dirac equation). For quarks within the de Sitter micro-space (4) the situation is more complex and we remand the discussion to other works [11].
Classification of Elementary Interactions
The content of the previous sections can be summarized by saying that the systematics of “particles” (base states of QFT) is constrained by the rules of “distinction” with which they emerge from a structured vacuum. It is therefore not surprising that, for the same reasons, in this scheme also the systematics of particle couplings is determined unambiguously. We will not develop a detailed theory of elementary interactions, determining their structure, calculating masses and mixing constant and so on; these more technical aspects will be presented in subsequent works. Instead, we want to expose the basic logic of the emergence of interactions, as before we have explained that of matter and spacetime.
In classifying the possible interactions, we must first distinguish those involving individual elementary fermions by those involving physical particles as a whole. As regards the former, these will be represented by interaction vertices of the type:
where this writing means that the elementary fermion represented by the biquaternion (a, b) is transformed into elementary fermion represented by biquaternion (a′, b′), with emission of the interaction quantum (r, q). Is a = a′ + r; b = b′ + q. All “crossed” versions of this process are also eligible, for example the absorption of quantum (r, q) by the fermion (a′, b′) with production of fermion (a, b); or the creation of the pair formed by fermions (a, b), (–a′, –b′) with annihilation of quantum (r, q); or the annihilation of (a, b), (–a′, –b′) with production of (r, q). The classification of interactions of this kind thus coincides with that of interaction quanta (r, q).
We can represent (r, q) as the union of (a′, b′) and (–a, –b), this latter canceling (a, b). This quantum will act or only on the external edges of the fermion glyph (weak isospin and generation), or only on its internal edges (color). The sets of distinctions which are relevant in these two cases are indeed disjoint; the relative interactional domains are therefore also disconnected.
In the first case the total color of the elements (a′, b′) and (–a, –b) must be white, and this implies b′ = b. The quantum can be represented by the not ordered pair [a′, –a] of the real parts of (a′, b′) and (–a, –b). The operation which converts a′ in –a can only be one of the two elementary trasformations acting on the external edges. The first one consists in reversing the direction of the edges, keeping their identifications as fixed. In this case a′ and –a represent the same axis taken with opposite signs and we have the three possibilities [–i, +i], [–j, +j], [–k, +k]. The second operation consists of the reconfiguration of identifications between external edges, keeping their direction as fixed. In this case a′ and –a represent two axes not necessarily equal, taken with the same sign. We have the possibilities [–i, –j], [+k, +j], and so on.
Let us consider a fermion of any color (including then leptons) whose real part, summed to an element of a pair of the first type gives a null result. The survival of the other element is equivalent to the creation of an outgoing fermion of same color and same real part. For example:
which transforms an electron into an electron. It is easily recognized in this scheme the mode of action of quanta γ (photon) and Z0. When the sum of the real part of the same fermion and an element of a pair of the second type gives a null result, the survival of the other element is equivalent to the creation of an outgoing fermion of same color and the real part of opposite sign. For examxple:
which converts an electron into a neutrino ν1, or:
which converts an electron into a neutrino ν2. It is recognized in this scheme the mode of action of quanta W+, W− and the mixing of flavors of the electroweak interaction. The electron is converted into an electronic neutrino which consists of an oscillating superposition of neutrinos of different generation and mass; an u quark is converted into a d′ quark which is a superposition of d, s, b quarks and so on.
The photon is distinct from the boson Z0 by virtue of its coupling with the electrical charge. The meaning of this physical quantity will be discussed in section Are elementary interactions a form of computation? For now we limit ourselves to remark that the electric charge Q of a given elementary fermion can be defined in terms of its biquaternion (a, b). If n is the number of color states of the internal edge associated with that fermion (1 for leptons, 3 for quarks), then the following relation holds:
where the sign plus holds for values of the expression in square parenthesis exceeding 1, otherwise the sign minus holds.
Coming back to the quantum (r, q), if it instead acts only on the internal edges of the elementary fermion glyph, then it must be r = 0 that is a′ = a. The quantum is represented by the not ordered pair [b′, –b] of the imaginary parts of (a′, b′) and (–a, –b). The only possible action on internal edges leaving glyph topology and spatiotemporal explication of its elementary fermions unchanged is the permutation of the i, j, k axes. This permutation must be cyclic if the chirality of the triad has to be preserved.
The operation which sends b′ in –b can therefore only consist of the cyclic permutation of two axes. This means that b′ and –b must be the same or different axes with opposite sign. We have then the possibilities [–i, +j], [–k, +j], and so on. The leptons are changed in themselves by the transformation [1, –1], while the colors of the quarks inside a hadron will be exchanged while maintaining the total color white. For example the process:
describes the conversion of a down red quark in a down green quark. The interaction quanta are in this case the gluons, mediators of the color interaction. As can be seen, they are very special quanta because unlike (for example) photons they do not propagate on spacetime between different interaction vertices. Instead, they are directly exchanged between two quarks within a single vertex of strong interaction. These quarks can belong to the same hadron or to different hadrons with superposed de Sitter micro-spaces.
Summarizing: the charge centers localized within a leptonic or hadronic microcosm, projected onto ordinary spacetime, are delocalized within this microcosm, more precisely within de Sitter's horizon of the tangent point-event O. In the case of leptons, such delocalization can be assumed homogeneous given the absence of interactions with other centers of charge. Basically, a homogeneous phase plane wave can be assumed in the particle rest frame, which decays to null values beyond the horizon of O. In the hadron case there are several centers of charge that can interact with each other (quarks) and their spatial delocalization must therefore be described by appropriate internal orbitals of the particle. Two centers of charge can interact electromagnetically or through weak interaction, whether they belong to the same particle or to different particles. These interactions consist in the exchange of gauge quanta γ, Z0, W+,W− that can be real or virtual. If one of these quanta is exchanged in a virtual process, then the uncertainty about the distance of its propagation is in the order of ℏ/mc, where m is the mass of the quantum.
In the hadronic case we must also consider the exchange of color between the quarks belonging to the same hadron or to different hadrons whose microuniverses have at least partially overlapping space-time projections. In this second case the interaction takes place in the overlapping region. The exchange of gluons is always virtual.
The spatial localization of a quantum in an interaction event is that of the charge centers coupled with the quantum in that event.
So far we have talked of interactions involving individual elementary fermions. There are also interactions involving the physical particle (lepton, hadron) associated with the full glyph, seen as a whole. The first of these will be the merging of several hadrons and their subsequent dissociation in other hadrons. This “hadrodynamical” interaction is the second level of what is commonly called “strong interaction,” while the first level is the color interaction inside each hadron. The more relevant distinction between the picture of hadronic processes presented in this paper and the current one is related to the relationship between a quark and the hadron it belongs to, which never is given up (although the hadron itself can change), in any stage of interaction. In fact, the glyph of a quark can only appear as a portion of a hadron glyph. The exchange of color (color permutations) between the quarks belonging to the same hadron can be described by introducing gauge quanta called gluons, but we must keep in mind that this exchange is always virtual: a gluon is emitted by a quark and absorbed by another quark of the same hadron. The gluons, therefore, never come out of the hadron microcosm in which they are exchanged (they cannot be freely emitted or absorbed). It is possible to have a gluonic exchange between quarks of different hadrons only if the projections, on ordinary spacetime, of their de Sitter microspaces admit a region of intersection; in this case the exchange can take place within this region. Naturally, even an exchange of this kind is virtual. The gluons therefore never appear as real particles (asymptotic states).
For example, the vertex np*π−* (where n, p e π fields are those of neutron, proton and pion respectively) can be seen as the emission of a gluon by a quark up inside the neutron, accompanied by the gluon decay in a up and anti-up quark pair within the neutron microspace. The newly generated pair belongs to a neutral pion with its own de Sitter microspace, at least partially superposed to that of neutron. Subsequently, the neutral pion exchanges its own up quark with a down quark of the neutron turning into a negative pion. The neutron thus becomes a proton. The hadrodynamical interaction is thus intertwined with the color interaction in a way that preserves, at each instant, the belonging of a quark to a specific hadron.
The second interaction of this category is that gravitational, linked to the gauging of the four-momentum of the global physical particle (lepton, hadron). We must note that the quadruplet [1, i, j, k] precedes, from a logical point of view, the emergence of spacetime and therefore cannot depend on the spacetime coordinates. In this sense there is a global frame of reference and “absolute” accelerations measured in it. However, Einstein taught us that it is possible to describe the gravitational field through an accessory metric. This metric defines a geometry in which the motion of an object in free fall in a gravitational field is described as free motion. From our point of view this means that it is necessary to generalize the Minkowski metric through the introduction of a connection, according to the criteria of general relativity.
Connection to the Standard Model
The reasoning outlined in the previous section leads to a description of weak and strong interactions very similar to that offered by the Standard Model (SM). In this section we expose in particular the connection to the electroweak sector of the SM Lagrangian.
We must first consider the situation in which the internal edges of the universal oscillator are defined in number and direction but its external edges are not. At this stage, the type of center of charge (lepton, anti-lepton, quark, anti-quark) is perfectly defined, but not its electric charge and weak isospin or the generation to which it belongs. Let us now forget this latter (we will address the problem of flavors mixing later in this section) and focus on a doublet of centers of charge connected by weak interaction within a single generation, for example (e−,ν1). Of course, the conclusions we come up to will be applicable to any other similar doublet, for example (u,d).
It is necessary to keep in mind that the single center of charge does not have a mass, because the mass is a feature of the particle understood as a whole (de Sitter space with centers of charge inside it), not of the single center. However, as we have seen in section Fragmentation of the void, the single center is located in the spacetime and therefore it is possible to define for it a wave function (in effect, a second quantization operator) depending on the spacetime coordinates. This topic has been dealt with in section Field operators, where field operators of centers of charge are shown to be fermionic. One can assume, based on the topics at the end of that section, that all the centers of charge have spin and this assumption is consistent with the experience. Fermions of spin without mass are described by the equation:
where Φ is the fermionic field operator. This equation admits two distinct solutions, corresponding to two separate helicity states associated with the particle and the antiparticle respectively [12]. Since the type of center of charge (quark or anti-quark, lepton or anti-lepton) is defined, the helicity is also defined. It cannot be mutated by the electroweak interaction in itself, because this latter acts on the number and direction of the external edges of the universal oscillator, while the type of center of charge (and hence the fermion helicity) is defined by the direction of the internal edges. Thus, we have two possibilities for the operator Φ: (1) it is an iso-doublet consisting of two fermionic fields of the same helicity and weak isospin opposite, or their respective anti-fields of equal helicity, opposite to the former; (2) it is a singlet of weak isospin consisting of one of the fermionic fields with helicity opposite to that of the first doublet or the anti-fermionic fields with helicity opposite to that of the second doublet. The components of each doublet are coupled by an inversion of weak isospin, while singlets are coupled by neutral currents.
We decide to call “electron” (with charge −1) and “neutrino” the fermions that in weak processes are coupled as the components of the doublet with left-hand helicity, respectively corresponding to an external edge entering or exiting the minor vertex. The possible values of Φ are therefore:
The first two values are charge-conjugate, as the third and fifth and respectively the fourth and sixth are. We note that the right-hand doublet neutrino-electron and the left-hand doublet antineutrino-positron cannot exist, neither the components of a doublet can also appear as singlets. In these cases, indeed, the rule that associates a given helicity to a fermionic field (respectively doublet or singlet) and a helicity opposite to the corresponding anti-fermionic field would be violated.
We can introduce the “weak isospin” of the left-hand pair electron-neutrino as follows:
and consequently opposite values for the right-hand positron-antineutrino pair:
The weak isospin of singlets is set to zero by definition. We have the processes:
which crossed become:
The last interaction distinguishes electron by neutrino: only external edges entering the minor vertex are coupled, with a strength Q given by (28).
Thus, there are gauge bosons derived by the fusion of two units of “weak isospin” I = . These bosons will be the following:
The first three quanta form an isospin triplet I = 1 and are associated with three fields that appear as generators of a group SU(2) of gauge transformations. The fourth forms a singlet of isospin I = 0 and is associated with a field that is the generator of a group U(1) of gauge transformations; in practice a group of local rephasements of the fermionic wave function. The iso-singlets of (30) can be coupled with themselves through A3 and B. The gauge group of the electroweak interactions is therefore the direct product SU(2) x U(1) of the SU(2) group associated with weak interactions and the U(1) group associated with electromagnetic interactions. The bosons W+, W−, Z0 and γ are therefore linear combinations of A1, A2, A3, B determined according to the conventional scheme following the gauging of Equation (29). We omit the details of the construction, which can be found in every textbook on the subject (here we conform to the notation of 13], but we underline that at this stage the gauge invariance is exact because there are no mass terms. In fact, we are talking about centers of charge, not particles. The Lagrangian at this point is the following:
where L is the left-handed iso-doublet. , where Dμ is the covariant derivative operator and the emisymmetric tensors G and F are respectively connected to B and A fields according to the usual relations.
The passage from the centers of charge to the particles involves the appearance of mass terms. The origin of these terms is a difficult topic that goes beyond the scope of this work. Note, however, that each center of charge is subject to a spatiotemporal localization within the particle to which it belongs, as we have seen in section Fragmentation of the void. When an interaction occurs during which are created, annihilated or recombined (in different particles) virtual centers of charge, to be created or annihilated are always pairs of centers with opposite quantum numbers. The spatial and temporal scale of the appearance of these pairs is limited, in accordance with the uncertainty principle, and characterized by a finite Compton length for each center of charge. In Equation (31) additional terms must therefore appear that, suitably developed, generate the coupling terms of each center of charge with itself, with a coupling constant proportional to the reciprocal of the respective Compton length. Of course, the appearance of these terms will destroy the gauge invariance.
These terms, as they relate to individual centers of charge, will not be precisely “mass terms” except in the case of particles containing a single center of charge (leptons). Instead, the hadron mass has not to be confused with the inverses (in natural units) of Compton lengths of constituent quarks.
It is possible to generate these terms by introducing couplings between a doublet of a given helicity and a singlet of opposite helicity, mediated by a Higgs field ϕ. This field plausibly represents the state of the universal oscillator when the direction of the internal edge associated with the center of charge (and hence the helicity, or the fact that the center is a fermion or an anti-fermion) has not yet been defined. Coupling with the Higgs field is therefore a dynamic description of the adynamic definition of this direction in conjunction with an electroweak interaction. From the point of view of the dynamics of this interaction, the status of the oscillator logically prior to this definition is a property of the vacuum, and in this sense the Higgs field is a property of the vacuum. Since ϕ does not have to change neither the spin nor the charge of the fields with which it is coupled, it must be a neutral scalar field. In addition, it must be an iso-doublet, because it must mediate the coupling of an iso-doublet with an iso-singlet. In general, ϕ will therefore be an iso-spinor with two complex components:
The functions ϕi(x) (i = 1, 2, 3, 4), where x is a point-event of the Minkowski space, are real; it is then possible to define the function:
We impose to ϕ the particular property of not vanish in the vacuum. This is possible if the generic point-event O is assumed to be the origin of a de Sitter micro-space of radius ℏ c/ν where v = 2M0c2. This space originates as a double fluctuation consisting of the creation and annihilation of the two sheets (past and future) of the de Sitter horizon of O. The creation (respectively, annihilation) of the future sheet involves a contribution ετ (respectively, –ετ) to the field amplitude ϕ (respectively, ) in O. The creation (respectively, annihilation) of the past sheet involves a contribution ετ′ (respectively, –ετ′) to the field amplitude ϕ (respectively, ) in O. Both the (uncorrelated) dichotomous and counterfactuals variables τ e τ′ take the values −1,+1 with equal probability ρ = . The first coupling is proportional to:
The same result is obtained for the second coupling, replacing τ with –τ′ in (34). The total coupling is therefore proportional to the product of these two half-couplings, i.e., . This leads to the following Lagrangian of the free Higgs field (σ = 0,1,2,3):
where:
and Dσ is a covariant derivative. We will return on the meaning of (36) in next section. The idea is anyway clear. At the energy scale represented by the expectation value of the Higgs field in the vacuum, each type of charge center is dissociated into virtual pairs of opposite quantum numbers, and also the de Sitter space that encloses these pairs is in a fluctuating, virtual state. This situation corresponds to the state of the universal oscillator in which the orientation of the internal edge related to that center of charge (and therefore the fact that it is a fermion or an anti-fermion) has not yet been defined. This energy scale is therefore greater than or equal to the reciprocal (in natural units) of the smaller Compton length that a center of charge can have. Below the de Sitter radius corresponding to this length, de Sitter micro-universes corresponding to elementary particles are not formed, i.e., there is no particle formation. Thus, the Higgs field represents the mentioned state of the universal oscillator at every point in space time. This makes the role played by the Higgs field in the renormalization of the standard model understandable.
The signature of the (36) is the only one compatible with the existence of minima which differ from the vacuum. We now choose a minimum ν of (36) and we pose, exploiting the gauge invariance:
where the dependence of ϕ on x is entirely contained in H. In this way an energy scale |ν| is defined which sizes the Compton lengths of centers of charge interacting with the field (37). Now we can add to (31) both the term (35) and the interactions between the Higgs and the centers of charge:
where the suffix C indicates the charge conjugation. With the usual transformations the total Lagrangian thus obtained becomes that of SM. In particular, the first term of (38) becomes the sum of a coupling term with H and a coupling term with ν. The latter takes the form:
The second term of (38) undergoes a similar transformation. The significance of the two coupling constants f becomes clear if we remark that fν/21/2 = (ℏ/λComc) where λCom is the Compton length of the center of charge. This is to say that the centers of charge (electron and neutrino respectively) “localized” in x are subjected to virtual dissociation within a typical radius λCom around x. Summing up: In the free propagation of a particle, the fermionic fields associated with its centers of charge are coupled with the expectation value in the vacuum of ϕ and this coupling generates their Compton lengths.
In section Classification of elementary interactions it has been seen that weak interactions lead to a mixing of flavors. Mixing can be taken into account by repeating the previous reasoning for each of the leptonic doublet:
While right-handed leptons form singlets. Thus we have:
where the mixing matrix U is unitary. For what concerns the left-handed quarks, they form the doublets of weak isospin:
Instead, right-handed quarks form singlets of weak isospin. The following relation then holds:
where, again, the V matrix is unitary. Determining the mixing parameters remains an open problem.
Some Reflections on the Nature of the Higgs Field
Now let us return to the nature of the Higgs field φ. It is necessary to consider that so far this field has been studied in the context of the unitary evolution of field operators. But, to our knowledge, no inquiry has ever been made about the relationship between the Higgs field and the discontinuities of that evolution, namely the quantum jumps (QJ). We can assume that actually the centers of charge inside a particle are not coupled with the field φ = φ(xμ) (μ = 0,1,2,3), but rather with the field:
Let's now explain the symbols. First, Θ(x) = 1 for x ≥ 0; Θ(x) = 0 for x < 0. The time interval between the present moment (x0) and the quantum jump to which the particle quantum state undergoes, measured in the rest frame of reference of the particle to which the centers of charge belong, is denoted as τ. It should be noted that the coordinate x0 and τ are independent variables. The parameter T is connected to the particle mass M through the relation:
We formulate this hypothesis in the context of the transactional interpretation, which sees the QJ as a simultaneous emission of the particle wave function ψ toward the future of the QJ (τ > 0) and of the conjugate wave function ψ* toward the past (τ < 0); the latter actually represents the absorbed component of the particle field [14–17]. The (44) represents the field coupled to the centers of charge associated with ψ* while (45) represents the field coupled to the centers of charge associated with ψ.
Let us now look at the physical meaning of the hypothesis. The (44) becomes ξ = φ before the jump (i.e., for τ < 0), while ξ = φexp(–τ/T) after the jump (τ > 0). For (45) we have instead the mirror situation ξ = φexp(τ/T) for τ < 0, while ξ = φ for τ > 0. Therefore, in conclusion, the coupling of the centers of charge with ξ coincides with the usual one with φ, except for the appearance of exponential tails of ξ of duration ≈ T around τ = 0. In the first tail, related to the absorbed wave function ψ*, the coupling vanishes and the centers return to the “original” condition of gauge invariance. Instead, this coupling is reset in the second tail relative to the emitted wave function ψ, starting from the gauge invariance condition. The interested reader can consult Chiatti [18] for a possible theoretical justification of these tails.
From this it is understood that the Higgs field φ, autonomous and independent of the specific particle, is in fact the limit case of the field ξ dependent on the specific particle. The tails of ξ in correspondence with a discontinuity in the evolution of the quantum state of the particle are the particle, which is actually localized on the temporal domain. This field manifests itself when a massive particle undergoes a quantum jump. Operating with the energy operator on (44) and on (45) respectively we find:
The “absorbed” energy –iℏ/T and the “emitted” energy +iℏ/T add up to zero, as it must be given the impossibility of net exchanges of energy with the vacuum. They are imaginary, and thus define the line width of a transient consisting of the particle that contains the centers of charge. This result conforms to the notion of particles as events rather than objects. The localization energy Mc2 is conveyed by ψ* and ψ respectively, and is exchanged with the vacuum as described elsewhere [3].
From what we have said there exist, for τ > 0, centers of charge associated with the asymptotic state ψ regularly coupled with φ as well as centers of charge associated with ψ* whose coupling with φ is evanescent. For τ < 0 there is a mirror situation with centers of charge associated with the asymptotic state ψ* regularly coupled with φ together with centers of charge associated with ψ whose coupling with φ is evanescent. Of course, the net charge is conveyed by asymptotic states so that the total charge of the centers of charge associated with tails must be zero for each value of τ. The charges whose coupling with φ is evanescent are therefore the virtual ones that in the QFT description dress the net charges conveyed by the asymptotic states. With this we have that the perturbative effects are limited in this description to a range of extension ≈ T around τ = 0 and do not affect the asymptotic states (if these latter are free). In other words, this description seems to correspond approximately to QFT after performing the renormalization procedure, with the consequent subtraction of free propagation diagrams. As can be seen, the coupling described by (44), (45) represents at the same time: (1) the localization of the particle in the temporal domain; (2) the self-interaction of the particle induced by its real interaction with the external world (the same that, inter alia, causes the QJ); (3) the coupling of the centers of charge inside the particle with the Higgs field.
In the case of particles containing a single center of charge (leptons) the coupling constant with the Higgs field, multiplied by the expectation value in the vacuum of this latter, is the mass of the particle while the time constant T of (44), (45) is the reciprocal of that mass. In the case of quarks, the first quantity is the reciprocal (in natural units) of the Compton length of the quark while T, which is the same for all quarks of the same hadron, is the reciprocal of the hadron mass. All these considerations assume a free asymptotic state ψ, although this restriction is not necessary. The asymptotic state can also contain virtual interactions, such as in the case of an electron in a stationary atomic orbital, which exchanges virtual photons with the nucleus.
At this point we can come back to the initial question of this section, that is, the true nature of the Higgs field. The Higgs field exists because there are fluctuations in the vacuum (corresponding to the state of the universal oscillator with undefined orientation of the internal edge) and suppressing these fluctuations costs energy. The centers of charge inside a particle are coupled, in the time neighborhood of a QJ, with this fluctuations field. The coupling energy is given by the product of the coupling constant for the expectation value of the Higgs field in the vacuum. This product is the reciprocal of the Compton length of the center of charge. The suppression/reset of the coupling is manifested in the form of exponential tails in (44), (45), and in the actualization of an asymptotic state for the particle that contains conserved centers of charge. This actualization is the creation of the charges; the decay constant of this process is the reciprocal of the mass of the particle. When an energy equal to that of coupling between the centers of charge inside a particle and the expectation value of the Higgs field is made available in the QJ, that particle may appear as a virtual transient phenomenon; its actual creation requires that energy equal to the mass of the particle is available. The energy ν of the fluctuations of the Higgs field is the reciprocal, in natural units, of the shortest Compton length of the pair constituted by a center of charge and its anti-center. This “limit” Compton length will then be the smallest value attributable to the radius of the de Sitter space associated with the localization of a particle.
The center of charge with the shortest Compton length is the quark top; the reciprocal of its Compton length amounts to M0 ≈170 GeV and the double of this value is 340 GeV. In accordance with our interpretation, the value of ν is estimated to be 346 GeV. It is also noteworthy that the quark top does not form hadrons, which support the hypothesis that it is placed precisely at the extreme limit beyond which the particle formation is no longer possible (or very difficult).
Are Elementary Interactions a Form of Computation?
In this section we intend to explain, before going on to the conclusions, some reflections on the role played by the algebraic structures in the present approach. To our best knowledge, the peculiarity of this role distinguishes our proposal from other algebraic models, available in literature, also aimed at the theoretical justification of the Standard Model. In particular we want to briefly discuss some correspondences and differences with the recent (and fascinating) works of Furey [19, 20, 21, 22] and Stoica [23]. It is first necessary to consider that the questions explicitly formulated by both these authors as motivations for their research are the same ones that we have set ourselves and which we have illustrated in the introductory part of this work. These questions can be summarized in one: is there a general theoretical principle that explains why the elementary particles that we see in nature are those that are, and interact with the modalities we actually observe? Furey and Stoica both answer affirmatively, as we do. Like us, even these authors hypothesize the existence of an algebraic constraint that selects the field operators of the Standard Model. The identification of this constraint thus becomes the fundamental goal of the research.
While these intentions are common to the two approaches (ours and those of these authors), an important difference must be found in the understanding of the constraint. Furey and Stoica assume that the Standard Model can be formulated in terms of a Clifford algebra on a numerical field representative of a division algebra (real numbers, complex numbers, quaternions or octonions) or on direct products of such fields. The operators of the Standard Model then become elements of this Clifford algebra, more precisely ideals, and this fact represents the constraint sought. Both Furey and Stoica show that a complex Clifford algebra Cl(6) can accommodate a single generation of elementary fermions (quarks and leptons) of the Standard Model. Furey goes further, succeeding in demonstrating: (1) that the ideals of an algebraic structure built on the chains of multiplications between octonions can be put in correspondence with the three generations of elementary fermions [21]; (2) that the ideals of a complex quaternionic algebra can be put in correspondence both with Dirac spinors and with spacetime quadrivectors [19, 22].
One may ask what is the physical reason which privileges such algebraic structures. Field operators are used to describe interactions, so it seems plausible that logical constraints on their algebra derive from the nature of interactions. In this regard Furey makes interesting considerations [19]. Her proposal is to interpret interactions as algebraic operations, emphasizing how both are irreversible. There is no way to uniquely return to the addends 3 and 2 from the result 5 of their sum, as there is no way to restore the wave function immediately after its collapse. The physical world, understood as a network of interaction events, is then a locally limited graph provided with a partial order relation, i.e., a causal set. The nodes of this graph are operations and the flow on the graph takes place in accordance with the rules of a specific algebra.
Our idea is similar, in the sense that the vertices of interaction are our starting point. However, there are three important differences compared to the Furey proposal:
(1) We consider the lesson of non-locality as relevant and assume that this concept has a far wider scope than the entanglement that is, we think, a mere consequence. Our algebraic structure constitutes an aspatial and timeless background, which connects to the spatio-temporal domain in discrete events that are the vertices of interaction. Real interactions are considered here, corresponding to the collapse of the quantum wave function. The interactions therefore have a double dynamic role (due to their influence on the unitary evolution of the wave function) and adynamic (collapse).
(2) The connection between the atemporal structure and the temporal domain does not end with a single algebraic operation, but consists in a succession of operations of distinction (in the sense of Spencer-Brown) on the elements of the structure.
(3) The structure is selected by its self-duality (section Fragmentation of the void), which is seen as synonymous with the condition of maximum elementarity (vacuum). The particles come out of this condition, or return into it, in conjunction with an interaction.
This difference between the starting points of our work and those of Furey and Stoica immediately leads to a difference in formalism. The algebraic structure considered in section Directed glyphs and hypercomplex numbers for the description of matter is in fact constituted by biquaternionic units, not by quaternions in a general sense. No multiplication of these units for a real number is defined, nor any addition operation (we only use the weaker notion of cancellation of opposite units in section Conclusions). The considered algebraic structure is therefore drastically simpler than those examined by Furey and Stoica, because no numerical field is present. The original quaternionic units from which this “material” structure is defined can be described as the vertices of a spherical triangle. Their relations remain the same regardless of the choice of the arrangement of the triad of vertices on the sphere, and the value of the spherical radius. This allows the definition of a spatio-temporal order co-emergent with the matter (section Fragmentation of the void).
To each particle involved in a real interaction event, described in quantum mechanics by the collapse of the wave function, corresponds to a succession of algebraic operations that leads to the spatio-temporal manifestation of that particle, with its centers of charge. These centers are the elementary fermions of the Standard Model. These operations, carried out in succession, cause the universal oscillator to pass from the symmetrical vacuum state to that corresponding to the specific elementary particle. Quantum amplitudes can be associated with these transitions (section Field operators), which are connected to a vacuum state through Fock operators and it is only at this stage that the addition appears as requested by the superposition principle. Superpositions of amplitudes can be used to represent the quantum amplitude of the process before or after collapse.
It is the classification of field operators obtained in this way that can be compared with those derived from other approaches. In other words, the symmetry breaking associated with the collapse of the wave function occurs at a level that is not that of QFT and it leads to the base states of the QFT description; it is only at this point that the superposition of these latter is introduced, corresponding to the quantum (second quantization) amplitude of the QFT description. This is a different procedure from the usual one consisting in starting with a QFT state provided with a certain symmetry and then selecting base states from a process, also inside the QFT, of symmetry breaking. As for the superposition principle, it can be simply postulated, as is usual in quantum theory. In our opinion it can be justified on the basis of the transactional approach (section Field operators).
We note that the field operators so defined belong to the conventional formalism based on spinors, and it does not seem necessary to resort to more esoteric numerical fields such as quaternions or octonions. The vacuum state is here, by construction, the authentic state of absence of particles and not the lowest eigenvalue state as in the construction of Furey [19, 20]. A further difference from the work of Furey and Stoica is that the present approach leads to quarks correctly assembled into mesons, baryons and anti-baryons which are the actual asymptotic states emerging from (or entering) a vertex of interaction, while an approach based on a algebra Cl(6) or similar is necessarily limited to centers of charge (quarks or leptons).
Having emphasized the differences, we now see the convergences. In our approach the vertices of interaction are localizations of certain packets of physical quantities on spacetime; these packages are the particles. The interactions are therefore distinguished by the type of quantities that localize. For example, the photon γ localizes the electric charge, the Z0 the weak charge. In [1, 2] it has been seen how each center of charge (lepton or quark) corresponds to a “first type bootstrap graph.” The connection between these graphs and the glyphs is described in [2]. If we omit, in this description, the fermionic generation, each graph corresponds to an ordered triple of three eigenvalues σ(i) = ± 1, i = 1,2,3. The graphs are then graded as follows:
Of consequence:
1) The Z0 couples with every σ(i) localizing it in time domain;
2) The W± couples with every σ(i) localizing it in time domain and inverting it;
3) The γ couples with every σ(i) = +1, localizing it in time domain and distinguishing it from σ(i) = −1.
The weak charge of a center of charge is then:
The electric charge is instead:
The different gauge quanta of electroweak interaction correspond respectively to the localization of the σ(i), the distinction of the two signs of the σ(i) and the inversion of these signs. The gradation of the centers of charge corresponds to the left ideals of the algebra Cl(6), as evidenced by Furey [19, 20]. In particular, by applying the principle of quantum superposition to color states, the same multiplets of the SU(3) group considered in [19] are obtained. The singlet and the antisinglet correspond to the two leptons, while the two triplets correspond to the two quarks. The electric charge operator (50) is invariant for color permutations and is the operator number of positive σ(i), consistently with Furey's results. However, unlike Furey's work, the three generations are obtained through the real part of the biquaternion associated with each center of charge, without recourse to the octonions. In particular, it does not seem necessary to assume the non-commutativity of the “ordinary” imaginary unity with the other units other than 1.
Glyphs can be interpreted in terms of the logic of Peirce's relations [2]; the correspondence between this logic and Clifford's algebras is shown in [24]. However, the fact that our approach is structured on two levels, one timeless and the other diachronic, leads to greater caution in the modeling of physical phenomena through algebras. If it seems plausible to model the atemporal level using an abstract algebraic structure, the transition to the diachronic level (the only experimentally accessible in a direct way) could correspond to the choice of a specific representation of this structure by nature. If this were the case, quantum theories could have algebraic ancestors, but they would assume the necessary relational meaning only if they were formulated in that representation. A strict test for every algebraic approach is represented by the calculation of the mixing angles, because the setting of such a calculation depends on the level (dynamic or timeless) to which the superposition principle appears. While, to our knowledge, nothing has been done for the mixing of quark flavors and neutrino oscillations, Stoica has derived a good estimate of the Weinberg angle [23]. His calculation is based on an algebraic structure directly implanted on QFT operators; the duplication of this result is therefore an open problem for our approach.
Finally, we point out that the five questions of the “binary system” considered by Furey [19] are the glyph edges, and the binary answers to these questions are the directions assigned to these edges (with the difference that the sign of the electric charge is replaced, in the Furey system, by the couple weak isospin + helicity). The same information is coded in before mentioned “first type bootstrap graphs.”
Conclusions
In this work we have attempted to analyse what occurs when an elementary particle is localized in spacetime in correspondence of an interaction vertex, following its coupling with other particles. The most important results, in our opinion, are those reported in sections Field operators and Classification of elementary interactions. We hypothesize that the localization of a particle (and the centers of charge inside it) in spacetime domain can be described in three successive stages starting from an algebraic background whose elements are balanced with their own opposite. This background therefore represents a “vacuum” not associated with a definite spacetime position; it represents rather a condition of delocalization over the entire spacetime. The spacetime localization induced by an interaction corresponds to a second stage in the course of which the balance is disrupted; the retarded and advanced time components of the background separate, while only one of the two spatial structures included in it is selected by the interaction: the triadic or the anti-triadic one. An asymptotic state appears that corresponds to a lepton, meson or baryon in one case; to an antilepton, meson or antibaryon in the other one. It is at this stage that the color associated with the individual centers of charge appears. In the third stage of the process, which by now concerns the individual center of charge, the interaction selects the weak isospin of the center and the generation to which it belongs. The center will therefore appear as a lepton or antilepton if its color is white; otherwise it will appear as a quark.
The entire process of manifestation (or de-manifestation) of a particle and its centers of charge can be represented in a natural way by means of a specific graph called glyph. All the glyphs associated with the different particles can be derived from a single graph, the universal oscillator, through distinctions or identifications of the edges of this latter. In this sense it can be said that the different elementary particles that appear as asymptotic states of the interaction (leptons, mesons, baryons) are “states” or “excitations” of the universal oscillator. These excitations can be represented as QFT operators of creation or annihilation of the elementary fermions that constitute the centers of charge of each particle. This result links up the description suggested here with the conventional QFT formalism with the advantage that QFT base states are inferred from first principles, instead that merely postulated. Also the systematics of possible asymptotic states (“particles”) is consequently constrained.
While the excitations of the universal oscillator represent the various particles, the transitions of the oscillator from one state to another represent the interactions between particles; these, too, therefore can be classified. The picture that emerges reproduces various essential aspects of the Standard Model, including the yet mysterious mixing of flavors in weak interaction. The essential addition to the Standard Model concerns the strong interaction: during the interaction the single quark can change the hadron it belongs to, but it always belongs to a well-defined hadron. The membership of quarks consists of their participation to the glyph of the same hadron. This relation cannot be mapped with the usual concept of “interaction.” In Bohmian terms [25, 26] we can say that the color degree of freedom belongs to an explication level of physical reality other than -for example- that of the electroweak interaction. Although these arguments are developed elsewhere [11, 18], it is interesting to note that in this approach the QCD description can be anyway recovered, with the unexpected gift of a solution for classical problems of hadronization and confinement. From this point of view the concept of de Sitter micro-space associated with elementary particles, with a radius sized by the “chronon,” is of particular relevance. It refers to a whole literature concerning precisely the structure of the strong interaction and the quark confinement [27–31]. A geometric view of the Higgs field [32] reconnects the peculiarities of this field to the time localization of the particles and the centers of charge contained therein. In subsequent work will be examined more appropriately the dynamical aspects of particle structure implied by this approach and those associated with the particle mass spectrum.
Author Contributions
The author confirms being the sole contributor of this work and approved it for publication.
Conflict of Interest Statement
The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
The handling editor is currently co-organizing a Research Topic with the author LC, and confirms the absence of any other collaboration.
References
1. Chiatti L. Bootstrapping the QFT, a new road to the elementary particles spectrum. El J Theor Phys. (2013) 9:33–48.
3. Licata I, Chiatti L. Timeless Approach to Quantum Jumps. Quanta (2015) 4:10–26 doi: 10.12743/quanta.v4i1.31
4. Moschella U. The de Sitter and anti-de Sitter sightseeing tour. In: Damour T, Darrigol O, Duplantier B, Rivesseau V. editors. Einstein 1905-2005; Progress in Mathematical Physics Vol. 47. Berlin: Springer (2006). p. 120–133. doi: 10.1007/3-7643-7436-5_4
5. Arcidiacono G. L'universo di de Sitter, il gruppo di Fantappié e la cosmologia del Big-Bang. Collectanea Mathematica (1988) 39:55–65. (in Italian).
9. Kauffman LH, Varela F. Form dynamics. J. Soc Biol Struct. (1980) 3:171–206. doi: 10.1016/0140-1750(80)90008-1
11. Chiatti L, Licata I. Particle model from quantum foundations. Quant Stud Math Found. (2017) 4:181–204 doi: 10.1007/s40509-016-0094-6
12. Landau LD, Lifsits EM. Relativistic Quantum Theory (Theoretical Physics Course vol. IV) MIR. Moscow:SSSR (1973).
14. Cramer JG. Generalized absorber theory and the Einstein-Podolsky-Rosen paradox. Phys Rev D (1980) 22:362–76. doi: 10.1103/PhysRevD.22.362
15. Cramer JG. The transactional interpretation of quantum mechanics. Rev Mod Phys. (1986) 58:647–87. doi: 10.1103/RevModPhys.58.647
16. Cramer JG. The transactional interpretation of quantum mechanics and quantum nonlocality. arXiv [Preprint] (2015). arXiv:1503.0(0039) [quant-ph].
17. Kastner RE. The Transactional Interpretation of Quantum Mechanics: The Reality of Possibility. Cambridge:Cambridge University Press (2013).
18. Chiatti L. Quantum jumps and electrodynamical description. Intern J Quant Found. (2017) 3:100–18.
20. Furey C. Charge quantization from a number operator. Phys Lett B (2015) 742:195–9. doi: 10.1016/j.physletb.2015.01.023
24. Beil RG. Peirce, Clifford and Dirac. Int Journ Theor Phys. (2004) 43:1301–15. doi: 10.1023/B:IJTP.0000048616.72226.3a
26. Bohm D. Quantum theory as an indication of a new order in physics B; Implicate and explicate order in physical law. Found Phys. (1973) 3:139–68 doi: 10.1007/BF00708436
27. Motoyoshi A. On the mass spectrum of the extended hadrons. Lett Nuovo Cim. (1985) 44:379–82. doi: 10.1007/BF02746699
29. Recami E. Multi-verses, micro-universes and elementary particles (hadrons). arXiv [Preprint] (2005). arXiv:physics/0505149.
30. Recami E, Castorin P. On quark confinement: hadrons as “strong black holes.” Lett Nuovo Cim. (1976) 15:347–50. doi: 10.1007/BF02725894
31. Maltz J. de Sitter Harmonies: Cosmological Spacetimes as Resonances. Phys. Rev. D (2017) 95:066006 doi: 10.1103/PhysRevD.95.066006
Keywords: elementary particles, quantum field theory, origin of time, emerging matter, foundations of quantum physics
Citation: Chiatti L (2018) Thinking Non Locally: The Atemporal Roots of Particle Physics. Front. Phys. 6:95. doi: 10.3389/fphy.2018.00095
Received: 02 December 2017; Accepted: 10 August 2018;
Published: 31 August 2018.
Edited by:
George Jaroszkiewicz, Independent Researcher, Walton on the Wolds, United KingdomReviewed by:
Ignazio Licata, ISEM Institute for Scientific Methodology, ItalyFrancesco Caravelli, Los Alamos National Laboratory (DOE), United States
Christian Corda, Istituto Universitario di Ricerca Scientifica Santa Rita, Italy
Copyright © 2018 Chiatti. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Leonardo Chiatti, bGVvbmFyZG8uY2hpYXR0aUBhc2wudnQuaXQ=
 Leonardo Chiatti
Leonardo Chiatti