- Department of Behavioural Ecology and Evolutionary Genetics, Max Planck Institute for Ornithology, Seewiesen, Germany
Introduction: Conflict Over Care and Conditional Cooperation
In species with biparental care, conflict arises because each parent would be better off if it could reduce its share in offspring care and leave the greater share of care to their partner (Trivers, 1972; Lessells, 2012). Understanding how this conflict is resolved has been a major focus in research on social evolution (see Harrison et al., 2009). Traditional theoretical studies on how individuals should optimize their provisioning effort predict that parents should respond to changes in the provisioning effort of their partner by changing their own effort to a lesser extent in the opposite direction (Houston and Davies, 1985; McNamara et al., 1999; Lessells and McNamara, 2012). Parents should thus partially compensate for reduction in care by their partner, which leads to inefficient outcomes, because parents will care less than they would in the absence of conflict. Although some empirical studies provided support for these predictions, others reported results that do not match the predictions of these models (Harrison et al., 2009). Johnstone et al. (2014) therefore proposed an alternative model that suggests that individuals may benefit from adopting a strategy of “conditional cooperation,” reducing their provisioning effort if they were the last to feed the offspring and increasing their provisioning effort if their partner was the last to feed. Using a game theory model, they show that such a strategy is evolutionarily stable and that it leads to more efficient outcomes than the strategies proposed by the previous models. If backed up by empirical evidence, the conditional cooperation model would entail a major shift in our understanding of sexual conflict over care, because it implies that sexual conflict does not necessarily negatively impact offspring care.
Testing Conditional Cooperation: More Alternation Than Chance?
The conditional cooperation model predicts that parents take turns in provisioning their offspring, because they reduce their effort after each feeding visit and increase it again after their partner visited. Consequently, empirical studies examined whether parents that are provisioning their brood indeed take turns more often than expected by chance (Bebbington and Hatchwell, 2016; Koenig and Walters, 2016; Iserbyt et al., 2017; Savage et al., 2017; Leniowski and Wegrzyn, 2018). Almost all these studies report that the degree of alternation was higher than expected by chance and conclude that parents adjust their provisioning effort to one another. However, before concluding that parents habitually adjust their provisioning behavior to one another and that conditional cooperation is a widespread phenomenon, we need to take a closer look at the validity of the empirical approach. The conclusion that turn taking is higher than expected by chance critically depends on the way in which “chance” levels of turn taking are determined. How, then, should we determine the amount expected by chance? As we will argue, this is not a simple matter.
Several studies calculated “chance” levels of alternation by simply randomizing the order in which visits took place (Koenig and Walters, 2016; Iserbyt et al., 2017; Leniowski and Wegrzyn, 2018). A similar approach is the runs test which examines whether the order in which nest visits take place differs from randomness (Khwaja et al., 2017; see also Johnstone et al., 2014; Savage et al., 2017). A problem with these approaches, however, is that they ignore the fact that a substantial degree of alternation may arise simply from the manner in which individuals provision. Each individual needs a certain amount of time after every nest visit during which it cannot visit the nest again (“refractory period”): it needs to move away from the nest, find food and return to the nest. Clearly, a nest visit is then more likely to be followed by a visit of the other parent than by another visit of the same parent. If parents engage in little else than feeding and often need a similar amount of time to find food, a pattern of turn taking will inevitably emerge, even when each parent's provisioning behavior is entirely independent of that of the other parent. Even high levels of alternation per se are not sufficient to conclude that individuals engage in conditional cooperation, because this may arise simply when each parent is consistent in the time between visits (inter-visit interval). What is needed is a test of whether parents take turns more often than expected by chance that takes into account the distribution of the inter-visit intervals of each parent.
To do this, some studies have used a more refined approach and calculated chance levels of alternation by randomizing not the order of the nest visits per se, but the inter-visit intervals of the nest visits of each parent (Johnstone et al., 2014; Bebbington and Hatchwell, 2016; Savage et al., 2017). In this way, the distribution of the actual inter-visit intervals is preserved, thereby taking into account that nest visits take a certain amount of time, while any pattern in the visit sequence that results from parents responding to each other's behavior is removed. The three studies that used this approach found that the alternation rate of the actual visit sequence was higher than that of the randomized visit sequences, and hence concluded that parents took turns more often than expected by chance (Johnstone et al., 2014; Bebbington and Hatchwell, 2016; Savage et al., 2017). Is this proof that parents actively respond to the provisioning behavior of their partner? Whilst this approach is more sophisticated than the methods described above and circumvents the problem of the refractory period, the method to calculate chance levels of turn-taking remains problematic. Here is why.
The Problem With Randomizing Inter-Visit Intervals
It is true that the re-ordering of inter-visit intervals removes any pattern in the visit sequence that results from parents responding to each other's behavior. However, it also takes away any correlation between male and female visit behavior that results from both parents responding to the same external stimuli (Schlicht et al., 2016). The “chance” scenario against which the observational data are compared is thus not only stripped of the effect that parents may have on each other (necessary to test for conditional cooperation), but also from any other factors that may have introduced a correlation between the inter-visit intervals of both parents. Below, we use two simple examples to illustrate that this will lead to a higher level of turn-taking in the observed than in the randomized sequence, even in the absence of parents responding to each other.
Imagine a nest in which parents provision independent of each other's behavior under constant conditions. Randomization of the inter-visit intervals will not affect the level of turn taking (Figure 1A). Now imagine the same scenario, but where both parents gradually increase the amount of time between visits, for instance because of worsening weather conditions. In this case, randomization of the inter-visit intervals from the entire observation period will lead to a reduced level of turn taking (Figure 1B). Such a specific situation of a gradual change can be controlled for (Johnstone et al., 2016; Savage et al., 2017), but there is a multitude of factors that can influence the inter-visit intervals of both parents and this may be both suddenly or gradually, for brief or longer periods, and in both directions (increasing or decreasing). For instance, imagine a situation where the presence of a predator during part of the observation period causes both parents to temporarily increase the amount of time between visits. Randomization of the inter-visit intervals from the entire observation period will again lead to a reduced level of turn taking (Figure 1C).
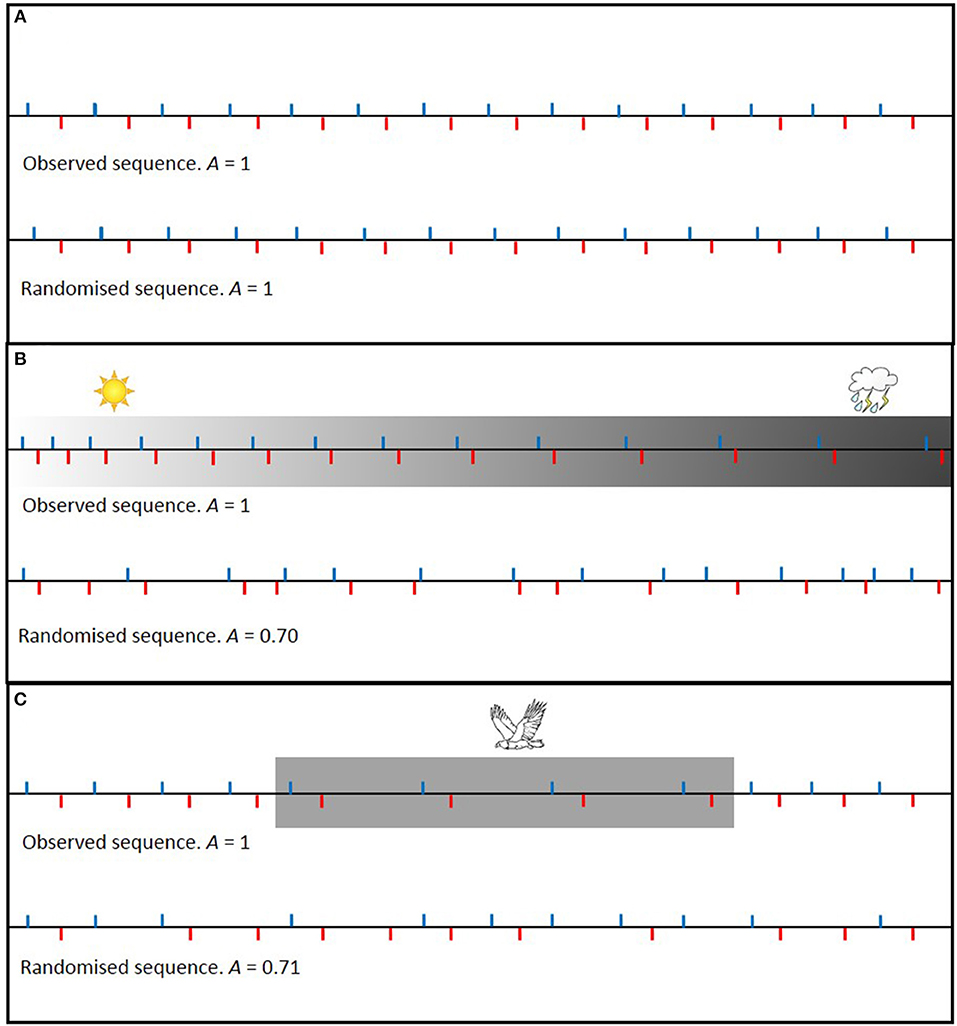
Figure 1. Schematic examples of how randomizing the order of inter-visit intervals affects the level of alternation (A). A = F/(t − 1), where F is the number of visits that was followed by a visit of the other parent and t is the total number of visits during the observation period (Bebbington and Hatchwell, 2016). Blue ticks indicate a nest visit by parent 1 and red ticks indicate a nest visit by parent 2. For the purpose of illustration, we assume that both parents make the same number of nest visits during the observation period and that the amount of time needed between visits is the same for every feeding trip (unless affected by external stimuli). In (A) visit rates do not change over time and randomization does not affect the level of alternation. In (B,C) however, inter-visit intervals of both parents change in the same way over time, e.g., due to deteriorating weather conditions or due to the presence of a predator, and randomization of the inter-visit intervals leads to a reduced level of alternation.
In the simple examples illustrated in Figure 1 inter-visit intervals are fixed and pair members share the feeding equally for the sake of simplicity. When inter-visit intervals are more variable or when parents differ in their provisioning effort, more “noise” is introduced, but the same principle applies. To demonstrate this, we simulated visit sequences that differed in the degree of both within- and between-parent variation in inter-visit intervals (Figure 2). We then simulated the presence of an external stimulus (e.g., predator) during the middle part of the sequence that doubled the median length of inter-visit intervals for both parents (as per Figure 1C). We calculated the alternation levels for the simulated sequences, as well as for the randomized simulated sequences. The results of these simulations—in which the presence of active turn taking was explicitly excluded—show that the level of alternation is higher in the original sequence than in the randomized sequence for all values of within- and between-parent variation in inter-visit intervals. The difference is large when both parents are investing equally and individual variation is low, and decreases with increasing differences in parental investment and with increasing individual variation. Nevertheless, even in the extreme scenario where one parent visits three times as much as the other one and within-individual variation is large, the level of alternation in the observed sequence is higher than that in the randomized sequence. Thus, when parents adjust their provisioning effort in the same way in response to external stimuli, the level of alternation of the actual nest visit sequence will be higher than that of the randomized sequence in many cases.
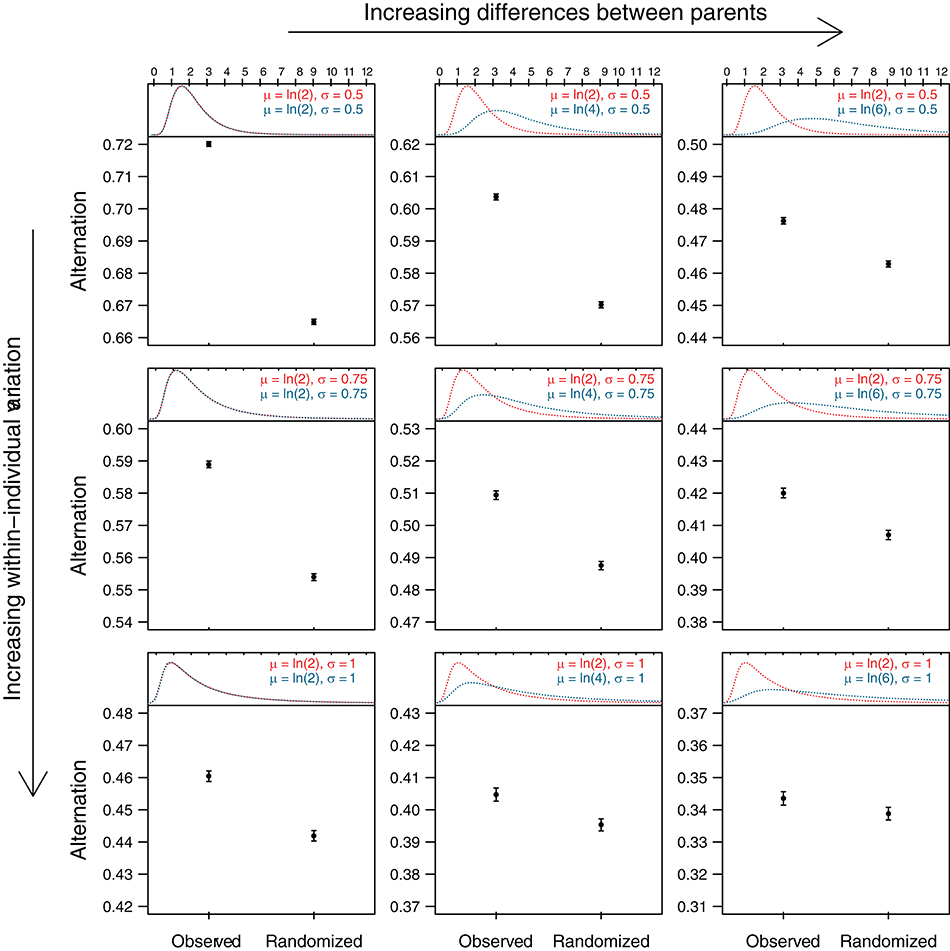
Figure 2. Observed and randomized levels of alternation for simulated sequences with different levels of within- and between-parent variation in inter-visit intervals (IVI). We simulated feeding sequences for pairs of provisioning parents where IVIs of each parent were drawn from a log-normal distribution with parameters μ and σ (i.e., the natural logarithm of the variable is normally distributed with mean μ and standard deviation σ). The median IVI (eμ) of one parent could be either the same, twice as much, or three times as much as that of the other parent, and σ of either parent could be 0.5, 0.75, or 1. The top part of each panel shows the resulting distributions with the IVI (in minutes) on x-axis. To simulate the effect of an external stimulus that increased IVI's (e.g., a predator), we created for each combination of within- and between-parent variation another set of IVI's in which the median was increased by a factor of two (distributions not shown). Feeding sequences of 120 min were then constructed, in which the first and last 30 min were randomly drawn (for each parent separately) from the original simulated IVI's, while the middle 60 min were drawn from the simulated IVI's that reflected the presence of a predator [as per scenario (C) in Figure 1]. We then generated 1,000 such sequences for each combination of within- and between-parent variation and calculated the level of alternation (see legend of Figure 1) before and after randomization. The lower part of each panel shows means and standard errors of these “observed” and “randomized” alternation levels. Code for data generation is provided in the Supplementary Materials.
The presence of factors that affect the provisioning behavior of both parents in the same way is not just a hypothetical possibility. There is ample evidence that males and females often respond in a similar manner to external stimuli. Examples include chick begging levels, presence of predators, presence of ephemeral food sources, weather conditions, and the time of day (Kilner and Johnstone, 1997; Budden and Wright, 2001; Geiser et al., 2008; Low et al., 2008; Zanette et al., 2011; García-Navas and Sanz, 2012; LaManna and Martin, 2016), but there may be a multitude of other factors that influence the provisioning behavior of both parents. Some studies have taken into account several of these factors and controlled for them statistically (Johnstone et al., 2014). However, if any such factor(s) remain uncontrolled for, the conclusion that parents alternated their visits more than expected by chance and must therefore have responded to each other's behavior is unsound. When an observed visit sequence has a higher level of alternation than the randomized sequence, it is simply not possible to establish whether this is because parents responded to each other or because they responded to the same external stimuli. Given the extensive evidence that the latter is commonplace, this should probably be regarded as the more parsimonious explanation.
Conclusions and Future Directions
Unless all factors that affect both male and female feeding effort are known and controlled for, either statistically or experimentally, it will be challenging – indeed, perhaps impossible – to establish whether individuals take turns more often than expected by chance based on observational data alone. We therefore tentatively conclude that the answer to the question in the title probably ought to be “not much.” However, we believe there is ample scope for advances in this field if more rigorous tests are implemented. One possibility is to manipulate the provisioning effort of one parent and monitor whether the other parent adjusts its pattern of provisioning accordingly (e.g., Hinde, 2006). In such experiments, however, care should be taken that the hunger level of the offspring is not affected by the treatment, because this would influence the behavior of the parents and confound the interpretation of the results. This may be achieved by manipulating provisioning effort on the scale of single nest visits, e.g., by playing back extra begging calls to a parent during a single nest visit to reduce its subsequent inter-visit interval (Santema et al., 2017). The prediction would be that a faster return to the nest by the manipulated parent should lead to a faster return to the nest by the other, unmanipulated, parent. Another possibility is to manipulate the information that parents have about the provisioning effort of their partner (e.g., Iserbyt et al., 2015). Clever experimental designs in captivity or in the wild may be able to achieve this. For instance, the extent to which pair members can observe each other's provisoning effort could be manipulated. We believe that experiments of this kind are needed to move this field forward; only more discriminatory tests will tell us whether conditional cooperation really plays a role in the family life of birds and other animals.
Author Contributions
All authors contributed critically to the ideas presented in this paper. ES performed the simulations with input from PS and BK. PS wrote the paper with extensive input from ES and BK. All authors approved it for publication.
Funding
This work was supported by the Max Planck Society.
Conflict of Interest Statement
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Supplementary Material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fevo.2019.00094/full#supplementary-material
References
Bebbington, K., and Hatchwell, B. J. (2016). Coordinated parental provisioning is related to feeding rate and reproductive success in a songbird. Behav. Ecol. 27, 652–659. doi: 10.1093/beheco/arv198
Budden, A. E., and Wright, J. (2001). Begging in nestling birds. Curr. Ornithol. 16, 83–118. doi: 10.1007/978-1-4615-1211-0_2
García-Navas, V., and Sanz, J. J. (2012). Environmental and within-nest factors influencing nestling-feeding patterns of Mediterranean blue tits (Cyanistes caeruleus). Condor 114, 612–621. doi: 10.1525/cond.2012.110120
Geiser, S., Arlettaz, R., and Schaub, M. (2008). Impact of weather variation on feeding behaviour, nestling growth and brood survival in wrynecks, Jynx torquilla. J. Ornithol. 149, 597–606. doi: 10.1007/s10336-008-0305-y
Harrison, F., Barta, Z., Cuthill, I., and Szekely, T. (2009). How is sexual conflict over parental care resolved? A meta-analysis. J. Evol. Biol. 22, 1800–1812. doi: 10.1111/j.1420-9101.2009.01792.x
Hinde, C. A. (2006). Negotiation over offspring care? A positive response to partner-provisioning rate in great tits. Behav. Ecol. 17, 6–12. doi: 10.1093/beheco/ari092
Houston, A. I., and Davies, N. B. (1985). “The evolution of cooperation and life-history in the dunnock,” in Behavioural Ecology, eds R. M. Sibly and R. H. Smith, (Oxford: Blackwell Scientific), 471–487.
Iserbyt, A., Farrell, S., Eens, M., and Müller, W. (2015). Sex-specific negotiation rules in a costly conflict over parental care. Anim. Behav. 100, 52–58. doi: 10.1016/j.anbehav.2014.11.014
Iserbyt, A., Fresneau, N., Kortenhoff, T., Eens, M., and Müller, W. (2017). Decreasing parental task specialization promotes conditional cooperation. Sci. Rep. 7:6565. doi: 10.1038/s41598-017-06667-1
Johnstone, R. A., Manica, A., Fayet, A. L., Stoddard, M. C., Rodriguez-Gironés, M. A., and Hinde, C. A. (2014). Reciprocity and conditional cooperation between great tit parents. Behav. Ecol. 25, 216–222. doi: 10.1093/beheco/art109
Johnstone, R. A., Manica, A., Fayet, A. L., Stoddard, M. C., Rodriguez-Gironés, M. A., and Hinde, C. A. (2016). Evidence for conditional cooperation: a response to Schlicht et al. Behav. Ecol. 27, e6–e7. doi: 10.1093/beheco/arw051
Khwaja, N., Preston, S. A. J., Hatchwell, B. J., Briskie, J. V., Winney, I. S., and Savage, J. L. (2017). Flexibility but no turn-taking in provisioning riflemen (Acanthisitta chloris). Anim. Behav. 125, 25–31. doi: 10.1016/j.anbehav.2016.12.021
Kilner, R., and Johnstone, R. A. (1997). Begging the question: are offspring solicitation behaviours signals of need? Trends Ecol. Evol. 12, 11–15. doi: 10.1016/S0169-5347(96)10061-6
Koenig, W. D., and Walters, E. L. (2016). Provisioning patterns in the cooperatively breeding acorn woodpecker: does feeding behaviour serve as a signal? Anim. Behav. 119, 124–134. doi: 10.1016/j.anbehav.2016.06.002
LaManna, J. A., and Martin, T. E. (2016). Costs of fear: behavioural and life-history responses to risk and their demographic consequences vary across species. Ecol. Lett. 19, 403–413. doi: 10.1111/ele.12573
Leniowski, K., and Wegrzyn, E. (2018). Synchronisation of parental behaviours reduces the risk of nest predation in a socially monogamous passerine bird. Sci. Rep. 8:7385. doi: 10.1038/s41598-018-25746-5
Lessells, C. M. (2012). “Sexual conflict,” in The Evolution of Parental Care, eds N. J. Royle, P. T. Smiseth, and M. Kolliker (Oxford: Oxford University Press), 150–170. doi: 10.1093/acprof:oso/9780199692576.003.0009
Lessells, C. M., and McNamara, J. M. (2012). Sexual conflict over parental investment in repeated bouts: negotiation reduces overall care. Proc. R. Soc. B 279, 1506–1514. doi: 10.1098/rspb.2011.1690
Low, M., Eggers, S., Arlt, D., and Pärt, T. (2008). Daily patterns of nest visits are correlated with ambient temperature in the northern wheatear. J. Ornithol. 149, 515–519. doi: 10.1007/s10336-008-0300-3
McNamara, J. M., Gasson, C. E., and Houston, A. I. (1999). Incorporating rules for responding into evolutionary games. Nature 401, 368–371. doi: 10.1038/43869
Santema, P., Schlicht, E., Schlicht, L., and Kempenaers, B. (2017). Blue tits do not return faster to the nest in response to either short-or long-term begging playbacks. Anim. Behav. 123, 117–127. doi: 10.1016/j.anbehav.2016.10.016
Savage, J. L., Browning, L. E., Manica, A., Russell, A. F., and Johnstone, R. A. (2017). Turn-taking in cooperative offspring care: by-product of individual provisioning behavior or active response rule? Behav. Ecol. Sociobiol. 71:162. doi: 10.1007/s00265-017-2391-4
Schlicht, E., Santema, P., Schlicht, R., and Kempenaers, B. (2016). Evidence for conditional cooperation in biparental care systems? A comment on Johnstone et al. Behav. Ecol. 27, e2–e5. doi: 10.1093/beheco/arw036
Trivers, R. L. (1972). “Parental investment and sexual selection,” in Sexual Selection and the Descent of Man 1871–1971, ed B. Campbell (Chicago, IL: Aldine Press), 136–179.
Keywords: parental care, sexual conflict, conditional cooperation, alternation, provisioning
Citation: Santema P, Schlicht E and Kempenaers B (2019) Testing the Conditional Cooperation Model: What Can We Learn From Parents Taking Turns When Feeding Offspring? Front. Ecol. Evol. 7:94. doi: 10.3389/fevo.2019.00094
Received: 09 October 2018; Accepted: 11 March 2019;
Published: 12 April 2019.
Edited by:
James Luke Savage, University of Cambridge, United KingdomReviewed by:
Arne Iserbyt, University of Antwerp, BelgiumCamilla Anne Hinde, Wageningen University & Research, Netherlands
Copyright © 2019 Santema, Schlicht and Kempenaers. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Peter Santema, cHNhbnRlbWFAb3JuLm1wZy5kZQ==
†Bart Kempenaers orcid.org/0000-0002-7505-5458
 Peter Santema
Peter Santema Emmi Schlicht
Emmi Schlicht Bart Kempenaers
Bart Kempenaers