A Theoretical Study on Laser Cooling Feasibility of Group IVA Hydrides XH (X = Si, Ge, Sn, and Pb): The Role of Electronic State Crossing

The feasibility of direct laser cooling of SiH, GeH, SnH, and PbH is investigated and assessed based upon first principles. The internally contracted multi-reference configuration interaction method with the Davidson correction is applied. Very good agreement is obtained between our computed spectroscopic constants and the available experimental data. We find that the locations of crossing point between the B²Σ⁻ and A²Δ states have the tendency of moving downwards from CH to SnH relative to the bottom of the corresponding A²Δ potential, which precludes the laser cooling of GeH, SnH, and PbH. By including the spin-orbit coupling effects and on the basis of the ${\text{A}}^2{\Delta }_{5/2}\to$${\text{X}}^2{\Pi }_{3/2}$ transition, we propose a feasible laser cooling scheme for SiH using three lasers with wavelengths varying from 400 to 500 nm, which features a very large vibrational branching ratio (0.9954) and a very short radiative lifetime (575 ns). Moreover, similar studies are extended to carbon monosulfide (CS) with a feasible laser cooling scheme proposed. The importance of electronic state crossing in molecular laser cooling is underscored, and our work suggests useful caveats to the choice of promising candidates for producing ultracold molecules.

1. Introduction

Laser cooling of diatomic molecule is an issue of great interest owing to their promising applications in many fields such as quantum computing and precision measurement (Carr et al., 2009; Hudson et al., 2011; Yan et al., 2013; Baron et al., 2014). Direct laser cooling of SrF molecules to μK level was firstly achieved with only three laser beams (Shuman et al., 2010). In addition, transverse and longitudinal laser cooling experiment was applied to YO (Hummon et al., 2013) and CaF (Zhelyazkova et al., 2014), respectively. So far only a few molecules including SrF, CaF, and YO have been cooled to the ultracold temperature experimentally, and there is an urgent need to search for more suitable molecular candidates for laser cooling. Recently, a number of theoretical efforts have been made in searching for promising molecular laser cooling candidates (Wells and Lane, 2011a; Fu et al., 2016a; Cao et al., 2019; González-Sánchez et al., 2019; Xu et al., 2019). It is generally accepted (Di Rosa, 2004; Fu et al., 2016b) that, a suitable laser-cooling candidate must meet the following three criteria: highly diagonal Franck-Condon factors (FCFs), a short lifetime, and no intermediate electronic-state interference. Here we reveal that the crossing between two electronic states may damage the cooling scheme, and may be regarded as the fourth criterion that should be checked beforehand in choosing laser-cooling candidates. We will demonstrate this point by investigating the laser cooling of group IVA hydrides.

There have been a lot of studies on SiH and GeH (Kleman and Werhagen, 1953a; Ram et al., 1998), while SnH and PbH have not been sufficiently studied (Alekseyev et al., 1996; Zhao et al., 2017). SiH radical has been attracting great interest over many years, since it plays a significant role in many industrial processes such as plasma vapor deposition (Ram et al., 1998). In 1930, the emission spectrum of SiH was observed using an arc source, and a strong transition near 410 nm was assigned to as the A²Δ→X²Π transition (Jackson, 1930). In 1969, the lifetime of the A²Δ state was measured as 700±100 ns with phase-shift technique (Smith, 1969). Later, the high-resolution spectrograms of 325 nm bands for SiH were measured with rotational analysis (Bollmark et al., 1971). In 1979, the accurate spectroscopic parameters and molecular constants of SiH were summarized and reported in the literature (Huber and Herzberg, 1979). In 1980, the emission spectroscopy of SiH in a silane glow-discharge was measured with a moderate spectrometer (Perrin and Delafosse, 1980). In 1989, the spectrum of SiH radical was observed over the laser wavelength between 426 and 430 nm by resonance-enhanced multiphoton ionization spectroscopy, and new state was tentatively assigned as ²Π state with further computer simulation (Johnson and Hudgens, 1989). Parallel to extensive studies on SiH, the spectra of GeH are well-studied in experiment, especially for the low-lying electronic states. In 1953, the spectra of A⁴Σ⁻→X²Π and A²Δ→X²Π band for GeH were first observed (Kleman and Werhagen, 1953a,b). Subsequently, the spectroscopic constants of the A²Δ state of GeH were deduced with rotational analysis (Klynning and Lindgren, 1966). In 1989, the radiative lifetime of the A²Δ state of GeH was measured as 93±10 ns with laser-induced fluorescence technique (Bauer et al., 1989). In 1938, the emission spectrum of PbH was firstly detected using arc-excitation in hydrogen atmosphere (Watson, 1938). In 2008, the near-infrared emission spectra of ${\text{X}}^2{\Pi }_{3/2}{\to }^2{\Pi }_{1/2}$ transitions of PbH were observed using high-resolution Fourier-transform spectrometry (Setzer et al., 2008).

Theoretically, in 1967 the first ab initio calculations on the SiH radical were performed with the Hartree-Fock method (Cade and Huo, 1967), in which the potential energy curve (PEC) of the ground-state was obtained. In 1983, the PECs of SiH were evaluated with the multi-reference double-excitation configuration interaction calculations (Lewerenz et al., 1983). In recent years, there are many calculations about spectroscopic constants of SiH (Ram et al., 1998; Kalemos et al., 2002; Shi et al., 2008, 2013; Zhang et al., 2018). In 2013, the PECs of eleven Ω states generated from seven Λ-S states of SiH radical were calculated by the internally contracted multi-reference configuration interaction method with the Davidson correction (icMRCI+Q) (Shi et al., 2013). In 2001, the X²Π and a⁴Σ⁻ states of GeH were investigated with the MRCI approach and the spectroscopic parameters for the two states were reported (Bruna and Grein, 2001). In 2015, the lifetimes of A²Δ and a⁴Σ⁻ states of GeH were obtained (Li et al., 2015). Some ab initio calculations on SnH and PbH were also reported (Alekseyev et al., 1996; Zhao et al., 2017).

The A²Δ→X²Π transition of CH was pointed out to be dominated by diagonal bands, making it a potential cooling candidate (Wells and Lane, 2011b). SiH is considered to be suitable for laser cooling by Zhang et al. (2018), although they ignored the SOC effects in their laser cooling schemes. In addition, they did not consider the effect of electronic state crossing. According to previous investigations (Fu et al., 2017; Xia et al., 2017), the SOC effects are important for laser cooling molecules and will be taken into account in this work. There have not been theoretical studies reported on laser cooling of GeH, SnH, and PbH. In this work, by means of highly accurate ab initio and dynamical calculations, we investigate the laser cooling of group IVA hydrides and the importance of electronic state crossing in molecular laser cooling is underscored. Additionally, based on the fourth criterion mentioned above, laser cooling of CS is investigated.

The paper is organized as follows. The theoretical methods and computational details are briefly described in section 2. The results and discussion are shown in section 3. The conclusions are given in section 4.

2. Methods and Computational Details

In this paper, all the ab initio calculations of SiH, GeH, SnH, and PbH are performed using the MOLPRO 2012.1 program package (Werner et al., 2012). For each Λ-S state, the energies are computed using the complete active space self-consistent field (CASSCF) (Werner and Knowles, 1985) approach followed by the icMRCI+Q (Langhoff and Davidson, 1974). The lowest electronic configuration of Si is (core)3s²3p² corresponding to the atomic states ³P and ¹D. In combination with the 1 s function of a H atom, the lowest electronic configuration of SiH in X²Π is invariably (core)4σ²5σ²6σ⁰2π¹ and the next is (core)4σ²5σ¹6σ⁰2π² leading to the multiplets ⁴Σ⁻, ²Δ, ²Σ⁻, and ²Σ⁺, which could be considered as involving a pσ→pπ transition within the Si atom. In asymptote region, the 5σ molecular orbital origins from the 1s on hydrogen, while the 6σ and 2π correspond to the 3p on silicon.

The choice of active space is very important in the CASSCF and MRCI+Q calculations (Liu et al., 2009; Yu and Bian, 2011, 2012). The active space of SiH used here consists of ten orbitals corresponding to Si 2p3s3p4p_π and H 1s, and is referred to as (11e, 10o), which is carefully chosen to include proper orbitals, and large enough for the present calculations. Our test calculations indicate that the full valence space is inadequate, and thus additional five orbitals are added into the active space. The inner shell orbitals are included to account for the core-valence correlation effects and the outer virtual orbitals are added to give a better description on the dissociation behavior and Rydberg character, especially for excited electronic states (Shen et al., 2017). In fact, a completely satisfactory set could neither be found by increasing the active orbital space nor by including more states (Simah et al., 1999). The best compromise we could achieve is to distribute the eleven electrons in 10 active orbitals. As for the basis set, we use the aug-cc-pV5Z basis sets for Si and H atom. In the SOC calculations, the SO eigenstates are obtained by diagonalizing Ĥ^el+Ĥ^SO in the basis of eigenfunctions of Ĥ^el. In addition, the Ĥ^el and Ĥ^SO are obtained from the icMRCI+Q calculations and icMRCI+Q waves functions, respectively. The active space for GeH in the present work is (9e, 9o), which consists of the Ge 3d_π4s4p5p_π and H 1s orbitals. The accuracy and computational performance are compromised, thus the active space of GeH includes the 3d_π orbitals, valence orbitals and 5p_π orbitals. The aug-cc-pV5Z-DK basis sets are used for GeH. Here, the active space for SnH consists of the Sn 4d5s5p and H 1s orbitals and is referred as (15e, 10o). The aug-cc-pVQZ basis sets are used for H and aug-cc-pwCVQZ-PP with ECP28MDF effective core potentials for Sn. Similarly, the active space for PbH is (15e, 10o), which consists of the Pb 5d6s6p and H 1s orbitals. We use the aug-cc-pVQZ basis sets for H and aug-cc-pwCVQZ-PP with ECP60MDF for Pb. In addition, the active space used for the study of CS is (10e, 8o) corresponding to the C 2s2p and S 3s3p, and we use the aug-cc-pV5Z basis sets for both C and S atoms.

The Einstein spontaneous emission coefficient $A_{{\nu }^{\prime }\nu }$ is calculated by the following expression (Herzberg, 1950),

$\begin{array}{ll}{A}_{{\nu }^{\prime }\nu }=\frac{16{\pi }^3}{3{ϵ}_0\hslash }\frac{S(J^{\prime },J)}{2J^{\prime }+1}{v}^3\left|{\text{Ψ}}_{{\nu }^{\prime },J^{\prime }}\right|M(r)|{\text{Ψ}}_{\nu ,J}{|}^2& (1)\end{array}$

where $A_{{\nu }^{\prime }\nu }$ is in units of s⁻¹, ε₀ is the vacuum permittivity in units of F· cm⁻¹, M(r) is the transition dipole function in Debye unit, ν is emission frequency in units of cm⁻¹, ${\text{Ψ}}_{{\nu }^{\prime },J^{\prime }}$ and Ψ_{ν, J} are normalized radial wave functions, ℏ is the Planck constant and S(J′, J) is the Hönl-London rotational intensity factor. The radiative lifetime for a given vibrational level ν′ can be obtained by the following equation,

$\begin{array}{ll}{\tau }_{{\nu }^{\prime }\nu }=1/{\displaystyle \sum _{\nu }}{A}_{{\nu }^{\prime }\nu }& (2)\end{array}$

The spectroscopic parameters of group IVA hydrides, including the equilibrium bond length (R_e), the harmonic vibrational constant (ω_e), the rotational constant (B_e), and adiabatic relative electronic energy referred to the ground state (T_e) are obtained using the Le Royν′s LEVEL program (Le Roy, 2007).

3. Results and Discussion

3.1. PECs of the Λ-S States and Spectroscopic Constants

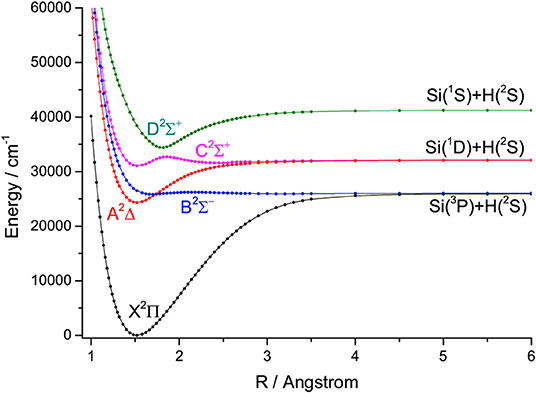
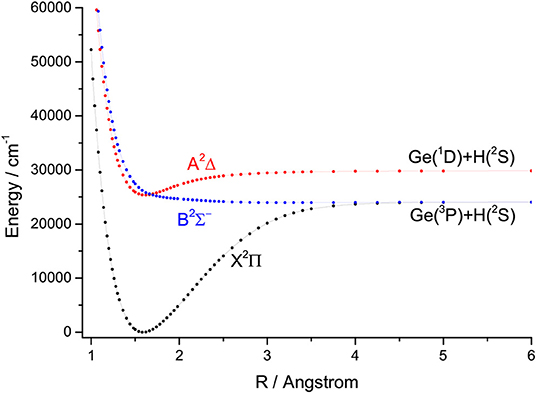
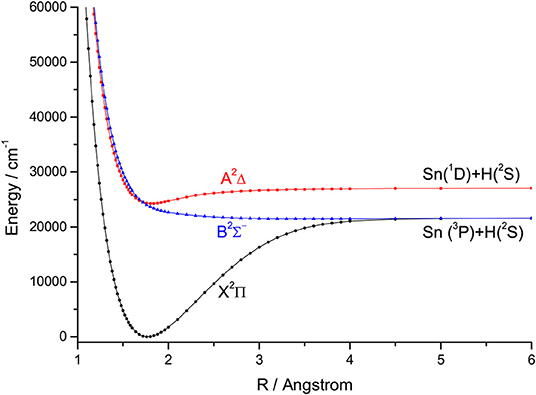
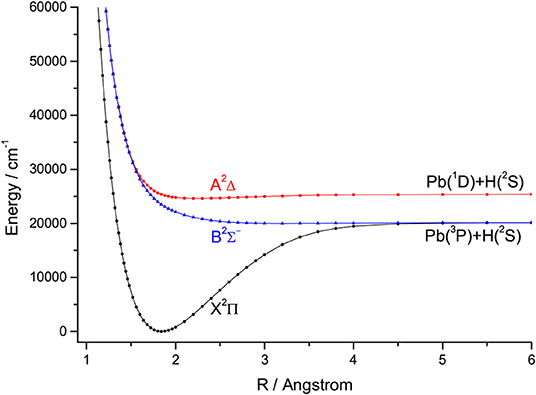
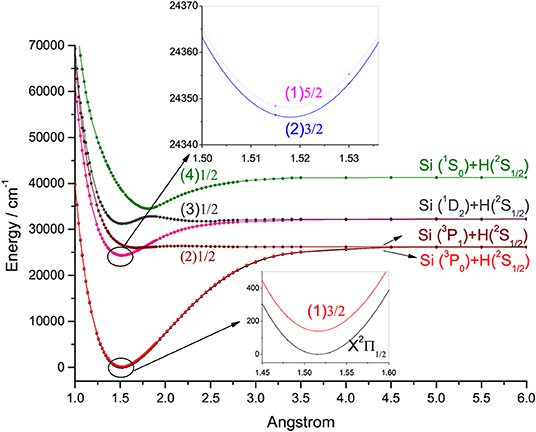
For the group IVA hydrides, the main configuration of the ground state (X²Π) is (core)4σ²5σ²6σ⁰2π¹. The second lowest state here is the A²Δ, whose main configuration is (core)4σ²5σ¹6σ⁰2π². The PECs of SiH, GeH, SnH, and PbH computed using the icMRCI+Q method are shown in Figures 1–4, respectively. The weights of ionic configuration in the X²Π and A²Δ states decrease gradually with increasing internuclear distances. As seen in Figure 1, the five states of SiH correlate to the three dissociation limits. The X²Π and A²Δ states are the two energetically lowest lying electronic state and correlate to the neutral atomic Si(³P)+H(²S) limit and Si(¹D)+H(²S) limit, respectively. From Figures 2–4, we can see that the X²Π and A²Δ states of GeH, SnH, and PbH correlate to the neutral atomic Ge/Sn/Pb(³P)+H(²S) limit and Ge/Sn/Pb(¹D)+H(²S) limit, respectively. The PEC of the B²Σ⁻ crosses with that of A²Δ will be discussed in details in section 3.2. The PECs of the B²Σ⁻ and X²Π correlate to the same neutral atomic Si(³P)+H(²S) limit.

FIGURE 1

Figure 1. Potential energy curves of SiH for the five Λ-S states at the icMRCI+Q level.

FIGURE 2

Figure 2. Potential energy curves of GeH for the three Λ-S states at the icMRCI+Q level.

FIGURE 3

Figure 3. Potential energy curves of SnH for the three Λ-S states at the icMRCI+Q level.

FIGURE 4

Figure 4. Potential energy curves of PbH for the three Λ-S states at the icMRCI+Q level.

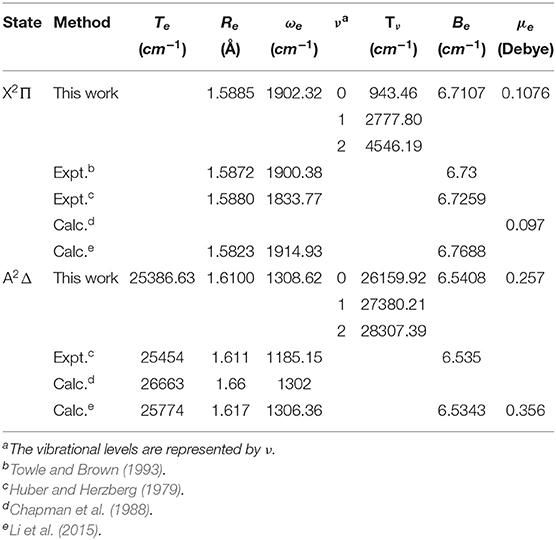
Since the spectroscopic constants of the X²Π and A²Δ states have been measured in experiment for SiH, GeH, SnH, and PbH, comparing with experimental data could provide an indicator of the reliability of our calculations. We present our calculated spectroscopic constants of SiH, GeH, and SnH in Tables 1, 2 and Table S1, together with previous theoretical and experimental values for comparison. The calculated R_e and ω_e of the X²Π of SiH are 1.5200 Å and 2047.71 cm⁻¹, respectively, which are in good agreement with the corresponding experiment data (1.5201 Å and 2041.80 cm⁻¹) (Huber and Herzberg, 1979). The contributions from non-valence orbitals to correlation energy are relatively small for the ground state, however, they become very important for the excited states and lead to noticeable differences. In particular, the experimental T_e is 24300.4 cm⁻¹ (Huber and Herzberg, 1979) and our calculated one with a better active space is 24299.20 cm⁻¹, whereas that obtained by Zhang et al. (2018) is 25080.92 cm⁻¹, which deviates from experimental value by about 780 cm⁻¹. When additional virtual orbitals are taken into account by Kalemos et al. (2002), the deviation is reduced to around 200 cm⁻¹. The spectroscopic constants of GeH obtained from the active space (9e, 9o) are shown in Table 2. The calculated R_e and ω_e of the X²Π of GeH are 1.5885 Å and 1902.32 cm⁻¹, respectively, which are in very good agreement with the corresponding experiment data (1.5872 Å and 1900.38 cm⁻¹) (Towle and Brown, 1993). The A²Δ state of GeH, the experimental T_e is 25454 cm⁻¹ (Huber and Herzberg, 1979), whereas our calculated T_e is 25386.63 cm⁻¹, which is much improvement compared with previous computational value 25774 cm⁻¹ (Li et al., 2015).

TABLE 1

Table 1. Spectroscopic constants of the A²Δ and X²Π states for SiH.

TABLE 2

Table 2. Spectroscopic constants of the A²Δ and X²Π states for GeH.

The permanent dipole moments (PDMs) and absolute values transition dipole moments (TDMs) for the A²Δ→X²Π transition of SiH at the icMRCI+Q level are represented in Figure 5. The TDMs for the A²Δ→X²Π transition of SiH are 0.6231 D at R_e and decrease with the increasing bond length. The calculated TDMs for the A²Δ→X²Π transition of GeH are given in Figure S1. The Einstein A coefficients and vibrational branching ratio of the A²Δ→X²Π transition of GeH are listed in Table S2. The calculated radiative lifetime for the A²Δ(ν′ = 0) state of SiH is 613 ns, which is in very good agreement with the experimental value (534 ± 23 ns) (Bauer et al., 1984). The FCFs $f_{{\nu }^{\prime }\nu }$ of the A²Δ→X²Π transition for GeH are calculated and plotted in Figure S2. In this work, the f₀₀ (0.995) of SiH is in excellent agreement with the value (0.994) derived from experiment (Smith and Liszt, 1971). The computational value of f₀₀ for GeH (0.940) is in very good agreement with the experimental value 0.928 (Erman et al., 1983).

FIGURE 5

Figure 5. The permanent dipole moments (PDMs) and transition dipole moments (TDMs) for the X²Π and A²Δ states of SiH at the icMRCI+Q level.

3.2. The Comparison of the Feasibility of Laser Cooling for Group IVA Hydrides

There are some similar properties of group IVA hydrides. The inner closed-shell orbitals are occupied with 2, 10, 28, 46, and 78 electrons for CH, SiH, GeH, SnH, and PbH, respectively. The equilibrium bond length R_e increases regularly from CH to PbH, while the harmonic constant ω_e decreases in the order of CH>SiH>GeH>SnH>PbH (Huber and Herzberg, 1979; Alekseyev et al., 1996; Zhao et al., 2017). The comparison of the feasibility of laser cooling for group IVA hydrides is summarized here. An amplified view of crossing regions of PECs for SiH, GeH, SnH, and PbH is shown in Figure 6, we see that the dissociation energies of the A²Δ states of SiH, GeH, SnH, and PbH are 7735.89, 4465.21, 2849.26, and 798.36 cm⁻¹, respectively. This trend is consistent when the second-row CH is included and the corresponding dissociation energy is 16641.68 cm⁻¹ (Wells and Lane, 2011b). The depths of the A²Δ state decrease from CH to PbH, and it supports only one vibrational level for PbH. In equilibrium region, two electrons anti-parallelly are distributed on one (n$p_x^{\uparrow \downarrow }$/n$p_y^{\uparrow \downarrow }$) and two (n$p_x^{\uparrow }n{p}_y^{\downarrow }$) sp³ hybridized orbitals for the B²Σ⁻ and A²Δ states, respectively. The hybridized orbital effect vanishes as the internuclear distance increases to the asymptotic region. Where, n$p_x^{\uparrow \downarrow }$/n$p_y^{\uparrow \downarrow }$ goes to X(³P) and n$p_x^{\uparrow }n{p}_y^{\downarrow }$ goes to X(¹D). Therefore, there is a crossing point between the B²Σ⁻ and A²Δ states of group IVA hydrides. The electronic state crossing between the B²Σ⁻ and A²Δ states can lead to nonradiative transition (Wu et al., 2019), and may cause predissociation. This kind of electronic state crossing in a diatomic molecule will become potential energy surface intersections in the polyatomic cases involving multiple electronic states (Liu et al., 2003; Zhao et al., 2006). We find that the locations of crossing point between the B²Σ⁻ and A²Δ states have the tendency of moving downwards from CH to SnH relative to the bottom of the corresponding A²Δ state potential. The locations of crossing point between the B²Σ⁻ and A²Δ states of GeH and SnH are 591 and 255 cm⁻¹ lower than the corresponding vibrational level ν′ = 0 in the A²Δ, while that of third-row SiH is 670 cm⁻¹ higher than the vibrational level ν′ = 0 in the A²Δ. This trend is consistent when the second-row CH is included since the corresponding crossing point is 3,000 cm⁻¹ higher (Wells and Lane, 2011b).

FIGURE 6

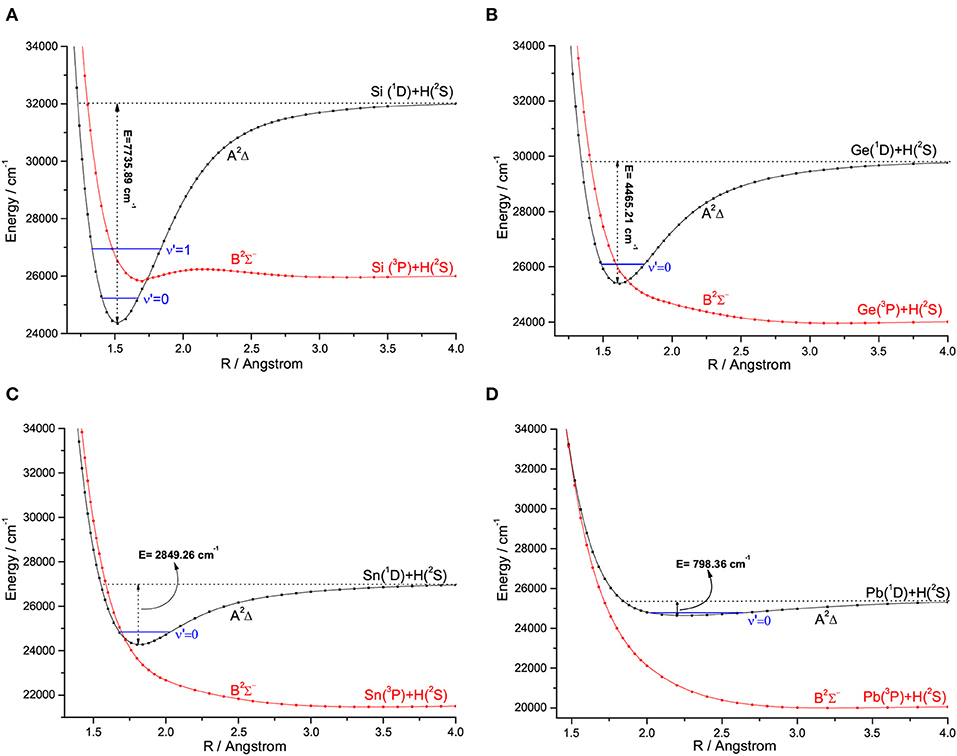
Figure 6. An amplified view of crossing regions of potential energy curves for SiH (A), GeH (B), SnH (C), and PbH (D).

The location of crossing point between the B²Σ⁻ and A²Δ states of SiH is higher than the vibrational level ν′ = 0 in the A²Δ, indicating that laser cooling of SiH in the A²Δ→X²Π transition may not be affected by electronic state crossing. However, our results imply that the crossing between the B²Σ⁻ and A²Δ states of GeH will lead to predissociation of all vibrational levels of the A²Δ state, which is backed up by experiments of Erman et al. (1983) using high frequency deflection technique. They reported that, 80–90% of the GeH molecules excited to the A²Δ state decay via predissociation to their ground state atomic constituents, and fewer than 20% of the molecules follow the regular decay route to the ground state. Furthermore, the A²Δ states of SnH and PbH have a similar problem to that of GeH, although there has been no relevant experimental measurements reported. It is clear that GeH and SnH can not be used for laser cooling due to the electronic state crossing. In addition, the small Franck-Condon factor f₀₀ (0.08) of PbH also suggests that it is not suitable for laser cooling.

It seems that both CH and SiH are very good laser cooling candidates, and we make a comparison in the following. The f₀₀ of SiH (0.995) is close to that of CH (0.9957) (Wells and Lane, 2011b). The radiative lifetimes of the A²Δ state of SiH and CH are 575 and 536 ns (Wells and Lane, 2011b), respectively. The T_Doppler and T_recoil of SiH (6.65 and 3.89 μK) are also similar to that of CH (7.13 and 7.91 μK) (Wells and Lane, 2011b). The calculated pump and repump wavelengths for SiH and CH are all in the visible region. The electronic state crossing between the B²Σ⁻ and A²Δ states of CH is higher than that of SiH, with both crossing points located above the corresponding vibrational ν′ = 0 levels in the A²Δ state. Generally speaking, a larger atomic mass difference for the diatomic candidate is desirable by experimentalists, and in this respect, SiH is better than CH. Furthermore, we will propose a scheme using two spin-orbit states for SiH in the next section, which is more feasible than the one using two Λ-S states for CH. The A²Δ→X²Π transition of CH was used to establish a laser cooling scheme, and the SOC effects were not included (Wells and Lane, 2011a). With the inclusion of the SOC effects into the icMRCI wave functions in our calculations, accurate Ω states are determined. The calculated spectroscopic constants of SiH are in very good accordance with experimental measurements. The prospect for the production of ultracold SiH molecules by means of direct laser cooling method is discussed below.

3.3. Laser Cooling Scheme for SiH

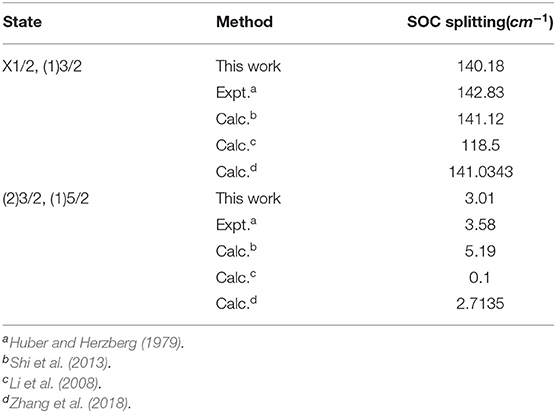
When the SOC effects are taken into account, seven Ω states, involving four states with Ω = 1/2, two states with Ω = 3/2 and one state with Ω = 5/2, are generated from the five Λ-S states of SiH. The PECs of the Ω states are depicted in Figure 7. The SOC splitting of the X²Π and A²Δ states of SiH is shown in Table 3. As seen, the energy separation of the ${\text{X}}^2{\Pi }_{1/2}$ and ${\text{X}}^2{\Pi }_{3/2}$ is 140.18 cm⁻¹ in this work, which is in excellent agreement with the experimental value (142.83 cm⁻¹) (Huber and Herzberg, 1979). For the ${\text{A}}^2{\Delta }_{3/2}$ and ${\text{A}}^2{\Delta }_{5/2}$ states, the energy separation is 3.01 cm⁻¹.

FIGURE 7

Figure 7. Potential energy curves of SiH for the seven Ω states at the icMRCI+Q level.

TABLE 3

Table 3. The spin-orbit coupling (SOC) splitting for SiH.

We find that, the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition is suitable for laser cooling in three possible transitions, ${\text{A}}^2{\Delta }_{3/2}$→${\text{X}}^2{\Pi }_{1/2}$, ${\text{A}}^2{\Delta }_{3/2}$→${\text{X}}^2{\Pi }_{3/2}$, and ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$. In the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition, SiH molecules will jump from the ${\text{X}}^2{\Pi }_{3/2}$(ν = 0) to the ${\text{A}}^2{\Delta }_{5/2}$(ν′ = 0), then the ${\text{A}}^2{\Delta }_{5/2}$(ν′ = 0) state will decay to the ${\text{X}}^2{\Pi }_{3/2}$ rather than ${\text{X}}^2{\Pi }_{1/2}$ according to the selection rules. The ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition can ensure a closed-loop cooling cycle, while the other two transitions can not. The three-laser cooling scheme proposed in the following using the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition is more feasible than the one proposed by Zhang et al. (2018) without including the SOC effects. More importantly, they did not consider the effect of electronic state crossing, and proposed a laser cooling cycle for SiH involving the ν′ = 1 vibrational level of the A²Δ state, which would predissociation before the radiative transition and can not be used to establish laser cooling cycles.

The TDMs of SiH for the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition at the icMRCI+Q level are represented in Figure 8. The TDMs of SiH is 0.6157 D at R_e. The FCFs values of the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition for SiH are computed and shown in Figure 9. As seen, the Δν = 0 transitions are significantly larger than those for the off-diagonal terms. The present value of f₀₀ (0.9949) is so large that the spontaneous decays to ν = 1,2 vibrational levels in the ${\text{X}}^2{\Pi }_{3/2}$ are significantly restrained. Additionally, the relative strengths of the photon loss pathways are more directly related to the vibrational branching ratios than the FCFs in the laser cooling cycle (Lane, 2015). Furthermore, we calculate the Einstein A coefficients $A_{{\nu }^{\prime }\nu }$ and vibrational branching ratios $R_{{\nu }^{\prime }\nu }$ of the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition for SiH. The $A_{{\nu }^{\prime }\nu }$ and $R_{{\nu }^{\prime }\nu }$ of the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition are listed in Table 4. As seen in Table 4, a very large A₀₀ (1.73 × 10⁶ s⁻¹) and very low scattering probabilities into off-diagonal bands (R₀₁ = 3.61 × 10⁻³, R₀₂ = 9.83 × 10⁻⁴, R₀₃ = 9.20 × 10⁻⁸) of SiH contribute to a desirable condition for rapid and efficient laser cooling. It should be noted that R₀₀ (0.9954) is slightly larger than f₀₀ (0.9949), indicating that the probability of spontaneous decay to the ${\text{X}}^2{\Pi }_{3/2}$(ν = 0) increases when the variations in transition wavelength are taken into account.

FIGURE 8

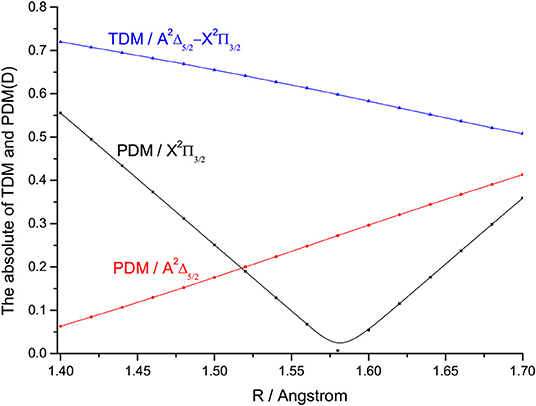
Figure 8. The permanent dipole moments (PDMs) and transition dipole moments (TDMs) for the ${\text{X}}^2{\Pi }_{3/2}$ and ${\text{A}}^2{\Delta }_{5/2}$ states of SiH at the icMRCI+Q level.

FIGURE 9

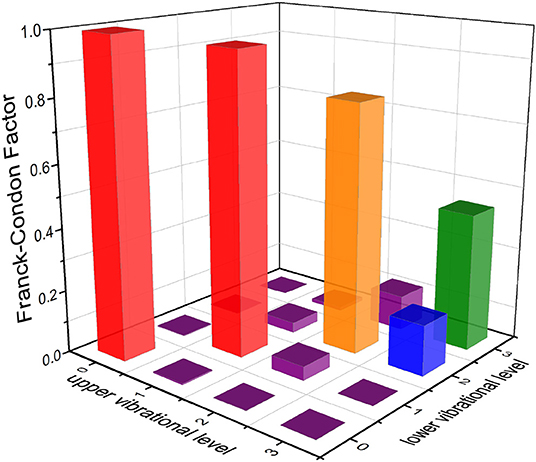
Figure 9. Franck-Condon factors of the ${\text{A}}^2{\Delta }_{5/2}$(ν′ ≤ 3)→${\text{X}}^2{\Pi }_{3/2}$(ν ≤ 3) transition for SiH, calculated at the icMRCI+Q level.

TABLE 4

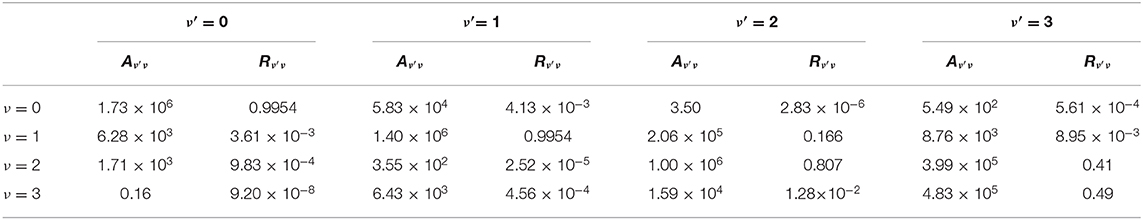
Table 4. Calculated Einstein A coefficients $A_{{\nu }^{\prime }\nu }$ and vibrational branching ratio $R_{{\nu }^{\prime }\nu }$ of the ${\text{A}}^2{\Delta }_{5/2}$ (ν′) → ${\text{X}}^2{\Pi }_{3/2}(\nu )$ transition for SiH.

The vibrational branching ratios $R_{{\nu }^{\prime }\nu }$ is determined by the following expression:

$\begin{array}{ll}{R}_{{\nu }^{\prime }\nu }=A_{{\nu }^{\prime }\nu }/{\displaystyle \sum _{\nu }}{A}_{{\nu }^{\prime }\nu }& (3)\end{array}$

Moreover, the Doppler temperature (T_Doppler) is the achievable minimum temperature of translational cooling with the Doppler method, it is obtained by the following expression:

$\begin{array}{ll}{T}_{Doppler}=h/(4k_B\pi \tau ),& (4)\end{array}$

where k_B and h are Boltzmann′s constant and Planck′s constant, respectively (You et al., 2016). The radiative lifetime (τ) for the ${\text{A}}^2{\Delta }_{5/2}$(ν′ = 0) of SiH is 575 ns and T_Doppler is 6.65 μK. Additionally, the recoil temperature (T_recoil) is calculated by the following expression:

$\begin{array}{ll}{T}_{recoil}=h^2/(m{k}_B{\lambda }^2),& (5)\end{array}$

and the obtained T_recoil for the ${\text{A}}^2{\Delta }_{5/2}({\nu }^{\prime }=0)\to {\text{X}}^2{\Pi }_{3/2}(\nu =0)$ transition of SiH is 3.89 μK.

The proposed scheme to facilitate the laser cooling of SiH is shown in Figure 10. The large R₀₀ (0.9954) of SiH indicates that the ${\text{A}}^2{\Delta }_{5/2}({\nu }^{\prime }=0)\to {\text{X}}^2{\Pi }_{3/2}(\nu =0)$ transition has the largest probabilities. A desirable laser cooling cycle needs to solve the vibrational branching loss. Therefore, the off-diagonal vibrational branching ratios $R_{{\nu }^{\prime }\nu }$ of SiH are calculated, and the probabilities of decay from the ${\text{A}}^2{\Delta }_{5/2}$(ν ′ = 0) to the ${\text{X}}^2{\Pi }_{3/2}$(ν = 1, 2) are firstly obtained (R₀₁ = 3.61 × 10⁻³ and R₀₂ = 9.83 × 10⁻⁴). Besides, the probabilities of the unwanted decay channels are also computed by using R₀₃₊+R₀₂×R₁₃₊. The negligible value of 1.0 × 10⁻⁵ means that the present scheme will allow for at least 1.0 × 10⁵ photon absorption/emission cycles, which are sufficient enough to decelerate SiH in a cryogenic beam, in principle (Shuman et al., 2010). The laser cooling scheme takes the transition ${\text{A}}^2{\Delta }_{5/2}({\nu }^{\prime }=0)\leftarrow {\text{X}}^2{\Pi }_{3/2}(\nu =0)$ as the main pump, ${\text{A}}^2{\Delta }_{5/2}({\nu }^{\prime }=0)\leftarrow {\text{X}}^2{\Pi }_{3/2}(\nu =1)$ and ${\text{A}}^2{\Delta }_{5/2}({\nu }^{\prime }=0)\leftarrow {\text{X}}^2{\Pi }_{3/2}(\nu =2)$ as the first and second vibrational repump, respectively. Accurate T_e is crucial for estimating the pump and repump wavelengths in laser cooling cycles, and our calculated T_e values, which are very close to experimental ones, give confidence in the subsequent study on laser cooling, especially, for SiH. The calculated value of wavelength λ₀₁ should be larger than λ₀₀, however, the wavelengths λ₀₁ obtained by Zhang et al. (2018), is 376.88 nm, which is smaller than that of their main pump. In our laser-driven cycling, the calculated pump and repump wavelengths of λ₀₀, λ₀₁, and λ₀₂ are 412.6, 449.7, and 491.8 nm, respectively. The required wavelengths are all in the range of 400–500 nm and can be produced with the frequency doubled semiconductor laser, which has been used for the laser cooling experiment of the strontium atom (Wang et al., 2009). The SiH molecules will stay in the laser cooling cycle until the decay to ν≥3 (${\text{X}}^2{\Pi }_{3/2}$).

FIGURE 10

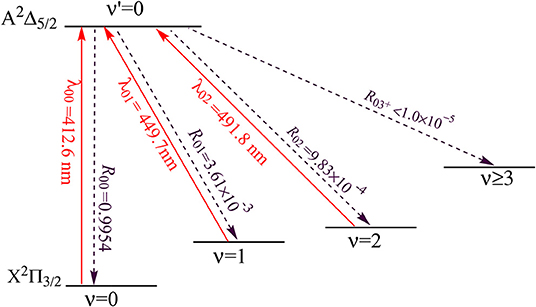
Figure 10. Proposed three-laser cooling scheme for SiH using the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transitions. Solid arrows indicate laser-driven transitions at certain wavelengths ${\lambda }_{{\nu }^{\prime }\nu }$. Dashed arrows indicate spontaneous decays from the ${\text{A}}^2{\Delta }_{5/2}({\nu }^{\prime }=0)$ state with the calculated $R_{{\nu }^{\prime }\nu }$.

3.4. Laser Cooling Scheme for CS

We further find that CS is a promising candidate based on the criteria mentioned above. The PECs of CS, obtained at the icMRCI+Q level, is shown in Figure 11. Our calculated R_e and ω_e of the X¹Σ⁺ of CS are 1.5380 Å and 1288.63 cm⁻¹, respectively, which are in very good accordance with the corresponding experimental values (1.5349 Å and 1285.1 cm⁻¹) (Huber and Herzberg, 1979). As for the A²Δ state of CS, our calculated T_e 39175.96 cm⁻¹ is in agreement with the experimental value 38,904 cm⁻¹ (Huber and Herzberg, 1979). The calculated vibrational branching ratio R₀₀ is 0.885, which is highly diagonal.

FIGURE 11

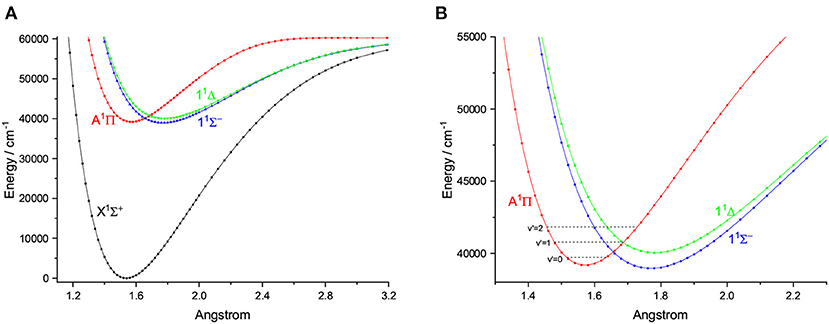
Figure 11. Potential energy curves of CS for the four Λ-S states at the icMRCI+Q level (A) and an amplified view of crossing regions of potential energy curves (B).

As shown in Figure 11, the PEC of A¹Π crosses with those of two other electronic states, however, from an amplified view of the crossing regions we see that, the two crossing points are higher than the ν′ = 0 vibrational level of the A¹Π state. So this kind of crossing would not affect laser cooling cycles using the A¹Π(ν′ = 0)→X¹Σ⁺(ν <3) transition. A suitable laser cooling scheme using the A¹Π→X¹Σ⁺ transition of CS is proposed and shown in Figure 12. The scheme takes the transition A¹Π(ν′ = 0)→X¹Σ⁺(ν = 0) as the main pump, A¹Π(ν′ = 0)→X¹Σ⁺(ν = 1) and A¹Π(ν′ = 0)→X¹Σ⁺(ν = 2) as the first and second vibrational repump, respectively. The radiative lifetime of the A¹Π state is 70 ns, whereas the T_Doppler and T_recoil of the A¹Π→X¹Σ⁺ transition are 54.61 and 6.64 μK, respectively. The large R₀₀, short radiative lifetime and ultracold temperatures suggest CS as a promising candidate for rapid and efficient laser cooling.

FIGURE 12

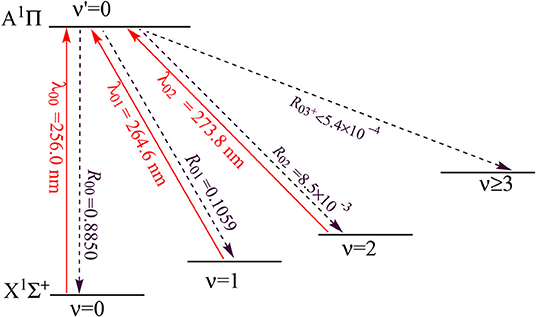
Figure 12. Proposed three-laser cooling scheme for CS using the A¹Π→X¹Σ⁺ transitions. Solid arrows indicate laser-driven transitions at certain wavelengths ${\lambda }_{{\nu }^{\prime }\nu }$. Dashed arrows indicate spontaneous decays from the A¹Π(ν′ = 0) state with the calculated $R_{{\nu }^{\prime }\nu }$.

4. Conclusions

The fourth criterion for molecular laser cooling is proposed in this work, that is, there is no electronic-state crossing, or the crossing point is high enough in energy. Its importance is demonstrated by investigating the laser cooling feasibility of group IVA hydrides and carbon monosulfide.

Ab initio and dynamical calculations are performed, and the calculated spectroscopic constants are in very good agreement with the available experimental data. We find that the locations of crossing point between the B²Σ⁻ and A²Δ states have the tendency of moving downwards from CH to SnH relative to the bottom of the corresponding A²Δ potential, and this would lead to failure of cooling GeH and SnH. The potential wells of the A²Δ state of group IVA hydrides become shallower and shallower from CH to PbH, with the Franck-Condon factor decreasing, which results in a very small Franck-Condon factor for PbH. It is clear that PbH is not a suitable candidate for laser cooling either. We further propose a practical and efficient laser-cooling scheme for SiH using the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition. The calculated excitation energy is 24299.20 cm⁻¹, which is in perfect agreement with the experimental data (24300.4 cm⁻¹). This allows us accurately estimate the pump and repump wavelengths in laser cooling cycles, which is shown to vary from 400 to 500 nm and are easily accessible in experiment. The Doppler temperature and recoil temperature of SiH for the ${\text{A}}^2{\Delta }_{5/2}$→${\text{X}}^2{\Pi }_{3/2}$ transition are 6.65 and 3.89 μK, respectively. The computed radiative lifetime is 575 ns, and the vibrational branching ratio is highly diagonally distributed with the R₀₀ being 0.9954. Furthermore, we performed additional calculations, and find that, the carbon monosulfide (CS) is a promising candidate which meets the four criteria, and we further propose a suitable laser cooling scheme. We hope that this work will be helpful in searching for promising candidates for producing ultracold molecules.

Data Availability Statement

All datasets generated for this study are available within the article and from the corresponding author on request.

Author Contributions

DL carried out the MRCI calculations. DL, MF, HM, and WB analyzed the data and interpreted the results. ZD and CC provided computing assistance. DL, HM, and WB developed the theoretical scheme and wrote the paper. WB supervised the research and proposed the fourth criterion for molecular laser cooling.

Funding

This work was supported by the National Natural Science Foundation of China (Nos. 21773251, 21973098), the Beijing National Laboratory for Molecular Sciences and Chinese Academy of Sciences.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2020.00020/full#supplementary-material

References

Alekseyev, A. B., Liebermann, H. P., Buenker, R. J., and Hirsch, G. (1996). Ab initio study of the low-lying states of SnH. Mol. Phys. 88, 591–603. doi: 10.1080/00268979609482439

CrossRef Full Text | Google Scholar

Baron, J., Campbell, W. C., Demille, D., Doyle, J. M., Gabrielse, G., Gurevich, Y. V., et al. (2014). Order of magnitude smaller limit on the electric dipole moment of the electron. Science 343, 269–272. doi: 10.1126/science.1248213

PubMed Abstract | CrossRef Full Text | Google Scholar

Bauer, W., Becker, K. H., Düren, R., Hubrich, C., and Meuser, R. (1984). Radiative lifetime measurements of SiH (A²Δ) by laser-induced fluorescence. Chem. Phys. Lett. 108, 560–561. doi: 10.1016/0009-2614(84)85054-X

CrossRef Full Text | Google Scholar

Bauer, W., Engelhardt, B., Wiesen, P., and Becker, K. H. (1989). Lifetime measurements of GeH and CH in the A²Δ, ν′ = 0 state by laser induced fluorescence. Chem. Phys. Lett. 158, 321–324. doi: 10.1016/0009-2614(89)87344-0

CrossRef Full Text | Google Scholar

Betrencourt, M., Boudjaadar, D., Chollet, P., Guelachvili, G., and Morillon-Chapey, M. (1986). Infrared emission spectrum of the radical ²⁸SiH: observation and analysis of the rovibrational bands 1−0, 2−1, and 3−2 in the X²Π ground state. J. Chem. Phys. 84, 4121–4126. doi: 10.1063/1.450081

CrossRef Full Text | Google Scholar

Bollmark, P., Klynning, L., and Pages, P. (1971). Rotational analysis of the 3250 Å bands of SiH and SiD. Phys. Scripta 185, 219–222. doi: 10.1088/0031-8949/3/5/004

CrossRef Full Text | Google Scholar

Bruna, P. J., and Grein, F. (2001). Ab initio study of the X³Σ⁻, a¹Δ, b¹Σ⁺ states of GeH⁻, and electron affinity of GeH. J. Mol. Spectrosc. 599, 261–269. doi: 10.1016/S0022-2860(01)00829-8

CrossRef Full Text | Google Scholar

Cade, P. E., and Huo, W. M. (1967). Electronic structure of diatomic molecules. VI.A. Hartree-Fock wavefunctions and energy quantities for the ground states of the second-row hydrides, AH. J. Chem. Phys. 47, 614–648. doi: 10.1063/1.1711938

CrossRef Full Text | Google Scholar

Cao, J., Li, F., Xia, W., and Bian, W. (2019). van der Waals interactions in bimolecular reactions. Chinese J. Chem. Phys. 32, 157–166. doi: 10.1063/1674-0068/cjcp1901007

CrossRef Full Text | Google Scholar

Carr, L., Demille, D., Krems, R., and Ye, J. (2009). Cold and ultracold molecules: science, technology and applications. New J. Phys. 11:055049. doi: 10.1088/1367-2630/11/5/055049

CrossRef Full Text | Google Scholar

Chapman, D. A., Li, J., Balasubramanian, K., and Lin, S. H. (1988). Theoretical study of electric dipole and transition moments of GeH, SnH and PbH. J. Chem. Phys. 88, 3826–3833. doi: 10.1063/1.453883

CrossRef Full Text | Google Scholar

Di Rosa, M. D. (2004). Laser-cooling molecules: concept, candidates, and supporting hyperfine-resolved measurements of rotational lines in the A-X(0,0) band of CaH. Eur. Phys. J. D 31, 395–402. doi: 10.1140/epjd/e2004-00167-2

CrossRef Full Text | Google Scholar

Erman, P., Gustafsson, O., and Larsson, M. (1983). New predissociations in GeH affecting all the A²Δ state levels. Phys. Scripta 27, 256–260. doi: 10.1088/0031-8949/27/4/006

CrossRef Full Text | Google Scholar

Fu, M., Cao, J., Ma, H., and Bian, W. (2016a). Laser cooling of copper monofluoride: a theoretical study including spin-orbit coupling. RSC Adv. 6, 100568–100576. doi: 10.1039/C6RA07835D

CrossRef Full Text | Google Scholar

Fu, M., Ma, H., Cao, J., and Bian, W. (2016b). Extensive theoretical study on electronically excited states of calcium monochloride: molecular laser cooling and production of ultracold chlorine atoms. J. Chem. Phys. 144:184302. doi: 10.1063/1.4948631

PubMed Abstract | CrossRef Full Text | Google Scholar

Fu, M., Ma, H., Cao, J., and Bian, W. (2017). Laser cooling of CaBr molecules and production of ultracold Br atoms: a theoretical study including spin-orbit coupling. J. Chem. Phys. 146:134309. doi: 10.1063/1.4979566

PubMed Abstract | CrossRef Full Text | Google Scholar

González-Sánchez, L., Gómez-Carrasco, S., Santadaría, A., Wester, R., and Gianturco, F. (2019). Collisional quantum dynamics for MgH⁻ (¹Σ⁺) with He as a buffer gas: ionic state-changing reactions in cold traps. Front. Chem. 7:64. doi: 10.3389/fchem.2019.00064

PubMed Abstract | CrossRef Full Text | Google Scholar

Herzberg, G. (1950). Spectra of Diatomic Molecules, Vol. 235. New York, NY: Van Nostrand Reinhold.

Google Scholar

Huber, K. P., and Herzberg, G. (1979). Molecular Spectra and Molecular Structure IV: Constants of Diatomic Molecules. New York, NY: Van Nostrand Reinhold.

Google Scholar

Hudson, J. J., Kara, D. M., Smallman, I. J., Sauer, B. E., Tarbutt, M. R., and Hinds, E. A. (2011). Improved measurement of the shape of the electron. Nature 473, 493–496. doi: 10.1038/nature10104

PubMed Abstract | CrossRef Full Text | Google Scholar

Hummon, M. T., Yeo, M., Stuhl, B. K., Collopy, A. L., Xia, Y., and Ye, J. (2013). 2D Magneto-optical trapping of diatomic molecules. Phys. Rev. Lett. 110:143001. doi: 10.1103/PhysRevLett.110.143001

PubMed Abstract | CrossRef Full Text | Google Scholar

Jackson, C. V. (1930). The spectrum of silicon hydride. Proc. R. Soc. Lond. Ser. A 126, 373–405. doi: 10.1098/rspa.1930.0015

CrossRef Full Text | Google Scholar

Johnson, R. D. III., and Hudgens, J. W. (1989). New electronic state of silylidyne and silylidyned radicals observed by resonance-enhance multiphoton ionization spectroscopy. J. Phys. Chem. 93, 6268–6270. doi: 10.1021/j100354a003

CrossRef Full Text | Google Scholar

Kalemos, A., Mavridis, A., and Metropoulos, A. (2002). An accurate description of the ground and excited states of SiH. J. Chem. Phys. 116, 6529–6540. doi: 10.1063/1.1461817

CrossRef Full Text | Google Scholar

Kleman, B., and Werhagen, E. (1953a). A²Δ→X²Π system in germanium hydride (GeH). Arkiv Fysik 6:359.

Google Scholar

Kleman, B., and Werhagen, E. (1953b). A⁴Σ⁻→X²Π system in germanium hydride (GeH). Arkiv Fysik 6:399.

Google Scholar

Klynning, L., and Lindgren, B. (1966). Rotational analysis of the ²Δ→²Π band system of germanium hydride and of deuteride germanium. Arkiv Fysik 32:575.

Google Scholar

Lane, I. C. (2015). Production of ultracold hydrogen and deuterium production via Doppler-cooled Feshbach molecules. Phys. Rev. A 92:022511. doi: 10.1103/PhysRevA.92.022511

CrossRef Full Text | Google Scholar

Langhoff, S. R., and Davidson, E. R. (1974). Configuration interaction calculations on the nitrogen molecule. Int. J. Quant. Chem. 8, 61–72. doi: 10.1002/qua.560080106

CrossRef Full Text | Google Scholar

Le Roy, R. J. (2007). LEVEL 8.0: A Computer Program for Solving the Radial Schrödinger Equation for Bound and Quasibound Levels, Chemical Physics Research Report CPRR-663. University of Waterloo. Available online at: http://leroy.uwaterloo.ca

Google Scholar

Lewerenz, M., Bruna, P. J., Peyerimhoff, S. D., and Buenker, R. J. (1983). Ab initio MRD-CI study of the electronic spectrum of SiH. Mol. Phys. 49, 1–24. doi: 10.1080/00268978300101001

CrossRef Full Text | Google Scholar

Li, G., Gao, T., and Zhang, Y. (2008). The splitting of low-lying or low excited states for hydride molecules (cations) of the third period under spin-orbit coupling. Chinese Phys. B 17, 2040–2047. doi: 10.1088/1674-1056/17/6/018

CrossRef Full Text | Google Scholar

Li, R., Zhai, Z., Zhang, X., Jin, M., Xu, H., and Yan, B. (2015). All-electron spin-orbit configuration interaction study on the valence and low-lying Rydberg electronic states of GeH. J. Quant. Spectrosc. Radiat. Transf. 157, 42–53. doi: 10.1016/j.jqsrt.2015.02.009

CrossRef Full Text | Google Scholar

Liu, C., Zhang, D., and Bian, W. (2003). Theoretical investigation of the reaction of Co⁺ with OCS. J. Phys. Chem. A 107, 8618–8622. doi: 10.1021/jp034693s

CrossRef Full Text | Google Scholar

Liu, K., Yu, L., and Bian, W. (2009). Extensive theoretical study on various low-lying electronic states of silicon monochloride cation including spin-orbit coupling. J. Phys. Chem. A 113:1678. doi: 10.1021/jp809618y

PubMed Abstract | CrossRef Full Text | Google Scholar

Perrin, J., and Delafosse, E. (1980). Emission spectroscopy of SiH in a silane glow-discharge. J. Phys. D Appl. Phys. 13:759. doi: 10.1088/0022-3727/13/5/009

CrossRef Full Text | Google Scholar

Ram, R. S., Engleman, R., and Bernath, P. F. (1998). Fourier transform emission spectroscopy of the A²Δ→X²Π transition of SiH and SiD. J. Mol. Spectrosc. 190, 341–352. doi: 10.1006/jmsp.1998.7582

PubMed Abstract | CrossRef Full Text | Google Scholar

Setzer, K. D., Borkowska-Burnecka, J., Zyrnicki, W., and Fink, E. H. (2008). High-resolution fourier-transform study of the ${\text{X}}_2{}^2{\Pi }_{3/2}\to {\text{X}}_1{}^2{\Pi }_{1/2}$ fine structure transitions of PbH and PbD. J. Mol. Spectrosc. 252, 176–184. doi: 10.1016/j.jms.2008.08.003

CrossRef Full Text | Google Scholar

Shen, Z., Ma, H., Zhang, C., Fu, M., Wu, Y., Bian, W., et al. (2017). Dynamical importance of van der Waals saddle and excited potential surface in C(¹D)+D₂ complex-forming reaction. Nat. Commun. 8:14094. doi: 10.1038/ncomms14094

PubMed Abstract | CrossRef Full Text | Google Scholar

Shi, D., Li, P., Zhu, Z., and Sun, J. (2013). Spin-orbit coupling splitting in the X²Π, A²Δ, B²Σ⁻, C²Σ⁺, D²Σ⁺, F²Π and a⁴Σ⁻ Λ-S states of SiH radical. Spectrochim. Acta Part A 115, 259–268. doi: 10.1016/j.saa.2013.06.038

CrossRef Full Text | Google Scholar

Shi, D., Zhang, J., Sun, J., Zhu, Z., Yu, B., and Liu, Y. (2008). Investigations on spectroscopic properties of SiH (X²Π) radical using coupled-cluster theory in combination with the correlation-consistent quintuple basis set. J. Mol. Struct. Theochem 851, 30–34. doi: 10.1016/j.theochem.2007.10.033

CrossRef Full Text | Google Scholar

Shuman, E. S., Barry, J. F., and Demille, D. (2010). Laser cooling of a diatomic molecule. Nature 467, 820–823. doi: 10.1038/nature09443

PubMed Abstract | CrossRef Full Text | Google Scholar

Simah, D., Hartke, B., and Werner, H. J. (1999). Photodissociation dynamics of H₂S on new coupled ab initio potential energy surfaces. J. Chem. Phys. 111, 4523–4534. doi: 10.1063/1.479214

CrossRef Full Text | Google Scholar

Smith, W. H. (1969). Lifetimes and total transition probabilities for NH, SiH, and SiD. J. Chem. Phys. 51, 520–524. doi: 10.1063/1.1672027

CrossRef Full Text | Google Scholar

Smith, W. H., and Liszt, H. S. (1971). Franck-Condon factors and absolute oscillator strengths for NH, SiH, S₂ and SO. J. Quant. Spectrosc. Radiat. Transf. 11, 45–54. doi: 10.1016/0022-4073(71)90160-9

CrossRef Full Text | Google Scholar

Towle, J. P., and Brown, J. M. (1993). The infrared spectrum of the GeH radical. Mol. Phys. 79, 249–261. doi: 10.1080/00268979300100211

CrossRef Full Text | Google Scholar

Wang, S., Wang, Q., Lin, Y., Wang, M., Lin, B., Zang, E., et al. (2009). Cooling and trapping ⁸⁸Sr atoms with 461 nm laser. Chinese Phys. Lett. 26:093202. doi: 10.1088/0256-307X/26/9/093202

CrossRef Full Text | Google Scholar

Watson, W. W. (1938). The spectrum of lead hydride. Phys. Rev. 54, 639–642. doi: 10.1103/PhysRev.53.639

CrossRef Full Text | Google Scholar

Wells, N., and Lane, I. C. (2011a). Electronic states and spin-forbidden cooling transitions of AlH and AlF. Phys. Chem. Chem. Phys. 13, 19018–19025. doi: 10.1039/c1cp21313j

PubMed Abstract | CrossRef Full Text | Google Scholar

Wells, N., and Lane, I. C. (2011b). Prospects for ultracold carbon via charge exchange reactions and laser cooled carbides. Phys. Chem. Chem. Phys. 13, 19036–19051. doi: 10.1039/c1cp21304k

PubMed Abstract | CrossRef Full Text | Google Scholar

Werner, H.-J., and Knowles, P. J. (1985). A second order multiconfiguration SCF procedure with optimum convergence. J. Chem. Phys. 82, 5053–5063. doi: 10.1063/1.448627

CrossRef Full Text | Google Scholar

Werner, H.-J., Knowles, P. J., Lindh, R., Manby, F. R., Schütz, M., Celani, P., et al. (2012). Molpro, Version 2012.1, A Package of Ab Initio Programs. Available online at: http://www.molpro.net

Google Scholar

Wu, Y., Cao, J., Ma, H., Zhang, C., Bian, W., Nunez-Reyes, D., et al. (2019). Conical intersection–regulated intermediates in bimolecular reactions: insights from C(¹D) + HD dynamics. Sci. Adv. 5:eaaw0446. doi: 10.1126/sciadv.aaw0446

PubMed Abstract | CrossRef Full Text | Google Scholar

Xia, W., Fu, M., Ma, H., and Bian, W. (2017). A theoretical study on laser cooling of silicon monofluoride. Chem. Phys. 485–486, 29–34. doi: 10.1016/j.chemphys.2017.01.007

CrossRef Full Text | Google Scholar

Xu, S., Xia, M., Yin, Y., Gu, R., Xia, Y., and Yin, J. (2019). Determination of the normal A²Π state in MgF with application to direct laser cooling of molecules. J. Chem. Phys. 150:084302. doi: 10.1063/1.5083898

PubMed Abstract | CrossRef Full Text | Google Scholar

Yan, B., Moses, S. A., Gadway, B., Covey, J. P., Hazzard, K. R. A., Rey, A. M., et al. (2013). Observation of dipolar spin-exchange interactions with lattice-confined polar molecules. Nature 501, 521–525. doi: 10.1038/nature12483

PubMed Abstract | CrossRef Full Text | Google Scholar

You, Y., Yang, C. L., Zhang, Q. Q., Wang, M. S., Ma, X. G., and Liu, W. W. (2016). Ab initio studies on the spin-forbidden cooling transitions of the LiRb molecule. Phys. Chem. Chem. Phys. 18:19838. doi: 10.1039/C6CP01618A

PubMed Abstract | CrossRef Full Text | Google Scholar

Yu, L., and Bian, W. (2011). Extensive theoretical study on electronically excited states and predissociation mechanisms of sulfur monoxide including spin-orbit coupling. J. Comput. Chem. 32, 1577–1588. doi: 10.1002/jcc.21737

PubMed Abstract | CrossRef Full Text | Google Scholar

Yu, L., and Bian, W. (2012). Electronically excited-state properties and predissociation mechanisms of phosphorus monofluoride: a theoretical study including spin-orbit coupling. J. Chem. Phys. 137:014313. doi: 10.1063/1.4731635

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhang, Y., Dou, G., Qi, J., and Cui, J. (2018). Ab inito study on the electronic structure and laser cooling of SiH. Comput. Theor. Chem. 1134, 8–14. doi: 10.1016/j.comptc.2018.04.014

CrossRef Full Text | Google Scholar

Zhao, H., Bian, W., and Liu, K. (2006). A theoretical study of the reaction of O(³P) with isobutene. J. Phys. Chem. A 110, 7858–7866. doi: 10.1021/jp060583k

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, S., Li, R., Zhang, H., and Li, H. (2017). MRCI+Q calculations on spectroscopic properties of excited states of PbH including spin-orbit coupling. Chem. Phys. Lett. 671, 92–99. doi: 10.1016/j.cplett.2017.01.016

CrossRef Full Text | Google Scholar

Zhelyazkova, V., Cournol, A., Wall, T. E., Matsushima, A., Hudson, J. J., Hinds, E. A., et al. (2014). Laser cooling and slowing of CaF molecules. Phys. Rev. A 89, 12707–12715. doi: 10.1103/PhysRevA.89.053416

CrossRef Full Text | Google Scholar